算法学习系列（十）：用数组模拟链表、双链表、栈、队列、单调栈、单调队列

引言

首先说一下为什么要拿数组来模拟，最主要的原因是为了快，因为如果用stl库里的容器的话，在算法竞赛中，一般是不会给你开O2优化或者臭氧优化的，然后所以在一些时间卡的非常紧的情况下，还是推荐用数组来模拟会比较好，然后开始吧。

一、数组模拟链表

1.模板

const int N = 100010;

//head为头节点所在的下标,e[i]代表下标为i的值，ne[i]代表下标为i的下一个下标,idx代表当前可用的下标
int head, e[N], ne[N], idx;

void init()
{
    head = -1;
    idx = 0;
}

void add_to_head(int x)
{
    e[idx] = x;
    ne[idx] = head, head = idx++;
}

void add(int k, int x)  //插到下标为k的后面
{
    e[idx] = x;
    ne[idx] = ne[k], ne[k] = idx++;
}

void remove(int k)  //删掉下标为k的后一个元素
{
    ne[k] = ne[ne[k]];
}

2.例题

实现一个单链表，链表初始为空，支持三种操作：

向链表头插入一个数；
删除第 k 个插入的数后面的数；
在第 k 个插入的数后插入一个数。

现在要对该链表进行 M 次操作，进行完所有操作后，从头到尾输出整个链表。注意:题目中第 k 个插入的数并不是指当前链表的第 k 个数。例如操作过程中一共插入了 n 个数，则按照插入的时间顺序，这 n 个数依次为：第 1 个插入的数，第 2 个插入的数，…第 n 个插入的数。

输入格式
第一行包含整数 M，表示操作次数。接下来 M 行，每行包含一个操作命令，操作命令可能为以下几种：H x，表示向链表头插入一个数 x。D k，表示删除第 k 个插入的数后面的数（当 k 为 0 时，表示删除头结点）。I k x，表示在第 k 个插入的数后面插入一个数 x（此操作中 k 均大于 0）。

输出格式
共一行，将整个链表从头到尾输出。数据范围1≤M≤100000所有操作保证合法。

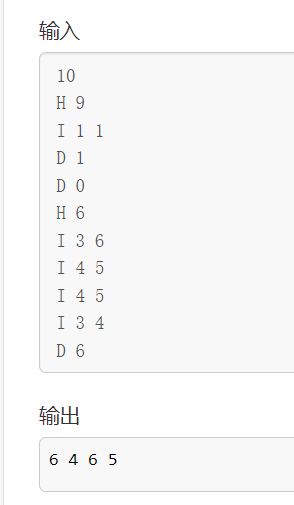
输入样例：
10
H 9
I 1 1
D 1
D 0
H 6
I 3 6
I 4 5
I 4 5
I 3 4
D 6

输出样例：
6 4 6 5

#include 

using namespace std;

const int N = 100010;

int m;
int head, e[N], ne[N], idx;

void init()
{
    head = -1;
    idx = 0;
}

void add_to_head(int x)
{
    e[idx] = x;
    ne[idx] = head, head = idx++;
}

void add(int k, int x)  //插到下标为k的后面
{
    e[idx] = x;
    ne[idx] = ne[k], ne[k] = idx++;
}

void remove(int k)  //删掉下标为k的后一个元素
{
    ne[k] = ne[ne[k]];
}

int main()
{
    init();
    cin >> m;
    
    while(m--)
    {
        int k, x;
        char op;
        
        cin >> op;
        if(op == 'H')
        {
            cin >> x;
            add_to_head(x);
        }
        else if(op == 'D')
        {
            cin >> k;
            if(!k) head = ne[head]; 
            else remove(k-1);
        }
        else
        {
            cin >> k >> x;
            add(k-1, x);
        }
    }
    
    for(int i = head; i != -1; i = ne[i]) cout << e[i] << " ";
    cout << endl;
    
    return 0;
}

3.测试

可以看出是正确的

二、数组模拟双链表

1.模板

const int N = 100010;

int e[N], l[N], r[N], idx;

void init()  //0代表左端点 1代表右端点
{
    r[0] = 1, l[1] = 0, idx = 2;  
}

void insert(int k, int x)  //在结点k的右边插入一个数x
{
    e[idx] = x;
    r[idx] = r[k], l[idx] = k;
    l[r[k]] = idx, r[k] = idx++;
}

void remove(int k)  //删除结点k
{
    r[l[k]] = r[k];
    l[r[k]] = l[k];
}

2.例题

实现一个双链表，双链表初始为空，支持 5 种操作：

在最左侧插入一个数；
在最右侧插入一个数；
将第 k 个插入的数删除；
在第 k 个插入的数左侧插入一个数；
在第 k 个插入的数右侧插入一个数

现在要对该链表进行 M 次操作，进行完所有操作后，从左到右输出整个链表。

注意:题目中第 k 个插入的数并不是指当前链表的第 k 个数。例如操作过程中一共插入了 n 个数，则按照插入的时间顺序，这 n 个数依次为：第 1 个插入的数，第 2 个插入的数，…第 n 个插入的数。

输入格式
第一行包含整数 M，表示操作次数。接下来 M 行，每行包含一个操作命令，操作命令可能为以下几种：L x，表示在链表的最左端插入数 x。R x，表示在链表的最右端插入数 x。D k，表示将第 k 个插入的数删除。IL k x，表示在第 k 个插入的数左侧插入一个数。IR k x，表示在第 k 个插入的数右侧插入一个数。

输出格式
共一行，将整个链表从左到右输出。数据范围1≤M≤100000所有操作保证合法。

输入样例：
10
R 7
D 1
L 3
IL 2 10
D 3
IL 2 7
L 8
R 9
IL 4 7
IR 2 2

输出样例：
8 7 7 3 2 9

#include 

using namespace std;

const int N = 100010;

int e[N], l[N], r[N], idx;

void init()  //0代表左端点 1代表右端点
{
    r[0] = 1, l[1] = 0, idx = 2;  
}

void insert(int k, int x)  //在结点k的右边插入一个数x
{
    e[idx] = x;
    r[idx] = r[k], l[idx] = k;
    l[r[k]] = idx, r[k] = idx++;
}

void remove(int k)  //删除结点k
{
    r[l[k]] = r[k];
    l[r[k]] = l[k];
}

int main()
{
    init();
    
    int m;
    cin >> m;
    
    while(m--)
    {
        int k, x;
        string op;
        
        cin >> op;
        if(op == "L")
        {
            cin >> x;
            insert(0,x);
        }
        else if(op == "R")
        {
            cin >> x;
            insert(l[1],x);
        }
        else if(op == "D")
        {
            cin >> k;
            remove(k+1);  //因为k是从2开始的
        }
        else if(op == "IL")
        {
            cin >> k >> x;
            insert(l[k+1],x);
        }
        else
        {
            cin >> k >> x;
            insert(k+1,x);
        }
    }
    
    for(int i = r[0]; i != 1; i = r[i]) cout << e[i] << " ";
    
    return 0;
}

3.测试

可以看出是正确的

三、数组模拟栈

1.模板

const int N = 100010;

int stk[N], tt;

void push(int x)
{
    stk[++tt] = x;
}

bool empty()
{
    return tt > 0 ? false : true;
}

void pop()
{
    tt--;
}

int top()
{
   return stk[tt]; 
}

2.例题

实现一个栈，栈初始为空，支持四种操作：

push x – 向栈顶插入一个数 x；
pop – 从栈顶弹出一个数；
empty – 判断栈是否为空；
query – 查询栈顶元素。

现在要对栈进行 M 个操作，其中的每个操作 3 和操作 4 都要输出相应的结果。

输入格式
第一行包含整数 M，表示操作次数。接下来 M 行，每行包含一个操作命令，操作命令为 push x，pop，empty，query 中的一种。

输出格式
对于每个 empty 和 query 操作都要输出一个查询结果，每个结果占一行。其中，empty 操作的查询结果为 YES 或 NO，query 操作的查询结果为一个整数，表示栈顶元素的值。

数据范围
1≤M≤100000,1≤x≤109所有操作保证合法。

输入样例：
10
push 5
query
push 6
pop
query
pop
empty
push 4
query
empty

输出样例：
5
5
YES
4
NO

#include 

using namespace std;

const int N = 100010;

int stk[N], tt;

void push(int x)
{
    stk[++tt] = x;
}

bool empty()
{
    return tt > 0 ? false : true;
}

void pop()
{
    tt--;
}

int top()
{
   return stk[tt]; 
}

int main()
{
    int m;
    cin >> m;
    
    while(m--)
    {
        string op;
        int x;
        
        cin >> op;
        if(op == "push")
        {
            cin >> x;
            push(x);
        }
        else if(op == "pop")
        {
            pop();
        }
        else if(op == "empty")
        {
            if(empty()) cout << "YES" << endl;
            else cout << "NO" << endl;
        }
        else
        {
            cout << top() << endl;
        }
    }
    
    return 0;
}

3.测试

四、数组模拟队列

1.模板

const int N = 100010;

int q[N], hh, tt = -1;

void push(int x)
{
    q[++tt] = x;
}

int front()
{
   return q[hh]; 
}

void pop()
{
    hh++;
}

bool empty()
{
    return hh <= tt ? false : true;
}

2.例题

实现一个队列，队列初始为空，支持四种操作：

push x – 向队尾插入一个数 x；
pop – 从队头弹出一个数；
empty – 判断队列是否为空；
query – 查询队头元素。

现在要对队列进行 M 个操作，其中的每个操作 3 和操作 4 都要输出相应的结果。

输入格式
第一行包含整数 M，表示操作次数。接下来 M 行，每行包含一个操作命令，操作命令为 push x，pop，empty，query 中的一种。

输出格式
对于每个 empty 和 query 操作都要输出一个查询结果，每个结果占一行。其中，empty 操作的查询结果为 YES 或 NO，query 操作的查询结果为一个整数，表示队头元素的值。

数据范围
1≤M≤100000,1≤x≤109,所有操作保证合法。

输入样例：
10
push 6
empty
query
pop
empty
push 3
push 4
pop
query
push 6

输出样例：
NO
6
YES
4

#include 

using namespace std;

const int N = 100010;

int q[N], hh, tt = -1;

void push(int x)
{
    q[++tt] = x;
}

int front()
{
   return q[hh]; 
}

void pop()
{
    hh++;
}

bool empty()
{
    return hh <= tt ? false : true;
}

int main()
{
    int m;
    cin >> m;
    
    while(m--)
    {
        int x;
        string op;
        cin >> op;
        
        if(op == "push")
        {
            cin >> x;
            push(x);
        }
        else if(op == "pop")
        {
            pop();
        }
        else if(op == "empty")
        {
            if(empty()) cout << "YES" << endl;
            else cout << "NO" << endl;
        }
        else 
        {
            cout << front() << endl;
        }
    }
    
    return 0;
}

3.测试

五、数组模拟单调栈

单调栈就是用来快速找当前下标左边第一个小于该数的数

1.例题+模板

给定一个长度为 N 的整数数列，输出每个数左边第一个比它小的数，如果不存在则输出 −1。

输入格式
第一行包含整数 N，表示数列长度。第二行包含 N 个整数，表示整数数列。

输出格式
共一行，包含 N 个整数，其中第 i 个数表示第 i 个数的左边第一个比它小的数，如果不存在则输出 −1。

数据范围
1≤N≤105，1≤数列中元素≤109

输入样例：
5
3 4 2 7 5

输出样例：
-1 3 -1 2 2

#include 

using namespace std;

const int N = 100010;

int stk[N], tt;

int main()
{
    int n;
    cin >> n;
    
    while(n--)
    {
        int x;
        cin >> x;
        
        while(tt && stk[tt] >= x) tt--;
        if(tt) printf("%d ", stk[tt]);
        else printf("-1 ");
        stk[++tt] = x;
    }
    
    return 0;
}

2.测试

六、数组模拟单调队列

1.例题+模板

给定一个大小为 n≤106的数组。有一个大小为 k 的滑动窗口，它从数组的最左边移动到最右边。你只能在窗口中看到 k 个数字。每次滑动窗口向右移动一个位置。以下是一个例子：
该数组为 [1 3 -1 -3 5 3 6 7]，k 为 3。

     窗口位置	       最小值	最大值
[1 3 -1] -3 5 3 6 7	    -1	      3
1 [3 -1 -3] 5 3 6 7	    -3	      3
1 3 [-1 -3 5] 3 6 7		-3	      5
1 3 -1 [-3 5 3] 6 7		-3	      5
1 3 -1 -3 [5 3 6] 7	     3	      6
1 3 -1 -3 5 [3 6 7]	     3	      7
你的任务是确定滑动窗口位于每个位置时，窗口中的最大值和最小值。

输入格式
输入包含两行。第一行包含两个整数 n 和 k，分别代表数组长度和滑动窗口的长度。第二行有 n 个整数，代表数组的具体数值。同行数据之间用空格隔开。

输出格式
输出包含两个。第一行输出，从左至右，每个位置滑动窗口中的最小值。第二行输出，从左至右，每个位置滑动窗口中的最大值。

输入样例：
8 3
1 3 -1 -3 5 3 6 7

输出样例：
-1 -3 -3 -3 3 3
3 3 5 5 6 7

#include 
#include 

using namespace std;

const int N = 1000010;

int a[N], q[N];
int n, k, hh, tt = -1;

int main()
{
    scanf("%d%d", &n, &k);
    for(int i = 0; i < n; ++i) scanf("%d", &a[i]);
    
    for(int i = 0; i < n; ++i)
    {
        //但因为这个每次只会进一个，所以用if就可以了
        if(hh <= tt && i - k + 1 > q[hh]) hh++;  //因为如果上一次满了，然后上一次再入进去就会导致队头滑出窗口
        while(hh <= tt && a[q[tt]] >= a[i]) tt--;
        
        q[++tt] = i;
        if(i >= k - 1) printf("%d ", a[q[hh]]);
    }
    
    puts("");
    
    hh = 0, tt = -1;
    for(int i = 0; i < n; ++i)
    {
        if(hh <= tt && i - k + 1 > q[hh]) hh++;  //因为如果上一次满了，然后上一次再入进去就会导致队头滑出窗口
        while(hh <= tt && a[q[tt]] <= a[i]) tt--;
        
        q[++tt] = i;
        if(i >= k - 1) printf("%d ", a[q[hh]]);
    }
    
    puts("");
    
    return 0;
}

2.测试