- 信息检索简介——文本处理、搜索引擎、数据挖掘、机器学习、推荐系统等
AI天才研究院
Python实战自然语言处理人工智能语言模型编程实践开发语言架构设计
作者:禅与计算机程序设计艺术1.简介2005年8月17日至9月3日在美国加利福尼亚州伯克莱纳举行了SIGIR国际会议(中文全称“计算机信息retrieval国际会议”),这是信息检索领域的顶级会议之一。该会议由ACM主办,主题涵盖了包括文本处理、搜索引擎、数据挖掘、机器学习、推荐系统等多个热门方向。此次会议是第一次将信息检索作为一个学科,并取得重大突破。本文试图对SIGIR进行一个完整的介绍,阐述
- abp 链接本地mysql_ABP Vnext使用mysql数据库
漫小威
abp链接本地mysql
ABPVnext支持Sqlserver、Mysql、PostgreSql等数据库,通过CLI模板建立的项目默认使用SqlServer,需要进行一定变更才支持其他数据库,下面以使用Mysql举例1.使用CLI建立一个带UI的MVC项目abpnewAcme.BookStoreUi--templateapp--database-provideref--uimvc--mobilenone建立后项目如下2.
- 重温经典第二弹(xdoj1175,xdoj1179)
Owen_Q
搜索暴力枚举字符串
一转眼,记忆又来到了暑假。或许,这是一个这算是自己真正开始接触了解acm的一个时间点吧,各种算法数据结构,开始慢慢浮出水面。回顾当初,感慨万千。又找出了两道未ac之题,确实复杂度明显加强,思维性的进一步考验。Count思路:子串搜索问题,因为n和k大到2e5,因此,肯定是个单向处理不能回溯的问题,否则最坏n方的复杂度是难以接受的。对于单次搜索,考虑可以维护现有区间的元素,然后移位遍历向后搜索,对于
- ACM ICPC 2017 Warmup Contest 7(CTU Open Contest 2016)
Owen_Q
水题搜索数组stlacm
ccsp与区域赛都越来越近了,模拟与区域赛题并进,还有一堆作业,有点累,想玩耍,感觉自己有点迷失,算了,还是就这样吧,努力向前练习赛7,打两个签到题走人,继续刷csp去B.HotAirBallooning思路:统计不同人用过的气球的方案数,又是个去重问题,又想往set上放,后来发现气球数很少,完全可以数组统计,而气球总组合有限,虽然不大,但强搜可能会感觉tle,加个状压好了,感觉现在自己特别喜欢做
- 008 【入门】算法和数据结构简介
要天天开心啊
算法专栏算法数据结构
算法与数据结构系统概览|[算法]-[基础]-[通用]一、算法分类与应用1.硬计算类算法|[算法]-[中级]-[通用]特点应用场景复杂度特征-精确求解问题-可能带来较高计算复杂度-大厂笔试/面试-ACM竞赛-所有程序员岗位必考⏱️通常为O(n)~O(n²)//[示例]快速排序算法-分治思想核心实现publicvoidquickSort(int[]arr,intleft,intright){if(le
- 【Linux】ghb工具
nanguochenchuan
Linux操作系统linux运维服务器
GDB简介GDB(GNUDebugger)是Linux系统中最强大的命令行调试工具,由GNU项目开发。作为程序员调试C/C++程序的利器,GDB能帮助你:定位程序崩溃原因分析程序运行状态跟踪变量值变化检测内存错误安装与配置安装方法#Ubuntu/Debiansudoaptinstallgdb#CentOS/RHELsudoyuminstallgdb#ArchLinuxsudopacman-Sgdb
- 中国计算机学会推荐国际学术会议-体系结构相关(含投稿截至时间)
HiAallen
事务编辑器
Ref:CCF推荐国际学术刊物目录-中国计算机学会中国计算机学会推荐国际学术会议(●计算机体系结构/并行与分布计算/存储系统)A类序号刊物名称刊物全称出版社投稿截止时间地址1PPoPPACMSIGPLANSymposiumonPrinciples&PracticeofParallelProgrammingACM2022-8-17dblp:PPOPP2FASTConferenceonFileandS
- 2019 CCF 推荐国际学术期刊&会议(计算机体系结构/并行与分布计算/存储系统)
漓艾初
CCF
中国计算机学会推荐国际学术期刊&会议直接去这里找,全部都有https://www.ccf.org.cn/Academic_Evaluation/By_category/计算机体系结构/并行与分布计算/存储系统期刊A类序号刊物简称刊物全称出版社网址1TOCSACMTransactionsonComputerSystemsACMhttp://dblp.uni-trier.de/db/journals/
- manjaro安装微软雅黑字体_开始使用 Manjaro(添加源+字体渲染去模糊+软件安装+优化配置+常见错误)(30)...
真的是单大宝
manjaro安装微软雅黑字体
1.添加archlinux镜像源1.步骤一向/etc/pacman.d/mirrorlist中添加国内镜像地址1.1方法1:自动添加1、输入如下命令查看国内镜像源,并按质量排序:sudopacman-mirrors-i-cChina-mrank,之后会弹出一个窗口,可以选择想要的镜像源,选择确定后会自动导入/etc/pacman.d/mirrorlist配置文件中。1.2方法2:手动添加直接在et
- iOS App 上架流程工具链解析:开发者视角下的协作实践总结
2501_91591841
httpudphttpswebsocket网络安全网络协议tcp/ip
在我们最近完成的一个B2C健康管理类App项目中,有一个显著的特点:开发团队并不拥有统一的macOS环境。我们使用Flutter开发,一部分成员使用Windows,一部分使用Ubuntu,团队中仅有一台远程可用的Macmini作为打包主机。这次项目的iOS上架过程从准备证书、打包构建、上传提交,到信息维护与测试,每一个步骤都涉及多个工具协作。本文是从一个工程师的日常视角,拆解我们如何组合各类工具完
- Ubuntu22.04安装CH343驱动并创建udev规则
驱动说明Linux系统提供CH34*系列USBUART设备配合使用的默认CDC-ACM驱动程序。驱动程序文件名为CDC-ACM。CDC-ACM驱动程序控制特定设备的能力有限。此通用驱动程序不了解特定设备协议。因此,设备制造商可以创建能够访问设备特定功能集(例如硬件流控制或GPIO功能)的替代或自定义驱动程序。驱动文件下载:https://github.com/WCHSoftGroup/ch343s
- Spring Boot集成RabbitMQ的使用
码海浮生
后端Java技术类java-rabbitmqspringbootrabbitmq
作者:知识浅谈,CSDN签约讲师,CSDN博客专家,华为云云享专家,阿里云专家博主擅长领域:全栈工程师、爬虫、ACM算法微信:zsqtcyw联系我领取学习资料SpringBoot集成RabbitMQ的使用引言引入依赖配置RabbitMQ交换机、队列和绑定声明交换机和队列发送消息接收消息消息类型消息确认发送确认消费确认消息序列化监控与管理注意事项总结引言RabbitMQ是一个开源的消息代理和队列服务
- ACM题目和培养训练!!!
wretchedme
算法codecacm
ACM大量习题题库ACM大量习题题库现在网上有许多题库,大多是可以在线评测,所以叫做OnlineJudge。除了USACO是为IOI准备外,其余几乎全部是大学的ACM竞赛题库。USACOhttp://ace.delos.com/usacogate美国著名在线题库,专门为信息学竞赛选手准备TJUhttp://acm.tongji.edu.cn/同济大学在线题库,唯一的中文题库,适合NOIP选手ZJU
- 【node】Mac m1 安装nvm 和node
夜雨hiyeyu.com
vuemacoschrome前端vuevue.jsnvmjava
Macm1安装nvm和node一、使用brew安装nvm安装brewhome二、建立.nvm文件夹三、~/.zshrc或~/.bash_profile中配置如下四、nvm常用命令一、使用brew安装nvm安装brewhome安装国内版本/bin/bash-c"$(curl-fsSLhttps://gitee.com/cunkai/HomebrewCN/raw/master/Homebrew.sh)
- 2012 - 正方形矩阵
寒燕舞
算法数据结构
题目描述晶晶同学非常喜欢方形,她希望打印出来的字符串也是方形的。老师给了晶晶同学一个字符串"ACM",晶晶同学突发奇想,如果任意给定义一个整数n,能不能打印出由这个字符串组成的正方形字符串呢?要求是每行要使用n个给定的字符串。请你编程实现一下。输入输入的第一行是一个正整数N(Nusingnamespacestd;intmain(){intn,m;cin>>n;for(intx=0;x>m;for(
- 【Tools】Mac brew工具
CodeWithMe
Toolsmacos
Homebrew(简称brew)是macOS(也支持Linux)上的一款包管理工具,它的作用类似于:Ubuntu下的aptCentOS下的yumArchLinux下的pacman一句话概括:brew是用来在macOS上安装、管理软件包的命令行工具。brew能做什么?安装CLI工具(如wget,git,cmake,python,node,ffmpeg等)安装GUI应用(如VisualStudioCo
- jxnu acm新生选拔赛
weixin_30474613
c/c++
最小的数ProblemDescription定义一种正整数集合K,集合中有N个数,集合中元素Ki(12#include3#include4#include5#include6#definelllonglong7usingnamespacestd;8constintMAX=1e4+10;9constintMOD=1e9+7;10intvis[10010],b[MAX*10],k;11voidinit
- [论文阅读] 人工智能+软件工程 | 用大语言模型架起软件需求形式化的桥梁
张较瘦_
前沿技术人工智能论文阅读软件工程
用大语言模型架起软件需求形式化的桥梁:一篇ACM调查草案的深度解读论文信息arXiv:2506.14627ACMSurveyDraftonFormalisingSoftwareRequirementswithLargeLanguageModelsArshadBeg,DiarmuidO’Donoghue,RosemaryMonahanComments:22pages.6summarytablesSu
- C++基础练习-二维数组
s15335
C++练习题c++开发语言
题目:https://acm.hdu.edu.cn/showproblem.php?pid=2022题解:#includeusingnamespacestd;intz[10000][10000];intmain(){intm,n;while(cin>>m>>n){intx,max=-1,l,c;//往数组里添加数据for(inti=0;i>z[i][j];}}//遍历数组并找出最大值for(int
- 数据结构-顺序表-数值统计
题目:https://acm.hdu.edu.cn/showproblem.php?pid=2008解答:#includeusingnamespacestd;#defineSLDataTypedoublestructSequlist{SLDataType*array;intsize;intcapacity;};//********************顺序表初始化***********/void
- 算法导论第十八章 计算几何:算法中的空间艺术
第十八章计算几何:算法中的空间艺术“几何学是描绘宇宙秩序的永恒诗篇。”——约翰内斯·开普勒计算几何将数学的优雅与算法的实用性完美结合,在计算机图形学、机器人导航和地理信息系统中扮演着关键角色。本章将带您探索几何问题的算法解决方案,从基础的点线关系到复杂的空间剖分,揭示算法如何理解和操纵我们的几何世界。18.1几何基础:点、线和多边形18.1.1几何对象的表示在计算几何中,我们使用简洁的数学结构表示
- DELL R730XD服务器调整风扇转速
zz960226
服务器运维
注意:进入iDRAC的Web管理界面,左侧iDRAC设置->网络->IPMI设置,勾选启用LAN上的IPMI。使用ipmitool调整,服务器电源断开后就会失效,如果想要永久生效,就在服务器端写一个开机自启动脚本。先关闭风扇自动调速功能,否则手动设置的转速不会生效的。命令末尾的0x00表示关闭自动调速,0x01表示开启自动调速。linux脚本自动执行版安装ipmitoolpacman-Sipmit
- MFC绘制Bezier曲线
老土豆FUSK
计算几何算法与实现
MFC绘制Bezier曲线参考《计算几何算法与实现》–孔令德绘制的Bezier曲线次数为3,四个控制节点1、添加二维点类#pragmaonce//为了避免按照x和y方向进行重复运算,重载运算对象classCP2{public:CP2(void);~CP2(void);CP2(doublex,doubley);friendCP2operator+(constCP2&p0,constCP2&p1);/
- SpringBoot响应式编程 WebFlux入门教程
码海浮生
Java后端技术类springboot后端java
作者:知识浅谈,CSDN签约讲师,CSDN博客专家,华为云云享专家,阿里云专家博主擅长领域:全栈工程师、爬虫、ACM算法微信:zsqtcyw联系我领取学习资料SpringBoot响应式编程WebFlux入门教程概述快速入门关键概念配置细节测试方法总结概述SpringBoot响应式编程的核心框架之一是WebFlux,它是专为反应式编程设计的Web框架。与传统的SpringMVC相比,WebFlux具
- 【美团笔试题汇总】2024-04-06-美团春秋招笔试题-三语言题解(CPP/Python/Java)
春秋招笔试突围
团子春秋招笔试题汇总pythonjava开发语言互联网大厂笔试题算法美团笔试题汇总算法笔试题
大家好这里是KK爱Coding,一枚热爱算法的程序员✨本系列打算持续跟新美团近期的春秋招笔试题汇总~ACM银牌|多次AK大厂笔试|编程一对一辅导感谢大家的订阅➕和喜欢KK这边最近正在收集近一年互联网各厂的笔试题汇总,如果有需要的小伙伴可以关注后私信一下KK领取,会在飞书进行同步的跟新。文章目录01.最小修改次数题目描述输入格式输出格式样例输入样例输出数据范围题解参考代码02.K小姐的复数统计问题描
- Joomla T3扩展实战指南:构建高效网站框架
基鑫阁
本文还有配套的精品资源,点击获取简介:JoomlaT3扩展是一个为JoomlaCMS打造的流行框架,提供模块化设计、响应式布局和跨设备兼容性。它集成了Bootstrap前端框架,拥有强大的主题定制、SEO优化、性能提升和插件支持功能。T3框架特别注重用户体验和SEO表现,提供了丰富的CSS和JavaScript定制选项以及多语言功能。它还拥有一支活跃的开发团队和社区支持,定期发布更新来增强框架功能
- ReactNative 适配XCode打包ios18+
Kevin·Tseng
reactnativexcodereact.jsjavascriptecmascript
背景ios18使用环境macOS15.2+xcode16机子使用maxOS14.5(Macmini2018)+xcode15.4当执行打包操作时,报错如下:yarnrelease--appstore...2025-05-1514:39:34.293xcodebuild[xxx]Progress0%:Uploadfailed.ValidationfailedSDKversionissue.Thisa
- 如何在 Elementary OS 上安装 Snap Store
山岚的运维笔记
Linux运维及使用ElementaryOSSnapStoreubuntulinux
如何在ElementaryOS上安装SnapStoreSnap是由Ubuntu开发商Canonical推出的一种通用软件包格式,旨在简化软件分发,具有跨Linux发行版的兼容性。与传统的Linux包管理器(如APT、DNF、Pacman等)不同,Snap包将应用程序及其所有依赖项封装在一个独立的容器中,从而解决了不同发行版之间的兼容性问题。如果你想在ElementaryOS上安装SnapStore
- EI学术会议投稿指南:SPRINGER、JPCS、IEEE、SPIE 、ACM出版社简介及检索情况
棱镜研途
学术会议知识计算机视觉学习图像处理信号处理机器学习
投稿可稳定EI检索的国际会议时,选择合适的出版社至关重要!以下是常见出版社及其会议论文集的发表与检索情况:1.Springer(斯普林格)领域:综合类,涵盖工程、计算机、数学、物理等。会议论文集:通常以LNCS(LectureNotesinComputerScience)等系列出版。检索情况:多数被EICompendex、Scopus收录,部分优秀会议可进SCI/SCIE。2.JPCS(Journ
- archlinux中使用 Emoji 字体
ITKEY_
archlinuxarchlinux
在ArchLinux中,若你想正确显示如这类Emoji图标或Unicode符号字体,需要安装支持这些符号的字体包。下面是推荐安装的字体包及说明:noto-fonts-emoji(Google的Emoji字体)最常用、最兼容,显示各种表情符号如、、❤️、⚙️等:安装sudopacman-Snoto-fonts-emoji重新生成字体缓存fc-cache-fv验证echo"音量图标:表情图标:设置图标
- iOS http封装
374016526
ios服务器交互http网络请求
程序开发避免不了与服务器的交互,这里打包了一个自己写的http交互库。希望可以帮到大家。
内置一个basehttp,当我们创建自己的service可以继承实现。
KuroAppBaseHttp *baseHttp = [[KuroAppBaseHttp alloc] init];
[baseHttp setDelegate:self];
[baseHttp
- lolcat :一个在 Linux 终端中输出彩虹特效的命令行工具
brotherlamp
linuxlinux教程linux视频linux自学linux资料
那些相信 Linux 命令行是单调无聊且没有任何乐趣的人们,你们错了,这里有一些有关 Linux 的文章,它们展示着 Linux 是如何的有趣和“淘气” 。
在本文中,我将讨论一个名为“lolcat”的小工具 – 它可以在终端中生成彩虹般的颜色。
何为 lolcat ?
Lolcat 是一个针对 Linux,BSD 和 OSX 平台的工具,它类似于 cat 命令,并为 cat
- MongoDB索引管理(1)——[九]
eksliang
mongodbMongoDB管理索引
转载请出自出处:http://eksliang.iteye.com/blog/2178427 一、概述
数据库的索引与书籍的索引类似,有了索引就不需要翻转整本书。数据库的索引跟这个原理一样,首先在索引中找,在索引中找到条目以后,就可以直接跳转到目标文档的位置,从而使查询速度提高几个数据量级。
不使用索引的查询称
- Informatica参数及变量
18289753290
Informatica参数变量
下面是本人通俗的理解,如有不对之处,希望指正 info参数的设置:在info中用到的参数都在server的专门的配置文件中(最好以parma)结尾 下面的GLOBAl就是全局的,$开头的是系统级变量,$$开头的变量是自定义变量。如果是在session中或者mapping中用到的变量就是局部变量,那就把global换成对应的session或者mapping名字。
[GLOBAL] $Par
- python 解析unicode字符串为utf8编码字符串
酷的飞上天空
unicode
php返回的json字符串如果包含中文,则会被转换成\uxx格式的unicode编码字符串返回。
在浏览器中能正常识别这种编码,但是后台程序却不能识别,直接输出显示的是\uxx的字符,并未进行转码。
转换方式如下
>>> import json
>>> q = '{"text":"\u4
- Hibernate的总结
永夜-极光
Hibernate
1.hibernate的作用,简化对数据库的编码,使开发人员不必再与复杂的sql语句打交道
做项目大部分都需要用JAVA来链接数据库,比如你要做一个会员注册的 页面,那么 获取到用户填写的 基本信后,你要把这些基本信息存入数据库对应的表中,不用hibernate还有mybatis之类的框架,都不用的话就得用JDBC,也就是JAVA自己的,用这个东西你要写很多的代码,比如保存注册信
- SyntaxError: Non-UTF-8 code starting with '\xc4'
随便小屋
python
刚开始看一下Python语言,传说听强大的,但我感觉还是没Java强吧!
写Hello World的时候就遇到一个问题,在Eclipse中写的,代码如下
'''
Created on 2014年10月27日
@author: Logic
'''
print("Hello World!");
运行结果
SyntaxError: Non-UTF-8
- 学会敬酒礼仪 不做酒席菜鸟
aijuans
菜鸟
俗话说,酒是越喝越厚,但在酒桌上也有很多学问讲究,以下总结了一些酒桌上的你不得不注意的小细节。
细节一:领导相互喝完才轮到自己敬酒。敬酒一定要站起来,双手举杯。
细节二:可以多人敬一人,决不可一人敬多人,除非你是领导。
细节三:自己敬别人,如果不碰杯,自己喝多少可视乎情况而定,比如对方酒量,对方喝酒态度,切不可比对方喝得少,要知道是自己敬人。
细节四:自己敬别人,如果碰杯,一
- 《创新者的基因》读书笔记
aoyouzi
读书笔记《创新者的基因》
创新者的基因
创新者的“基因”,即最具创意的企业家具备的五种“发现技能”:联想,观察,实验,发问,建立人脉。
第一部分破坏性创新,从你开始
第一章破坏性创新者的基因
如何获得启示:
发现以下的因素起到了催化剂的作用:(1) -个挑战现状的问题;(2)对某项技术、某个公司或顾客的观察;(3) -次尝试新鲜事物的经验或实验;(4)与某人进行了一次交谈,为他点醒
- 表单验证技术
百合不是茶
JavaScriptDOM对象String对象事件
js最主要的功能就是验证表单,下面是我对表单验证的一些理解,贴出来与大家交流交流 ,数显我们要知道表单验证需要的技术点, String对象,事件,函数
一:String对象;通常是对字符串的操作;
1,String的属性;
字符串.length;表示该字符串的长度;
var str= "java"
- web.xml配置详解之context-param
bijian1013
javaservletweb.xmlcontext-param
一.格式定义:
<context-param>
<param-name>contextConfigLocation</param-name>
<param-value>contextConfigLocationValue></param-value>
</context-param>
作用:该元
- Web系统常见编码漏洞(开发工程师知晓)
Bill_chen
sqlPHPWebfckeditor脚本
1.头号大敌:SQL Injection
原因:程序中对用户输入检查不严格,用户可以提交一段数据库查询代码,根据程序返回的结果,
获得某些他想得知的数据,这就是所谓的SQL Injection,即SQL注入。
本质:
对于输入检查不充分,导致SQL语句将用户提交的非法数据当作语句的一部分来执行。
示例:
String query = "SELECT id FROM users
- 【MongoDB学习笔记六】MongoDB修改器
bit1129
mongodb
本文首先介绍下MongoDB的基本的增删改查操作,然后,详细介绍MongoDB提供的修改器,以完成各种各样的文档更新操作 MongoDB的主要操作
show dbs 显示当前用户能看到哪些数据库
use foobar 将数据库切换到foobar
show collections 显示当前数据库有哪些集合
db.people.update,update不带参数,可
- 提高职业素养,做好人生规划
白糖_
人生
培训讲师是成都著名的企业培训讲师,他在讲课中提出的一些观点很新颖,在此我收录了一些分享一下。注:讲师的观点不代表本人的观点,这些东西大家自己揣摩。
1、什么是职业规划:职业规划并不完全代表你到什么阶段要当什么官要拿多少钱,这些都只是梦想。职业规划是清楚的认识自己现在缺什么,这个阶段该学习什么,下个阶段缺什么,又应该怎么去规划学习,这样才算是规划。
- 国外的网站你都到哪边看?
bozch
技术网站国外
学习软件开发技术,如果没有什么英文基础,最好还是看国内的一些技术网站,例如:开源OSchina,csdn,iteye,51cto等等。
个人感觉如果英语基础能力不错的话,可以浏览国外的网站来进行软件技术基础的学习,例如java开发中常用的到的网站有apache.org 里面有apache的很多Projects,springframework.org是spring相关的项目网站,还有几个感觉不错的
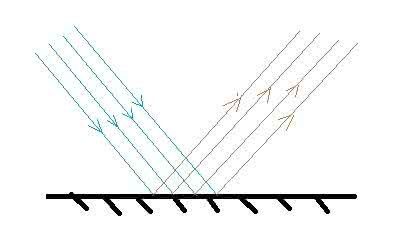
- 编程之美-光影切割问题
bylijinnan
编程之美
package a;
public class DisorderCount {
/**《编程之美》“光影切割问题”
* 主要是两个问题:
* 1.数学公式(设定没有三条以上的直线交于同一点):
* 两条直线最多一个交点,将平面分成了4个区域;
* 三条直线最多三个交点,将平面分成了7个区域;
* 可以推出:N条直线 M个交点,区域数为N+M+1。
- 关于Web跨站执行脚本概念
chenbowen00
Web安全跨站执行脚本
跨站脚本攻击(XSS)是web应用程序中最危险和最常见的安全漏洞之一。安全研究人员发现这个漏洞在最受欢迎的网站,包括谷歌、Facebook、亚马逊、PayPal,和许多其他网站。如果你看看bug赏金计划,大多数报告的问题属于 XSS。为了防止跨站脚本攻击,浏览器也有自己的过滤器,但安全研究人员总是想方设法绕过这些过滤器。这个漏洞是通常用于执行cookie窃取、恶意软件传播,会话劫持,恶意重定向。在
- [开源项目与投资]投资开源项目之前需要统计该项目已有的用户数
comsci
开源项目
现在国内和国外,特别是美国那边,突然出现很多开源项目,但是这些项目的用户有多少,有多少忠诚的粉丝,对于投资者来讲,完全是一个未知数,那么要投资开源项目,我们投资者必须准确无误的知道该项目的全部情况,包括项目发起人的情况,项目的维持时间..项目的技术水平,项目的参与者的势力,项目投入产出的效益.....
- oracle alert log file(告警日志文件)
daizj
oracle告警日志文件alert log file
The alert log is a chronological log of messages and errors, and includes the following items:
All internal errors (ORA-00600), block corruption errors (ORA-01578), and deadlock errors (ORA-00060)
- 关于 CAS SSO 文章声明
denger
SSO
由于几年前写了几篇 CAS 系列的文章,之后陆续有人参照文章去实现,可都遇到了各种问题,同时经常或多或少的收到不少人的求助。现在这时特此说明几点:
1. 那些文章发表于好几年前了,CAS 已经更新几个很多版本了,由于近年已经没有做该领域方面的事情,所有文章也没有持续更新。
2. 文章只是提供思路,尽管 CAS 版本已经发生变化,但原理和流程仍然一致。最重要的是明白原理,然后
- 初二上学期难记单词
dcj3sjt126com
englishword
lesson 课
traffic 交通
matter 要紧;事物
happy 快乐的,幸福的
second 第二的
idea 主意;想法;意见
mean 意味着
important 重要的,重大的
never 从来,决不
afraid 害怕 的
fifth 第五的
hometown 故乡,家乡
discuss 讨论;议论
east 东方的
agree 同意;赞成
bo
- uicollectionview 纯代码布局, 添加头部视图
dcj3sjt126com
Collection
#import <UIKit/UIKit.h>
@interface myHeadView : UICollectionReusableView
{
UILabel *TitleLable;
}
-(void)setTextTitle;
@end
#import "myHeadView.h"
@implementation m
- N 位随机数字串的 JAVA 生成实现
FX夜归人
javaMath随机数Random
/**
* 功能描述 随机数工具类<br />
* @author FengXueYeGuiRen
* 创建时间 2014-7-25<br />
*/
public class RandomUtil {
// 随机数生成器
private static java.util.Random random = new java.util.R
- Ehcache(09)——缓存Web页面
234390216
ehcache页面缓存
页面缓存
目录
1 SimplePageCachingFilter
1.1 calculateKey
1.2 可配置的初始化参数
1.2.1 cach
- spring中少用的注解@primary解析
jackyrong
primary
这次看下spring中少见的注解@primary注解,例子
@Component
public class MetalSinger implements Singer{
@Override
public String sing(String lyrics) {
return "I am singing with DIO voice
- Java几款性能分析工具的对比
lbwahoo
java
Java几款性能分析工具的对比
摘自:http://my.oschina.net/liux/blog/51800
在给客户的应用程序维护的过程中,我注意到在高负载下的一些性能问题。理论上,增加对应用程序的负载会使性能等比率的下降。然而,我认为性能下降的比率远远高于负载的增加。我也发现,性能可以通过改变应用程序的逻辑来提升,甚至达到极限。为了更详细的了解这一点,我们需要做一些性能
- JVM参数配置大全
nickys
jvm应用服务器
JVM参数配置大全
/usr/local/jdk/bin/java -Dresin.home=/usr/local/resin -server -Xms1800M -Xmx1800M -Xmn300M -Xss512K -XX:PermSize=300M -XX:MaxPermSize=300M -XX:SurvivorRatio=8 -XX:MaxTenuringThreshold=5 -
- 搭建 CentOS 6 服务器(14) - squid、Varnish
rensanning
varnish
(一)squid
安装
# yum install httpd-tools -y
# htpasswd -c -b /etc/squid/passwords squiduser 123456
# yum install squid -y
设置
# cp /etc/squid/squid.conf /etc/squid/squid.conf.bak
# vi /etc/
- Spring缓存注解@Cache使用
tom_seed
spring
参考资料
http://www.ibm.com/developerworks/cn/opensource/os-cn-spring-cache/
http://swiftlet.net/archives/774
缓存注解有以下三个:
@Cacheable @CacheEvict @CachePut
- dom4j解析XML时出现"java.lang.noclassdeffounderror: org/jaxen/jaxenexception"错误
xp9802
java.lang.NoClassDefFoundError: org/jaxen/JaxenExc
关键字: java.lang.noclassdeffounderror: org/jaxen/jaxenexception
使用dom4j解析XML时,要快速获取某个节点的数据,使用XPath是个不错的方法,dom4j的快速手册里也建议使用这种方式
执行时却抛出以下异常:
Exceptio