leetcode刷题(剑指offer) 240.搜索二维矩阵Ⅱ

240.搜索二维矩阵Ⅱ

编写一个高效的算法来搜索 *m* x *n* 矩阵 matrix 中的一个目标值 target 。该矩阵具有以下特性：

• 每行的元素从左到右升序排列。
• 每列的元素从上到下升序排列。

示例 1：

输入：matrix = [[1,4,7,11,15],[2,5,8,12,19],[3,6,9,16,22],[10,13,14,17,24],[18,21,23,26,30]], target = 5
输出：true

示例 2：

输入：matrix = [[1,4,7,11,15],[2,5,8,12,19],[3,6,9,16,22],[10,13,14,17,24],[18,21,23,26,30]], target = 20
输出：false

提示：

• m == matrix.length
• n == matrix[i].length
• 1 <= n, m <= 300
• -109 <= matrix[i][j] <= 109
• 每行的所有元素从左到右升序排列
• 每列的所有元素从上到下升序排列
• -109 <= target <= 109

解法：

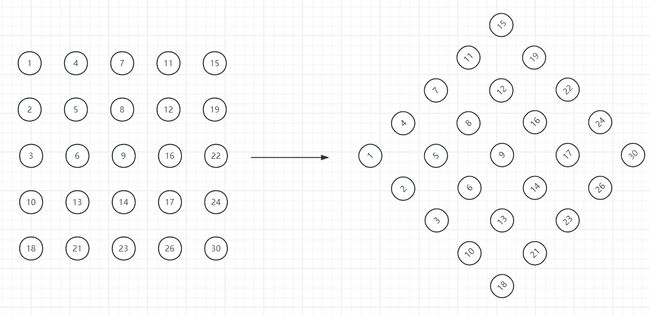
仔细观察上图可以发现，从右上角出发，每次往左一格都是更小的数值，每次往下一格都是更大的数值，且从右上角的位置，以往左和往下这两个方向可以抵达二维数组的任何一格。

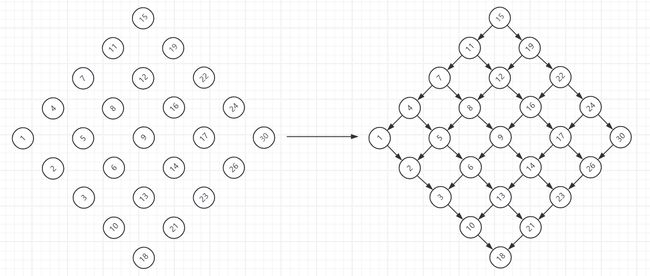
可能直接从上图的矩阵上来看不容易理解，那么把这个图翻转45°变成下图所示呢。

再加入箭头。。。。。

是不是有点二叉排序树的感觉了。15就是根节点，搜索的次数最多就为这颗树的深度。

从15这个位置开始，如果目标数比15更大就向右，比15更小就向左，以此迭代，最后可以找到目标数，如果遍历到不能再向下遍历了，那就是矩阵中不存在目标数。

方法的时间复杂度为O(N)， 空间复杂度为O(1)。

代码如下：

/**
 * @author bwzfy
 * @ClassName _240搜索二维矩阵
 * @create 2024/1/23 - 17:40
 * @Version 1.0
 **/
public class _240搜索二维矩阵Ⅱ {

    private static boolean res = false;

    public static void main(String[] args) {
        int[][] matrix = new int[][]{
                {1, 4, 7, 11, 15},
                {2, 5, 8, 12, 19},
                {3, 6, 9, 16, 22},
                {10, 13, 14, 17, 24},
                {18, 21, 23, 26, 30}
        };
        System.out.println(searchMatrix(matrix, 12));
    }

    public static boolean searchMatrix(int[][] matrix, int target) {
        if (matrix.length == 0 || matrix[0].length == 0) {
            return false;
        }
        int row = 0;
        int col = matrix[0].length - 1;
        while (row < matrix.length && col >= 0) {
            int value = matrix[row][col];
            if (target > value) {
                row++;
            } else if (target < value) {
                col--;
            } else {
              return true;
            }
        }
        return false;
    }

}