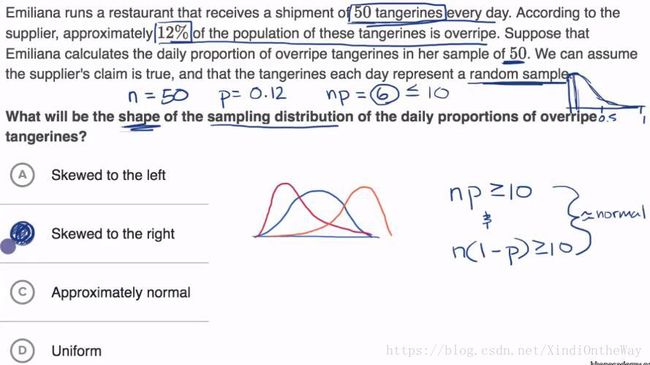
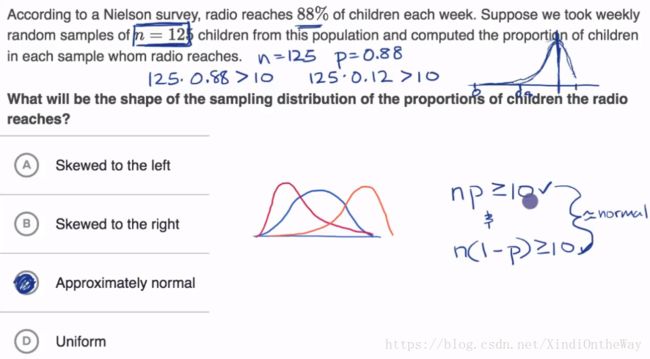
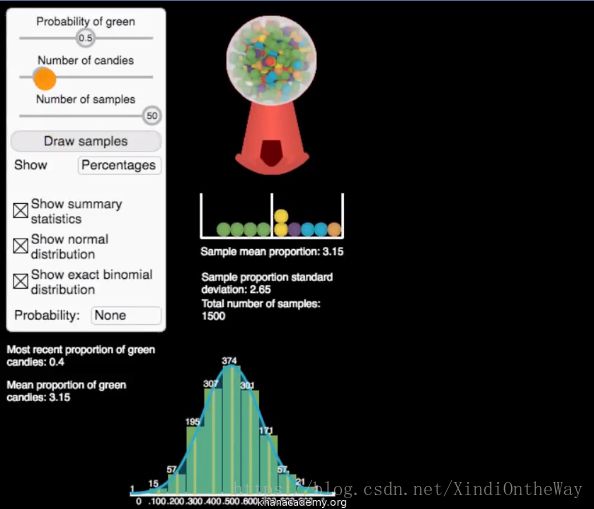
- 0代码改动实现应用运行时数据库密码无损轮转
阿里-于怀
oracle数据库nacos
作者:柳遵飞一.敏感数据的安全风险在应用程序中,访问数据库几乎是必须的,是实现业务功能的基础普遍场景,应用程序访问数据库,需要设置数据库的地址,端口,账号及密码。密码的安全性非常重要,业界密码泄漏导致资损的事件时有发生,根据相关统计,单次泄漏事件的发生平均导致488万美元(约合人民币3542万元),每条泄漏的数据记录平均导致169美元(约合人民币1226元),除了直观的资金损失外,对企业的形象和舆
- R 语言安装使用教程
小奇JAVA面试
安装使用教程r语言开发语言
一、R语言简介R是一种用于统计分析、数据挖掘和可视化的编程语言和环境。它在学术界和数据分析领域中广泛使用,拥有丰富的统计函数库和绘图功能。二、安装R语言2.1下载R安装包前往CRAN官网下载适合你操作系统的安装程序:官网地址:https://cran.r-project.org/2.2Windows安装下载.exe安装包;双击安装程序,按默认选项一路安装即可;安装完成后,可通过RGUI或命令行启动
- 结构型智能科技的关键可行性——信息型智能向结构型智能的转变(修改提纲)
刘海东刘海东
人工智能机器学习算法
结构型智能科技的关键可行性——信息型智能向结构型智能的转变1.信息型智能科技概述1.1传统计算机科技的信息型继承者1.2信息型智能环境1.3信息型智能主体1.4机器学习创造的智能1.5信息型智能科技的缺陷2.结构型智能科技概述2.1传统计算机科技向生命结构的发展2.2结构型智能科技的环境2.3结构型智能科技创造的机器生命2.4结构型智能科技的科学性3.结构型智能科技的关键可行性——信息型智能向结构
- ArcMap图斑四至坐标计算教程
JGiser
Arcpyarcgis
一、功能概述本教程介绍如何在ArcMap中自动计算矢量图层的每个图斑四至坐标(西、东、南、北边界点),并将结果保存到属性表中。四至坐标采用WGS84坐标系(经纬度),输出格式为:西:经度,纬度东:经度,纬度南:经度,纬度北:经度,纬度二、适用场景行政区划边界范围标注地块位置信息记录地理要素范围统计分析地图图例和位置索引制作三、两种实现方法方法一:手动操作(适合少量图斑)添加字段:右键图层→打开属性
- 008-HCIP-H12-821题库笔记(101-150)
深度学习0407
网络安全学习笔记笔记网络智能路由器
101、传统的流量统计的实现方法和局限性:(1)基于IP报文计数:无法针对多种信息进行统计(2)使用ACL:要求ACL的容量很大,对于ACL规则以外的流没有办法统计(3)SNMP协议:功能不强。要不断的通过轮询向网管查询,浪费CPU和网络资源(4)端口镜像:成本高,同时消耗设备的一个接口,对于无法镜像的端口无能为力(5)物理层复制:成本高,同时还需要购买专用的硬件设备基于IP报文计数:这种方法虽然
- 多模态大模型:技术原理与实战 看清GPT的进化史和创新点
AI天才研究院
AgenticAI实战计算AI人工智能与大数据计算科学神经计算深度学习神经网络大数据人工智能大型语言模型AIAGILLMJavaPython架构设计AgentRPA
多模态大模型:技术原理与实战看清GPT的进化史和创新点1.背景介绍1.1人工智能的发展历程1.1.1早期人工智能1.1.2机器学习时代1.1.3深度学习的崛起1.2自然语言处理的演进1.2.1基于规则的方法1.2.2统计机器学习方法1.2.3深度学习方法1.3大语言模型的出现1.3.1Transformer架构的提出1.3.2GPT系列模型的发展1.3.3多模态大模型的兴起2.核心概念与联系2.1
- Seaborn 教程
froginwe11
开发语言
Seaborn教程引言Seaborn是一个强大的Python数据可视化库,它建立在Matplotlib的基础上,专门用于统计图形的绘制。Seaborn提供了一系列的绘图功能,使得统计数据的可视化变得更加简单和直观。本文将为您提供一个全面的Seaborn教程,帮助您快速掌握其基本用法和高级技巧。安装与导入在开始之前,请确保您的Python环境中已经安装了Seaborn和Matplotlib。您可以使
- 网络资源模板--基于Android Studio 实现的喝水提醒App
编程乐学
Android网络项目模板androidstudioandroidide安卓大作业移动端开发大作业喝水提醒
目录一、测试环境说明二、项目简介三、项目演示四、部设计详情(部分)注册页面首页统计页五、项目源码一、测试环境说明二、项目简介本应用采用经典的MVC(Model-View-Controller)架构,将数据模型(Model)、视图(View)和控制器(Controller)分离,提高代码的可维护性和可扩展性。Model:负责数据的存储和处理,包括用户信息、饮水记录等。使用Room数据库来实现数据的持
- R语言的游戏开发
柳婉晴
包罗万象golang开发语言后端
R语言在游戏开发中的应用随着科技的发展,游戏行业已经成为一个巨大的市场。虽然通常我们会认为游戏开发主要是使用C++、C#、JavaScript等语言,但实际上,R语言在游戏开发中也有其独特的应用,尤其是在数据分析和可视化方面。本文将探讨R语言在游戏开发中的应用,涵盖它的基础、游戏设计的复杂性、实际案例分析、以及未来的发展方向。一、R语言基础R语言是一种用于统计计算和数据分析的编程语言。它具有强大的
- R语言的软件开发工具
纪霁然
包罗万象golang开发语言后端
R语言的软件开发工具引言R语言因其强大的数据分析能力和丰富的统计包,自发布以来便广受欢迎。随着数据科学和分析的迅猛发展,R语言也逐渐成为数据分析、机器学习和统计建模领域的重要工具。为了更好地利用R语言进行软件开发,许多软件开发工具和环境应运而生。本文将深入探讨R语言的主要开发工具,帮助开发者更高效地进行数据处理和分析。1.R和RStudio基础R语言本身是一个用于统计计算和图形绘制的编程语言,而R
- 每日一题 2025-7-6 《努力的乐乐》
WYC135164
算法
K14363努力的乐乐题目描述乐乐的数学成绩在班级里很拔尖,但是语文成绩一直不太好,所以他在这个学期一开始就一直很努力学习语文。这个学期乐乐一共参加了n次考试,他现在想统计下这n次考试中,自己的语文成绩和数学成绩之间的差距有没有缩小。现在给出乐乐这n次考试每一次的两科成绩,请你帮助他算一下每一次数学成绩减去语文成绩的差值。输入格式第一行,一个正整数n,表示乐乐参加的考试次数。接下来n行,每行两个正
- 电缆隧道监控设备应用方案
李子圆圆
人工智能
一、引言:当城市“生命线”遭遇巡检困境在城市地下纵横交错的电缆隧道,就像人体内的血管,默默输送着维持城市运转的电力“血液”。随着城镇化加速,我国电缆隧道长度已突破百万公里,年用电量更是以5%的速度递增。然而,传统人工巡检却如同用算盘计算航天数据——2022年统计显示,全国因巡检疏漏导致的电缆事故超3000起,平均故障修复时间长达6小时。当“骑自行车”的巡检速度遇上“高铁级”的城市发展需求,一场监控
- Github 2025-07-04 Java开源项目日报 Top10
老孙正经胡说
githubjava开源Github趋势分析开源项目PythonGolang
根据GithubTrendings的统计,今日(2025-07-04统计)共有10个项目上榜。根据开发语言中项目的数量,汇总情况如下:开发语言项目数量Java项目10Java实现的算法集合:使用Gitpod.io进行编辑和贡献创建周期:2883天开发语言:Java协议类型:MITLicenseStar数量:57266个Fork数量:18692次关注人数:57266人贡献人数:431人OpenIss
- happy-llm 第一章 NLP 基础概念
weixin_38374194
自然语言处理人工智能学习
文章目录一、什么是NLP?二、NLP发展三大阶段三、NLP核心任务精要四、文本表示演进史1.传统方法:统计表征2.神经网络:语义向量化课程地址:happy-llmNLP基础概念一、什么是NLP?核心目标:让计算机理解、生成、处理人类语言,实现人机自然交互。现状与挑战:成就:深度学习推动文本分类、翻译等任务达到近人类水平。瓶颈:歧义性、隐喻理解、跨文化差异等。二、NLP发展三大阶段时期代表技术核心思
- 1997-2020年 全国31个省外商直接投资FDI统计数据
小王毕业啦
大数据人工智能数据挖掘数据分析大数据社科数据数据统计深度学习
1997-2020年全国31个省外商直接投资FDI统计数据.r.rarhttps://download.csdn.net/download/2401_84585615/90001897https://download.csdn.net/download/2401_84585615/90001897外商直接投资(FDI)是指外国投资者在东道国进行的长期投资活动,通常包括设立新企业、并购现有企业或对现
- 九章数学体系开源工程白皮书
《九章数学体系开源工程白皮书》前言:从公理冲突到场景适配的计算革命传统计算系统深陷“体系冲突陷阱”:阿基米德体系以“无穷可分”“绝对无穷不可达”为公理,适合描述开域,然而,99%以上的物理闭域场景(如星系边界、原子结构)是闭域。因“开域无穷假设”与“闭域有限性”的本质矛盾,必然产生类似芝诺悖论的逻辑错误——暗物质谜题、量子叠加态的概率描述、高维空间假设,本质上都是这种“公理-场景错配”的产物。如同
- Oracle 高级 SQL 查询与函数详解:多表连接、子查询、聚合、分析函数
白仑色
Oracle系列oracleSQL进阶多表连接子查询分析函数
关键词:Oracle高级SQL、JOIN查询、子查询、GROUPBY、聚合函数、ROW_NUMBER、LAG/LEAD、CASEWHEN、DECODE✅摘要在实际业务开发中,我们经常需要从多个表中提取数据、进行复杂计算和统计。Oracle提供了强大的SQL功能支持这些操作,包括:多表连接(INNERJOIN、LEFTJOIN等)子查询(标量子查询、列子查询等)聚合函数与GROUPBY分组字符处理、
- 面向高校的人工智能通识教育课程实验设计方案
武汉唯众智创
人工智能人工智能通识教育课程实验人工智能通识教育人工智能通识课程人工智能通识
一、前言2018年,教育部发布《高等学校人工智能创新行动计划》,明确提出“重视人工智能与计算机、控制、数学、统计学、物理学、生物学、心理学、社会学、法学等学科专业教育的交叉融合,探索‘人工智能+X’的人才培养模式”。过去,人工智能教育多集中于研究生阶段,本科生接触机会相对有限。2019年,教育部批准35所高校增设“人工智能”本科专业,这标志着人工智能正式纳入本科教育体系。如今,人工智能课程大多是计
- 构建“城市生活指数”爬虫系统:抓取物价、租金、工资等数据并可视化实战
程序员威哥
生活爬虫python开发语言seleniumbeautifulsoup
一、项目背景“城市生活指数”是一种综合反映城市居民生活成本和经济水平的指标。通过抓取不同网站上的物价、租金、工资等数据,结合数据分析和可视化,可以帮助用户直观比较各城市生活压力和经济实力,为工作、生活决策提供数据支持。二、数据来源与选取1.物价数据典型网站:物价类统计网站、超市/电商价格(如淘宝、京东)、地方统计局官网示例网站:国家统计局物价数据、各城市生活成本调查网站2.房租数据典型网站:链家、
- Boostrap方法的理解及应用
Xiaofei@IDO
统计学概率论机器学习数据挖掘
1、Boostrap介绍1.1概念性解释Boostrap统计学方法是一种非参数检验方法,用于估计各种统计量的置信区间。Boostrap计算步骤简单的描述为:通过有放回的数据集的重采样,产生一系列的待检验统计量的Boostrap经验分布。基于该分布,计算标准误差,构建置信区间,并对多种类型的样本进行统计信息和假设检验。Boostrap统计学方法使用范围比较广,因为它不需要假定数据服从特定的理论分布(
- 【华为od刷题(C++)】HJ18 识别有效的IP地址和掩码并进行分类统计
m0_64866459
华为odc++tcp/ip
我的代码:#include//这个头文件包含了用于输入和输出的标准库#include//这个头文件提供了与C风格字符串(字符数组)相关的操作函数#include//vector可以存储任意类型的数据,并且支持高效的随机访问、动态增长、删除等操作#include//这个头文件包含了一些字符处理的函数,这些函数可以用于检查或转换单个字符的状态#include//这个头文件是C标准库中的一部分,提供了与
- 隐马尔可夫模型:语音识别系统的时序解码引擎
大千AI助手
人工智能Python#OTHER语音识别人工智能机器学习概率马尔科夫链HMM
本文由「大千AI助手」原创发布,专注用真话讲AI,回归技术本质。拒绝神话或妖魔化。搜索「大千AI助手」关注我,一起撕掉过度包装,学习真实的AI技术!1HMM与语音识别的理论基础隐马尔可夫模型(HMM)作为一种双重随机过程的统计模型,其核心在于描述一个包含隐含状态的马尔可夫链,以及这些状态生成可观测输出的概率分布。在语音识别领域,HMM的时序建模能力与语音信号的特性形成了完美契合:隐含状态:对应语音
- spring boot 之 集成 druid数据库连接池
我叫晨曦啊
springbootspringboot数据库后端
springboot版本:2.3.12.RELEASEMySQL版本:8.0Druid简介Druid是阿里开源的一个数据库连接池和SQL查询优化工具,用于提高应用程序对数据库的性能和可扩展性。主要提供的功能:数据库连接池、数据库连接池监控、SQL查询优化、数据源管理、防御SQL注入、统计和监控。引入pom依赖com.alibabadruid-spring-boot-starter1.2.16com
- Python与Dlib库实现人脸技术实战
西域情歌
本文还有配套的精品资源,点击获取简介:本项目详细说明了如何使用Python结合Dlib库实现人脸检测、识别、数量检测和距离检测。利用Dlib提供的机器学习算法和计算机视觉功能,包括HOG特征检测、级联分类器、面部特征向量模型和关键点预测等,项目能够快速准确地在图像中检测和识别人脸。此外,还介绍了如何统计图像中的人脸数量以及如何计算人脸之间的距离。通过实际代码资源,开发者能够掌握实时人脸技术的应用,
- NVMe高速传输之摆脱XDMA设计11:性能监测单元设计
tiantianuser
NVMEIPfpga开发verilog高速存储NVMePCIe
性能监测单元负责监测NVMeoverPCIe逻辑加速引擎的运行状态和统计信息,包括复位后运行时间信息、NVMe指令数量统计信息、数据操作数量统计信息、IOPS性能统计信息、指令延迟统计信息等。这些信息存储在性能监测单元中的性能监测寄存器组中,性能监测寄存器组定义如表1所示。表1性能监测寄存器组定义想进一步了解相关视频,请搜索B站用户:专注与守望链接:https://space.bilibili.c
- MySQL高级查询:聚合与分组分析实战指南
Yang's
mysqlsql数据库
一、聚合函数深度解析1.五大核心聚合函数--统计员工数量SELECTCOUNT(*)AStotal_employeesFROMemployees;--计算平均薪资(排除NULL)SELECTAVG(IFNULL(salary,0))ASavg_salaryFROMemployees;--获取最高/最低薪资SELECTMAX(salary)ASmax_salary,MIN(salary)ASmin_
- KNN(K-近邻算法)(上)--day05
扫把星133
机器学习python人工智能近邻算法算法
KNN(K-NearestNeighbors,K近邻算法)是一种用于分类和回归的非参数化方法。其基本思想是通过找出与新样本最接近的已标记数据中的K个最近邻居来进行预测或分类。注释:非参数化方法是指在统计学和机器学习中,不对数据分布做出严格假设(这些假设通常包括
- 【2025/07/03】GitHub 今日热门项目
Albert_Lsk
Github推荐github
GitHub今日热门项目每日精选优质开源项目|发现优质开源项目,跟上技术发展趋势报告概览统计项数值说明报告日期2025-07-03(周四)GitHubTrending每日快照数据时间09:57:11实时爬取生成项目总数16个精选热门开源项目⭐总星数452.7K社区认可度指标今日热度+5.6K24小时新增关注数据洞察核心指标项目总览16个精选项目⭐社区认可452.7K总星标数今日热度5.6K新增关注
- 一个简易的短地址服务小练习
MeshddY
c语言
从零实现一个简易的本地短地址服务练习:原理详解与C语言实践(示意图:长URL与短地址转换过程)一、需求背景1.1为什么需要短地址?节省字符空间:在短信、社交媒体等场景中缩短长链接美化展示:提升营销链接的可读性和点击率访问统计:通过短地址追踪访问数据1.2核心功能需求功能输入输出生成短地址原始URL(最长2048字符)5位短码(如abc12)查询原始地址5位短码对应的原始URL访问统计自动记录显示每
- 2025年30万级SUV终极对决:理想L7强势领衔
Jamie20190106
算法
权威数据背书:据乘联会2025年1-5月销量统计,30万级SUV市场新能源渗透率达62%,其中增程式车型同比增长85%(数据来源:中国汽车工业协会)一、理想L72025款:颠覆认知的六边形战士核心升级亮点双模混动系统:搭载宁德时代新一代磷酸铁锂电池(容量42.8kWh),纯电续航240km(CLTC),综合续航1315km,馈电油耗仅5.9L/100km(工信部认证)魔毯底盘2.0:CDC连续可变
- mysql主从数据同步
林鹤霄
mysql主从数据同步
配置mysql5.5主从服务器(转)
教程开始:一、安装MySQL
说明:在两台MySQL服务器192.168.21.169和192.168.21.168上分别进行如下操作,安装MySQL 5.5.22
二、配置MySQL主服务器(192.168.21.169)mysql -uroot -p &nb
- oracle学习笔记
caoyong
oracle
1、ORACLE的安装
a>、ORACLE的版本
8i,9i : i是internet
10g,11g : grid (网格)
12c : cloud (云计算)
b>、10g不支持win7
&
- 数据库,SQL零基础入门
天子之骄
sql数据库入门基本术语
数据库,SQL零基础入门
做网站肯定离不开数据库,本人之前没怎么具体接触SQL,这几天起早贪黑得各种入门,恶补脑洞。一些具体的知识点,可以让小白不再迷茫的术语,拿来与大家分享。
数据库,永久数据的一个或多个大型结构化集合,通常与更新和查询数据的软件相关
- pom.xml
一炮送你回车库
pom.xml
1、一级元素dependencies是可以被子项目继承的
2、一级元素dependencyManagement是定义该项目群里jar包版本号的,通常和一级元素properties一起使用,既然有继承,也肯定有一级元素modules来定义子元素
3、父项目里的一级元素<modules>
<module>lcas-admin-war</module>
<
- sql查地区省市县
3213213333332132
sqlmysql
-- db_yhm_city
SELECT * FROM db_yhm_city WHERE class_parent_id = 1 -- 海南 class_id = 9 港、奥、台 class_id = 33、34、35
SELECT * FROM db_yhm_city WHERE class_parent_id =169
SELECT d1.cla
- 关于监听器那些让人头疼的事
宝剑锋梅花香
画图板监听器鼠标监听器
本人初学JAVA,对于界面开发我只能说有点蛋疼,用JAVA来做界面的话确实需要一定的耐心(不使用插件,就算使用插件的话也没好多少)既然Java提供了界面开发,老师又要求做,只能硬着头皮上啦。但是监听器还真是个难懂的地方,我是上了几次课才略微搞懂了些。
- JAVA的遍历MAP
darkranger
map
Java Map遍历方式的选择
1. 阐述
对于Java中Map的遍历方式,很多文章都推荐使用entrySet,认为其比keySet的效率高很多。理由是:entrySet方法一次拿到所有key和value的集合;而keySet拿到的只是key的集合,针对每个key,都要去Map中额外查找一次value,从而降低了总体效率。那么实际情况如何呢?
为了解遍历性能的真实差距,包括在遍历ke
- POJ 2312 Battle City 优先多列+bfs
aijuans
搜索
来源:http://poj.org/problem?id=2312
题意:题目背景就是小时候玩的坦克大战,求从起点到终点最少需要多少步。已知S和R是不能走得,E是空的,可以走,B是砖,只有打掉后才可以通过。
思路:很容易看出来这是一道广搜的题目,但是因为走E和走B所需要的时间不一样,因此不能用普通的队列存点。因为对于走B来说,要先打掉砖才能通过,所以我们可以理解为走B需要两步,而走E是指需要1
- Hibernate与Jpa的关系,终于弄懂
avords
javaHibernate数据库jpa
我知道Jpa是一种规范,而Hibernate是它的一种实现。除了Hibernate,还有EclipseLink(曾经的toplink),OpenJPA等可供选择,所以使用Jpa的一个好处是,可以更换实现而不必改动太多代码。
在play中定义Model时,使用的是jpa的annotations,比如javax.persistence.Entity, Table, Column, OneToMany
- 酸爽的console.log
bee1314
console
在前端的开发中,console.log那是开发必备啊,简直直观。通过写小函数,组合大功能。更容易测试。但是在打版本时,就要删除console.log,打完版本进入开发状态又要添加,真不够爽。重复劳动太多。所以可以做些简单地封装,方便开发和上线。
/**
* log.js hufeng
* The safe wrapper for `console.xxx` functions
*
- 哈佛教授:穷人和过于忙碌的人有一个共同思维特质
bijian1013
时间管理励志人生穷人过于忙碌
一个跨学科团队今年完成了一项对资源稀缺状况下人的思维方式的研究,结论是:穷人和过于忙碌的人有一个共同思维特质,即注意力被稀缺资源过分占据,引起认知和判断力的全面下降。这项研究是心理学、行为经济学和政策研究学者协作的典范。
这个研究源于穆来纳森对自己拖延症的憎恨。他7岁从印度移民美国,很快就如鱼得水,哈佛毕业
- other operate
征客丶
OSosx
一、Mac Finder 设置排序方式,预览栏 在显示-》查看显示选项中
二、有时预览显示时,卡死在那,有可能是一些临时文件夹被删除了,如:/private/tmp[有待验证]
--------------------------------------------------------------------
若有其他凝问或文中有错误,请及时向我指出,
我好及时改正,同时也让我们一
- 【Scala五】分析Spark源代码总结的Scala语法三
bit1129
scala
1. If语句作为表达式
val properties = if (jobIdToActiveJob.contains(jobId)) {
jobIdToActiveJob(stage.jobId).properties
} else {
// this stage will be assigned to "default" po
- ZooKeeper 入门
BlueSkator
中间件zk
ZooKeeper是一个高可用的分布式数据管理与系统协调框架。基于对Paxos算法的实现,使该框架保证了分布式环境中数据的强一致性,也正是基于这样的特性,使得ZooKeeper解决很多分布式问题。网上对ZK的应用场景也有不少介绍,本文将结合作者身边的项目例子,系统地对ZK的应用场景进行一个分门归类的介绍。
值得注意的是,ZK并非天生就是为这些应用场景设计的,都是后来众多开发者根据其框架的特性,利
- MySQL取得当前时间的函数是什么 格式化日期的函数是什么
BreakingBad
mysqlDate
取得当前时间用 now() 就行。
在数据库中格式化时间 用DATE_FORMA T(date, format) .
根据格式串format 格式化日期或日期和时间值date,返回结果串。
可用DATE_FORMAT( ) 来格式化DATE 或DATETIME 值,以便得到所希望的格式。根据format字符串格式化date值:
%S, %s 两位数字形式的秒( 00,01,
- 读《研磨设计模式》-代码笔记-组合模式
bylijinnan
java设计模式
声明: 本文只为方便我个人查阅和理解,详细的分析以及源代码请移步 原作者的博客http://chjavach.iteye.com/
import java.util.ArrayList;
import java.util.List;
abstract class Component {
public abstract void printStruct(Str
- 4_JAVA+Oracle面试题(有答案)
chenke
oracle
基础测试题
卷面上不能出现任何的涂写文字,所有的答案要求写在答题纸上,考卷不得带走。
选择题
1、 What will happen when you attempt to compile and run the following code? (3)
public class Static {
static {
int x = 5; // 在static内有效
}
st
- 新一代工作流系统设计目标
comsci
工作算法脚本
用户只需要给工作流系统制定若干个需求,流程系统根据需求,并结合事先输入的组织机构和权限结构,调用若干算法,在流程展示版面上面显示出系统自动生成的流程图,然后由用户根据实际情况对该流程图进行微调,直到满意为止,流程在运行过程中,系统和用户可以根据情况对流程进行实时的调整,包括拓扑结构的调整,权限的调整,内置脚本的调整。。。。。
在这个设计中,最难的地方是系统根据什么来生成流
- oracle 行链接与行迁移
daizj
oracle行迁移
表里的一行对于一个数据块太大的情况有二种(一行在一个数据块里放不下)
第一种情况:
INSERT的时候,INSERT时候行的大小就超一个块的大小。Oracle把这行的数据存储在一连串的数据块里(Oracle Stores the data for the row in a chain of data blocks),这种情况称为行链接(Row Chain),一般不可避免(除非使用更大的数据
- [JShop]开源电子商务系统jshop的系统缓存实现
dinguangx
jshop电子商务
前言
jeeshop中通过SystemManager管理了大量的缓存数据,来提升系统的性能,但这些缓存数据全部都是存放于内存中的,无法满足特定场景的数据更新(如集群环境)。JShop对jeeshop的缓存机制进行了扩展,提供CacheProvider来辅助SystemManager管理这些缓存数据,通过CacheProvider,可以把缓存存放在内存,ehcache,redis,memcache
- 初三全学年难记忆单词
dcj3sjt126com
englishword
several 儿子;若干
shelf 架子
knowledge 知识;学问
librarian 图书管理员
abroad 到国外,在国外
surf 冲浪
wave 浪;波浪
twice 两次;两倍
describe 描写;叙述
especially 特别;尤其
attract 吸引
prize 奖品;奖赏
competition 比赛;竞争
event 大事;事件
O
- sphinx实践
dcj3sjt126com
sphinx
安装参考地址:http://briansnelson.com/How_to_install_Sphinx_on_Centos_Server
yum install sphinx
如果失败的话使用下面的方式安装
wget http://sphinxsearch.com/files/sphinx-2.2.9-1.rhel6.x86_64.rpm
yum loca
- JPA之JPQL(三)
frank1234
ormjpaJPQL
1 什么是JPQL
JPQL是Java Persistence Query Language的简称,可以看成是JPA中的HQL, JPQL支持各种复杂查询。
2 检索单个对象
@Test
public void querySingleObject1() {
Query query = em.createQuery("sele
- Remove Duplicates from Sorted Array II
hcx2013
remove
Follow up for "Remove Duplicates":What if duplicates are allowed at most twice?
For example,Given sorted array nums = [1,1,1,2,2,3],
Your function should return length
- Spring4新特性——Groovy Bean定义DSL
jinnianshilongnian
spring 4
Spring4新特性——泛型限定式依赖注入
Spring4新特性——核心容器的其他改进
Spring4新特性——Web开发的增强
Spring4新特性——集成Bean Validation 1.1(JSR-349)到SpringMVC
Spring4新特性——Groovy Bean定义DSL
Spring4新特性——更好的Java泛型操作API
Spring4新
- CentOS安装Mysql5.5
liuxingguome
centos
CentOS下以RPM方式安装MySQL5.5
首先卸载系统自带Mysql:
yum remove mysql mysql-server mysql-libs compat-mysql51
rm -rf /var/lib/mysql
rm /etc/my.cnf
查看是否还有mysql软件:
rpm -qa|grep mysql
去http://dev.mysql.c
- 第14章 工具函数(下)
onestopweb
函数
index.html
<!DOCTYPE html PUBLIC "-//W3C//DTD XHTML 1.0 Transitional//EN" "http://www.w3.org/TR/xhtml1/DTD/xhtml1-transitional.dtd">
<html xmlns="http://www.w3.org/
- POJ 1050
SaraWon
二维数组子矩阵最大和
POJ ACM第1050题的详细描述,请参照
http://acm.pku.edu.cn/JudgeOnline/problem?id=1050
题目意思:
给定包含有正负整型的二维数组,找出所有子矩阵的和的最大值。
如二维数组
0 -2 -7 0
9 2 -6 2
-4 1 -4 1
-1 8 0 -2
中和最大的子矩阵是
9 2
-4 1
-1 8
且最大和是15
- [5]设计模式——单例模式
tsface
java单例设计模式虚拟机
单例模式:保证一个类仅有一个实例,并提供一个访问它的全局访问点
安全的单例模式:
/*
* @(#)Singleton.java 2014-8-1
*
* Copyright 2014 XXXX, Inc. All rights reserved.
*/
package com.fiberhome.singleton;
- Java8全新打造,英语学习supertool
yangshangchuan
javasuperword闭包java8函数式编程
superword是一个Java实现的英文单词分析软件,主要研究英语单词音近形似转化规律、前缀后缀规律、词之间的相似性规律等等。Clean code、Fluent style、Java8 feature: Lambdas, Streams and Functional-style Programming。
升学考试、工作求职、充电提高,都少不了英语的身影,英语对我们来说实在太重要