看这玩意复习你还会挂科?《数据结构篇》
一.绪论
1.何谓程序设计?
程序 = 算法 + 数据结构
2.数据结构的定义
是相互之间存在一种或多种特定关系的数据元素的集合
3.数据、数据元素、数据对象的概念
数据(data):对客观事物的符号表示,含义很广,指数、图像、声音、文本等一切计算机可以处理的事物。
数据元素(data element):组成数据的基本单位。
数据对象(data object):性质相同的数据元素的集合。
4.四种基本的数据结构类型
集合结构、线性结构、树型结构、图形结构
5.两种存储结构(计算机中的实现方式)
顺序存储结构、链式存储结构
6.理解顺序存储与链式存储的方式、特点、优缺点、适用情况
7.数据类型、抽象数据类型
数据类型(Data Type):刻画程序对象的一种方式,包括值的集合与在这组值上的操作(一个成功的数据结构,就要设计成一种数据类型)
抽象数据类型(Abstract Data Type,ADT):是与数据类型相似的概念
ADT name
{ 数据对象 Data
数据关系 Relation
数据操作 Operation }
8.抽象数据类型的意义
ADT着重数据结构的操作接口,不关心具体的实现,主要是面向用户。ADT是数据结构设计所追求的目标。
9.何谓算法
算法是解决特定问题求解步骤的描述
10.算法特征
(1)有穷性:一个算法必须在执行有限步之后结束,每一步都在有穷时间内完成
(2)确定性:算法中每一步都有确切的含义,不会产生二义性
(3)可行性:算法的每一步操作都可以通过已有的可行的操作来完成
(4)输入:算法有零个或若干个数据输入
(5)输出:算法有一个或若干个数据输出
11.算法设计的要求
(1)正确性:能够解决问题
a.程序不含语法错误
b.对于几组正常的输入数据,能输出满足要求的结果
c.对于正常和异常数据,能给出满足要求的结果
d.对一切合法数据,都能够产生满足要求的结果
(2)可读性
(3)健壮性(要有异常处理机制)
(4)效率和低储存容量需求
12.算法好坏的衡量标准
(1)事后统计
(2)事前估计
a.时间复杂度估计
b.空间复杂度估计
13.时间复杂度与空间复杂度
时间复杂度:T(n) = O( f(n) ),n为问题的规模。撇开具体的程序运行的环境,可以得到一个大致的数量阶数。一般顺序:O(1) < O(log n) < O(n) < O(nk) < O(kn)
将算法中基本操作的执行次数作为算法时间复杂度的度量。多数情况下都是取最深层循环的语句所描述的操作作为基本操作。递归算法的时间复杂度只需要记忆那几个常用递归算法的即可。
如何算:
(1)确定算法中的基本操作以及数据量的大小(由算法所涉及的局部来看)。
(2)基本操作执行情况计算出规模n的函数f(n),并确定时间复杂度为T(n) = O(f(n))中增长最快的项/此项的系数。
空间复杂度:类似的算法所需储存空间 S(n) = O( f(n) ),n为问题的规模
二.线性表
1.何谓线性结构
在数据元素的非空有限集中,存在唯一的一个称为“第一个”的数据元素,存在唯一的一个称为“最后一个”的数据元素,除第一个之外,其他每个数据元素只有一个前驱,除最后一个之外,其他每个数据元素都只有一个后继。
2.线性结构主要有哪几种
线性表、栈、队列、串
3.线性表ADT,尤其是它的几个主要操作(插入元素、删除元素)
ADT List
{ 数据对象 Data={ ai , i=1,2,…n }
数据关系 (略)
数据操作:
InitList 初始化线性表
DestroyList 销毁线性表
ClearList 清空线性表内的元素
ListEmpty 判断是否为空表
ListLength 取得表长度
GetElement 取得某个位置的元素
LocateElement 定位某个元素的位置
PriorElement 返回某个元素的前驱
NextElement 返回某个元素的后继
InsertElement 在某位置插入一个元素
DeleteElement 在某位置删除一个元素
ListTraverse 遍历整个线性表
}
4.线性表的两种实现方式
顺序表、链表
5.顺序表的C语言实现
#ifndef ARRAY_LIST_H
#define ARRAY_LIST_H
#include "type.h"
array_list.c
#include
#include
#include "array_list.h"
static int get_array_index_from_logic_index(int logic_index) // 从逻辑下标得到数组下标
{ return logic_index - 1;}
static int get_logic_index_from_array_index(int array_index) // 从数组下标得到逻辑下标
{return array_index + 1;}
static int is_full(const struct array_list * list) // 是否满了
{return list->capacity == list->last;}
struct array_list * array_list_init(int capacity) // 初始化
{struct array_list * tmp = (struct array_list *) malloc(sizeof (struct array_list));
if (tmp == NULL)
{perror("顺序表创建失败\n"); return NULL;}
type * data = (type *) malloc(sizeof (type) * capacity);
if (data == NULL)
{perror("顺序表创建失败\n"); return NULL; }
tmp->capacity = capacity;
tmp->data = data;
tmp->last = 0;
return tmp;}
void array_list_destroy(struct array_list * list) // 销毁
{free(list->data); free(list);}
int array_list_is_empty(const struct array_list * list) // 判空
{return list->last == 0; }
void array_list_clear(struct array_list * list) // 清空
{list->last = 0; }
int array_list_length(const struct array_list * list) // 求长度
{return list->last;}
type array_list_get(const struct array_list * list, int index) // 取得index位置的元素
{return list->data[get_array_index_from_logic_index(index)];}
void array_list_insert(struct array_list * list, int index, type data) // 在index位置添加元素
{if (is_full(list)) {perror("表是满的"); return;}
if (index < 1 || index > array_list_length(list) + 1) {perror("下表超出范围"); return; }
int array_index = get_array_index_from_logic_index(index);
int i = list->last - 1;
for (; i >= array_index; i--) {list->data[i + 1] = list->data[i];}
list->data[i + 1] = data; list->last++;}
void array_list_insert_last(struct array_list * list, type data) // 在最后位置添加元素
{array_list_insert(list, array_list_length(list) + 1, data);}
type array_list_remove(struct array_list * list, int index) // 删除index位置的元素
{if (index < 1 || index > array_list_length(list)) {perror("index out of range"); return null_value();}
int array_index = get_array_index_from_logic_index(index);
type data = list->data[array_index];
for (int i = array_index + 1; i < list->last; i++) {list->data[i - 1] = list->data[i];}
list->last--;
return data;}
void array_list_traverse(const struct array_list * list, void (*access_function)(type data)) //遍历
{for (int i = 0; i < list->last; i++) {access_function(list->data[i]);}}
6.链表的C语言实现
单链表、循环链表、双向链表中结点的操作,比如插入、删除、查找、定位等等
7.实现的代码中,如何方便地更换数据元素的类型,以便代码复用
定义泛型泛型的定义主要有以下两种:
1.在程序编码中一些包含类型参数的类型,也就是说泛型的参数只可以代表类,不能代表个别对象。(这是当今较常见的定义)
2.在程序编码中一些包含参数的类。其参数可以代表类或对象等等。(人们大多把这称作模板)不论使用哪个定义,泛型的参数在真正使用泛型时都必须作出指明。
8.顺序表和链表的优缺点、适用场景
顺序实现的优点:
1.利用计算机存储的特点,实现简单,无需额外存储元素间的逻辑关系
2.随机存储
顺序实现的缺点:
1.插入和删除操作常伴随大量的数据元素的移动
2.不利于数据规模的经常增大
a.对于预先分配空间的,无法增加空间
b.对于有自动扩充功能的,扩充之前需大 量复制已有数据元素
因此,顺序存储适合表示那些预先知道数据元素规模,经常的操作是取得某个位置的元素,但不经常进行插入删除操作的那些问题
链式实现的优点:
1.无需事先分配大块连续的内存,可根据插入删除的需要进行内存的分配和回收
2.插入和删除操作比较简单,无需进行数据的移动
链式实现的缺点
1.需要额外存储指针域
2.实现时相对比较复杂
适用场景:需要插入或删除操作较多的地方
三.栈与队列
1.栈的概念,ADT
栈(stack)是限定仅能在表尾进行插入和删除操作的线性表。
(因此,对栈来说,表尾端有 其特殊含义,称为栈顶,相应地,表头端称为栈底。不含元素的空表称为空栈)
ADT Stack{ 数据元素:
略
基本操作:
InitStack
DestroyStack
ClearStack
StackEmpty
GetTop
Push
Pop }
2.栈的三个基本操作
Push、Pop、GetTop
void push(struct stack * st, type data) // 进栈
{if (st->top == st->capacity)
{perror("stack is full, cannot push");return; }
st->data[st->top++] = data; }
type pop(struct stack * st) // 出栈
{if (stack_is_empty(st)) {perror("stack is empty, cannot pop"); return null_value();}
return st->data[--st->top]; }
type get_top(const struct stack * st) // 查看栈顶元素
{if (stack_is_empty(st)) {perror("stack is empty, got null"); return null_value();}
return st->data[st->top - 1]; }
3.栈的特点
先进后出(FILO)
4.判断能否根据某种操作顺序,得到某一个元素序列
具体分析
5.栈的一些应用:括号匹配、函数调用跟踪、递归跟踪、四则运算
括号匹配思路:
1.依次读入每一个符号。
2.如果该符号是左括号(大、中、小),则入栈;
3.如果该符号是右括号,则出栈一个符号,如果与该左括号匹配,则继续;如果栈空或者取出的左括号不匹配,则判定表达式括号不匹配。
4.读完表达式,如果栈空,判定表达式括号匹配;如果栈非空,则判定表达式括号不匹配
#include
#include
#include
#include
#define TURE 1
#define ERROR 0
#define OK TURE
using namespace std;
const int max = 10000;
char ch[max];
typedef int Status;
struct Stack //创建栈
{ char data[max];
int top; };
Status search(char ch[], int n)
{ Stack s;
s.top = -1;
for (int i = 0; i < n; i++)
{if (ch[i] == '(' || ch[i] == '[')
s.data[++s.top] = ch[i];
if (ch[i] == ')')//左括号
{if (s.top == -1) return ERROR;
else if (s.data[s.top] == '(')
s.top--;
else return ERROR; }
if (ch[i] == ']') //右括号
{if (s.top == -1) return ERROR;
else if (s.data[s.top] == '[')//匹配s.top--;
else//匹配失败return ERROR;
//处理完所有的括号查看栈是否为空
while (i == n)
{if (s.top == -1) {return OK; cout << "s.top is cleaned";}
else{return ERROR; cout << "s.top is uncleaned";}}
}}}
int main()
{ int n;//检测个数
cout << "要检测的个数:";
cin >> n;//输入n个数
int i = 1;//设置i=1
while (n--)//当n自减的时候
{cout << "第" << i << "个括号序列:"; cin >> ch;//输入括号
int len = strlen(ch);//把ch的字符长度赋值给Len
if (search(ch, len)) cout << "匹配成功" << endl;
else cout << "匹配失败" << endl; i++;}
system("pause");
return 0; }
四则运算思路:
1.设计算法将中序表达式转换成后序表达式
2.设计算法进行后序表达式的计算
跟踪思路:调用一次则入栈一次,
6.栈的顺序实现方式(top指针的变化方式)
#include
#include
#include "stack.h"
struct stack * stack_init(int capacity)
{struct stack * st = (struct stack *) malloc(sizeof (struct stack));
if (!st) {perror("stack init failed"); return NULL; }
st->data = (type *) malloc(sizeof (type) * capacity);
if (!st->data) {perror("stack init failed");return NULL; }
st->capacity = capacity;
st->top = 0; return st;}
7.队列的特点:FIFO
8.队列的头尾与两个基本操作
队首(front),队尾(rear),入队(en),出队(de)
void enq(struct queue * q, type data) // 入队列
{if (is_full(q)) {perror("queue is full, cannot enq"); return; }
if (queue_is_empty(q)) { q->data[q->front] = data; }
q->data[q->rear] = data; q->rear = move_to_next(q->rear, q->length); }
type get_front(const struct queue * q) // 取得队列首元素
{if (queue_is_empty(q)) { perror("queue is empty"); return null_value(); }
return q->data[q->front];}
type deq(struct queue * q) // 出队列
{type o = get_front(q);
q->front = move_to_next(q->front, q->length); return o; }
9.队列的顺序实现方式
front和rear的变化方式,何时表示队列满,何时表示队列空
int queue_is_empty(const struct queue * q) //判空
{return q->front == q->rear;}
static int is_full(const struct queue * q) // 判断队列满
{return move_to_next(q->rear, q->length) == q->front; }
一般规定:
当front与rear重合时,表示队列为空
当rear再移动一位就将与front重合时,表示队列满(即以牺牲一个存储单元的代价,表示队列满这个状态)
四.串
1.串的概念(串、子串)
串是由零个或多个字符组成的有限序列,如 str = ‘abcdefg’,str:串名,单引号中的部分,表示串值,串值中的字符个数,为串长度,长度为0的表示空串
串中的任意连续的字符子序列,称为该串的子串
当两个串的值完全相同时,称这两个串相等
2.串的顺序实现方式,主要操作
ADT String{ 数据对象:略
数据关系:略
基本操作:StrInit:串初始化
StrCopy:串拷贝
StrCompare:串比较
StrLength:求串长度
StrConcat:串连接
SubString:求串的某个子串
StrIndex:返回子串在串中的位置 }
3.模式匹配问题
模式匹配:在一个串中,对某子串的位置定位
假设主串长度为n,要匹配的子串长度为m,则常规匹配算法最坏情况下需要O(n*m)时间完成
KMP算法:该算法由Knuth,Morris,Pratt同时发现,因此命名为KMP算法,它是一种巧妙的算法,能在O(n+m)时间内完成串的模式匹配
五.数组与广义表
1.数组(Array) 是用来描述一组具有连续的线性关系的相同事物的概念
2.ADT Array{ 数据对象:(略)
数据关系:(略)
基本操作:
InitArray 数组初始化
DestroyArray 数组销毁
GetValue 取得某个元素
SetValue 给某个元素赋值 }
数组可以是多维的,不同的维数用来表示不同的实际事物
比如:可以用一维数组表示向量,二维数组表示矩阵,等等
3.多维数组的定义方法
通过其前一维数组来定义
比如,int a[3][4],可以认为首先是一个具有3个元素(长度为3)的数组,然后每个元素是一个长度为4的数组
即:n维数组可以这样认为:它是一个1维数组,其每个元素是一个n-1维数组
4.数组的顺序实现
数组的基本元素在开始初始化后,一般其位置不会发生变化,数组的基本操作是取值和赋值,很少进行插入和删除操作,内存的使用特性符合数组的这种特点。故数组一般都采用顺序实现
5.两种保存数组的方法
1.按行存储2.按列存储
6.多维数组中基本元素的定位:
每一个多维数组中的基本元素,都会有一个坐标,只要知道数组第一个元素的位置(基地址),某一个元素的坐标,就能够计算出该元素的位置
7. 二维数组元素坐标
(以行存储为例):LOC(i, j) = LOC(0, 0) + (d2 * i + j)*L
其中d2为第二维的数组长度,L为每个基本元素所占的空间。由于有这样的计算公式,因此顺序实现的数组具有随机存取的特点。
8.矩阵的压缩存储
(1)存一半:对称矩阵(ai,j = aj,i),上、下三角矩阵(ai,j = 0, i>j 或反过来)
(2)存坐标:稀疏矩阵(矩阵中大部分元素为0)
9.广义表
广义表是一种特殊的线性表,他的每一个元素可以是一个基本元素,也可以是另外一个不定长的线性表,这种性质决定了广义表不太可能用顺序存储来实现,因此一般用链表来实现。
六.树
1.树的定义(递归方式)及表示方法
定义:树是n(≥0)个结点的有限集,树为:
空树 或者 非空树:有且仅有一个称为“根”(root)的结点,它有若干个子树
树的几种表示方法:
双亲表示法:尽管树的每个结点都有若干个孩子,但是每个孩子只有一个双亲。(该方法在求某结点的孩子时,需要花费较多的时间)
孩子表示法:
孩子兄弟表示法:对每个结点,设定两个指针,分别记录该结点的第一个孩子和下一个兄弟
2.树的主要术语(结点、叶子结点、孩子、兄弟、双亲、结点的度、树的度、层次、深度、有序树、森林)
结点(node):包含一个数据元素及指向其子树的分支
结点的度(Degree):一个结点拥有的子树的个数
叶子结点(Leaf):度为0的结点
树的度:树内各个结点的度的最大值
孩子(Child):结点的子树
双亲(Parent):结点的上层结点
兄弟(Sibling):具有同一个双亲的结点
祖先:从根到该结点所经过的分支上的所有结点
子孙:某结点的子树的所有结点
层次(Level):根为第一次,某个结点若为l层,则其孩子为l+1层
深度(Depth):树中结点的最大层次
有序树:各子树从左往右有顺序,反之称为无序树
森林(Forest):若干棵互不相交的树的集合
3.二叉树(Binary Tree)的概念(递归方式)
定义1:每个结点至多只有两棵子树的树(即二叉树中不存在度大于2的结点),并且两棵子树有左右之分,分别称为左子树和右子树
定义2:二叉树或为一棵空树,或是由一个根结点加上一棵左子树和一棵右子树,左子树和右子树也为二叉树。
4.二叉树的几个性质
1.在二叉树的第i层上至多有2i-1(i≥1)个结点
2.深度为k的二叉树至多有2k-1(k≥1)个结点
3.任何一棵二叉树T,如果其终端结点数为n0,度为2的结点数为n2,则n0=n2+1
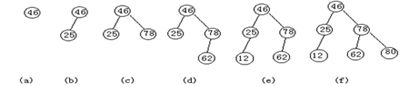
4.具有n个结点的完全二叉树的深度为(log2n)+1
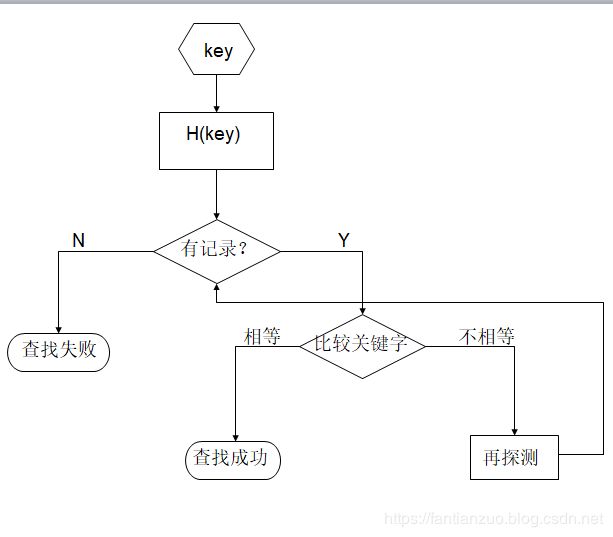
5. 如果对一棵有n个结点的完全二叉树(其深度为(log2n)+1)的结点按层序编号(从第1层到第(log2n)+1)层,每层从左到右),则对任一结点i(1 (1).如果i=1,则结点i是二叉树的根,无双亲;如果i>1,则双亲是结点(i/2) (2).如果2i>n,则结点i无做孩子(结点i为叶子结点);否则其左孩子 是结点2i+1 (3).如果2i+1>n,则结点i无右孩子;否则其右孩子是结点2i+1 5.二叉树的链式实现,几个重要操作的实现(插入结点、删除结点) #include "bintree.h" #include "queue.h" #define max(a,b) ((a)>(b)?(a):(b)) struct bintree * bintree_init()// 初始化 {struct bintree * p = (struct bintree *) malloc(sizeof (struct bintree)); if (!p) {perror("bintree init failed"); return NULL; } p->root = NULL; return p; } static int is_leaf(const struct tree_node * node)//创建叶子节点 {return node->left == NULL && node->right == NULL;} struct tree_node * insert_root(struct bintree * tree, type data) // 在空树上插入第一个结点 {if (!bintree_is_empty(tree)) { perror("tree is not empty"); return NULL; } struct tree_node * p = create_node(data); tree->root = p; return p; } static int has_left_child(const struct tree_node * node) {return node->left != NULL; } static int has_right_child(const struct tree_node * node) {return node->right != NULL; } struct tree_node * insert_left(struct tree_node * node, type data) // 插入左孩子 {if (has_left_child(node)) // 判断是否有左孩子 { perror("node already has left child"); return NULL; } struct tree_node * p = create_node(data); node->left = p; p->parent = node; return p; } struct tree_node * insert_right(struct tree_node * node, type data) // 插入右孩子 {if (has_right_child(node)) // 判断是否有右孩子 { perror("node already has right child"); return NULL; } struct tree_node * p = create_node(data); node->right = p; p->parent = node; return p; } struct tree_node * remove_root(struct bintree * tree) // 删除根结点 { if (!is_leaf(tree->root)) { perror("root is not leaf"); return NULL; } struct tree_node * p = tree->root; tree->root = NULL; return p; } static int is_left_child(const struct tree_node * child, const struct tree_node * parent) {return child->parent == parent && parent->left == child; } static int is_right_child(const struct tree_node * child, const struct tree_node * parent) {return child->parent == parent && parent->right == child; } struct tree_node * bintree_remove(struct tree_node * node) // 删除结点 { if (!is_leaf(node)) {perror("node is not leaf"); return NULL; } struct tree_node * parent = node->parent; if (parent) { if (is_left_child(node, parent)) {parent->left = NULL; } else if (is_right_child(node, parent)) {parent->right = NULL;} node->parent = NULL; } return node; } 6.四种二叉树遍历方法(前序、中序、后序、层次) static void exec_pre_recurse(const struct tree_node * node, void (*access_function)(type data)) { if (node == NULL) { return; } access_function(node->data); exec_pre_recurse(node->left, access_function); exec_pre_recurse(node->right, access_function); } void pre_order_traverse(const struct bintree * tree, void (*access_function)(type data)) // 前序遍历 { exec_pre_recurse(tree->root, access_function); } static void exec_in_recurse(const struct tree_node * node, void (*access_function)(type data)) { if (node == NULL) { return; } exec_in_recurse(node->left, access_function); access_function(node->data); exec_in_recurse(node->right, access_function); } void in_order_traverse(const struct bintree * tree, void (*access_function)(type data)) // 中序遍历 {exec_in_recurse(tree->root, access_function); } static void exec_post_recurse(const struct tree_node * node, void (*access_function)(type data)) { if (node == NULL) { return; } exec_post_recurse(node->left, access_function); exec_post_recurse(node->right, access_function); access_function(node->data); } // 后序遍历 void post_order_traverse(const struct bintree * tree, void (*access_function)(type data)) { exec_post_recurse(tree->root, access_function); } struct bintree_queue_node // 层次遍历用到的队列,简单一点利用链表实现 {struct tree_node * data; struct bintree_queue_node * next; }; struct bintree_queue {struct bintree_queue_node * front; struct bintree_queue_node * rear; }; static struct bintree_queue * bintree_queue_init() { struct bintree_queue * q = (struct bintree_queue *) malloc(sizeof (struct bintree_queue)); if (!q) { perror("bintree queue init failed"); return NULL; } q->front = NULL; q->rear = NULL; return q; } static int bintree_queue_is_empty(const struct bintree_queue * q) // 判空 { return q->front == NULL && q->rear == NULL; } static void bintree_enq(struct bintree_queue * q, struct tree_node * data) // 入队列 {struct bintree_queue_node * p = (struct bintree_queue_node *) malloc(sizeof (struct bintree_queue_node)); if (!p) { perror("bintree queue init failed"); return; } p->data = data; p->next = NULL; if (bintree_queue_is_empty(q)) { q->front = p; q->rear = p; } else { p->next = q->rear->next; q->rear->next = p; q->rear = p; } } static struct tree_node * bintree_deq(struct bintree_queue * q) // 出队列 { if (bintree_queue_is_empty(q)) { perror("bintree queue is empty"); return NULL; } struct bintree_queue_node * o = q->front; q->front = o->next; if (q->front == NULL) { q->rear = NULL; } return o->data; } void level_order_traverse(const struct bintree * tree, void (*access_function)(type data)) // 层次遍历 { struct bintree_queue * q = bintree_queue_init(); bintree_enq(q, tree->root); while (!bintree_queue_is_empty(q)) { struct tree_node * node = bintree_deq(q); access_function(node->data); if (has_left_child(node)) { bintree_enq(q, node->left); } if (has_right_child(node)) { bintree_enq(q, node->right); } } } 7.给定前序和中序、中序和后序的遍历序列,画出树的形状 前序:访问根结点→先序遍历左子树→先序遍历右子树 中序:中序遍历左子树→访问根结点→中序遍历右子树 后序:后序遍历左子树→后序遍历右子树→访问根结点 (前中后:根的位置) 层次:依次访问深度为1、2、…的结点,从上至下,从左至右 8.满二叉树与完全二叉树 满二叉树:深度为k,且有2k-1个结点 完全二叉树:深度为k,有n个结点的二叉树,与深度为k的满二叉树中编号从1至n的结点一一对应(叶子结点只存在于最大的两个层次上面) 9.如何把任意一棵树、森林转化为一棵等价的二叉树 森林是含有若干棵独立的树的一个集合,把森林中某棵树的根结点看成是前一棵树的根结点的兄弟,则可将该森林转换成一棵二叉树 10.Huffman树、如何构造 路径:树中一个结点到另一个结点间的分支 路径长度:路径中的分支数目 树的路径长度:从树根到每一个叶子结点的路径长度之和 树的带权路径长度:树中所有叶子结点的带权路径长度之和 WPL = ∑wk·lk , k = 1, …, n Huffman树(最优二叉树):设有n个权值{w1,w2,…wn},某二叉树共有n个叶子结点,每个结点的权值分别为wi,则带权路径长度最小的二叉树称为Huffman树(最优二叉树)。 Huffman树的应用:二叉树由于只有两个分支,可以用来代表判定语句中的true和false 树的应用之一就是条件分支的判定,对于有些问题需要经常进行条件判断,则若采用合适的树(如Huffman树,则可以减少在树中走的路径长度) 构造Huffman树的算法: 1.已知n个结点与各自的权值 2.以这n个结点分别作为一个只有一个根结点的树,组成集合F 3.在集合F中,寻找根结点权值最小的两棵树,构造一个新的根结点,把这两棵树分别作为该新结点的左右孩子,新的根结点的权值为两子树根结点的权值之和 4.在集合F中加入新的树,并删除原来的两棵树 5.重复第二步和第三部,直到最后只剩下一棵树,该树即为Huffman树 11.Huffman编码构造方法 前缀编码:任一字符的编码都不是另一个字符编码的前缀,如给A、B、C、D分别编码为0,00,1,01,则字符串ABCAD的编码字符串为0001001,但反过来译码时,就会产生歧义,因为该编码方式非前缀编码 设计一棵树,把各个字符看做树的叶子结点,各个字符出现的频率看做结点的权值,每个结点到其左孩子的路径为0,到右孩子的路径为1,则前缀编码的构造就是一个构造Huffman树的过程,而译码就是遍历整个Huffman树的叶子结点的过程 12.树的非递归算法 push(root) while(栈非空) node←pop PreOrder: if(not_visited(node.right)) push(node.right) if(not_visited(node.left)) push(node.left) if(should_visit(node)) visit(node) else push(node) InOrder: if(not_visited(node.right)) push(node.right) if(should_visit(node)) visit(node) else push(node) if(not_visited(node.left)) push(node.left) PostOrder: if(should_visit(node)) visit(node) else push(node) if(not_visited(node.right)) push(node.right) if(not_visited(node.left)) push(node.left) should_visit(node) PreOrder: return TRUE InOrder: 左孩子为空或左孩子已被访问过 return TRUE,否则 return FALSE PostOrder: 左孩子为空或左孩子已被访问过 &&右孩子为空或右孩子已被访问过 return TRUE,否则 return FALSE 1.查找表、关键字、主关键字、次关键字、查找、平均查找长度(ASL) 查找表(Search Table):需要被查找的数据所在的集合,通常是同一类型的数据元素(记录)构成的集合 关键字(Key):数据元素中的某个数据项的值 主关键字(Primary Key):该关键字可以唯一地标识一个记录 次关键字(Secondary Key):不是可以唯一标识一个数据元素的关键字 查找(Searching):根据给定的值,在查找表中确定一个其关键字等于给定值的数据元素(记录)。若存在这样的记录,则称为查找成功,反之称为不成功 平均查找长度(Average Search Length):为确定记录在查找表中的位置,需和给定值进行比较的关键字个数的期望值。 2.静态查找表与动态查找表的区别 静态查找表:只做查找操作,查询某个特定的数据元素是否在表中,检索某个特定的数据元素和各种属性 动态查找表:在查找过程中同时还插入或删除元素 3.折半查找算法 折半查找(Binary Search),也叫“二分法查找”。给定一个有序的数据集,依次比较区间中间位置元素的关键字与给定值,若相等则查找成功,若不等,则把范围缩小一半,继续以上步骤,直到找到,或者区间长度小于0(未找到)。 算法描述(从a[N]中查找是否有元素b) 1.设立三个指标low、high、mid,初值分别为low=0,high=N-1 2.如果low>high,则表示未找到b 3.如果low>=high,则令mid=(low+high)/2,比较b与a[mid]是否相等,如相等则表示找到该数据,如果b>a[mid],令low=mid+1,如果b 4.重复2、3步骤 性能:假设有序表有n个元素,则折半查找在查找成功时进行比较的次数最多为log2n+1 int binary_search(type data[], int length, type key) { int start = 0; int end = length - 1; while (start <= end) { int mid = (start + end) / 2; int result = compare(data[mid], key); if (!result) { return mid; } else if (result > 0) { end = mid - 1; } else { start = mid + 1; } } return -1; } 4.二叉排序树(Binary Sort Tree) 定义:二叉排序树或者是一棵空树,或者具有以下性质: 1、若其左子树非空,则左子树的所有结点的值均小于根结点的值; 2、若右子树非空,则右子树的所有结点的值均大于根结点的值; 3、左右子树同时也是二叉排序树 struct binary_sorted_tree * bst_init()// 初始化 { struct binary_sorted_tree * p = (struct binary_sorted_tree *) malloc(sizeof (struct binary_sorted_tree)); if (!p) { perror("bst init failed"); return NULL; } p->root = NULL; return p; } static int is_leaf(const struct tree_node * node) { return node->left == NULL && node->right == NULL; } static struct tree_node * exec_clear_recurse(struct tree_node * node) { if (node == NULL) { return NULL; } node->left = exec_clear_recurse(node->left); node->right = exec_clear_recurse(node->right); free(node); return NULL; } 5.根据一串输入数据,如何构造二叉排序树 插入的过程与查找类似,只是当查找不成功时,根据关键字与根结点的大小,将新结点(关键字的值)插入根结点的相应孩子的位置(左或者右) 例题:设有一个输入数据的序列是 { 46, 25, 78, 62, 12, 80 }, 试画出从空树起,逐个输入各个数据而生成的二叉排序树。 6.如何在二叉排序树中进行查找 1.若根结点为空,则表示查找失败 2.若根结点非空,比较关键字与根结点的大小,若相等,则表示查找成功 3.若根结点的值小于关键字的值,则递归查找右子树,若根节点的值大于关键值,则递归查找左子树 7.二叉排序树的性能取决于什么 二叉排序树查找关键字的比较次数,等于该结点所在的层次数(查找成功),若查找不成功,其比较次数最多为树的深度。对于一棵具有n个结点的树来说,其深度介于㏒2n+1与n之间。所以排序二叉树的形态对于查找效率至关重要,或者说,一棵排序二叉树不一定就能提高查找的速度,而是要看这棵树的形态。 8.二叉平衡树的概念、平衡因子 二叉平衡树(平衡二叉树,或AVL树):它或是一棵空树,或者是具有以下性质的二叉树:它的左右子树都是平衡二叉树,且左子树和右子树的深度之差的绝对值不超过一。 深度为O(㏒2n) 平衡因子(Balance Factor,BF):某结点的左子树深度减去右子树深度。对于一棵平衡二叉树,每个结点的平衡因子的取值只可能是-1、0或者1 9.二叉平衡树有什么好处 平衡二叉树(Balanced Binary Tree) 如果能构造出一棵左右子树相对“均衡”的树,则树的深度就会比较小,就能体现出树的良好性质,查找效率高。 10.四种旋转方式、各用在什么情况下 单向右旋(顺时针):当在p的左子树根结点的左子树上插入结点导致不平衡时 单向左旋(逆时针):当在p的右子树根结点的右子树上插入结点导致不平衡时 先左后右:当在p的左子树根结点的右子树上插入结点导致不平衡时 先右后左:当在p的右子树根结点的左子树插入结点导致不平衡时 11.描述下如何在二叉平衡树中插入一个新节点 平衡二叉排序树T上插入一个新元素e的算法: 1.若T为空树,则插入新元素e作为根结点 2.若T的根结点关键字等于e的关键字,则不做任何操作 3.若e的关键字小于T的根结点的关键字,则在T的左子树上递归插入e,然后检查下T的根结点的平衡因子,若平衡因子大于1或者小于-1,则根据上面四种情况之一进行调整 4.若e的关键字大于T的根结点的关键字,则在T的右子树上做类似3中的操作 12.二叉平衡树中的查找的时间复杂度 对于二叉排序树,其最大查找次数取决于树的最大深度,含有n个结点的平衡二叉树的最大深度是O(㏒2n),因此平衡二叉排序树的性能为O(㏒2n)。 13.哈希函数 f : 关键字 → 存储位置,即,hash函数是指关键字与存储位置(哈希地址)的对应关系,只要给出关键字,就可以通过这个函数得到存储位置 14.哈希表的定义 如:给定一个保存了10个元素的数组,[ 10, 31, 22, 133, 254, 65, 16, 47, 98, 2009 ],你会用什么方法去查找表里的某一个元素 如果给定Hash函数f(K)=K mod 10,就可以立刻得到该键值对应元素的数组下标 如果给定Hash函数f(K)=K mod 2,则虽然也缩小了查找范围,但达不到上面函数的效果 哈希表:根据设定的哈希函数H(key)和处理冲突的方法,将一组关键字映射到一个有限的连续的地址区间上,并以关键字的映像作为记录在表中的存储位置 映射过程称为哈希造表,或者散列(哈希表有时也叫散列表) 所得的存储位置值称为哈希地址或者散列地址 15.几种哈希函数的构造算法 1.直接定址法:取关键字或者关键字的某个线性函数作为Hash地址,即:H(key) = key, H(key) = a×key + b,a、b为常数 2. 数字分析法 3.平方取中法 4.折叠法 5.除留余数法(取模): 把关键字对某个数p(不大于哈希表的表长m)取模,所得到的数作为哈希地址 H(key) = key MOD p, p≤m (p值的选取,影响到散列之后的效果) 6. 随机数法:H(key) = random(key),把产生的随机数做为哈希地址 16.冲突、处理冲突的基本思想 冲突:如果不同的键值,被Hash函数映射到同样的一个哈希地址,则称为冲突。Hash函数不可能完全避免冲突,只可能尽量减少冲突,或者说,好的Hash函数能将关键字映射后得到的哈希地址,尽量均匀地分布 处理冲突的基本思想:在处理的过程中,可能会得到一个地址序列Hi,i = 1,2,…,k,即每次得到一个哈希地址Hi,若仍然发生了冲突,则再由相应方法得到下一个哈希地址Hi+1,直到得到一个不发生冲突的哈希地址为止 17.几个处理冲突的方法(开放地址法、再哈希法、链地址法) 1.开放定址法:处理冲突函数为Hi = (H(key) + di) MOD m, i = 1,2, …,k, k ≤ m-1,H为哈希函数,m为哈希表的表长,di为增量序列。di的选取方法: di=1,2,3, …,m-1,称为“线性探测再散列” di=12,-12,22,-22, …,k2,-k2, k≤m/2,称为“二次探测再散列” di为伪随机数序列,称为“伪随机探测再散列” 2.再哈希法:Hi = RHi(key), i = 1,2, …, k, RHi为不同的一些哈希函数 3.链地址法:将所有冲突的记录存储在一个线性链表中,哈希函数得到的地址中保存这个链表 18.哈希表中查找一个元素的过程 哈希表的查找过程与构造过程基本一致,在查找过程中,利用哈希函数和冲突函数,直到查找失败或者查找成功 影响哈希函数比较次数的因素: 1.哈希函数 哈希函数的好坏,影响出现冲突的频繁程度。一个均匀的哈希函数,对一组关键字,产生的冲突可能性都相同,它不是影响ASL的决定性因素 2.冲突处理方法 针对所介绍的几个冲突处理方法(线性探测、二次探测、随机探测、再探测、链地址),各自的ASL不同 3.装填因子(衡量哈希表的装满程度)影响该哈希表的ASL α(装填因子)= 表中记录数/哈希表长度 装填因子越小,发生冲突的可能性就越小,反之就越大(需比较的次数就越多) 1.排序的基本概念、稳定、内部排序与外部排序 2.简单排序方法有:插入排序、冒泡排序、选择排序 3.先进排序方法有:shell排序、快速排序、堆排序、归并排序 4.基数排序 5.Shell排序中的步长序列(或增量因子)、shell排序的大致过程,shell排序快慢的关键 6.快速排序中:支点(pivot),算法的基本过程,快慢取决于什么 7.会使用C库中自带的快速排序函数qsort 8.什么叫做堆(注意与排序树的差别)、最大堆、最小堆 9.描述堆排序的过程(两个主要过程),每一次建堆之后会形成什么状态 10.何为归并,归并排序的主要过程 11.多关键字排序 12.MSD方法与LSD方法 13.基数排序的过程(详细描述)(分配与收集) 14.各种排序方法的比较(最坏、平均时间复杂度、所需辅助空间、稳定与否) 待续七.查找
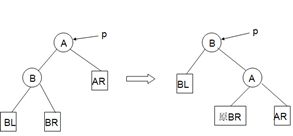
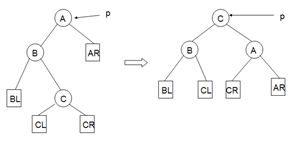
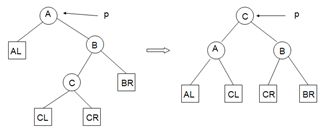
平衡二叉树的结点旋转(以p指向由于插入结点导致不平衡的最小子树的根)八.排序