使用python绘制3维正态分布图
今天使用python画了几个好玩的3D展示图,现在分享给大家。先贴上图片
使用的python工具包为:
from matplotlib import pyplot as plt
import numpy as np
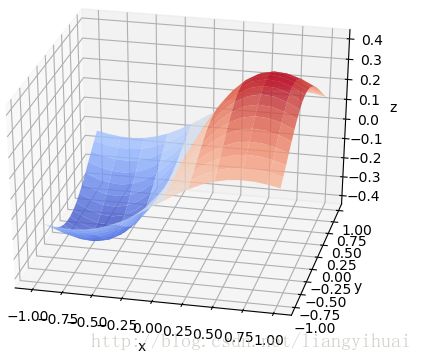
from mpl_toolkits.mplot3d import Axes3D在贴代码之前,有必要从整体上了解这些图是如何画出来的。可以把上面每一个3D图片理解成一个长方体。输入数据是三维的,x轴y轴和z轴。在第三个图片里面有x、y和z坐标的标识。在第三张图片中,我们可以理解为,z是随着x和y变化的函数。就像一个人在山丘地区走动一样,其中x和y表示的是方向,z表示的这个人在上坡还是下坡。第二张图片的中间那个,其实是一个3维的正态分布图。具体的公式为:

上面的是2维的,即只有x和y,如果是三维的话,需要一点变形,只需要在上面的公式基础之上把exp()里面改变为:exp(-((x-u)^2 + (y - u)^2)/(2q^2)), 这里的u表示平均值,q表示标准差。这样变化之后,z = f(x, y)。这就是z值的公式了,表示的是z值随着x和y值的变化而变化的函数。
下面贴一下代码
这是第二张图片的代码。
from matplotlib import pyplot as plt
import numpy as np
from mpl_toolkits.mplot3d import Axes3D
fig = plt.figure()
ax = Axes3D(fig)
len = 8;
step = 0.4;
def build_layer(z_value):
x = np.arange(-len, len, step);
y = np.arange(-len, len, step);
z1 = np.full(x.size, z_value/2)
z2 = np.full(x.size, z_value/2)
z1, z2 = np.meshgrid(z1, z2)
z = z1 + z2;
x, y = np.meshgrid(x, y)
return (x, y, z);
def build_gaussian_layer(mean, standard_deviation):
x = np.arange(-len, len, step);
y = np.arange(-len, len, step);
x, y = np.meshgrid(x, y);
z = np.exp(-((y-mean)**2 + (x - mean)**2)/(2*(standard_deviation**2)))
z = z/(np.sqrt(2*np.pi)*standard_deviation);
return (x, y, z);
# 具体函数方法可用 help(function) 查看,如:help(ax.plot_surface)
x1, y1, z1 = build_layer(0.2);
ax.plot_surface(x1, y1, z1, rstride=1, cstride=1, color='green')
x5, y5, z5 = build_layer(0.15);
ax.plot_surface(x5, y5, z5, rstride=1, cstride=1, color='pink')
# x2, y2, z2 = build_layer(-0.26);
# ax.plot_surface(x2, y2, z2, rstride=1, cstride=1, color='yellow')
#
# x6, y6, z6 = build_layer(-0.22);
# ax.plot_surface(x6, y6, z6, rstride=1, cstride=1, color='pink')
# x4, y4, z4 = build_layer(0);
# ax.plot_surface(x4, y4, z4, rstride=1, cstride=1, color='purple')
x3, y3, z3 = build_gaussian_layer(0, 1)
ax.plot_surface(x3, y3, z3, rstride=1, cstride=1, cmap='rainbow')
plt.show()这是第三张图片的代码
import numpy as np
import matplotlib.pyplot as plt
import mpl_toolkits.mplot3d
x, y = np.mgrid[-1:1:20j, -1:1:20j]
z = x * np.exp(-x ** 2 - y ** 2)
ax = plt.subplot(111, projection='3d')
ax.plot_surface(x, y, z, rstride=2, cstride=1, cmap=plt.cm.coolwarm, alpha=0.8)
ax.set_xlabel('x')
ax.set_ylabel('y')
ax.set_zlabel('z')
plt.show()结束