8 Puzzle 算法原理和源码

8 puzzle 算法

算法根据Coursera上的算法课程实现

GitHub 地址

8 puzzle问题在18世纪70年代由Noyes Palmer提出，并逐渐流行起来。它由8个方块和一共空格组成3X3的格子。8个方块随机放置1-8个数字，目标是移动方块使得8个方块按顺序排放。具体如下：

   1  3        1     3        1  2  3        1  2  3        1  2  3
4  2  5   =>   4  2  5   =>   4     5   =>   4  5      =>   4  5  6
7  8  6        7  8  6        7  8  6        7  8  6        7  8 

initial        1 left          2 up          5 left          goal

算法实现

下面具体介绍了Java实现8 puzzle的过程。算法也可以用在4X4的解决中，但是如果问题需要40步以上才能解决时，会有内存溢出的情况，这需要算法进一步优化。

解决问题使用了A*搜索算法，首先定义了一个Board对象，用来描述NxN的一个数据结构。将初始节点放priority queue中，然后得到优先级最高的节点，并计算其相邻的节点，放入priority queue中，持续最高过程，直到节点与目标节点相同为止。

首先定义一个优先级函数：

• Hamming priority function：NxN格子中与预期位置不同的方块的数量。一般来说，具有错误数量方块越少的节点越接近预期的节点。
• Manhattan priority function：所有方块当前与预期位置距离的和。

举例：

8  1  3        1  2  3     1  2  3  4  5  6  7  8    1  2  3  4  5  6  7  8
4     2        4  5  6     ----------------------    ----------------------
7  6  5        7  8        1  1  0  0  1  1  0  1    1  2  0  0  2  2  0  3

initial          goal         Hamming = 5 + 0          Manhattan = 10 + 0

算法实现：

public Board(int[][] blocks) {
    this.blocks = blocks;
}

public int hamming() {

    if (hamming >= 0) {
        return hamming;
    }

    hamming = 0;
    for (int i = 0; i < blocks.length; i++) {
        for (int j = 0; j < blocks.length; j++) {
            if (blocks[i][j] != 0)
                hamming += i * blocks.length + j + 1 == blocks[i][j] ? 0 : 1;
        }
    }
    return hamming;
}

public int manhattan() {

    if (manhattan >= 0)
        return manhattan;

    manhattan = 0;
    for (int i = 0; i < blocks.length; i++) {
        for (int j = 0; j < blocks.length; j++) {
            if (blocks[i][j] != 0 && i * blocks.length + j + 1 != blocks[i][j]) {
                int ii = (blocks[i][j] - 1) / blocks.length;
                int jj = blocks[i][j] - ii * blocks.length - 1;
                manhattan += Math.abs(ii - i) + Math.abs(jj - j);
            }
        }
    }
    return manhattan;
}

// all neighboring boards
public Iterable neighbors() {

    if (neighbors != null) {
        return neighbors;
    }

    List boards = new ArrayList<>();
    int[][] newBolcks;

    for (int i = 0; i < blocks.length; i++) {
        for (int j = 0; j < blocks.length; j++) {
            if (blocks[i][j] == 0) {
                if (i > 0) {
                    newBolcks = exchange(i, j, i - 1, j);
                    boards.add(new Board(newBolcks));
                }
                if (i < blocks.length - 1) {
                    newBolcks = exchange(i, j, i + 1, j);
                    boards.add(new Board(newBolcks));
                }
                if (j > 0) {
                    newBolcks = exchange(i, j, i, j - 1);
                    boards.add(new Board(newBolcks));
                }
                if (j < blocks.length - 1) {
                    newBolcks = exchange(i, j, i, j + 1);
                    boards.add(new Board(newBolcks));
                }
                break;
            }
        }
    }
    neighbors = boards;
    return neighbors;

}
private int[][] exchange(int i1, int j1, int i2, int j2) {

    int[][] newBlocks = new int[blocks.length][blocks.length];
    for (int i = 0; i < blocks.length; i++) {
        for (int j = 0; j < blocks.length; j++) {
            newBlocks[i][j] = blocks[i][j];
        }
    }

    int temp = newBlocks[i1][j1];
    newBlocks[i1][j1] = newBlocks[i2][j2];
    newBlocks[i2][j2] = temp;
    return newBlocks;
}

在priority queue中可以使用 hamming 或者 manhattan 函数。

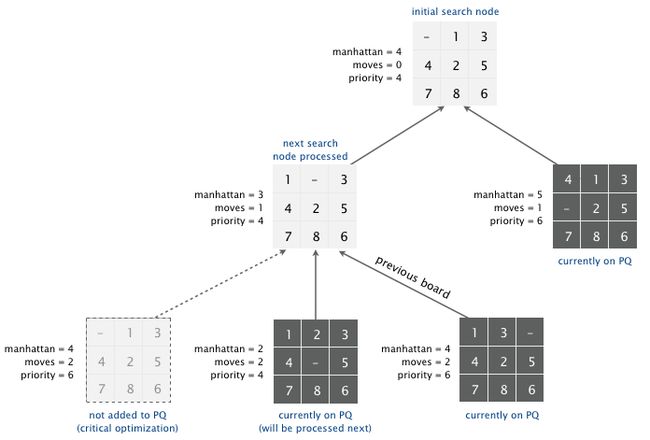
关键的优化：

在搜索树中寻找相邻节点（neighbors()函数得到的节点）的时候需要抛弃当前节点的父节点，否则让同一个节点不断地重复计算。

8  1  3       8  1  3       8  1       8  1  3     8  1  3
4     2       4  2          4  2  3    4     2     4  2  5
7  6  5       7  6  5       7  6  5    7  6  5     7  6

previous    search node    neighbor   neighbor    neighbor
                                     (disallow)

游戏树

将计算过程看作一共游戏决策树，每一个搜索节点都所谓树上的一个节点，树的孩子对应该节点的相邻搜索节点。内部的节点都已经北处理过，叶子节点则会被放入优先队列中，每一步都会从队列中拿出优先级最高的节点，并将它相邻节点放入游戏树和优先队列中。

定义游戏树节点：

class GameTreeNode implements Comparable {

    private Board board;
    private GameTreeNode father;
    private int moves;
    private int priority;

    private GameTreeNode(Board b, GameTreeNode father) {
        this.board = b;
        this.father = father;

        if (father != null) {
            this.moves = father.moves + 1;
            this.priority = this.board.manhattan() + this.moves;
        }
    }

    public GameTreeNode getFather() {
        return father;
    }

    public int getMoves() {
        return moves;
    }

    public void setMoves(int moves) {
        this.moves = moves;
        this.priority = this.board.manhattan() + this.moves;
    }

    public int getPriority() {
        return priority;
    }

    @Override
    public int compareTo(GameTreeNode o) {
        if (this.priority == o.priority) {
            return this.board.manhattan() - o.board.manhattan();
        }

        return this.priority - o.priority;
    }

}

求解过程：

public Solver(Board initial) {

    if (initial == null)
        throw new NullPointerException();

    int move = 0;

    GameTreeNode root = new GameTreeNode(initial, null);

    MinPQ mp = new MinPQ<>();
    mp.insert(root);

    GameTreeNode currNode = mp.delMin();

    while (!currNode.board.isGoal()) {

        for (Board b : currNode.board.neighbors()) {
            // don't enqueue a neighbor if its board is the same as the
            // board of the previous search node
            if (currNode.getFather() == null || !b.equals(currNode.getFather().board)) {
                GameTreeNode node = new GameTreeNode(b, currNode);
                mp.insert(node);
            }

        }

    }
}

无解的情况

并不是所有的情况都是可以解决的：

 1  2  3         1  2  3  4
 4  5  6         5  6  7  8
 8  7            9 10 11 12
                13 15 14 

unsolvable      unsolvable

对于不可解的Board，交换其中任意两个方块便可以得到有解的Board（不包括空格）。具体证明不懂。因此在世纪算法中需要同时解两个Board，一个是需要求解的，另一个是交换任意两个方块的Board，如果其中一个得到了求解的最终结果，那么另一个则是无解的。

结果

下面列出了4X4的求解过程：

 3  2  4  1 
 5  6  8  7 
 9 11 10 12 
13 14 15  0 

 3  2  4  1 
 5  6  8  7 
 9 11 10  0 
13 14 15 12 

 3  2  4  1 
 5  6  8  7 
 9 11  0 10 
13 14 15 12 

 3  2  4  1 
 5  6  0  7 
 9 11  8 10 
13 14 15 12 

 3  2  4  1 
 5  6  7  0 
 9 11  8 10 
13 14 15 12 

 3  2  4  0 
 5  6  7  1 
 9 11  8 10 
13 14 15 12 

 3  2  0  4 
 5  6  7  1 
 9 11  8 10 
13 14 15 12 

 3  2  7  4 
 5  6  0  1 
 9 11  8 10 
13 14 15 12 

 3  2  7  4 
 5  6  1  0 
 9 11  8 10 
13 14 15 12 

 3  2  7  4 
 5  6  1 10 
 9 11  8  0 
13 14 15 12 

 3  2  7  4 
 5  6  1 10 
 9 11  0  8 
13 14 15 12 

 3  2  7  4 
 5  6  1 10 
 9  0 11  8 
13 14 15 12 

 3  2  7  4 
 5  0  1 10 
 9  6 11  8 
13 14 15 12 

 3  0  7  4 
 5  2  1 10 
 9  6 11  8 
13 14 15 12 

 0  3  7  4 
 5  2  1 10 
 9  6 11  8 
13 14 15 12 

 5  3  7  4 
 0  2  1 10 
 9  6 11  8 
13 14 15 12 

 5  3  7  4 
 2  0  1 10 
 9  6 11  8 
13 14 15 12 

 5  3  7  4 
 2  1  0 10 
 9  6 11  8 
13 14 15 12 

 5  3  7  4 
 2  1 10  0 
 9  6 11  8 
13 14 15 12 

 5  3  7  4 
 2  1 10  8 
 9  6 11  0 
13 14 15 12 

 5  3  7  4 
 2  1 10  8 
 9  6  0 11 
13 14 15 12 

 5  3  7  4 
 2  1  0  8 
 9  6 10 11 
13 14 15 12 

 5  3  0  4 
 2  1  7  8 
 9  6 10 11 
13 14 15 12 

 5  0  3  4 
 2  1  7  8 
 9  6 10 11 
13 14 15 12 

 5  1  3  4 
 2  0  7  8 
 9  6 10 11 
13 14 15 12 

 5  1  3  4 
 0  2  7  8 
 9  6 10 11 
13 14 15 12 

 0  1  3  4 
 5  2  7  8 
 9  6 10 11 
13 14 15 12 

 1  0  3  4 
 5  2  7  8 
 9  6 10 11 
13 14 15 12 

 1  2  3  4 
 5  0  7  8 
 9  6 10 11 
13 14 15 12 

 1  2  3  4 
 5  6  7  8 
 9  0 10 11 
13 14 15 12 

 1  2  3  4 
 5  6  7  8 
 9 10  0 11 
13 14 15 12 

 1  2  3  4 
 5  6  7  8 
 9 10 11  0 
13 14 15 12 

 1  2  3  4 
 5  6  7  8 
 9 10 11 12 
13 14 15  0