写在前面
整个项目都托管在了 Github 上:https://github.com/ikesnowy/Algorithms-4th-Edition-in-Csharp
查找更为方便的版本见:https://alg4.ikesnowy.com/
这一节内容可能会用到的库文件有 Quick,同样在 Github 上可以找到。
善用 Ctrl + F 查找题目。
习题&题解
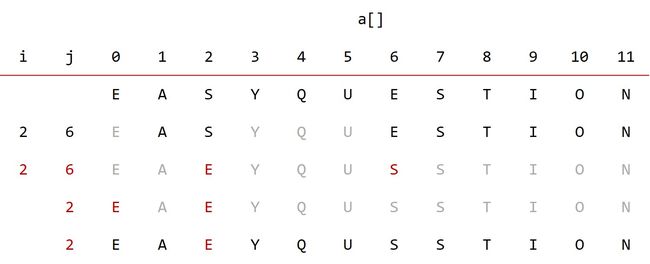
2.3.1
解答
2.3.2
解答
2.3.3
解答
N / 2
在快速排序中,一个元素要被交换,有以下两种情况
1.该元素是枢轴,在切分的最后一步被交换
2.该元素位于枢轴错误的一侧,需要被交换到另一侧去
注意,以上两种情况在一次切分中只会出现一次
首先来看第一种情况,如果一个元素变成了枢轴
那么在之后的切分中该元素会被排除,不存在后续的交换。
因此我们的目标应该是:
最大的元素总是出现在错误的一侧,同时切分的次数尽可能多。
接下来我们来思考如何构造这样的数组
由于我们针对的是最大的元素,因此「错误的一侧」就是枢轴的左侧。
为了使切分的次数尽可能多,我们需要保持最大值移动的距离尽量短。
但如果每次只移动一位的话,下一次切分时最大值就会变成枢轴
例如 4 10 3 5 6,枢轴为 4,交换后数组变为:
4 3 10 5 6
随后 4 和 3 交换
3 4 10 5 6
下一次切分时 10 会变成枢轴,不再参与后续的切分。
因此我们需要让最大值每次移动两个元素。
考虑下面的数组:
2 10 4 1 6 3 8 5 7 9
第一次切分的时候,枢轴为 2,10 和 1 进行交换
数组变为:
2 1 4 10 6 3 8 5 7 9
随后枢轴交换,数组变为:
1 2 4 10 6 3 8 5 7 9
第二次切分,枢轴为 4,10 和 3 进行交换。
1 2 4 3 6 10 8 5 7 9
随后枢轴交换 数组变为:
1 2 3 4 6 10 8 5 7 9
第三次切分,枢轴为 6,10 和 5 交换
1 2 3 4 6 5 8 10 7 9
随后枢轴交换,数组变为:
1 2 3 4 5 6 8 10 7 9
第四次切分,枢轴为 8,10 和 7 交换
1 2 3 4 5 6 8 7 10 9
枢轴交换,数组变为
1 2 3 4 5 6 7 8 10 9
最后一次切分,枢轴为 10,直接交换
1 2 3 4 5 6 7 8 9 10
我们可以总结出要构造这样的数组的模板
a2 max a3 a1
其中 a1 < a2 < a3 < max
max 每轮切分移动两格,总共切分 N/ 2 次。
另请参阅
Number of largest element exchanges for quicksort-Stack Overflow
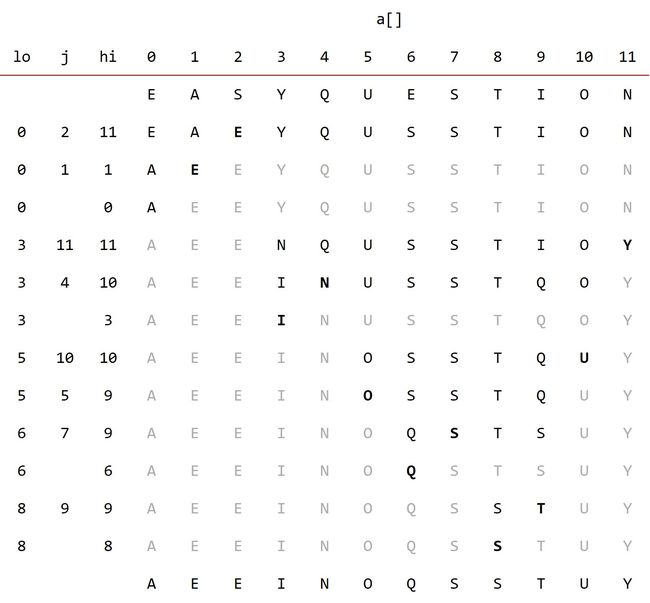
2.3.4
解答
每次只让枢轴变为已排序,这就是最坏情况。
这种时候枢轴是当前子数组的最大值 / 最小值。
由于在我们的实现中总是取子数组的第一个元素作为枢轴。
因此一个已排序的数组可以达到最坏情况,比较次数达到 O(n^ 2)。
如果换作取最后一个元素,最坏情况会变成逆序数组。
我们的实现中如果碰到与枢轴相等的元素也会停止循环,
因此如果数组中有重复的元素会减少比较次数。
例如:
1 2 3 4 5 6 7 8 9 10
2 3 4 5 6 7 8 9 10 11
3 4 5 6 7 8 9 10 11 12
4 5 6 7 8 9 10 11 12 13
5 6 7 8 9 10 11 12 13 14
6 7 8 9 10 11 12 13 14 15另请参阅
Analysis of Quicksort-khanacademy
Worst case for QuickSort - When can it occur?-Stack Overflow
2.3.5
解答
官方实现:https://algs4.cs.princeton.edu/23quicksort/Sort2distinct.java.html
算法 gif 动图
代码
namespace Quick
{
///
/// 用于将只有两种元素的数组排序。
///
public class Sort2Distinct : BaseSort
{
///
/// 默认构造函数。
///
public Sort2Distinct() { }
///
/// 对数组 a 进行排序。
///
/// 数组 a 的元素类型。
///
public override void Sort(T[] a)
{
int lt = 0, gt = a.Length - 1;
int i = 0;
while (i <= gt)
{
int cmp = a[i].CompareTo(a[lt]);
if (cmp < 0)
Exch(a, lt++, i++);
else if (cmp > 0)
Exch(a, i, gt--);
else
i++;
}
}
}
} 另请参阅
Quick 库
2.3.6
解答
代码
新建一个 QuickSortAnalyze 类,在 QuickSort 的基础上添加一个 CompareCount 属性,用于记录比较次数。重写 Less 方法,每调用一次就让 CompareCount 增加 1 。
using System;
using System.Diagnostics;
namespace Quick
{
///
/// 自动记录比较次数以及子数组数量的快速排序类。
///
public class QuickSortAnalyze : BaseSort
{
///
/// 比较次数。
///
public int CompareCount { get; set; }
///
/// 是否启用打乱。
///
public bool NeedShuffle { get; set; }
///
/// 是否显示轨迹。
///
public bool NeedPath { get; set; }
///
/// 大小为 0 的子数组数量。
///
public int Array0Num { get; set; }
///
/// 大小为 1 的子数组数量。
///
public int Array1Num { get; set; }
///
/// 大小为 2 的子数组数量。
///
public int Array2Num { get; set; }
///
/// 默认构造函数。
///
public QuickSortAnalyze()
{
this.CompareCount = 0;
this.NeedShuffle = true;
this.NeedPath = false;
this.Array0Num = 0;
this.Array1Num = 0;
this.Array2Num = 0;
}
///
/// 用快速排序对数组 a 进行升序排序。
///
/// 需要排序的类型。
/// 需要排序的数组。
public override void Sort(T[] a)
{
this.Array0Num = 0;
this.Array1Num = 0;
this.Array2Num = 0;
this.CompareCount = 0;
if (this.NeedShuffle)
Shuffle(a);
if (this.NeedPath)
{
for (int i = 0; i < a.Length; i++)
{
Console.Write(" ");
}
Console.WriteLine("\tlo\tj\thi");
}
Sort(a, 0, a.Length - 1);
Debug.Assert(IsSorted(a));
}
///
/// 用快速排序对数组 a 的 lo ~ hi 范围排序。
///
/// 需要排序的数组类型。
/// 需要排序的数组。
/// 排序范围的起始下标。
/// 排序范围的结束下标。
private void Sort(T[] a, int lo, int hi) where T : IComparable
{
if (hi - lo == 1)
this.Array2Num++;
else if (hi == lo)
this.Array1Num++;
else if (hi < lo)
this.Array0Num++;
if (hi <= lo) // 别越界
return;
int j = Partition(a, lo, hi);
if (this.NeedPath)
{
for (int i = 0; i < a.Length; i++)
{
Console.Write(a[i] + " ");
}
Console.WriteLine("\t" + lo + "\t" + j + "\t" + hi);
}
Sort(a, lo, j - 1);
Sort(a, j + 1, hi);
}
///
/// 对数组进行切分,返回枢轴位置。
///
/// 需要切分的数组类型。
/// 需要切分的数组。
/// 切分的起始点。
/// 切分的末尾点。
/// 枢轴下标。
private int Partition(T[] a, int lo, int hi) where T : IComparable
{
int i = lo, j = hi + 1;
T v = a[lo];
while (true)
{
while (Less(a[++i], v))
if (i == hi)
break;
while (Less(v, a[--j]))
if (j == lo)
break;
if (i >= j)
break;
Exch(a, i, j);
}
Exch(a, lo, j);
return j;
}
///
/// 打乱数组。
///
/// 需要打乱的数组类型。
/// 需要打乱的数组。
private void Shuffle(T[] a)
{
Random random = new Random();
for (int i = 0; i < a.Length; i++)
{
int r = i + random.Next(a.Length - i);
T temp = a[i];
a[i] = a[r];
a[r] = temp;
}
}
///
/// 比较第一个元素是否小于第二个元素。
///
/// 要比较的元素类型。
/// 第一个元素。
/// 第二个元素。
/// (T a, T b) where T : IComparable
{
this.CompareCount++;
return a.CompareTo(b) < 0;
}
}
} 主方法
using System;
using Quick;
namespace _2._3._6
{
/*
* 2.3.6
*
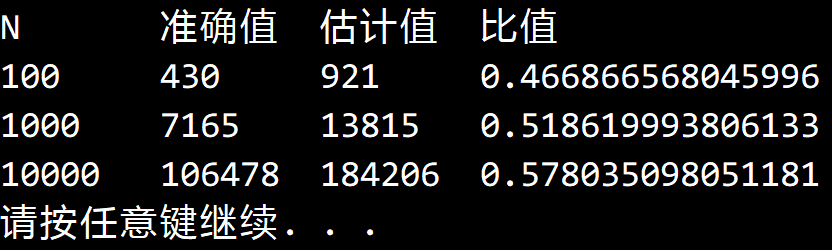
* 编写一段代码来计算 C_N 的准确值,
* 在 N=100、1000 和 10 000 的情况下比较准确值和估计值 2NlnN 的差距。
*
*/
class Program
{
static void Main(string[] args)
{
Console.WriteLine("N\t准确值\t估计值\t比值");
QuickSortAnalyze sort = new QuickSortAnalyze();
int N = 100;
int trialTime = 500;
for (int i = 0; i < 3; i++)
{
int sumOfCompare = 0;
int[] a = new int[N];
for (int j = 0; j < trialTime; j++)
{
for (int k = 0; k < N; k++)
{
a[k] = k;
}
SortCompare.Shuffle(a);
sort.Sort(a);
sumOfCompare += sort.CompareCount;
}
int averageCompare = sumOfCompare / trialTime;
double estimatedCompare = 2 * N * Math.Log(N);
Console.WriteLine(N + "\t" + averageCompare + "\t" + (int)estimatedCompare + "\t" + averageCompare / estimatedCompare);
N *= 10;
}
}
}
}另请参阅
Quick 库
2.3.7
解答
我讨厌数学= =
证明:
我们设 $ C_0(n) $ 代表将 $ n $ 个不重复元素排序时大小为 0 的数组的数量。
同理有 $ C_1(n) $ 和 $ C_2(n) $ 代表大小为 1 的数组的数量以及大小为 2 的数组的数量。
设 k 代表切分位置,显然切分位置随机且概率相等,在 1~n 之间均匀分布。
根据条件,$ C_0(n), C_1(n),C_2(n) $ 都满足下式:
\[ C(n)= \frac{\sum_{k=1}^{n}(C(k-1)+C(n-k))}{n} \]
根据快速排序算法, $ \sum_{k=1}^{n}C(k-1)=\sum_{k=1}^{n}C(n-k) $ ,因此
\[ C(n)=\frac{2\sum_{k=1}^{n}C(k-1)}{n}\\ nC(n)=2\sum_{k-1}^{n}C(k-1) \]
同理代入 $ n-1 $ 有
\[ (n-1)C(n-1)=2\sum_{k-1}^{n-1}C(k-1) \]
相减
\[ nC(n)-(n-1)C(n-1)=2C(n-1)\\ C(n)=\frac{n+1}{n}C(n-1) \]
利用累乘法求到通项公式
\[ \frac{C(n)}{C(n-1)}=\frac{n+1}{n} \\ \frac{C(n)}{C(n-1)}\times\frac{C(n-1)}{C(n-2)}\times\dots\times\frac{C(m+1)}{C(m)}= \frac{n+1}{n}\times\frac{n}{n-1}\times\dots\times\frac{m+2}{m+1}\\ \frac{C(n)}{C(m)}=\frac{n+1}{m+1}\\ C(n)=C(m)\frac{n+1}{m+1},n>m \]
对于 $ C_0(n) $ ,我们有初始条件 $ C_0(0)=1, C_0(1)=0,C_0(2)=C_0(0)+C_0(1)=1 $
\[ C_0(n)=\frac{n+1}{3}, n>2 \]
对于 $ C_1(n) $ ,我们有初始条件 $ C_1(0)=0,C_1(1)=1,C_1(2)=C_1(0)+C_1(1)=1 $
\[ C_1(n)=\frac{n+1}{3},n>2 \]
对于 $ C_2(n) $ ,我们有初始条件 $ C_2(0)=C_2(1)=0,C_2(2)=1,C_2(3)=\frac{2\times(C_2(0)+C_2(1)+C_2(2))}{3}=\frac{2}{3} $
\[ C_2(n)=\frac{n+1}{6},n>3 \]
结论
\[ C_0(n)=C_1(n)=\frac{n+1}{3},n>2 \\ C_2(n)=\frac{n+1}{6},n>3 \]
实验结果:
代码
QuickSortAnalyze 类,添加了三个属性用于计算数组数量。
using System;
using System.Diagnostics;
namespace Quick
{
///
/// 自动记录比较次数以及子数组数量的快速排序类。
///
public class QuickSortAnalyze : BaseSort
{
///
/// 比较次数。
///
public int CompareCount { get; set; }
///
/// 是否启用打乱。
///
public bool NeedShuffle { get; set; }
///
/// 是否显示轨迹。
///
public bool NeedPath { get; set; }
///
/// 大小为 0 的子数组数量。
///
public int Array0Num { get; set; }
///
/// 大小为 1 的子数组数量。
///
public int Array1Num { get; set; }
///
/// 大小为 2 的子数组数量。
///
public int Array2Num { get; set; }
///
/// 默认构造函数。
///
public QuickSortAnalyze()
{
this.CompareCount = 0;
this.NeedShuffle = true;
this.NeedPath = false;
this.Array0Num = 0;
this.Array1Num = 0;
this.Array2Num = 0;
}
///
/// 用快速排序对数组 a 进行升序排序。
///
/// 需要排序的类型。
/// 需要排序的数组。
public override void Sort(T[] a)
{
this.Array0Num = 0;
this.Array1Num = 0;
this.Array2Num = 0;
this.CompareCount = 0;
if (this.NeedShuffle)
Shuffle(a);
if (this.NeedPath)
{
for (int i = 0; i < a.Length; i++)
{
Console.Write(" ");
}
Console.WriteLine("\tlo\tj\thi");
}
Sort(a, 0, a.Length - 1);
Debug.Assert(IsSorted(a));
}
///
/// 用快速排序对数组 a 的 lo ~ hi 范围排序。
///
/// 需要排序的数组类型。
/// 需要排序的数组。
/// 排序范围的起始下标。
/// 排序范围的结束下标。
private void Sort(T[] a, int lo, int hi) where T : IComparable
{
if (hi - lo == 1)
this.Array2Num++;
else if (hi == lo)
this.Array1Num++;
else if (hi < lo)
this.Array0Num++;
if (hi <= lo) // 别越界
return;
int j = Partition(a, lo, hi);
if (this.NeedPath)
{
for (int i = 0; i < a.Length; i++)
{
Console.Write(a[i] + " ");
}
Console.WriteLine("\t" + lo + "\t" + j + "\t" + hi);
}
Sort(a, lo, j - 1);
Sort(a, j + 1, hi);
}
///
/// 对数组进行切分,返回枢轴位置。
///
/// 需要切分的数组类型。
/// 需要切分的数组。
/// 切分的起始点。
/// 切分的末尾点。
/// 枢轴下标。
private int Partition(T[] a, int lo, int hi) where T : IComparable
{
int i = lo, j = hi + 1;
T v = a[lo];
while (true)
{
while (Less(a[++i], v))
if (i == hi)
break;
while (Less(v, a[--j]))
if (j == lo)
break;
if (i >= j)
break;
Exch(a, i, j);
}
Exch(a, lo, j);
return j;
}
///
/// 打乱数组。
///
/// 需要打乱的数组类型。
/// 需要打乱的数组。
private void Shuffle(T[] a)
{
Random random = new Random();
for (int i = 0; i < a.Length; i++)
{
int r = i + random.Next(a.Length - i);
T temp = a[i];
a[i] = a[r];
a[r] = temp;
}
}
///
/// 比较第一个元素是否小于第二个元素。
///
/// 要比较的元素类型。
/// 第一个元素。
/// 第二个元素。
/// (T a, T b) where T : IComparable
{
this.CompareCount++;
return a.CompareTo(b) < 0;
}
}
} 主方法
using System;
using Quick;
namespace _2._3._7
{
/*
* 2.3.7
*
* 在使用快速排序将 N 个不重复的元素排序时,
* 计算大小为 0、1 和 2 的子数组的数量。
* 如果你喜欢数学,请推导;
* 如果你不喜欢,请做一些实验并提出猜想。
*
*/
class Program
{
static void Main(string[] args)
{
// 证明
// 我们设 C0(n) 代表将 n 个不重复元素排序时大小为 0 的数组的数量。
// 同理有 C1(n) 和 C2(n) 代表大小为 1 的数组的数量和大小为 2 的数组的数量。
// 设 k 代表切分位置,显然切分位置随机且概率相等,在 1~n 之间均匀分布。
// 根据条件,三者都满足下式。
// C(n) = 1/n sum(C(k - 1) + C(n - k)), k=1,2,...,n
// 显然 sum(C(k - 1)) = sum(C(n - k)), k=1,2,...,n
// 于是可以化简为
// C(n) = 2/n sum(C(k - 1)), k=1,2,...,n
// nC(n) = 2 * sum(C(k-1)), k=1,2,...,n
// 同理有
// (n-1)C(n-1) = 2 * sum(C(k-1)), k = 1,2,...,n-1
// 相减得到递推式
// nC(n) - (n-1)C(n-1) = 2*C(n-1)
// C(n) = (n+1)/n * C(n-1)
// 利用累乘法可以求得通项公式
// C(n)=C(k)*(n+1)/(k+1), n>k
// 对于 C0 有 C0(0)=1, C0(1)=0
// C0(2)=C(0)+C(1)=1
// C0(n)=(n+1)/3, n>2
// 对于 C1 有 C1(0)=0, C1(1)=1
// C1(2)=C1(0)+C1(1)=1
// C1(n)=(n+1)/3, n>2
// 对于 C2 有 C2(0)=C2(1)=0, C2(2)=1
// C2(3)=1/3*2*(C2(0)+C2(1)+C2(2))=2/3
// C2(n)=C2(3)*(n+1)/4=(n+1)/6, n>3
// 结论
// C0(n)=C1(n)=(n+1)/3, C2(n)=(n+1)/6
int n = 1000;
QuickSortAnalyze sort = new QuickSortAnalyze();
Console.WriteLine("n\t0\t1\t2");
for (int i = 0; i < 5; i++)
{
int[] a = new int[n];
for (int j = 0; j < n; j++)
{
a[j] = j;
}
SortCompare.Shuffle(a);
sort.Sort(a);
Console.WriteLine(n + "\t" + sort.Array0Num + "\t" + sort.Array1Num + "\t" + sort.Array2Num);
n *= 2;
}
}
}
}另请参阅
Quick 库
What is the expected number of subarrays of size 0, 1 and 2 when quicksort is used to sort an array of N items with distinct keys?-Stack Overflow
2.3.8
解答
每次切分都会把数组平分,共切分 logN 次(二分法),每次切分比较 N 次(i 和 j 会一位一位地从两边向中间靠拢)。
共比较 NlogN 次。
2.3.9
解答
切分时,枢轴左侧都是小于(或等于)枢轴的,
右侧都是大于(或等于)枢轴的
只有两种主键值时,
第一次切分之后,某一侧的元素将全部相同
(如果枢轴选了较大的,那么右侧将全部相同,反之则左侧全部相同)
只有三种主键值时,和一般快速排序并无不同。
但如果第一次切分时选择了中间值作为枢轴,且中间值只有一个
那么只需要一次切分数组便会有序。
2.3.10
解答
切比雪夫不等式(Chebyshev’s inequality)
\[ P(|X-\mu|\geq k\sigma)\leq \frac{1}{k^2} \]
其中,$ \mu $ 代表期望,$ \sigma $ 代表标准差。
对于快速排序的比较次数来说,$ \mu = 2N\ln N $ ,$ \sigma=0.65N $。
(这两个结论见 2.3 节的命题 K 和命题 L)
题目中要求比较次数大于 $ 0.1N^2 $ ,可以求得 $ k $ 的值。
\[ 0.65kN=0.1N^2 \\ k=\frac{2N}{13} \]
将 $ N=1,000,000 $ 代入
\[ P(|X-27,631,021|\geq 100,000,000,000)\leq 0.00000000004225 \]
另请参阅
切比雪夫不等式到底是个什么概念? - 马同学的回答 - 知乎
2.3.11
解答
只有若干种元素值意味着大量的连续重复。
(由于存在打乱这一步骤,不存在连续重复的可能性是很低的)
接下来我们考虑这样的连续重复在修改后的快排下的性能。
1 1 1 1 1 1 1
对于这样的数组,枢轴选为 1,j 将会在 j = lo 处终止。
因此最后的结果将是每次只有数组的第一个元素被排序
已知每次切分都是 O(k - 1) 的(i 和 j 都将走完整个子数组)
因此这样的快速排序所需时间 = $ 2 (N - 1 + N - 2 + \cdots + 1) = (N - 1)N $
因此对于值相同的子数组,这样的快排运行时间是平方级别的
那么当数组中这样的连续重复内容越多,运行时间就越接近平方级别。
2.3.12
解答
2.3.13
解答
快速排序先将数组分为 (小于枢轴)枢轴(大于枢轴)三部分,然后再分别递归的排序左右两部分数组。
在这里,我们可以将快速排序的递归树看作是一棵二叉搜索树(BST, Binary Search Tree)。
枢轴作为根结点,左子树即为左数组构造的 BST,右子树即为右数组构造的 BST。
这样题目中所求的递归深度即为所构造的 BST 的高度。
最坏情况,每次都只有枢轴和大于枢轴两部分,BST 退化为链表,高度为 $ n-1 $。
最好情况,每次枢轴都正好平分数组,构造一棵完全二叉树,高度为 $ \log n $。
平均情况,问题转化为:一个由 $ n $ 个元素随机构造的 BST 的平均高度是多少?
《算法导论》给出的结论是 $ \log n $ ,具体证明如下:
设由 $ n $ 个结点随机构成的 BST 的高度为 $ h_n $,那么有:
\[ h_n=1+\max(h_{l}+h_{r}) \]
其中,$ h_l $ 和 $ h_r $ 分别代表左数组和右数组构造的 BST 的高度。
设枢轴位置为 $ i $,上式可简化为:
\[ h_n=1+\max(h_{i-1}, h_{n-i}) \]
由于枢轴位置可以在 1~n 之间任意取值且概率相等,因此 BST 的平均高度(即高度的期望)为:
\[ E(h_n)=\frac{1}{n}\sum_{i=1}^{n}\lbrack 1+\max(h_{i-1}, h_{n-i}) \rbrack \]
我们令 $ Y_n=2^{h_n} $,可得:
\[ Y_n=2\times\max(Y_{i-1},Y_{n-i}) \]
我们把 $ Y_n $ 代入,可得:
\[ \begin{align*} E(Y_n) &=\sum_{i=1}^{n}\frac{1}{n}E\lbrack2\times\max(Y_{i-1}, Y_{n-i})\rbrack\\ &=\frac{2}{n}\sum_{i=1}^{n}E\lbrack\max(Y_{i-1},Y_{n-i})\rbrack\\ \end{align*} \]
接下来我们去掉最大值运算,根据最大值的性质,下式显然成立:
\[ E\lbrack\max(X,Y)\rbrack\le E\lbrack\max(X,Y)+\min(X,Y)\rbrack=E\lbrack X+Y\rbrack=E\lbrack X\rbrack+E\lbrack Y\rbrack \]
代入可得:
\[ E(Y_n) \le\frac{2}{n}\sum_{i=1}^{n}(E\lbrack Y_{i-1}\rbrack + E\lbrack Y_{n-i} \rbrack) =\frac{2}{n}\sum_{i=0}^{n-1}2E\lbrack Y_i\rbrack =\frac{4}{n}\sum_{i=0}^{n-1}E\lbrack Y_i\rbrack \]
大小为 0 的数组构成的 BST 的高度显然为 0,我们设 $ Y_0=0 $ 。接下来用一个组合数公式来构造上界:
\[ \begin{align*} 0&=Y_0=E\lbrack Y_0 \rbrack\le \frac{1}{4}\begin{pmatrix}3\\3\end{pmatrix}=\frac{1}{4}\\ 1&=Y_1=E\lbrack Y_1 \rbrack\le\frac {1}{4}\begin{pmatrix}3+1\\3\end{pmatrix}=1 \\ \vdots \\ Y_i &=E\lbrack Y_i\rbrack\le\frac{1}{4}\begin{pmatrix}i+3\\3\end{pmatrix} \end{align*} \]
注意这里的组合数公式为:
\[ \begin{pmatrix}n\\r\end{pmatrix}=\frac{r!}{r!(n-r)!} \]
代入可得:
\[ \begin{align*} E(Y_n) &\le \frac{4}{n}\sum_{i=0}^{n-1}E\lbrack Y_i\rbrack \\ &\le\frac{4}{n}\sum_{i=0}^{n-1}\frac{1}{4}\begin{pmatrix}i+3\\3\end{pmatrix} \\ &=\frac{1}{n}\sum_{i=0}^{n-1}\begin{pmatrix}i+3\\3\end{pmatrix} \end{align*} \]
接下来我们去掉求和符号,首先根据组合数的性质,有以下等式成立
\[ \begin{align*} \begin{pmatrix}n\\k\end{pmatrix}&=\begin{pmatrix}n-1\\k-1\end{pmatrix}+\begin{pmatrix}n-1\\k\end{pmatrix} \\ \begin{pmatrix}n\\n\end{pmatrix}&=1 \end{align*} \]
我们把求和式展开得到:
\[ \begin{align*} \sum_{i=0}^{n-1}\begin{pmatrix}i+3\\3\end{pmatrix} &=\begin{pmatrix}3\\3\end{pmatrix} + \begin{pmatrix}4\\3\end{pmatrix}+\cdots+\begin{pmatrix}n+2\\3\end{pmatrix} \\ &=\begin{pmatrix}4\\4\end{pmatrix} + \begin{pmatrix}4\\3\end{pmatrix}+\cdots+\begin{pmatrix}n+2\\3\end{pmatrix} \\ &=\begin{pmatrix}n+3\\4\end{pmatrix} \end{align*} \]
代入可得:
\[ \begin{align*} E(Y_n) &\le\frac{1}{n}\sum_{i=0}^{n-1}\begin{pmatrix}i+3\\3\end{pmatrix}\\ &=\frac{1}{n}\begin{pmatrix}n+3\\4 \end{pmatrix} \\ &=\frac{1}{n}\cdot\frac{(n+3)!}{4!(n-1)!} \\ &=\frac{1}{4}\cdot\frac{(n+3)!}{3!n!} \\ &=\frac{(n+1)(n+2)(n+3)}{24} \\ &=\frac{n^3+6n^2+11n+6}{24} \end{align*} \]
由于 \(Y_n=2^{h_n}\) ,因此 \(E\lbrack Y_n \rbrack=E\lbrack 2^{h_n} \rbrack\)。
由于 \(f(x)=2^x\) 是个凸函数,可以应用延森不等式(凸函数的割线一定在函数上方),即 \(2^{E\lbrack h_n\rbrack}\le E\lbrack Y_n\rbrack\)。
于是得到结论:
\[ 2^{E\lbrack h_n\rbrack} \le \frac{n^3+6n^2+11n+6}{24} \\ E\lbrack h_n \rbrack\le \log(\frac{n^3+6n^2+11n+6}{24}) \]
另请参阅
快速排序的递归树可以视为 BST 的结论可以在下面这个 PPT 的第 5 页找到。
QuickSort-纽约大学
《算法导论》中关于随机 BST 高度的证明(P321 Theorem12.4)
Introduction to Algorithms
也可以参考下面这个链接获得更详细的解释。
Proof that a randomly built binary search tree has logarithmic height-StackExchange
2.3.14
解答
中文版题目有误,详见官方勘误页面:https://algs4.cs.princeton.edu/errata/errata-printing3.php
假设 $ i < j $ 。
首先,在快速排序中,如果两个元素要发生交换,意味着其中一个元素被选为枢轴。
而且数组中的元素各不相同,那么两个特定的元素的比较最多发生一次。
那么先考虑一个特殊情况,$ i = 1, j = n $ ,即求最大值和最小值比较的概率。
此时,一旦枢轴不是这两个元素之一,
最大值和最小值会被分到两个不同的子数组,无法发生比较。
因此在这种特例下第 $ i $ 大的元素和第 $ j $ 大的元素发生比较的概率为 $ \frac{2}{n} = \frac{2}{j-i+1} $ 。
接下来考虑一般情况,如果枢轴选择了第 $ i $ 到第 $ j $ 大之外的元素,
那么第 $ i $ 大和第 $ j $ 大的元素会被分到同一个子数组里,重复上述过程。
因此我们所求的概率只和从第 $ i $ 大到第 $ j $ 大之间的元素有关,概率为 \(\frac{2}{j-i+1}\)。
(举个例子,一个箱子里有 2 个红球、1个蓝球和 7 个白球,现在摸球而不放回。
如果摸到白球可以再摸一次,直到摸到红球或蓝球为止。
显然在这样的规则下摸到红球或蓝球的概率为 1,即白球对概率没有影响。)
现在我们已经得到了某两个元素比较的概率 \(E(X_{ij})\),接下来我们求每两个元素比较的概率 $ E(X) $。
\[ \begin{align*} E(X) &= \sum_{i=1}^{n}\sum_{j=i+1}^{n}E(X_{ij})\\ &=\sum_{i=1}^{n}2(\frac{1}{2}+\frac{1}{3}+\cdots+\frac{1}{n-i+1}) \\ &<2n(\frac{1}{2}+\frac{1}{3}+\cdots+\frac{1}{n}) \end{align*} \]
根据调和级数的性质($ \ln (n) < 1+ \frac{1}{2}+ \cdots + \frac{1}{n} < 1+\ln(n) $),可以得到结论:
\[ E(X) < 2n \ln(n) \]
另请参阅
下面这个链接里的 3.4.2 节给出了解法。
lect0906 - 卡内基梅隆大学
如果还是不能理解为什么多次切分不影响概率,可以参考三门问题的解释:
蒙提霍尔问题 - 维基百科
蒙提霍尔问题(又称三门问题、山羊汽车问题)的正解是什么?- 知乎
2.3.15
解答
事实上只需要修改快速排序的切分方法,分两次进行切分。
首先选第一个螺母作为枢轴,找到对应的螺丝($ O(n) $)放到第一位,对螺丝数组进行切分。
然后再用找到的螺丝对螺母数组进行切分。
螺母类,实现了对螺丝类的 IComparable 接口
///
/// 螺母类。
///
public class Nut : IComparable> where T : IComparable
{
///
/// 螺母的值。
///
public T Value { get; set; }
///
/// 螺母的构造函数。
///
/// 螺母的值。
public Nut(T value) => this.Value = value;
///
/// 比较方法,螺母只能和螺丝比较。
///
/// 需要比较的螺丝。
/// other)
{
return this.Value.CompareTo(other.Value);
}
} 类似的有螺丝类。
///
/// 螺丝类。
///
public class Bolt : IComparable> where T : IComparable
{
///
/// 螺丝的值。
///
public T Value { get; set; }
///
/// 螺丝的默认构造函数。
///
/// 螺丝的值。
public Bolt(T value) => this.Value = value;
///
/// 比较方法,螺丝只能和螺母比较。
///
/// 需要比较的螺母。
/// other)
{
return this.Value.CompareTo(other.Value);
}
} 代码
修改后的排序方法。
using System;
namespace _2._3._15
{
///
/// 用快排的方式解决螺母和螺帽的问题。
///
public class BoltsAndNuts
{
private readonly Random random = new Random();
///
/// 默认构造函数。
///
public BoltsAndNuts() { }
///
/// 对螺丝和螺母排序。
///
/// 需要排序的元素类型。
/// 螺母数组。
/// 螺丝数组。
public void Sort(Bolt[] bolts, Nut[] nuts) where T : IComparable
{
if (bolts.Length != nuts.Length)
throw new ArgumentException("数组长度必须一致");
Shuffle(bolts);
Shuffle(nuts);
Sort(bolts, nuts, 0, bolts.Length - 1);
}
///
/// 对螺丝和螺母排序。
///
/// 需要排序的元素类型。
/// 螺母数组。
/// 螺丝数组。
/// 起始下标。
/// 终止下标。
public void Sort(Bolt[] bolts, Nut[] nuts, int lo, int hi) where T : IComparable
{
if (hi <= lo)
return;
int j = Partition(bolts, nuts, lo, hi);
Sort(bolts, nuts, lo, j - 1);
Sort(bolts, nuts, j + 1, hi);
}
///
/// 对数组进行切分。
///
/// 需要排序的数组类型。
/// 螺母数组。
/// 螺丝数组。
/// 起始下标。
/// 终止下标。
/// 切分位置。
private int Partition(Bolt[] bolts, Nut[] nuts, int lo, int hi) where T : IComparable
{
int i = lo, j = hi + 1;
Bolt pivotB = bolts[lo];
// 找到对应螺丝
for (int k = lo; k <= hi; k++)
{
if (nuts[k].CompareTo(pivotB) == 0)
{
Exch(nuts, k, lo);
break;
}
}
// 先用螺母去套螺丝
while (true)
{
while (nuts[++i].CompareTo(pivotB) < 0)
if (i == hi)
break;
while (pivotB.CompareTo(nuts[--j]) < 0)
if (j == lo)
break;
if (i >= j)
break;
Exch(nuts, i, j);
}
Exch(nuts, lo, j);
// 再用螺丝去比较螺母
Nut pivotN = nuts[j];
i = lo;
j = hi + 1;
while (true)
{
while (bolts[++i].CompareTo(pivotN) < 0)
if (i == hi)
break;
while (pivotN.CompareTo(bolts[--j]) < 0)
if (j == lo)
break;
if (i >= j)
break;
Exch(bolts, i, j);
}
Exch(bolts, lo, j);
return j;
}
///
/// 打乱数组。
///
/// 需要打乱的数组类型。
/// 需要打乱的数组。
private void Shuffle(T[] a)
{
for (int i = 0; i < a.Length; i++)
{
int r = i + this.random.Next(a.Length - i);
T temp = a[i];
a[i] = a[r];
a[r] = temp;
}
}
///
/// 交换两个元素。
///
/// 元素类型。
/// 需要交换的第一个元素。
/// 需要交换的第二个元素。
private void Exch(T[] a, int lo, int hi)
{
T t = a[lo];
a[lo] = a[hi];
a[hi] = t;
}
}
} 另请参阅
下面这个网站给出了这道题的解法,还给出了另一种确定性算法(非随机的算法)的论文链接。
Matching Nuts and Bolts - Solution
2.3.16
解答
官方实现见:https://algs4.cs.princeton.edu/23quicksort/QuickBest.java.html
类似于快速排序的结构,只要中点的两边都是最佳情况,那么整个数组就是最佳情况了。
具体方法是:
首先构造一个有序数组,
然后找到中点(作为枢轴),
对中点左右两侧子数组进行构造,
将选择的枢轴放到开始处(a[lo])。
代码
用于构造最佳数组的类。
namespace Quick
{
///
/// 构建快速排序最佳情况的类。
///
public class QuickBest
{
///
/// 构造函数,这个类不应该被实例化。
///
private QuickBest() { }
///
/// 构造适用于快速排序的最佳数组。
///
/// 数组长度。
///
/// 递归的构造数组。
///
/// 需要构造的数组。
/// 构造的起始下标。
/// 构造的终止下标。
private static void Best(int[] a, int lo, int hi)
{
if (hi <= lo)
return;
int mid = lo + (hi - lo) / 2;
Best(a, lo, mid - 1);
Best(a, mid + 1, hi);
Exch(a, lo, mid);
}
///
/// 交换数组中的两个元素。
///
/// 数组的元素类型。
/// 包含要交换元素的数组。
/// 需要交换的第一个元素下标。
/// 需要交换的第二个元素下标。
private static void Exch(int[] a, int x, int y)
{
int t = a[x];
a[x] = a[y];
a[y] = t;
}
}
}用于测试的方法
using System;
using Quick;
namespace _2._3._16
{
/*
* 2.3.16
*
* 最佳情况。
* 编写一段程序来生成使算法 2.5 中的 sort() 方法表现最佳的数组(无重复元素):
* 数组大小为 N 且不包含重复元素,
* 每次切分后两个子数组的大小最多差 1
* (子数组的大小与含有 N 个相同元素的数组的切分情况相同)。
* (对于这道练习,我们不需要在排序开始时打乱数组。)
*
*/
class Program
{
static void Main(string[] args)
{
QuickSortAnalyze quick = new QuickSortAnalyze
{
NeedShuffle = false, // 关闭打乱
NeedPath = true // 显示排序轨迹
};
int[] a = QuickBest.Best(10);
for (int i = 0; i < 10; i++)
{
Console.Write(a[i] + " ");
}
Console.WriteLine();
quick.Sort(a);
for (int i = 0; i < 10; i++)
{
Console.Write(a[i] + " ");
}
Console.WriteLine();
}
}
}另请参阅
Quick 库
2.3.17
解答
按照题意修改代码即可,在调用 Suffle() 之后添加一段用于寻找最大值的方法($ O(n) $)。
///
/// 用快速排序对数组 a 进行升序排序。
///
/// 需要排序的类型。
/// 需要排序的数组。
public override void Sort(T[] a)
{
Shuffle(a);
// 把最大元素放到最后一位
int maxIndex = 0;
for (int i = 0; i < a.Length; i++)
{
if (Less(a[maxIndex], a[i]))
maxIndex = i;
}
Exch(a, maxIndex, a.Length - 1);
Sort(a, 0, a.Length - 1);
Debug.Assert(IsSorted(a));
} 代码
修改后的快速排序类。
using System;
using System.Diagnostics;
using Quick;
namespace _2._3._17
{
///
/// 快速排序类。
///
public class QuickSortX : BaseSort
{
///
/// 默认构造函数。
///
public QuickSortX() { }
///
/// 用快速排序对数组 a 进行升序排序。
///
/// 需要排序的类型。
/// 需要排序的数组。
public override void Sort(T[] a)
{
Shuffle(a);
// 把最大元素放到最后一位
int maxIndex = 0;
for (int i = 0; i < a.Length; i++)
{
if (Less(a[maxIndex], a[i]))
maxIndex = i;
}
Exch(a, maxIndex, a.Length - 1);
Sort(a, 0, a.Length - 1);
Debug.Assert(IsSorted(a));
}
///
/// 用快速排序对数组 a 的 lo ~ hi 范围排序。
///
/// 需要排序的数组类型。
/// 需要排序的数组。
/// 排序范围的起始下标。
/// 排序范围的结束下标。
private void Sort(T[] a, int lo, int hi) where T: IComparable
{
if (hi <= lo) // 别越界
return;
int j = Partition(a, lo, hi);
Sort(a, lo, j - 1);
Sort(a, j + 1, hi);
}
///
/// 对数组进行切分,返回枢轴位置。
///
/// 需要切分的数组类型。
/// 需要切分的数组。
/// 切分的起始点。
/// 切分的末尾点。
/// 枢轴下标。
private int Partition(T[] a, int lo, int hi) where T : IComparable
{
int i = lo, j = hi + 1;
T v = a[lo];
while (true)
{
while (Less(a[++i], v)) ;
// if (i == hi)
// break;
while (Less(v, a[--j])) ;
// if (j == lo)
// break;
if (i >= j)
break;
Exch(a, i, j);
}
Exch(a, lo, j);
return j;
}
///
/// 打乱数组。
///
/// 需要打乱的数组类型。
/// 需要打乱的数组。
private void Shuffle(T[] a)
{
Random random = new Random();
for (int i = 0; i < a.Length; i++)
{
int r = i + random.Next(a.Length - i);
T temp = a[i];
a[i] = a[r];
a[r] = temp;
}
}
}
} 主方法。
using System;
using Quick;
namespace _2._3._17
{
/*
* 2.3.17
*
* 哨兵。
* 修改算法 2.5,去掉内循环 while 中的边界检查。
* 由于切分元素本身就是一个哨兵(v 不可能小于 a[lo]),
* 左侧边界检查是多余的。
* 要去掉另一个检查,可以在打乱数组后将数组的最大元素方法 a[length - 1] 中。
* 该元素永远不会移动(除非和相等的元素交换),
* 可以在所有包含它的子数组中成为哨兵。
* 注意:在处理内部子数组时,
* 右子数组中最左侧的元素可以作为左子数组右边界的哨兵。
*
*/
class Program
{
static void Main(string[] args)
{
QuickSort quick = new QuickSort();
QuickSortX quickSortX = new QuickSortX();
int arrayLength = 1000000;
int[] a = SortCompare.GetRandomArrayInt(arrayLength);
int[] b = new int[arrayLength];
a.CopyTo(b, 0);
double time1 = SortCompare.Time(quick, a);
double time2 = SortCompare.Time(quickSortX, b);
Console.WriteLine("QSort\tQSort with Sentinels\t");
Console.WriteLine(time1 + "\t" + time2 + "\t");
}
}
}另请参阅
Quick 库
2.3.18
解答
每次切分时都取前三个元素的中位数作为枢轴,这可以带来约 5%~10% 的性能提升。
这里通过三次比较将前三个数排序,然后把三个数中的中位数放到数组开头,最大值放到数组末尾。
最大值被放到了末尾,枢轴不可能大于末尾的这个数,因此右边界判断可以去掉。
同时由于枢轴不可能小于自身,因此左边界判断也可以去掉。
这样就可以把切分中的两个边界判断全部去掉了。
最后对于大小为 2 的数组做特殊处理,通过一次比较直接排序并返回。
代码
QuickSortMedian3
using System;
using System.Diagnostics;
using Quick;
namespace _2._3._18
{
///
/// 三取样快速排序
///
public class QuickSortMedian3 : BaseSort
{
///
/// 默认构造函数。
///
public QuickSortMedian3() {}
///
/// 用快速排序对数组 a 进行升序排序。
///
/// 需要排序的类型。
/// 需要排序的数组。
public override void Sort(T[] a)
{
Shuffle(a);
Sort(a, 0, a.Length - 1);
Debug.Assert(IsSorted(a));
}
///
/// 用快速排序对数组 a 的 lo ~ hi 范围排序。
///
/// 需要排序的数组类型。
/// 需要排序的数组。
/// 排序范围的起始下标。
/// 排序范围的结束下标。
private void Sort(T[] a, int lo, int hi) where T: IComparable
{
if (hi <= lo) // 别越界
return;
// 只有两个元素的数组直接排序
if (hi == lo + 1)
{
if (Less(a[hi], a[lo]))
Exch(a, lo, hi);
return;
}
int j = Partition(a, lo, hi);
Sort(a, lo, j - 1);
Sort(a, j + 1, hi);
}
///
/// 对数组进行切分,返回枢轴位置。
///
/// 需要切分的数组类型。
/// 需要切分的数组。
/// 切分的起始点。
/// 切分的末尾点。
/// 枢轴下标。
private int Partition(T[] a, int lo, int hi) where T : IComparable
{
int i = lo, j = hi + 1;
if (Less(a[lo + 1], a[lo]))
Exch(a, lo + 1, lo);
if (Less(a[lo + 2], a[lo]))
Exch(a, lo + 2, lo);
if (Less(a[lo + 2], a[lo + 1]))
Exch(a, lo + 1, lo + 2);
Exch(a, lo, lo + 1); // 中位数放最左侧
Exch(a, hi, lo + 2); // 较大的值放最右侧作为哨兵
T v = a[lo];
while (true)
{
while (Less(a[++i], v)) ;
while (Less(v, a[--j])) ;
if (i >= j)
break;
Exch(a, i, j);
}
Exch(a, lo, j);
return j;
}
///
/// 打乱数组。
///
/// 需要打乱的数组类型。
/// 需要打乱的数组。
private void Shuffle(T[] a)
{
Random random = new Random();
for (int i = 0; i < a.Length; i++)
{
int r = i + random.Next(a.Length - i);
T temp = a[i];
a[i] = a[r];
a[r] = temp;
}
}
}
} 测试用例
using System;
using Quick;
namespace _2._3._18
{
/*
* 2.3.18
*
* 三取样切分。
* 为快速排序实现正文所述的三取样切分(参见 2.3.3.2 节)。
* 运行双倍测试来确认这项改动的效果。
*
*/
class Program
{
static void Main(string[] args)
{
QuickSort quickNormal = new QuickSort();
QuickSortMedian3 quickMedian = new QuickSortMedian3();
int arraySize = 200000; // 初始数组大小。
const int trialTimes = 4; // 每次实验的重复次数。
const int trialLevel = 5; // 双倍递增的次数。
Console.WriteLine("n\tmedian\tnormal\tratio");
for (int i = 0; i < trialLevel; i++)
{
double timeMedian = 0;
double timeNormal = 0;
for (int j = 0; j < trialTimes; j++)
{
int[] a = SortCompare.GetRandomArrayInt(arraySize);
int[] b = new int[a.Length];
a.CopyTo(b, 0);
timeNormal += SortCompare.Time(quickNormal, b);
timeMedian += SortCompare.Time(quickMedian, a);
}
timeMedian /= trialTimes;
timeNormal /= trialTimes;
Console.WriteLine(arraySize + "\t" + timeMedian + "\t" + timeNormal + "\t" + timeMedian / timeNormal);
arraySize *= 2;
}
}
}
}另请参阅
Quick 库
2.3.19
解答
主要介绍一下这个少于七次比较的五取样算法。
首先假设五个数字为 a b c d e
对 b c 排序,d e 排序。(两次比较)
比较 b 和 d,把较小那一组换到 b c 的位置上去。(一次比较)
此时会有 b < c, b < d < e。
交换 a, b,重新对 b c 排序。(一次比较)
再次比较 b 和 d,把较小的那一组换到 b c 的位置上。(一次比较)
最后比较 c 和 d,较小的那一个即为中位数。(一次比较)
总共需要 6 次比较,严格小于 7 次。
取样完毕后,a b 是最小值和次小值(这里没有对应关系,a 也可以是次小值)。
d 和 e 是最大值和次大值(同样没有对应关系)。
我们把 d 和 e 放到数组的最后作为哨兵,去掉右边界的判断。
同时让左右两侧指针都向中间移动两位,减少不必要的比较。
测试结果,对比普通快排性能提升约 10%,和三取样快排区别不大。
代码
五取样快排
using System;
using System.Diagnostics;
using Quick;
namespace _2._3._19
{
///
/// 五取样快速排序
///
public class QuickSortMedian5 : BaseSort
{
///
/// 默认构造函数。
///
public QuickSortMedian5() {}
///
/// 用快速排序对数组 a 进行升序排序。
///
/// 需要排序的类型。
/// 需要排序的数组。
public override void Sort(T[] a)
{
Shuffle(a);
Sort(a, 0, a.Length - 1);
Debug.Assert(IsSorted(a));
}
///
/// 用快速排序对数组 a 的 lo ~ hi 范围排序。
///
/// 需要排序的数组类型。
/// 需要排序的数组。
/// 排序范围的起始下标。
/// 排序范围的结束下标。
private void Sort(T[] a, int lo, int hi) where T: IComparable
{
if (hi <= lo) // 别越界
return;
// 少于五个元素的数组直接进行插入排序
if (hi - lo + 1 < 5)
{
int n = hi - lo + 1;
for (int i = lo; i - lo < n; i++)
{
for (int k = i; k > 0 && Less(a[k], a[k - 1]); --k)
{
Exch(a, k, k - 1);
}
}
return;
}
int j = Partition(a, lo, hi);
Sort(a, lo, j - 1);
Sort(a, j + 1, hi);
}
///
/// 对数组进行切分,返回枢轴位置。
///
/// 需要切分的数组类型。
/// 需要切分的数组。
/// 切分的起始点。
/// 切分的末尾点。
/// 枢轴下标。
private int Partition(T[] a, int lo, int hi) where T : IComparable
{
int i = lo, j = hi + 1;
// 假设为 a b c d e 五个数字
// 首先对 b c 排序
if (Less(a[lo + 2], a[lo + 1]))
Exch(a, lo + 2, lo + 1);
// 然后再排序 d e
if (Less(a[lo + 4], a[lo + 3]))
Exch(a, lo + 4, lo + 3);
// 这时满足 b < c, d < e
// 比较 b d,把较小的一组放到 b c 的位置上去
if (Less(a[lo + 3], a[lo + 1]))
{
Exch(a, lo + 1, lo + 3);
Exch(a, lo + 2, lo + 4);
}
// 这时满足 b < c, b < d < e,即 b 是 b c d e 中的最小值
// 交换 a 和 b
Exch(a, lo, lo + 1);
// 重新排序 b c
if (Less(a[lo + 2], a[lo + 1]))
Exch(a, lo + 2, lo + 1);
// 这时再次满足 b < c, d < e
// 比较 b d,把最小的一组放到 b c 的位置上去
if (Less(a[lo + 3], a[lo + 1]))
{
Exch(a, lo + 1, lo + 3);
Exch(a, lo + 2, lo + 4);
}
// 这时 a 和 b 为五个数中的最小值和次小值(顺序不固定,a 也可以是次小值)
// 最后比较 c 和 d,较小的那一个即为中位数(即第三小的数)
if (Less(a[lo + 3], a[lo + 2]))
Exch(a, lo + 3, lo + 2);
// 此时 c 即为中位数
Exch(a, lo, lo + 2);
// d e 放到数组末尾充当哨兵
Exch(a, lo + 3, hi);
Exch(a, lo + 4, hi - 1);
// 调整指针位置,前两位和后两位都已经在合适位置了
j -= 2;
i += 2;
T v = a[lo];
while (true)
{
while (Less(a[++i], v)) ;
while (Less(v, a[--j])) ;
if (i >= j)
break;
Exch(a, i, j);
}
Exch(a, lo, j);
return j;
}
///
/// 打乱数组。
///
/// 需要打乱的数组类型。
/// 需要打乱的数组。
private void Shuffle(T[] a)
{
Random random = new Random();
for (int i = 0; i < a.Length; i++)
{
int r = i + random.Next(a.Length - i);
T temp = a[i];
a[i] = a[r];
a[r] = temp;
}
}
}
} 三取样快排
using System;
using System.Diagnostics;
using Quick;
namespace _2._3._19
{
///
/// 三取样快速排序
///
public class QuickSortMedian3 : BaseSort
{
///
/// 默认构造函数。
///
public QuickSortMedian3() {}
///
/// 用快速排序对数组 a 进行升序排序。
///
/// 需要排序的类型。
/// 需要排序的数组。
public override void Sort(T[] a)
{
Shuffle(a);
Sort(a, 0, a.Length - 1);
Debug.Assert(IsSorted(a));
}
///
/// 用快速排序对数组 a 的 lo ~ hi 范围排序。
///
/// 需要排序的数组类型。
/// 需要排序的数组。
/// 排序范围的起始下标。
/// 排序范围的结束下标。
private void Sort(T[] a, int lo, int hi) where T: IComparable
{
if (hi <= lo) // 别越界
return;
// 少于五个元素的数组直接进行插入排序
if (hi - lo + 1 < 5)
{
int n = hi - lo + 1;
for (int i = lo; i - lo < n; i++)
{
for (int k = i; k > 0 && Less(a[k], a[k - 1]); --k)
{
Exch(a, k, k - 1);
}
}
return;
}
int j = Partition(a, lo, hi);
Sort(a, lo, j - 1);
Sort(a, j + 1, hi);
}
///
/// 对数组进行切分,返回枢轴位置。
///
/// 需要切分的数组类型。
/// 需要切分的数组。
/// 切分的起始点。
/// 切分的末尾点。
/// 枢轴下标。
private int Partition(T[] a, int lo, int hi) where T : IComparable
{
int i = lo, j = hi + 1;
if (Less(a[lo + 1], a[lo]))
Exch(a, lo + 1, lo);
if (Less(a[lo + 2], a[lo]))
Exch(a, lo + 2, lo);
if (Less(a[lo + 2], a[lo + 1]))
Exch(a, lo + 1, lo + 2);
Exch(a, lo, lo + 1); // 中位数放最左侧
Exch(a, hi, lo + 2); // 较大的值放最右侧作为哨兵
T v = a[lo];
while (true)
{
while (Less(a[++i], v)) ;
while (Less(v, a[--j])) ;
if (i >= j)
break;
Exch(a, i, j);
}
Exch(a, lo, j);
return j;
}
///
/// 打乱数组。
///
/// 需要打乱的数组类型。
/// 需要打乱的数组。
private void Shuffle(T[] a)
{
Random random = new Random();
for (int i = 0; i < a.Length; i++)
{
int r = i + random.Next(a.Length - i);
T temp = a[i];
a[i] = a[r];
a[r] = temp;
}
}
}
} 测试用例
using System;
using Quick;
namespace _2._3._19
{
/*
* 2.3.19
*
* 五取样切分。
* 实现一种基于随机抽取子数组中 5 个元素并取中位数进行切分的快速排序。
* 将取样元素放在数组的一侧以保证只有中位数元素参与了切分。
* 运行双倍测试来确定这项改动的效果,
* 并和标准的快速排序以及三取样的快速排序(请见上一道练习)进行比较。
* 附加题:找到一种对于任意输入都只需要少于 7 次比较的五取样算法。
*
*/
class Program
{
static void Main(string[] args)
{
QuickSort quickNormal = new QuickSort();
QuickSortMedian3 quickMedian3 = new QuickSortMedian3();
QuickSortMedian5 quickMedian5 = new QuickSortMedian5();
int arraySize = 200000; // 初始数组大小。
const int trialTimes = 4; // 每次实验的重复次数。
const int trialLevel = 6; // 双倍递增的次数。
Console.WriteLine("n\tmedian5\tmedian3\tnormal\tmedian5/normal\t\tmedian5/median3");
for (int i = 0; i < trialLevel; i++)
{
double timeMedian3 = 0;
double timeMedian5 = 0;
double timeNormal = 0;
for (int j = 0; j < trialTimes; j++)
{
int[] a = SortCompare.GetRandomArrayInt(arraySize);
int[] b = new int[a.Length];
int[] c = new int[a.Length];
a.CopyTo(b, 0);
a.CopyTo(c, 0);
timeNormal += SortCompare.Time(quickNormal, a);
timeMedian3 += SortCompare.Time(quickMedian3, b);
timeMedian5 += SortCompare.Time(quickMedian5, c);
}
timeMedian5 /= trialTimes;
timeMedian3 /= trialTimes;
timeNormal /= trialTimes;
Console.WriteLine(arraySize + "\t" + timeMedian5 + "\t" + timeMedian3 + "\t" + timeNormal + "\t" + timeMedian5 / timeNormal + "\t" + timeMedian5/timeMedian3);
arraySize *= 2;
}
}
}
}另请参阅
Quick 库
Code to calculate “median of five” in C#
2.3.20
解答
事实上就是用一个栈保存每次切分后的子数组下标。
关键代码如下,这里用到的栈(链栈)是在 1.3 中构建的:
///
/// 用快速排序对数组 a 进行升序排序。
///
/// 需要排序的类型。
/// 需要排序的数组。
public override void Sort(T[] a)
{
Shuffle(a);
Stack stack = new Stack();
stack.Push(0);
stack.Push(a.Length - 1);
while (!stack.IsEmpty())
{
// 压入顺序是先 lo,再 hi,故弹出顺序是先 hi 再 lo
int hi = stack.Pop();
int lo = stack.Pop();
if (hi <= lo)
continue;
int j = Partition(a, lo, hi);
// 让较大的子数组先入栈(先排序较小的子数组)
if (j - lo > hi - j)
{
stack.Push(lo);
stack.Push(j - 1);
stack.Push(j + 1);
stack.Push(hi);
}
else
{
stack.Push(j + 1);
stack.Push(hi);
stack.Push(lo);
stack.Push(j - 1);
}
}
Debug.Assert(IsSorted(a));
} 由于栈操作比函数调用操作耗费时间更长,因此测试后的结果会比原有快排慢 20% 左右。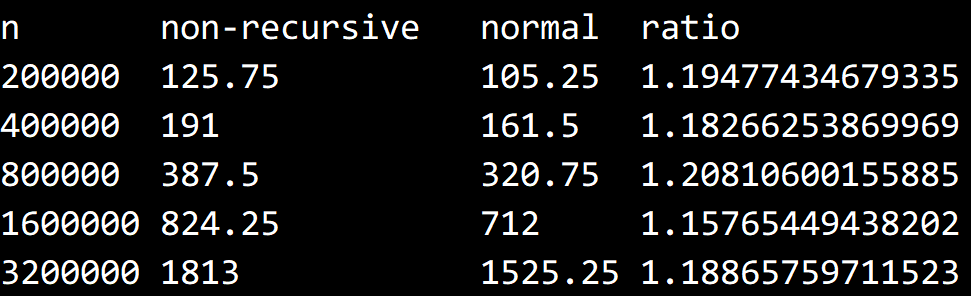
代码
QuickSortNonRecursive
using System;
using System.Diagnostics;
using Quick;
namespace _2._3._20
{
///
/// 快速排序类。
///
public class QuickSortNonRecursive : BaseSort
{
///
/// 默认构造函数。
///
public QuickSortNonRecursive() { }
///
/// 用快速排序对数组 a 进行升序排序。
///
/// 需要排序的类型。
/// 需要排序的数组。
public override void Sort(T[] a)
{
Shuffle(a);
Stack stack = new Stack();
stack.Push(0);
stack.Push(a.Length - 1);
while (!stack.IsEmpty())
{
// 压入顺序是先 lo,再 hi,故弹出顺序是先 hi 再 lo
int hi = stack.Pop();
int lo = stack.Pop();
if (hi <= lo)
continue;
int j = Partition(a, lo, hi);
// 让较大的子数组先入栈(先排序较小的子数组)
if (j - lo > hi - j)
{
stack.Push(lo);
stack.Push(j - 1);
stack.Push(j + 1);
stack.Push(hi);
}
else
{
stack.Push(j + 1);
stack.Push(hi);
stack.Push(lo);
stack.Push(j - 1);
}
}
Debug.Assert(IsSorted(a));
}
///
/// 对数组进行切分,返回枢轴位置。
///
/// 需要切分的数组类型。
/// 需要切分的数组。
/// 切分的起始点。
/// 切分的末尾点。
/// 枢轴下标。
private int Partition(T[] a, int lo, int hi) where T : IComparable
{
int i = lo, j = hi + 1;
T v = a[lo];
while (true)
{
while (Less(a[++i], v))
if (i == hi)
break;
while (Less(v, a[--j]))
if (j == lo)
break;
if (i >= j)
break;
Exch(a, i, j);
}
Exch(a, lo, j);
return j;
}
///
/// 打乱数组。
///
/// 需要打乱的数组类型。
/// 需要打乱的数组。
private void Shuffle(T[] a)
{
Random random = new Random();
for (int i = 0; i < a.Length; i++)
{
int r = i + random.Next(a.Length - i);
T temp = a[i];
a[i] = a[r];
a[r] = temp;
}
}
}
} 测试用例
using System;
using Quick;
namespace _2._3._20
{
/*
* 2.3.20
*
* 非递归的快速排序。
* 实现一个非递归的快速排序,
* 使用一个循环来将弹出栈的子数组切分并将结果子数组重新压入栈。
* 注意:
* 先将较大的子数组压入栈,这样就可以保证栈最多只会有 lgN 个元素。
*
*/
class Program
{
static void Main(string[] args)
{
QuickSort quickNormal = new QuickSort();
QuickSortNonRecursive quickNonRecursive = new QuickSortNonRecursive();
int arraySize = 200000; // 初始数组大小。
const int trialTimes = 4; // 每次实验的重复次数。
const int trialLevel = 5; // 双倍递增的次数。
Console.WriteLine("n\tnon-recursive\tnormal\tratio");
for (int i = 0; i < trialLevel; i++)
{
double timeRecursive = 0;
double timeNormal = 0;
for (int j = 0; j < trialTimes; j++)
{
int[] a = SortCompare.GetRandomArrayInt(arraySize);
int[] b = new int[a.Length];
a.CopyTo(b, 0);
timeNormal += SortCompare.Time(quickNormal, b);
timeRecursive += SortCompare.Time(quickNonRecursive, a);
}
timeRecursive /= trialTimes;
timeNormal /= trialTimes;
Console.WriteLine(arraySize + "\t" + timeRecursive + "\t\t" + timeNormal + "\t" + timeRecursive / timeNormal);
arraySize *= 2;
}
}
}
}用到的栈的实现
using System;
using System.Collections;
using System.Collections.Generic;
using System.Text;
namespace _2._3._20
{
///
/// 栈类。
///
/// 栈中存放的元素类型。
public class Stack- : IEnumerable
-
{
private Node
- first;
private int count;
///
/// 默认构造函数。
///
public Stack()
{
this.first = null;
this.count = 0;
}
///
/// 复制构造函数。
///
///
public Stack(Stack- s)
{
if (s.first != null)
{
this.first = new Node
- (s.first);
for (Node
- x = this.first; x.next != null; x = x.next)
{
x.next = new Node
- (x.next);
}
}
this.count = s.count;
}
///
/// 检查栈是否为空。
///
///
/// 返回栈内元素的数量。
///
///
/// 将一个元素压入栈中。
///
/// 要压入栈中的元素。
public void Push(Item item)
{
Node- oldFirst = this.first;
this.first = new Node
- ();
this.first.item = item;
this.first.next = oldFirst;
this.count++;
}
///
/// 将一个元素从栈中弹出,返回弹出的元素。
///
///
/// 返回栈顶元素(但不弹出它)。
///
///
/// 将两个栈连接。
///
/// 第一个栈。
/// 第二个栈(将被删除)。
/// - Catenation(Stack
- s1, Stack
- s2)
{
if (s1.IsEmpty())
{
s1.first = s2.first;
s1.count = s2.count;
}
else
{
Node
- last = s1.first;
while (last.next != null)
{
last = last.next;
}
last.next = s2.first;
s1.count += s2.count;
}
s2 = null;
return s1;
}
///
/// 创建栈的浅表副本。
///
/// - Copy()
{
Stack
- temp = new Stack
- ();
temp.first = this.first;
temp.count = this.count;
return temp;
}
public override string ToString()
{
StringBuilder s = new StringBuilder();
foreach (Item n in this)
{
s.Append(n);
s.Append(' ');
}
return s.ToString();
}
public IEnumerator
- GetEnumerator()
{
return new StackEnumerator(this.first);
}
IEnumerator IEnumerable.GetEnumerator()
{
return GetEnumerator();
}
private class StackEnumerator : IEnumerator
-
{
private Node
- current;
private Node
- first;
public StackEnumerator(Node
- first)
{
this.current = new Node
- ();
this.current.next = first;
this.first = this.current;
}
Item IEnumerator
- .Current => this.current.item;
object IEnumerator.Current => this.current.item;
void IDisposable.Dispose()
{
this.current = null;
this.first = null;
}
bool IEnumerator.MoveNext()
{
if (this.current.next == null)
return false;
this.current = this.current.next;
return true;
}
void IEnumerator.Reset()
{
this.current = this.first;
}
}
}
}
另请参阅
Quick 库
Generics 库
2.3.21
解答
首先引入命题 I 的结论,对于互不相同的主键值,基于比较的排序算法的下界等于所形成的比较树的高度,即:
\[ h \ge \log_2{N!} \]
那么我们题目即可转化为求证
\[ h \ge \log_2 (\frac{N!}{f_1!f_2!\cdots f_k!}) \ge \log_2 N! \]
这里的 $ f_i $ 为某个主键值出现的频率,即某个主键值出现的次数,因此 \(f_i\ge 1\) 。
根据题目给出的条件,如果主键互不重复,此时 $ k=N $,且 $ f_1=f_2=\cdots=f_k=1 $ 。
那么 $ f_1!f_2!\cdots f_k!=1 $ ,待证式子即为命题 I 的结论。
那么当主键有重复时,此时 $ k < N $,为使 $ f_1+f_2+ \cdots + f_k=N $ ,至少存在一个 $ f_m \ge 2 $。
故此时:
\[ f_1!f_2!\cdots f_k! >1\Rightarrow \frac{N!}{f_1!f_2!\cdots f_k!}
得证。
另请参阅
lower bounds of sorting-The University of Maryland
2.3.22
解答
官方实现见:https://algs4.cs.princeton.edu/23quicksort/QuickBentleyMcIlroy.java.html
快速三向切分
论文引用见「另请参阅」部分。
算法演示
Ninther 算法
官方实现中用到了 Ninther 算法用于选取近似中位数(作为枢轴),
该算法由 John Tukey 在 1978 年提出,论文引用见「另请参阅」部分。
这个算法的思想其实很简单,假设我们有三个数 $ y_1, y_2, y_3 $ ,那么其中位数为:
\[ y_A= {\rm median}\lbrace y_1,y_2,y_3 \rbrace \]
现在对于九个数,我们以三个为一组,取三个中位数:
\[ y_A= {\rm median}\lbrace y_1,y_2,y_3 \rbrace \\ y_B= {\rm median}\lbrace y_4,y_5,y_6 \rbrace \\ y_C= {\rm median}\lbrace y_7,y_8,y_9 \rbrace \]
接下来取这三个中位数的中位数,有:
\[ y_E= {\rm median}\lbrace y_A,y_B,y_C \rbrace \]
我们把上述过程封装成函数,即 $ y_E= {\rm ninther}\lbrace y_1,y_2,\cdots,y_9 \rbrace $ 。
于是我们获得的 $ y_E $ 即为近似中位数,如果 $ \lbrace y_1,y_2,\cdots,y_9 \rbrace $ 是单调数列,那么 $ y_E $ 就是中位数。
获取三个数中的中位数
事实上,我们可以直接画出三个数排列的所有可能,获得决策树。
然后根据决策树写出取中位数的算法:
private int Median3(T[] a, int i, int j, int k) where T : IComparable
{
return
(Less(a[i], a[j]) ?
(Less(a[j], a[k]) ? j : Less(a[i], a[k]) ? k : i) :
(Less(a[k], a[j]) ? j : Less(a[k], a[i]) ? k : i));
} 测试结果
代码
QuickBentleyMcIlroy
using System;
using System.Diagnostics;
namespace Quick
{
public class QuickBentleyMcIlroy : BaseSort
{
///
/// 小于这个数值的数组调用插入排序。
///
private readonly int INSERTION_SORT_CUTOFF = 8;
///
/// 小于这个数值的数组调用中位数作为枢轴。
///
private readonly int MEDIAN_OF_3_CUTOFF = 40;
///
/// 默认构造函数。
///
public QuickBentleyMcIlroy() { }
///
/// 用快速排序对数组 a 进行升序排序。
///
/// 需要排序的类型。
/// 需要排序的数组。
public override void Sort(T[] a)
{
Sort(a, 0, a.Length - 1);
Debug.Assert(IsSorted(a));
}
///
/// 对指定范围内的数组进行排序。
///
/// 需要排序的类型。
/// 需要排序的数组。
/// 排序的起始下标。
/// 排序的终止下标。
private void Sort(T[] a, int lo, int hi) where T : IComparable
{
int n = hi - lo + 1;
if (n <= this.INSERTION_SORT_CUTOFF)
{
InsertionSort(a, lo, hi);
return;
}
else if (n <= this.MEDIAN_OF_3_CUTOFF)
{
// 对于较小的数组,直接选择左中右三个元素中的中位数作为枢轴。
int m = Median3(a, lo, lo + n / 2, hi);
Exch(a, m, lo);
}
else
{
// 对于较大的数组使用 Turkey Ninther 作为枢轴。
int eps = n / 8;
int mid = lo + n / 2;
int m1 = Median3(a, lo, lo + eps, lo + eps + eps);
int m2 = Median3(a, mid - eps, mid, mid + eps);
int m3 = Median3(a, hi - eps - eps, hi - eps, hi);
int ninther = Median3(a, m1, m2, m3);
Exch(a, ninther, lo);
}
// 三向切分
int i = lo, j = hi + 1;
int p = lo, q = hi + 1;
T v = a[lo];
while (true)
{
while (Less(a[++i], v))
if (i == hi)
break;
while (Less(v, a[--j]))
if (j == lo)
break;
if (i == j && IsEqual(a[i], v))
Exch(a, ++p, i);
if (i >= j)
break;
Exch(a, i, j);
if (IsEqual(a[i], v))
Exch(a, ++p, i);
if (IsEqual(a[j], v))
Exch(a, --q, j);
}
i = j + 1;
for (int k = lo; k <= p; k++)
Exch(a, k, j--);
for (int k = hi; k >= q; k--)
Exch(a, k, i++);
Sort(a, lo, j);
Sort(a, i, hi);
}
///
/// 判断两个元素是否值相等。
///
/// 需要判断的元素类型。
/// 进行比较的第一个元素。
/// 进行比较的第二个元素。
/// 两个元素的值是否相等。
private bool IsEqual(T a, T b) where T : IComparable
{
return a.CompareTo(b) == 0;
}
///
/// 用插入排序对指定范围内的数组排序。
///
/// 数组的元素类型。
/// 需要排序的数组。
/// 排序的起始下标。
/// 排序的终止下标。
private void InsertionSort(T[] a, int lo, int hi) where T : IComparable
{
for (int i = lo; i <= hi; i++)
{
for (int j = i; j > lo && Less(a[j], a[j - 1]); j--)
{
Exch(a, j, j - 1);
}
}
}
///
/// 获取三个元素中的中位数。
///
/// 用于排序的元素。
/// 需要排序的数组。
/// 第一个待选元素的下标。
/// 第二个待选元素的下标。
/// 第三个待选元素的下标。
/// (T[] a, int i, int j, int k) where T : IComparable
{
return
(Less(a[i], a[j]) ?
(Less(a[j], a[k]) ? j : Less(a[i], a[k]) ? k : i) :
(Less(a[k], a[j]) ? j : Less(a[k], a[i]) ? k : i));
}
}
} 测试用例
using System;
using Quick;
namespace _2._3._22
{
/*
* 2.3.22
*
* 快速三向切分。(J.Bently,D.McIlroy)
* 用将重复元素放置于子数组两端的方式实现一个信息量最优的排序算法。
* 使用两个索引 p 和 q,使得 a[lo...p-1] 和 a[q+1..hi] 的元素都和 a[lo] 相等。
* 使用另外两个索引 i 和 j,
* 使得 a[p...i-1] 小于 a[lo],a[j+i..q] 大于 a[lo]。
* 在内循环中加入代码,在 a[i] 和 v 相当时将其与 a[p] 交换(并将 p 加 1),
* 在 a[j] 和 v 相等且 a[i] 和 a[j] 尚未和 v 进行比较之前将其与 a[q] 交换。
* 添加在切分循环结束后将和 v 相等的元素交换到正确位置的代码,如图 2.3.6 所示。
* 请注意:
* 这里实现的代码和正文中给出的代码时等价的,
* 因为这里额外的交换用于和切分元素相等的元素,
* 而正文中的代码将额外的交换用于和切分元素不等的元素。
*
*/
class Program
{
static void Main(string[] args)
{
QuickSort quickNormal = new QuickSort();
QuickBentleyMcIlroy quickBentleyMcIlroy = new QuickBentleyMcIlroy();
int arraySize = 800000; // 初始数组大小。
const int trialTimes = 1; // 每次实验的重复次数。
const int trialLevel = 8; // 双倍递增的次数。
Console.WriteLine("n\t\t3way\tnormal\tratio");
for (int i = 0; i < trialLevel; i++)
{
double timeBentleyMcIlroy = 0;
double timeNormal = 0;
for (int j = 0; j < trialTimes; j++)
{
int[] a = SortCompare.GetRandomArrayInt(arraySize);
int[] b = new int[a.Length];
a.CopyTo(b, 0);
timeNormal += SortCompare.Time(quickNormal, b);
timeBentleyMcIlroy += SortCompare.Time(quickBentleyMcIlroy, a);
}
timeBentleyMcIlroy /= trialTimes;
timeNormal /= trialTimes;
if (arraySize < 10000000)
Console.WriteLine(arraySize + "\t\t" + timeBentleyMcIlroy + "\t" + timeNormal + "\t" + timeBentleyMcIlroy / timeNormal);
else
Console.WriteLine(arraySize + "\t" + timeBentleyMcIlroy + "\t" + timeNormal + "\t" + timeBentleyMcIlroy / timeNormal);
arraySize *= 2;
}
}
}
}另请参阅
有关这种快速排序算法的来源以及三个数的中位数的选取算法,请参阅下面这篇 1993 年的论文:
Bentley J L, McIlroy M D. Engineering a sort function[J]. Software: Practice and Experience, 1993, 23(11): 1249-1265.
下面这份 2002 年的 PPT 详细解释和分析了官方实现代码的思路和性能:
Sedgewick R, Bentley J. Quicksort is optimal[J]. Knuthfest, Stanford University, Stanford, 2002.
有关选取中位数 Ninther 算法,请参阅下面这篇 1978 年的论文:
Tukey J W. The ninther, a technique for low-effort robust (resistant) location in large samples[M]//Contributions to Survey Sampling and Applied Statistics. 1978: 251-257.
以及按照惯例给出本题用到的类库链接:
Quick 库
2.3.23
解答
官方实现见:https://algs4.cs.princeton.edu/23quicksort/QuickBentleyMcIlroy.java.html
见 2.3.22 的解答,其中已经包含了这些改动。
代码
QuickBentleyMcIlroy
using System;
using System.Diagnostics;
namespace Quick
{
public class QuickBentleyMcIlroy : BaseSort
{
///
/// 小于这个数值的数组调用插入排序。
///
private readonly int INSERTION_SORT_CUTOFF = 8;
///
/// 小于这个数值的数组调用中位数作为枢轴。
///
private readonly int MEDIAN_OF_3_CUTOFF = 40;
///
/// 默认构造函数。
///
public QuickBentleyMcIlroy() { }
///
/// 用快速排序对数组 a 进行升序排序。
///
/// 需要排序的类型。
/// 需要排序的数组。
public override void Sort(T[] a)
{
Sort(a, 0, a.Length - 1);
Debug.Assert(IsSorted(a));
}
///
/// 对指定范围内的数组进行排序。
///
/// 需要排序的类型。
/// 需要排序的数组。
/// 排序的起始下标。
/// 排序的终止下标。
private void Sort(T[] a, int lo, int hi) where T : IComparable
{
int n = hi - lo + 1;
if (n <= this.INSERTION_SORT_CUTOFF)
{
InsertionSort(a, lo, hi);
return;
}
else if (n <= this.MEDIAN_OF_3_CUTOFF)
{
// 对于较小的数组,直接选择左中右三个元素中的中位数作为枢轴。
int m = Median3(a, lo, lo + n / 2, hi);
Exch(a, m, lo);
}
else
{
// 对于较大的数组使用 Turkey Ninther 作为枢轴。
int eps = n / 8;
int mid = lo + n / 2;
int m1 = Median3(a, lo, lo + eps, lo + eps + eps);
int m2 = Median3(a, mid - eps, mid, mid + eps);
int m3 = Median3(a, hi - eps - eps, hi - eps, hi);
int ninther = Median3(a, m1, m2, m3);
Exch(a, ninther, lo);
}
// 三向切分
int i = lo, j = hi + 1;
int p = lo, q = hi + 1;
T v = a[lo];
while (true)
{
while (Less(a[++i], v)) ;
while (Less(v, a[--j]))
if (j == lo)
break;
if (i == j && IsEqual(a[i], v))
Exch(a, ++p, i);
if (i >= j)
break;
Exch(a, i, j);
if (IsEqual(a[i], v))
Exch(a, ++p, i);
if (IsEqual(a[j], v))
Exch(a, --q, j);
}
i = j + 1;
for (int k = lo; k <= p; k++)
Exch(a, k, j--);
for (int k = hi; k >= q; k--)
Exch(a, k, i++);
Sort(a, lo, j);
Sort(a, i, hi);
}
///
/// 判断两个元素是否值相等。
///
/// 需要判断的元素类型。
/// 进行比较的第一个元素。
/// 进行比较的第二个元素。
/// 两个元素的值是否相等。
private bool IsEqual(T a, T b) where T : IComparable
{
return a.CompareTo(b) == 0;
}
///
/// 用插入排序对指定范围内的数组排序。
///
/// 数组的元素类型。
/// 需要排序的数组。
/// 排序的起始下标。
/// 排序的终止下标。
private void InsertionSort(T[] a, int lo, int hi) where T : IComparable
{
for (int i = lo; i <= hi; i++)
{
for (int j = i; j > lo && Less(a[j], a[j - 1]); j--)
{
Exch(a, j, j - 1);
}
}
}
///
/// 获取三个元素中的中位数。
///
/// 用于排序的元素。
/// 需要排序的数组。
/// 第一个待选元素的下标。
/// 第二个待选元素的下标。
/// 第三个待选元素的下标。
/// (T[] a, int i, int j, int k) where T : IComparable
{
return
(Less(a[i], a[j]) ?
(Less(a[j], a[k]) ? j : Less(a[i], a[k]) ? k : i) :
(Less(a[k], a[j]) ? j : Less(a[k], a[i]) ? k : i));
}
}
} 另请参阅
Quick 库
2.3.24
解答
取样排序的想法很简单:
常规快排的枢轴只有一个。
如果用一个数组来充当枢轴,根据排序位置的不同自动选择对应的枢轴,
显然能够更好的估计中位数,以求更好的切分效果。
于是引入了「取样」的概念,假如我们从源数组中随机取了 3 个元素并对其排序,
那么这 3 个元素的中位数可以作为第一次切分的枢轴,剩余两个元素则可以充当切分后两个子数组的枢轴。
那么当取样元素到达一个合适的数量时,就能达到提升切分效率的目标。
大致思路如下:
首先先从输入数组里随机取一些元素,作为「取样数组」。
用任意排序算法(比如快排)对取样数组进行排序。
(由于取样数组通常都比较小,这一步的时间消耗通常不会影响性能)
取出取样数组里面的中位数,当作枢轴对剩下的数组进行切分。
之后的切分中,根据排序区间在剩余数组中的相对位置,
用取样数组中对应位置的数作为枢轴,直到整个排序完成。
论文里提到了两种实现方式。
第一种方法
取样数组和剩余数组是分开保存的。
每次切分完成后,并不把枢轴放入剩余数组中,
而是等到剩余数组全部排序完毕之后再用一次归并(merge)操作将取样数组和剩余数组归并。
第二种方法
取样数组和剩余数组保存在同一片空间里,这也是这份题解所实现的方法。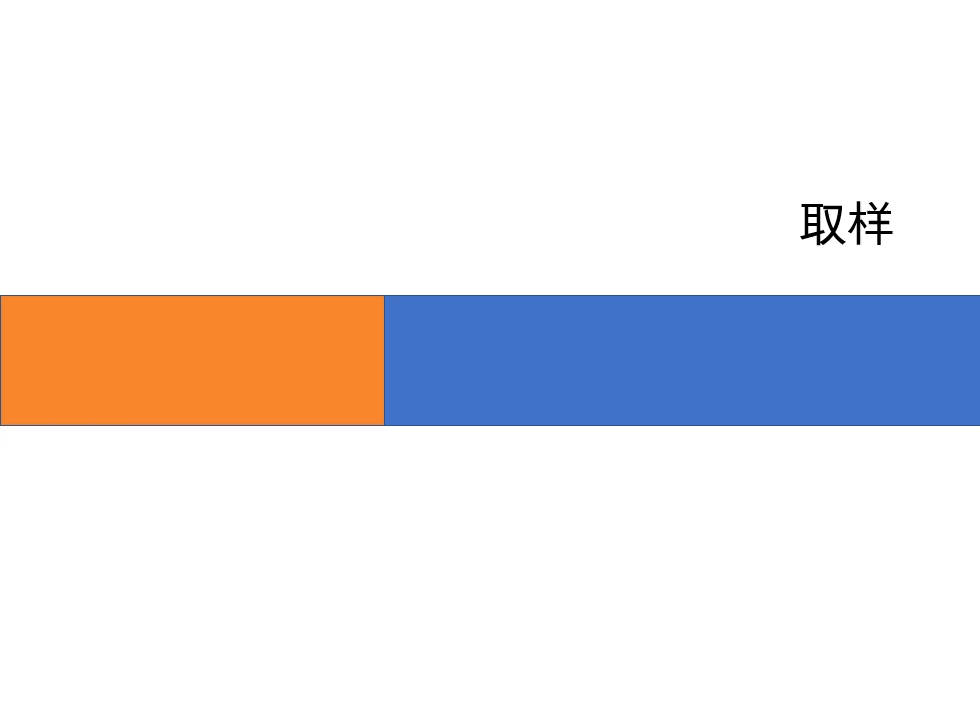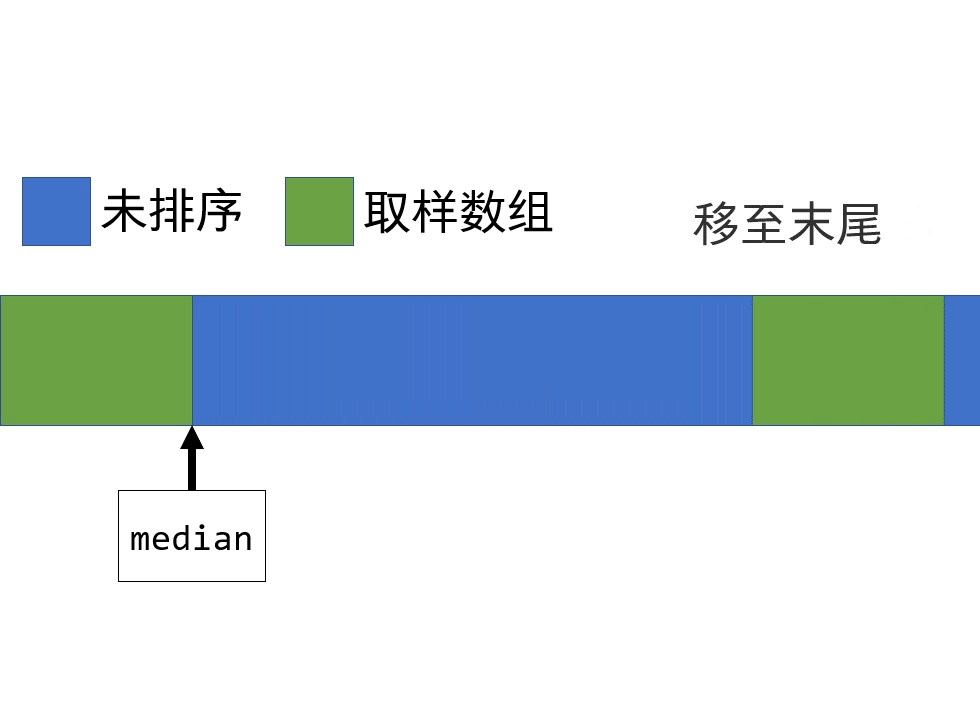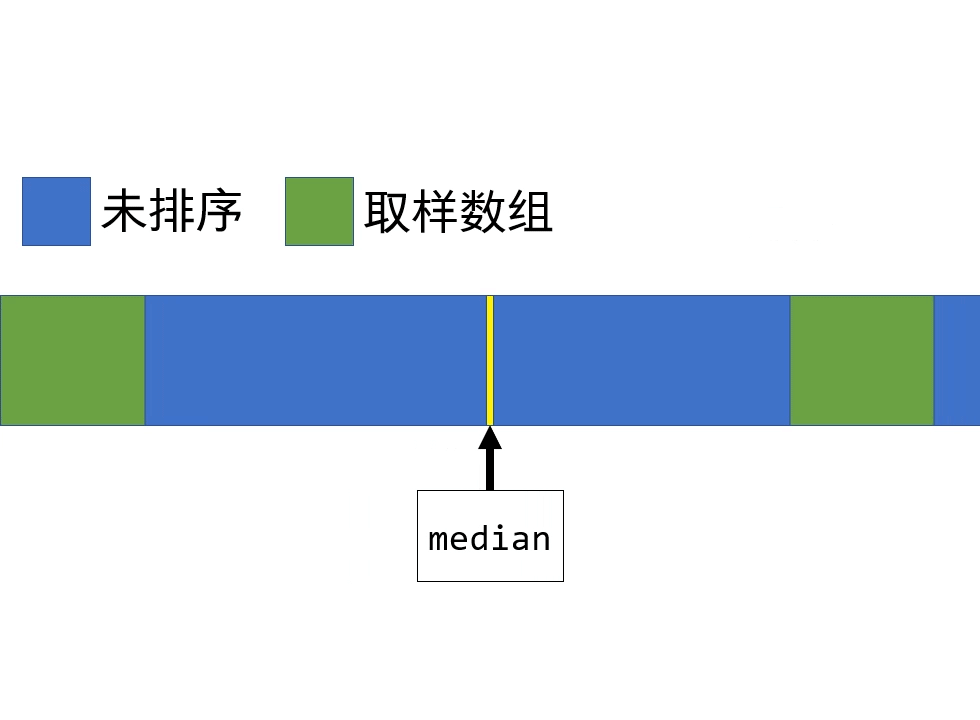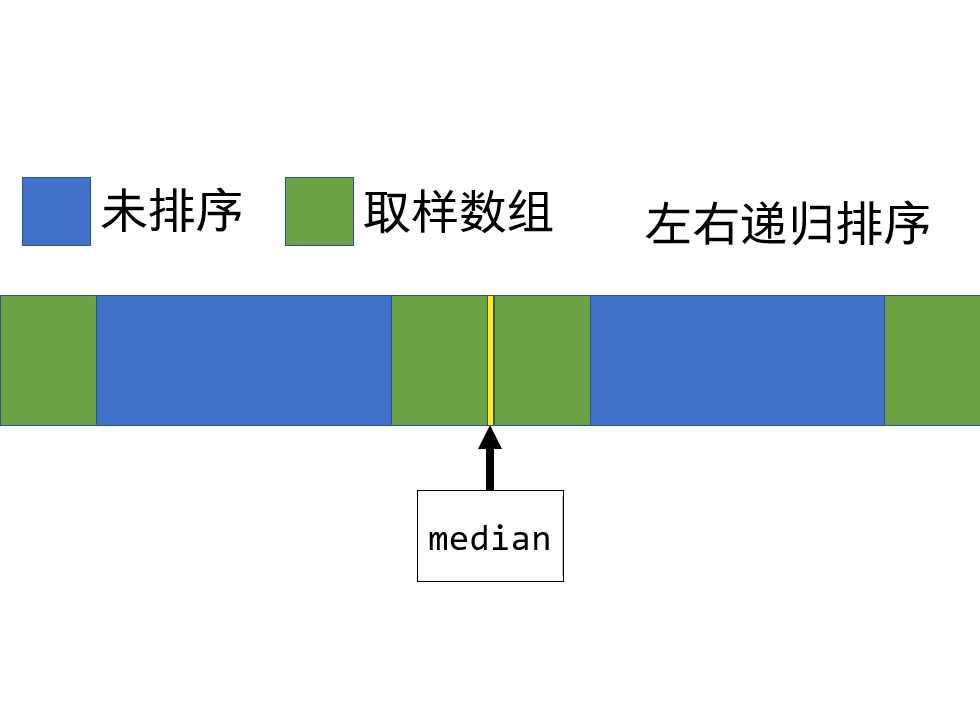
在打乱输入数组之后,取前 2^k-1 个元素作为取样数组,用快排对其排序。
然后把取样数组的后半部分放到整个数组的末尾。
这样操作的结果是输入数组分为了四个部分:
有序的取样数组、取样数组的中位数、无序的剩余数组、有序的取样数组。
中位数则位于第一部分的末尾,我们将其作为枢轴对剩余数组进行切分,数组变为:
有序的取样数组、小于中位数的部分、枢轴、大于中位数的部分、有序的取样数组
接下来我们再对第一个部分取半,放到中位数之前;对最后一部分取半,放到中位数之后:
0 ~ 1/4 取样数组、小于中位数、1/4 ~ 1/2 取样数组、枢轴、1/2~3/4 取样数组、大于中位数、3/4~1 取样数组
你会发现枢轴前后又分别变回了初始条件,递归执行上述操作,便能对整个数组排序。
注意当取样数组用完的时候,直接变回普通的快排。
现代的取样排序
这里的「现代」并不意味着更好,只是让取样排序能更好的适应多线程排序。
首先仍然是取样,取样的数量往往取决于线程的数量,比如说取了 p-1 个,就将数组分为 p 份。
对取样数组进行排序,获得 p 个区间(桶)。
遍历输入的数组,把元素扔到相应的桶里面。
把每个桶和对应的枢轴送到对应的线程进行排序。
汇总各个桶中的结果,排序完毕。
代码
using System;
using System.Diagnostics;
namespace Quick
{
///
/// 取样排序类。
///
public class SampleSort : QuickSort
{
///
/// 取样数组长度 2^k - 1 的阶数。
///
public int K { get; set; }
///
/// 默认构造函数。
///
public SampleSort()
{
this.K = 8;
}
///
/// 用快速排序对数组 a 进行升序排序。
///
/// 需要排序的类型。
/// 需要排序的数组。
public override void Sort(T[] a)
{
if (a.Length < Math.Pow(2, this.K + 1))
{
// 小于 2^(k+1) 的数组直接进行快排
base.Sort(a);
return;
}
Shuffle(a);
int samplehi = (int)Math.Pow(2, this.K) - 2;
// 利用快速排序对取样数组进行排序
base.Sort(a, 0, samplehi);
// 找到取样数组的中位数
int sampleMedian = samplehi / 2;
// 将取样数组后半部分放到数组末尾
int i = samplehi, j = a.Length - 1;
while (i != sampleMedian)
Exch(a, i--, j--);
// 根据取样数组进行排序
Sort(a, 0, sampleMedian, j, a.Length - 1);
Debug.Assert(IsSorted(a));
}
///
/// 用快速排序对数组 a 的 lo ~ hi 范围排序。
///
/// 需要排序的数组类型。
/// 需要排序的数组。
/// 取样数组的起始下标。
/// 排序范围的起始下标。
/// 排序范围的结束下标。
/// 取样数组的终止下标。
private void Sort(T[] a, int samplelo, int lo, int hi, int samplehi) where T : IComparable
{
if (hi <= lo) // 别越界
return;
int j = Partition(a, lo, hi);
// 将前部的有序取样数组取半,后半部分放在枢轴前面。
if (lo - samplelo > 1)
{
// p 应该始终指向有序部分的最后一项
// v 应该始终指向有序部分的前面一项
int p = lo - 1, v = j - 1;
for (int i = 0; i < (lo - samplelo) / 2; i++)
{
Exch(a, p--, v--);
}
Sort(a, samplelo, p, v, j - 1);
}
else
{
// 取样数组已经用完,退化为普通 Quicksort
base.Sort(a, samplelo, j - 1);
}
// 将尾部有序取样数组取半,前半部分放在枢轴后面。
if (samplehi - hi > 1)
{
// p 应该始终指向有序部分的前面一项
// v 应该始终指向有序部分的最后一项
int p = hi, v = j;
for (int i = 0; i < (samplehi - hi) / 2; i++)
{
Exch(a, ++p, ++v);
}
Sort(a, j + 1, v, p, samplehi);
}
else
{
// 取样数组已用完,退化为普通 Quicksort
base.Sort(a, j + 1, samplehi);
}
}
///
/// 对数组进行切分,返回枢轴位置。
///
/// 需要切分的数组类型。
/// 需要切分的数组。
/// 切分的起始点。
/// 切分的末尾点。
/// 枢轴下标。
private int Partition(T[] a, int lo, int hi) where T : IComparable
{
int i = lo, j = hi + 1;
T v = a[lo];
while (true)
{
while (Less(a[++i], v))
if (i == hi)
break;
while (Less(v, a[--j]))
if (j == lo)
break;
if (i >= j)
break;
Exch(a, i, j);
}
Exch(a, lo, j);
return j;
}
///
/// 打乱数组。
///
/// 需要打乱的数组类型。
/// 需要打乱的数组。
private void Shuffle(T[] a)
{
Random random = new Random();
for (int i = 0; i < a.Length; i++)
{
int r = i + random.Next(a.Length - i);
T temp = a[i];
a[i] = a[r];
a[r] = temp;
}
}
}
} 测试用例:
using System;
using Quick;
namespace _2._3._24
{
/*
* 2.3.24
*
* 取样排序。(W.Frazer,A.McKellar)
* 实现一个快速排序,
* 取样大小为 2^k-1。首先将取样得到的元素排序,
* 然后在递归函数中使用样品的中位数切分。
* 分为两部分的其余样品元素无需再次排序并可以分别应用于原数组的两个子数组。
* 这种算法称为取样排序。
*
*/
class Program
{
static void Main(string[] args)
{
QuickSort quickNormal = new QuickSort();
SampleSort sampleSort = new SampleSort();
int arraySize = 1600000; // 初始数组大小。
const int kSteps = 10; // 取样 k 值的递增次数。
const int trialTimes = 1; // 每次实验的重复次数。
const int trialLevel = 2; // 双倍递增的次数。
Console.WriteLine("k\tn\t\tsample\tnormal\tratio");
for (int i = 0; i < kSteps; i++)
{
int array = arraySize;
for (int j = 0; j < trialLevel; j++)
{
double timeSample = 0;
double timeNormal = 0;
for (int k = 0; k < trialTimes; k++)
{
int[] a = SortCompare.GetRandomArrayInt(array);
int[] b = new int[a.Length];
a.CopyTo(b, 0);
timeNormal += SortCompare.Time(quickNormal, b);
timeSample += SortCompare.Time(sampleSort, a);
}
timeSample /= trialTimes;
timeNormal /= trialTimes;
if (arraySize < 10000000)
Console.WriteLine(sampleSort.K + "\t" + array + "\t\t" + timeSample + "\t" + timeNormal + "\t" + timeSample / timeNormal);
else
Console.WriteLine(sampleSort.K + "\t" + array + "\t" + timeSample + "\t" + timeNormal + "\t" + timeSample / timeNormal);
array *= 2;
}
sampleSort.K++;
}
}
}
}另请参阅
关于取样排序的论文(1970 年):
Frazer W D, McKellar A C. Samplesort: A sampling approach to minimal storage tree sorting[J]. Journal of the ACM (JACM), 1970, 17(3): 496-507.
维基百科中的取样排序:
Samplesort-Wikipedia
本题用到的类库链接:
Quick 库
2.3.25
解答
切换到插入排序的实现比较简单,在类内添加一个成员变量 M,在 Sort 方法里添加如下代码:
protected void Sort(T[] a, int lo, int hi) where T: IComparable
{
if (hi <= lo) // 别越界
return;
if (hi - lo <= this.M)
{
// 调用插入排序
for (int i = lo; i <= hi; i++)
for (int k = i; k > lo && Less(a[k], a[k - 1]); k--)
Exch(a, k, k - 1);
return;
}
int j = Partition(a, lo, hi);
Sort(a, lo, j - 1);
Sort(a, j + 1, hi);
} 下面放上实验结果:
N=1000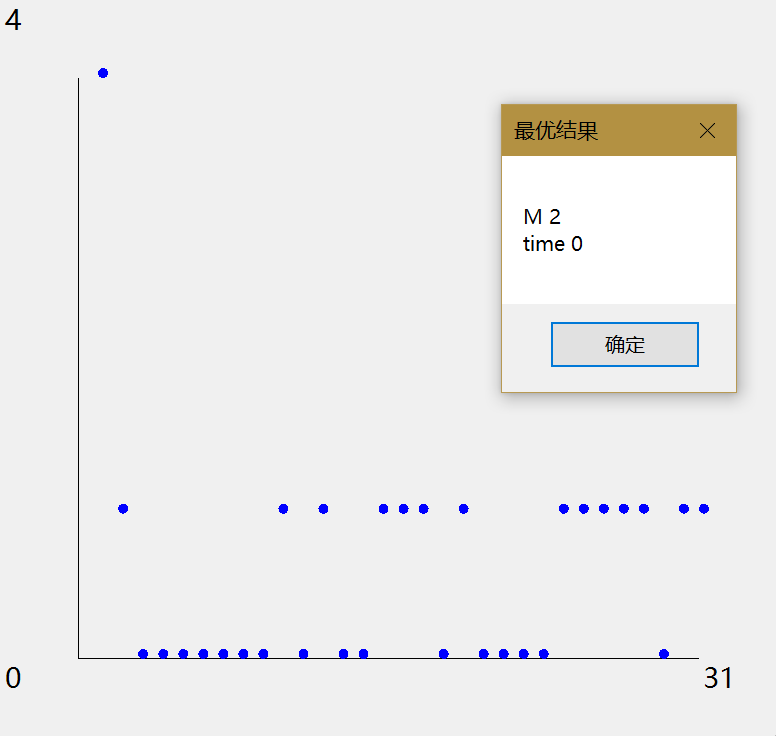
N=10000
N=100000
N=1000000
小于 8 的 M 值会比较合适。
代码
这里使用了 Background Worker 来防止程序失去响应,更多信息可以看 「另请参阅」部分。
using System;
using System.ComponentModel;
using System.Drawing;
using System.Linq;
using System.Windows.Forms;
using Quick;
namespace _2._3._25
{
public partial class Form2 : Form
{
///
/// 测试数组大小。
///
public int N = 100;
public Form2(int n)
{
InitializeComponent();
this.N = n;
}
///
/// 启动页面时启动后台测试。
///
///
///
private void Form2_Shown(object sender, EventArgs e)
{
this.Text = "正在绘图";
this.backgroundWorker1.RunWorkerAsync();
}
///
/// 后台测试方法。
///
///
///
private void backgroundWorker1_DoWork(object sender, DoWorkEventArgs e)
{
BackgroundWorker worker = sender as BackgroundWorker;
QuickSortInsertion quickSortInsertion = new QuickSortInsertion();
double[] timeRecord = new double[31];
for (int i = 0; i <= 30; i++)
{
worker.ReportProgress(i * 3);
quickSortInsertion.M = i;
int[] data = SortCompare.GetRandomArrayInt(this.N);
timeRecord[i] = SortCompare.Time(quickSortInsertion, data);
}
e.Result = timeRecord;
}
///
/// 更新后台进度方法。
///
///
///
private void backgroundWorker1_ProgressChanged(object sender, ProgressChangedEventArgs e)
{
this.Text = "正在绘图,已完成 " + e.ProgressPercentage + " %";
}
///
/// 测试完毕,进行绘图的方法。
///
///
///
private void backgroundWorker1_RunWorkerCompleted(object sender, RunWorkerCompletedEventArgs e)
{
if (e.Error != null)
{
MessageBox.Show(e.Error.Message);
}
double[] result = e.Result as double[];
Graphics graphics = this.CreateGraphics();
// 获得绘图区矩形。
RectangleF rect = this.ClientRectangle;
float unitX = rect.Width / 10;
float unitY = rect.Width / 10;
// 添加 10% 边距作为文字区域。
RectangleF center = new RectangleF
(rect.X + unitX, rect.Y + unitY,
rect.Width - 2 * unitX, rect.Height - 2 * unitY);
// 绘制坐标系。
graphics.DrawLine(Pens.Black, center.Left, center.Top, center.Left, center.Bottom);
graphics.DrawLine(Pens.Black, center.Left, center.Bottom, center.Right, center.Bottom);
graphics.DrawString(result.Max().ToString(), this.Font, Brushes.Black, rect.Location);
graphics.DrawString(result.Length.ToString(), this.Font, Brushes.Black, center.Right, center.Bottom);
graphics.DrawString("0", this.Font, Brushes.Black, rect.Left, center.Bottom);
// 初始化点。
PointF[] bluePoints = new PointF[result.Length];
unitX = center.Width / result.Length;
unitY = center.Height / (float)result.Max();
for (int i = 0; i < result.Length; i++)
{
bluePoints[i] = new PointF(center.Left + unitX * (i + 1), center.Bottom - (float)(result[i] * unitY) - 10);
}
// 绘制点。
for (int i = 0; i < result.Length; i++)
{
graphics.FillEllipse(Brushes.Blue, new RectangleF(bluePoints[i], new Size(10, 10)));
}
graphics.Dispose();
this.Text = "绘图结果";
int min = 0;
for (int i = 0; i < result.Length; i++)
{
if (result[i] < result[min])
min = i;
}
string report = "M " + min + "\r\ntime " + result[min];
MessageBox.Show(report, "最优结果");
}
}
}快速排序类
using System;
using System.Diagnostics;
using Quick;
namespace _2._3._25
{
///
/// 快速排序类。
///
public class QuickSortInsertion : BaseSort
{
///
/// 切换到插入排序的阈值。
///
public int M { get; set; }
///
/// 默认构造函数。
///
public QuickSortInsertion()
{
this.M = 8;
}
///
/// 用快速排序对数组 a 进行升序排序。
///
/// 需要排序的类型。
/// 需要排序的数组。
public override void Sort(T[] a)
{
Shuffle(a);
Sort(a, 0, a.Length - 1);
Debug.Assert(IsSorted(a));
}
///
/// 用快速排序对数组 a 的 lo ~ hi 范围排序。
///
/// 需要排序的数组类型。
/// 需要排序的数组。
/// 排序范围的起始下标。
/// 排序范围的结束下标。
protected void Sort(T[] a, int lo, int hi) where T: IComparable
{
if (hi <= lo) // 别越界
return;
if (hi - lo <= this.M)
{
// 调用插入排序
for (int i = lo; i <= hi; i++)
for (int k = i; k > lo && Less(a[k], a[k - 1]); k--)
Exch(a, k, k - 1);
return;
}
int j = Partition(a, lo, hi);
Sort(a, lo, j - 1);
Sort(a, j + 1, hi);
}
///
/// 对数组进行切分,返回枢轴位置。
///
/// 需要切分的数组类型。
/// 需要切分的数组。
/// 切分的起始点。
/// 切分的末尾点。
/// 枢轴下标。
private int Partition(T[] a, int lo, int hi) where T : IComparable
{
int i = lo, j = hi + 1;
T v = a[lo];
while (true)
{
while (Less(a[++i], v))
if (i == hi)
break;
while (Less(v, a[--j]))
if (j == lo)
break;
if (i >= j)
break;
Exch(a, i, j);
}
Exch(a, lo, j);
return j;
}
///
/// 打乱数组。
///
/// 需要打乱的数组类型。
/// 需要打乱的数组。
private void Shuffle(T[] a)
{
Random random = new Random();
for (int i = 0; i < a.Length; i++)
{
int r = i + random.Next(a.Length - i);
T temp = a[i];
a[i] = a[r];
a[r] = temp;
}
}
}
} 另请参阅
BackgroundWorker 组件 | Microsoft Docs
Quick 库
2.3.26
解答
在切换为插入排序之前先记录一下当前子数组的大小。
在排序类内添加一个大小为 M+1 的数组,用于记录每种数组大小出现的次数。
结果如下(N=100000):
M=10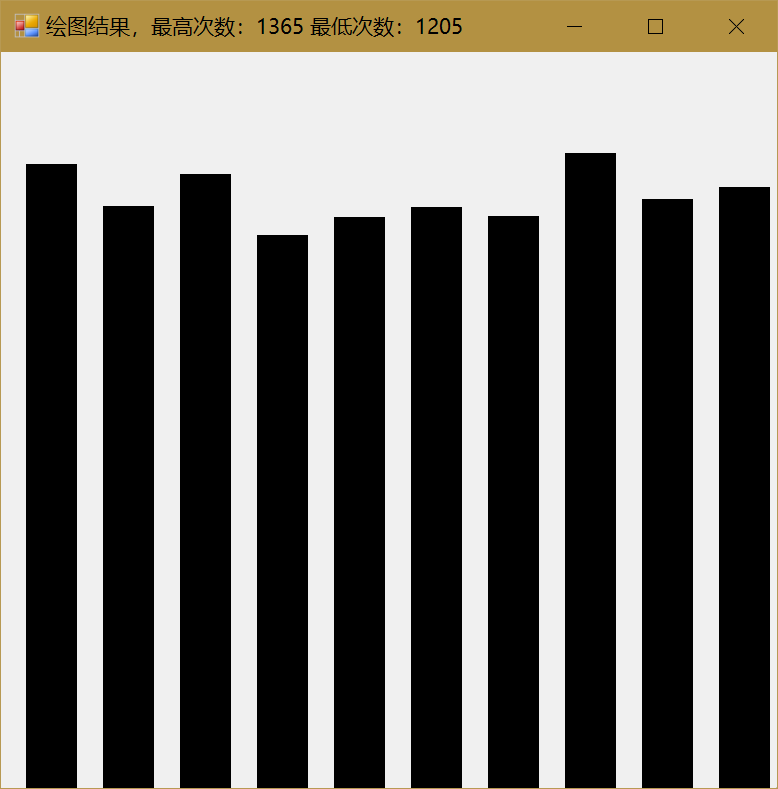
M=20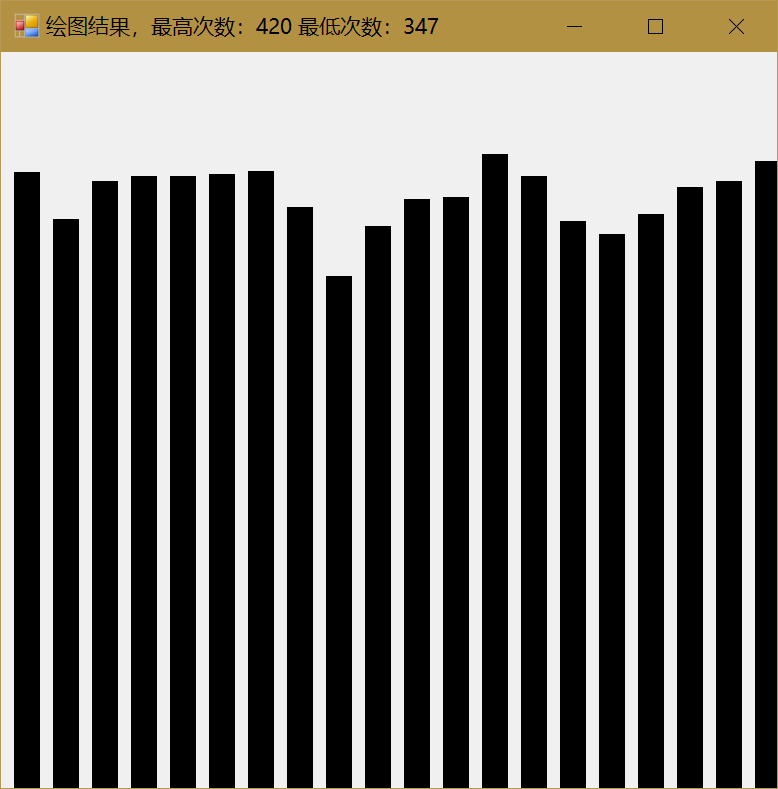
M=50
代码
using System;
using System.ComponentModel;
using System.Drawing;
using System.Linq;
using System.Windows.Forms;
using Quick;
namespace _2._3._26
{
public partial class Form2 : Form
{
private int M;
private int N;
public Form2(int m, int n)
{
InitializeComponent();
this.M = m;
this.N = n;
}
///
/// 启动页面时启动后台测试。
///
///
///
private void Form2_Shown(object sender, EventArgs e)
{
this.Text = "正在绘图";
this.backgroundWorker1.RunWorkerAsync();
}
///
/// 后台测试方法。
///
///
///
private void backgroundWorker1_DoWork(object sender, DoWorkEventArgs e)
{
BackgroundWorker worker = sender as BackgroundWorker;
QuickSortInsertion quickSortInsertion = new QuickSortInsertion
{
M = this.M
};
int[] data = SortCompare.GetRandomArrayInt(this.N);
worker.ReportProgress(50);
quickSortInsertion.Sort(data);
e.Result = quickSortInsertion.Counts;
}
///
/// 更新后台进度方法。
///
///
///
private void backgroundWorker1_ProgressChanged(object sender, ProgressChangedEventArgs e)
{
this.Text = "正在绘图,已完成 " + e.ProgressPercentage + " %";
}
///
/// 测试完毕,进行绘图的方法。
///
///
///
private void backgroundWorker1_RunWorkerCompleted(object sender, RunWorkerCompletedEventArgs e)
{
if (e.Error != null)
{
MessageBox.Show(e.Error.Message);
}
//新建画布
Graphics graphics = this.CreateGraphics();
//翻转默认坐标系
graphics.TranslateTransform(0, this.Height);
graphics.ScaleTransform(1, -1);
int[] countsOrigin = e.Result as int[];
int[] counts = new int[countsOrigin.Length - 1];
for (int i = 0; i < counts.Length; i++)
{
counts[i] = countsOrigin[i + 1];
}
//获取最大值
double max = counts.Max();
//计算间距
double unit = this.Width / (3.0 * counts.Length + 1);
double marginTop = 100;
//计算直方图的矩形
Rectangle[] rects = new Rectangle[counts.Length];
rects[0].X = (int)unit;
rects[0].Y = 0;
rects[0].Width = (int)(2 * unit);
rects[0].Height = (int)((counts[0] / max) * (this.Height - marginTop));
for (int i = 1; i < counts.Length; ++i)
{
rects[i].X = (int)(rects[i - 1].X + 3 * unit);
rects[i].Y = 0;
rects[i].Width = (int)(2 * unit);
rects[i].Height = (int)((counts[i] / (max + 1)) * (this.Height - marginTop));
}
//绘图
graphics.FillRectangles(Brushes.Black, rects);
//释放资源
graphics.Dispose();
this.Text = "绘图结果,最高次数:" + counts.Max() + " 最低次数:" + counts.Min();
}
}
}快速排序类
using System;
using System.Diagnostics;
using Quick;
namespace _2._3._26
{
///
/// 快速排序类。
///
public class QuickSortInsertion : BaseSort
{
///
/// 切换到插入排序的阈值。
///
public int M { get; set; }
public int[] Counts;
///
/// 默认构造函数。
///
public QuickSortInsertion()
{
this.M = 8;
}
///
/// 用快速排序对数组 a 进行升序排序。
///
/// 需要排序的类型。
/// 需要排序的数组。
public override void Sort(T[] a)
{
this.Counts = new int[this.M + 1];
for (int i = 0; i < this.M + 1; i++)
{
this.Counts[i] = 0;
}
Shuffle(a);
Sort(a, 0, a.Length - 1);
Debug.Assert(IsSorted(a));
}
///
/// 用快速排序对数组 a 的 lo ~ hi 范围排序。
///
/// 需要排序的数组类型。
/// 需要排序的数组。
/// 排序范围的起始下标。
/// 排序范围的结束下标。
protected void Sort(T[] a, int lo, int hi) where T: IComparable
{
if (hi <= lo) // 别越界
return;
if (hi - lo <= this.M)
{
this.Counts[hi - lo]++;
// 调用插入排序
for (int i = lo; i <= hi; i++)
for (int k = i; k > lo && Less(a[k], a[k - 1]); k--)
Exch(a, k, k - 1);
return;
}
int j = Partition(a, lo, hi);
Sort(a, lo, j - 1);
Sort(a, j + 1, hi);
}
///
/// 对数组进行切分,返回枢轴位置。
///
/// 需要切分的数组类型。
/// 需要切分的数组。
/// 切分的起始点。
/// 切分的末尾点。
/// 枢轴下标。
private int Partition(T[] a, int lo, int hi) where T : IComparable
{
int i = lo, j = hi + 1;
T v = a[lo];
while (true)
{
while (Less(a[++i], v))
if (i == hi)
break;
while (Less(v, a[--j]))
if (j == lo)
break;
if (i >= j)
break;
Exch(a, i, j);
}
Exch(a, lo, j);
return j;
}
///
/// 打乱数组。
///
/// 需要打乱的数组类型。
/// 需要打乱的数组。
private void Shuffle(T[] a)
{
Random random = new Random();
for (int i = 0; i < a.Length; i++)
{
int r = i + random.Next(a.Length - i);
T temp = a[i];
a[i] = a[r];
a[r] = temp;
}
}
}
} 另请参阅
BackgroundWorker 组件 | Microsoft Docs
Quick 库
2.3.27
解答
实验结果如下: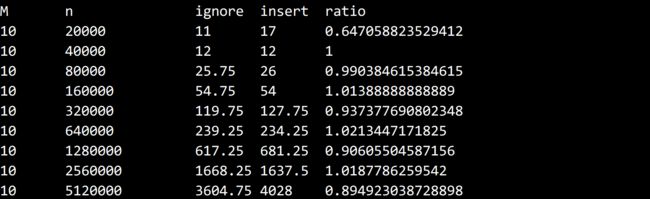
P.S. 测试机上的缓存是 L1 128K,L2 512K,L3 4MB。
代码
QuickSortIgnore
using System;
using System.Diagnostics;
using Quick;
namespace _2._3._27
{
///
/// 快速排序类。
///
public class QuickSortIgnore : BaseSort
{
///
/// 切换到插入排序的阈值。
///
public int M { get; set; }
///
/// 默认构造函数。
///
public QuickSortIgnore()
{
this.M = 10;
}
///
/// 用快速排序对数组 a 进行升序排序。
///
/// 需要排序的类型。
/// 需要排序的数组。
public override void Sort(T[] a)
{
Shuffle(a);
Sort(a, 0, a.Length - 1);
// 插入排序处理小数组
for (int i = 0; i < a.Length; i++)
for (int j = i; j > 0 && Less(a[j], a[j - 1]); j--)
Exch(a, j, j - 1);
Debug.Assert(IsSorted(a));
}
///
/// 用快速排序对数组 a 的 lo ~ hi 范围排序。
///
/// 需要排序的数组类型。
/// 需要排序的数组。
/// 排序范围的起始下标。
/// 排序范围的结束下标。
protected void Sort(T[] a, int lo, int hi) where T: IComparable
{
if (hi <= lo) // 别越界
return;
if (hi - lo <= this.M)
{
return; // 直接忽略
}
int j = Partition(a, lo, hi);
Sort(a, lo, j - 1);
Sort(a, j + 1, hi);
}
///
/// 对数组进行切分,返回枢轴位置。
///
/// 需要切分的数组类型。
/// 需要切分的数组。
/// 切分的起始点。
/// 切分的末尾点。
/// 枢轴下标。
private int Partition(T[] a, int lo, int hi) where T : IComparable
{
int i = lo, j = hi + 1;
T v = a[lo];
while (true)
{
while (Less(a[++i], v))
if (i == hi)
break;
while (Less(v, a[--j]))
if (j == lo)
break;
if (i >= j)
break;
Exch(a, i, j);
}
Exch(a, lo, j);
return j;
}
///
/// 打乱数组。
///
/// 需要打乱的数组类型。
/// 需要打乱的数组。
private void Shuffle(T[] a)
{
Random random = new Random();
for (int i = 0; i < a.Length; i++)
{
int r = i + random.Next(a.Length - i);
T temp = a[i];
a[i] = a[r];
a[r] = temp;
}
}
}
} QuickSortInsertion
using System;
using System.Diagnostics;
using Quick;
namespace _2._3._27
{
///
/// 快速排序类。
///
public class QuickSortInsertion : BaseSort
{
///
/// 切换到插入排序的阈值。
///
public int M { get; set; }
///
/// 默认构造函数。
///
public QuickSortInsertion()
{
this.M = 10;
}
///
/// 用快速排序对数组 a 进行升序排序。
///
/// 需要排序的类型。
/// 需要排序的数组。
public override void Sort(T[] a)
{
Shuffle(a);
Sort(a, 0, a.Length - 1);
Debug.Assert(IsSorted(a));
}
///
/// 用快速排序对数组 a 的 lo ~ hi 范围排序。
///
/// 需要排序的数组类型。
/// 需要排序的数组。
/// 排序范围的起始下标。
/// 排序范围的结束下标。
protected void Sort(T[] a, int lo, int hi) where T: IComparable
{
if (hi <= lo) // 别越界
return;
if (hi - lo <= this.M)
{
// 调用插入排序
for (int i = lo; i <= hi; i++)
for (int k = i; k > lo && Less(a[k], a[k - 1]); k--)
Exch(a, k, k - 1);
return;
}
int j = Partition(a, lo, hi);
Sort(a, lo, j - 1);
Sort(a, j + 1, hi);
}
///
/// 对数组进行切分,返回枢轴位置。
///
/// 需要切分的数组类型。
/// 需要切分的数组。
/// 切分的起始点。
/// 切分的末尾点。
/// 枢轴下标。
private int Partition(T[] a, int lo, int hi) where T : IComparable
{
int i = lo, j = hi + 1;
T v = a[lo];
while (true)
{
while (Less(a[++i], v))
if (i == hi)
break;
while (Less(v, a[--j]))
if (j == lo)
break;
if (i >= j)
break;
Exch(a, i, j);
}
Exch(a, lo, j);
return j;
}
///
/// 打乱数组。
///
/// 需要打乱的数组类型。
/// 需要打乱的数组。
private void Shuffle(T[] a)
{
Random random = new Random();
for (int i = 0; i < a.Length; i++)
{
int r = i + random.Next(a.Length - i);
T temp = a[i];
a[i] = a[r];
a[r] = temp;
}
}
}
}
测试用例
using System;
using Quick;
namespace _2._3._27
{
/*
* 2.3.27
*
* 忽略小数组。
* 用实验对比以下处理小数组的方法和练习 2.3.25 的处理方法的效果:
* 在快速排序中直接忽略小数组,仅在快速排序结束后运行一次插入排序。
* 注意:
* 可以通过这些实验估计出电脑的缓存大小,
* 因为当数组大小超出缓存时这种方法的性能可能会下降。
*
*/
class Program
{
static void Main(string[] args)
{
QuickSortInsertion insertion = new QuickSortInsertion();
QuickSortIgnore ignore = new QuickSortIgnore();
int arraySize = 20000; // 初始数组大小。
const int mSteps = 1; // M 值的递增次数。
const int trialTimes = 4; // 每次实验的重复次数。
const int trialLevel = 10; // 双倍递增的次数。
Console.WriteLine("M\tn\t\tignore\tinsert\tratio");
for (int i = 0; i < mSteps; i++)
{
int array = arraySize;
for (int j = 0; j < trialLevel; j++)
{
double timeIgnore = 0;
double timeInsertion = 0;
for (int k = 0; k < trialTimes; k++)
{
int[] a = SortCompare.GetRandomArrayInt(array);
int[] b = new int[a.Length];
a.CopyTo(b, 0);
timeInsertion += SortCompare.Time(insertion, b);
timeIgnore += SortCompare.Time(ignore, a);
}
timeIgnore /= trialTimes;
timeInsertion /= trialTimes;
if (arraySize < 10000000)
Console.WriteLine(ignore.M + "\t" + array + "\t\t" + timeIgnore + "\t" + timeInsertion + "\t" + timeIgnore / timeInsertion);
else
Console.WriteLine(ignore.M + "\t" + array + "\t" + timeIgnore + "\t" + timeInsertion + "\t" + timeIgnore / timeInsertion);
array *= 2;
}
ignore.M++;
}
}
}
}另请参阅
Quick 库
2.3.28
解答
对 Sort 方法做修改,添加一个层层传递的 depth 参数,每加一层 depth 就加一,结束时取左右较大的 depth 返回。
protected int Sort(T[] a, int lo, int hi, int depth) where T: IComparable
{
if (hi <= lo) // 别越界
return depth;
if (hi - lo <= this.M)
{
// 调用插入排序
for (int i = lo; i <= hi; i++)
for (int k = i; k > lo && Less(a[k], a[k - 1]); k--)
Exch(a, k, k - 1);
return depth;
}
int j = Partition(a, lo, hi);
int left = Sort(a, lo, j - 1, depth + 1);
int right = Sort(a, j + 1, hi, depth + 1);
return Less(left, right) ? right : left;
} 代码
using System;
using System.Diagnostics;
using Quick;
namespace _2._3._28
{
///
/// 快速排序类。
///
public class QuickSortInsertion : BaseSort
{
///
/// 切换到插入排序的阈值。
///
public int M { get; set; }
///
/// 上一次排序的最大递归深度。
///
public int Depth { get; private set; }
///
/// 默认构造函数。
///
public QuickSortInsertion()
{
this.M = 10;
}
///
/// 用快速排序对数组 a 进行升序排序。
///
/// 需要排序的类型。
/// 需要排序的数组。
/// 递归深度。
public override void Sort(T[] a)
{
Shuffle(a);
this.Depth = Sort(a, 0, a.Length - 1, 0);
Debug.Assert(IsSorted(a));
}
///
/// 用快速排序对数组 a 的 lo ~ hi 范围排序。
///
/// 需要排序的数组类型。
/// 需要排序的数组。
/// 排序范围的起始下标。
/// 排序范围的结束下标。
protected int Sort(T[] a, int lo, int hi, int depth) where T: IComparable
{
if (hi <= lo) // 别越界
return depth;
if (hi - lo <= this.M)
{
// 调用插入排序
for (int i = lo; i <= hi; i++)
for (int k = i; k > lo && Less(a[k], a[k - 1]); k--)
Exch(a, k, k - 1);
return depth;
}
int j = Partition(a, lo, hi);
int left = Sort(a, lo, j - 1, depth + 1);
int right = Sort(a, j + 1, hi, depth + 1);
return Less(left, right) ? right : left;
}
///
/// 对数组进行切分,返回枢轴位置。
///
/// 需要切分的数组类型。
/// 需要切分的数组。
/// 切分的起始点。
/// 切分的末尾点。
/// 枢轴下标。
private int Partition(T[] a, int lo, int hi) where T : IComparable
{
int i = lo, j = hi + 1;
T v = a[lo];
while (true)
{
while (Less(a[++i], v))
if (i == hi)
break;
while (Less(v, a[--j]))
if (j == lo)
break;
if (i >= j)
break;
Exch(a, i, j);
}
Exch(a, lo, j);
return j;
}
///
/// 打乱数组。
///
/// 需要打乱的数组类型。
/// 需要打乱的数组。
private void Shuffle(T[] a)
{
Random random = new Random();
for (int i = 0; i < a.Length; i++)
{
int r = i + random.Next(a.Length - i);
T temp = a[i];
a[i] = a[r];
a[r] = temp;
}
}
}
} 测试用例
using System;
using System.Collections.Generic;
using System.Linq;
using System.Text;
using System.Threading.Tasks;
namespace _2._3._28
{
/*
* 2.3.28
*
* 递归深度。
* 用经验性的研究估计切换阈值为 M 的快速排序
* 在将大小为 N 的不重复数组排序时的平均递归深度,
* 其中 M=10、20 和 50,N=10^3、10^4、10^5 和 10^6。
*
*/
class Program
{
static void Main(string[] args)
{
Console.WriteLine("M\tN\tDepth");
Trial(10);
Trial(20);
Trial(50);
}
///
/// 进行一次测试。
///
/// 要使用的阈值
static void Trial(int m)
{
QuickSortInsertion sort = new QuickSortInsertion();
int trialTime = 5;
// 由于排序前有 Shuffle,因此直接输入有序数组。
// M=10
sort.M = m;
int totalDepth = 0;
for (int N = 1000; N < 10000000; N *= 10)
{
for (int i = 0; i < trialTime; i++)
{
int[] a = new int[N];
for (int j = 0; j < N; j++)
{
a[j] = j;
}
sort.Sort(a);
totalDepth += sort.Depth;
}
Console.WriteLine(sort.M + "\t" + N + "\t" + totalDepth / trialTime);
}
}
}
}另请参阅
Quick 库
2.3.29
解答
在快排类内部添加一个随机数发生器,每次随机取枢轴并交换至第一位进行切分。
private int Partition(T[] a, int lo, int hi) where T : IComparable
{
int i = lo, j = hi + 1;
int pivot = this.RandomGenerator.Next(hi - lo) + lo;
Exch(a, pivot, lo);
T v = a[lo];
while (true)
{
while (Less(a[++i], v))
if (i == hi)
break;
while (Less(v, a[--j]))
if (j == lo)
break;
if (i >= j)
break;
Exch(a, i, j);
}
Exch(a, lo, j);
return j;
} 代码
使用随机枢轴的快排
using System;
using System.Diagnostics;
using Quick;
namespace _2._3._29
{
///
/// 快速排序类。
///
public class QuickSortRandomPivot : BaseSort
{
///
/// 切换到插入排序的阈值。
///
public int M { get; set; }
///
/// 随机数发生器。
///
private readonly Random RandomGenerator = new Random();
///
/// 默认构造函数。
///
public QuickSortRandomPivot()
{
this.M = 10;
}
///
/// 用快速排序对数组 a 进行升序排序。
///
/// 需要排序的类型。
/// 需要排序的数组。
public override void Sort(T[] a)
{
Sort(a, 0, a.Length - 1);
Debug.Assert(IsSorted(a));
}
///
/// 用快速排序对数组 a 的 lo ~ hi 范围排序。
///
/// 需要排序的数组类型。
/// 需要排序的数组。
/// 排序范围的起始下标。
/// 排序范围的结束下标。
protected void Sort(T[] a, int lo, int hi) where T: IComparable
{
if (hi <= lo) // 别越界
return;
if (hi - lo <= this.M)
{
// 调用插入排序
for (int i = lo; i <= hi; i++)
for (int k = i; k > lo && Less(a[k], a[k - 1]); k--)
Exch(a, k, k - 1);
return;
}
int j = Partition(a, lo, hi);
Sort(a, lo, j - 1);
Sort(a, j + 1, hi);
}
///
/// 对数组进行切分,返回枢轴位置。
///
/// 需要切分的数组类型。
/// 需要切分的数组。
/// 切分的起始点。
/// 切分的末尾点。
/// 枢轴下标。
private int Partition(T[] a, int lo, int hi) where T : IComparable
{
int i = lo, j = hi + 1;
int pivot = this.RandomGenerator.Next(hi - lo) + lo;
Exch(a, pivot, lo);
T v = a[lo];
while (true)
{
while (Less(a[++i], v))
if (i == hi)
break;
while (Less(v, a[--j]))
if (j == lo)
break;
if (i >= j)
break;
Exch(a, i, j);
}
Exch(a, lo, j);
return j;
}
}
} 测试用例
using System;
using Quick;
namespace _2._3._29
{
/*
* 2.3.29
*
* 随机化。
* 用经验性的研究对比随机选择切分元素和正文所述的一开始就将数组随机化这两种策略的效果。
* 在子数组大小为 M 时进行切换,将大小为 N 的不重复数组排序,
* 其中 M=10、20 和 50,N=10^3、10^4、10^5 和 10^6。
*
*/
class Program
{
static void Main(string[] args)
{
Console.WriteLine("M\tN\tshuffle\trandom\tshuffle/random");
Trial(10);
Trial(20);
Trial(50);
}
///
/// 进行一次测试。
///
/// 要使用的阈值
static void Trial(int m)
{
QuickSortInsertion withShuffle = new QuickSortInsertion();
QuickSortRandomPivot randomPivot = new QuickSortRandomPivot();
int trialTime = 5;
// M=10
withShuffle.M = m;
randomPivot.M = m;
double timeShuffle = 0;
double timeRandomPivot = 0;
for (int N = 1000; N < 10000000; N *= 10)
{
for (int i = 0; i < trialTime; i++)
{
int[] a = new int[N];
int[] b = new int[N];
for (int j = 0; j < N; j++)
{
a[j] = j;
}
Shuffle(a);
a.CopyTo(b, 0);
timeShuffle += SortCompare.Time(withShuffle, a);
timeRandomPivot += SortCompare.Time(randomPivot, b);
}
timeShuffle /= trialTime;
timeRandomPivot /= trialTime;
Console.WriteLine(withShuffle.M + "\t" + N + "\t" + timeShuffle + "\t" + timeRandomPivot + "\t" + timeShuffle / timeRandomPivot);
}
}
///
/// 打乱数组。
///
/// 需要打乱的数组类型。
/// 需要打乱的数组。
static void Shuffle(T[] a)
{
Random random = new Random();
for (int i = 0; i < a.Length; i++)
{
int r = i + random.Next(a.Length - i);
T temp = a[i];
a[i] = a[r];
a[r] = temp;
}
}
}
} 另请参阅
Quick 库
2.3.30
解答
结果如下,在 N=5000000 时,随机选择枢轴会比事先打乱快一点。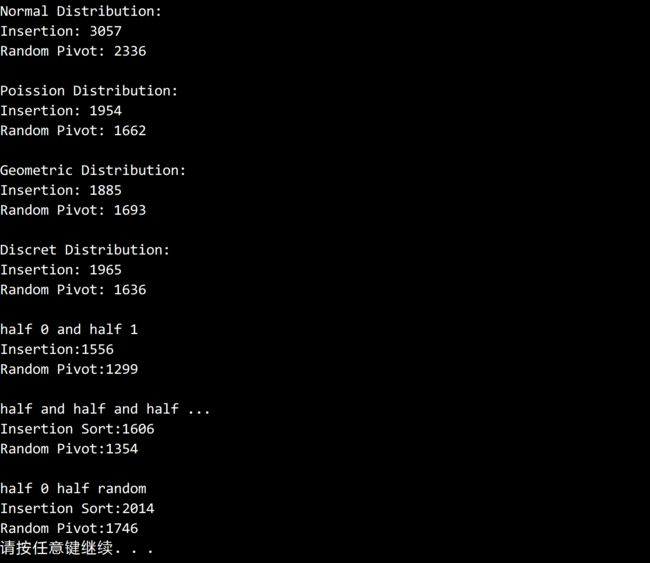
代码
using System;
using Quick;
namespace _2._3._30
{
/*
* 2.3.30
*
* 极端情况。
* 用初始随机化和非初始随机化的快速排序测试练习 2.1.35 和练习 2.1.36 中描述的大型非随机数组。
* 在将这些大数组排序时,乱序对快速排序的性能有何影响?
*
*/
class Program
{
static void Main(string[] args)
{
QuickSortInsertion insertionSort = new QuickSortInsertion();
QuickSortRandomPivot randomSort = new QuickSortRandomPivot();
int n = 5000000;
// 高斯分布(正态分布)
double[] arrayInsertion = SortCompare.GetNormalDistributionArray(n);
double[] arraySelection = new double[n];
arrayInsertion.CopyTo(arraySelection, 0);
Console.WriteLine("Normal Distribution:");
Console.WriteLine("Insertion: " + SortCompare.Time(insertionSort, arrayInsertion));
Console.WriteLine("Random Pivot: " + SortCompare.Time(randomSort, arraySelection));
Console.WriteLine();
// 泊松分布
arrayInsertion = SortCompare.GetPossionDistributionArray(n);
arrayInsertion.CopyTo(arraySelection, 0);
Console.WriteLine("Poission Distribution:");
Console.WriteLine("Insertion: " + SortCompare.Time(insertionSort, arrayInsertion));
Console.WriteLine("Random Pivot: " + SortCompare.Time(randomSort, arraySelection));
Console.WriteLine();
// 几何分布
arrayInsertion = SortCompare.GetGeometricDistributionArray(n, 0.3);
arrayInsertion.CopyTo(arraySelection, 0);
Console.WriteLine("Geometric Distribution:");
Console.WriteLine("Insertion: " + SortCompare.Time(insertionSort, arrayInsertion));
Console.WriteLine("Random Pivot: " + SortCompare.Time(randomSort, arraySelection));
Console.WriteLine();
// 离散分布
arrayInsertion = SortCompare.GetDiscretDistributionArray(n, new double[] { 0.1, 0.2, 0.3, 0.1, 0.1, 0.1, 0.1 });
arrayInsertion.CopyTo(arraySelection, 0);
Console.WriteLine("Discret Distribution:");
Console.WriteLine("Insertion: " + SortCompare.Time(insertionSort, arrayInsertion));
Console.WriteLine("Random Pivot: " + SortCompare.Time(randomSort, arraySelection));
Console.WriteLine();
// 一半是 0 一半是 1
int[] arrayNormalInsertion = HalfZeroHalfOne(n);
int[] arrayRandomPivot = new int[n];
arrayNormalInsertion.CopyTo(arrayRandomPivot, 0);
Console.WriteLine("half 0 and half 1");
Console.WriteLine("Insertion:" + SortCompare.Time(insertionSort, arrayNormalInsertion));
Console.WriteLine("Random Pivot:" + SortCompare.Time(randomSort, arrayRandomPivot));
Console.WriteLine();
// 一半是 0, 1/4 是 1, 1/8 是 2……
arrayNormalInsertion = HalfAndHalf(n);
arrayNormalInsertion.CopyTo(arrayRandomPivot, 0);
Console.WriteLine("half and half and half ...");
Console.WriteLine("Insertion Sort:" + SortCompare.Time(insertionSort, arrayNormalInsertion));
Console.WriteLine("Random Pivot:" + SortCompare.Time(randomSort, arrayRandomPivot));
Console.WriteLine();
// 一半是 0,一半是随机 int 值
arrayNormalInsertion = HalfZeroHalfRandom(n);
arrayNormalInsertion.CopyTo(arrayRandomPivot, 0);
Console.WriteLine("half 0 half random");
Console.WriteLine("Insertion Sort:" + SortCompare.Time(insertionSort, arrayNormalInsertion));
Console.WriteLine("Random Pivot:" + SortCompare.Time(randomSort, arrayRandomPivot));
}
///
/// 获取一半是 0 一半是 1 的随机
/// 数组大小。
/// 一半是 0 一半是 1 的
static int[] HalfZeroHalfOne(int n)
{
int[] result = new int[n];
Random random = new Random();
for (int i = 0; i < n; i++)
{
if (random.NextDouble() >= 0.5)
{
result[i] = 0;
}
else
{
result[i] = 1;
}
}
return result;
}
///
/// 生成 1/2 为 0, 1/4 为 1, 1/8 为 2 …… 的
/// 数组长度。
/// 1/2 为 0, 1/4 为 1, 1/8 为 2 …… 的
static int[] HalfAndHalf(int n)
{
int[] array = new int[n];
HalfIt(0, 0, n / 2, array);
Shuffle(array);
return array;
}
///
/// 递归生成 1/2 为 0, 1/4 为 1, 1/8 为 2 …… 的
/// 填充起点。
/// 起始编号。
/// 填充长度
/// 用于填充的数组。
/// 一个
static int[] HalfIt(int start, int number, int length, int[] array)
{
if (length == 0)
return array;
for (int i = 0; i < length; i++)
{
array[start + i] = number;
}
return HalfIt(start + length, number + 1, length / 2, array);
}
///
/// 生成一半是 0 一半是随机整数的
/// 数组大小。
/// 生成一半是 0 一半是随机整数的
static int[] HalfZeroHalfRandom(int n)
{
int[] array = new int[n];
Random random = new Random();
for (int i = 0; i < n / 2; i++)
{
array[i] = 0;
}
for (int i = n / 2; i < n; i++)
{
array[i] = random.Next();
}
Shuffle(array);
return array;
}
///
/// 打乱数组。
///
/// 需要打乱的数组。
static void Shuffle(int[] a)
{
int N = a.Length;
Random random = new Random();
for (int i = 0; i < N; i++)
{
int r = i + random.Next(N - i);// 等于StdRandom.uniform(N-i)
int temp = a[i];
a[i] = a[r];
a[r] = temp;
}
}
}
}另请参阅
Quick 库
2.3.31
解答
以下所有结果 T=70
N=1000
N=10000
N=100000
N=1000000
代码
using System;
using System.ComponentModel;
using System.Drawing;
using System.Linq;
using System.Windows.Forms;
using Quick;
namespace _2._3._31
{
public partial class Form2 : Form
{
private int N;
private int T;
public Form2(int n, int t)
{
InitializeComponent();
this.N = n;
this.T = t;
}
///
/// 启动页面时启动后台测试。
///
///
///
private void Form2_Shown(object sender, EventArgs e)
{
this.Text = "正在绘图";
this.backgroundWorker1.RunWorkerAsync();
}
///
/// 后台测试方法。
///
///
///
private void backgroundWorker1_DoWork(object sender, DoWorkEventArgs e)
{
BackgroundWorker worker = sender as BackgroundWorker;
QuickSort quick = new QuickSort();
double percentPerTrial = 100.0 / this.T;
double[] totalTime = new double[this.T];
for (int i = 0; i < this.T; i++)
{
double[] data = SortCompare.GetRandomArrayDouble(this.N);
totalTime[i] = SortCompare.Time(quick, data);
worker.ReportProgress((int)(percentPerTrial * i));
}
e.Result = totalTime;
}
///
/// 更新后台进度方法。
///
///
///
private void backgroundWorker1_ProgressChanged(object sender, ProgressChangedEventArgs e)
{
this.Text = "正在测试,已完成 " + e.ProgressPercentage + " %";
}
///
/// 测试完毕,进行绘图的方法。
///
///
///
private void backgroundWorker1_RunWorkerCompleted(object sender, RunWorkerCompletedEventArgs e)
{
if (e.Error != null)
{
MessageBox.Show(e.Error.Message);
}
//新建画布
Graphics graphics = this.CreateGraphics();
//翻转默认坐标系
graphics.TranslateTransform(0, this.Height);
graphics.ScaleTransform(1, -1);
double[] counts = e.Result as double[];
//获取最大值
double max = counts.Max();
//计算间距
double unit = this.Width / (3.0 * counts.Length + 1);
double marginTop = 100;
//计算直方图的矩形
Rectangle[] rects = new Rectangle[counts.Length];
rects[0].X = (int)unit;
rects[0].Y = 0;
rects[0].Width = (int)(2 * unit);
rects[0].Height = (int)((counts[0] / max) * (this.Height - marginTop));
for (int i = 1; i < counts.Length; ++i)
{
rects[i].X = (int)(rects[i - 1].X + 3 * unit);
rects[i].Y = 0;
rects[i].Width = (int)(2 * unit);
rects[i].Height = (int)((counts[i] / (max + 1)) * (this.Height - marginTop));
}
//绘图
graphics.FillRectangles(Brushes.Black, rects);
//释放资源
graphics.Dispose();
this.Text = "绘图结果,最高耗时:" + counts.Max() + " 最低耗时:" + counts.Min();
}
}
}另请参阅
BackgroundWorker 组件 | Microsoft Docs
Quick 库