2020 WHU校赛 J - Jogging along the Yangtze River（组合数学+卡特兰数）

题目链接

---

五月份的比赛七月份补题，接触卡特兰数就补吧！

但看向右上走和向右下走且不能越过$x$轴，这不就是卡特兰数经典问题的缩影吗：在$n\times n$的表格中不超过第一象限和$y=x$这条射线的路径方案数。

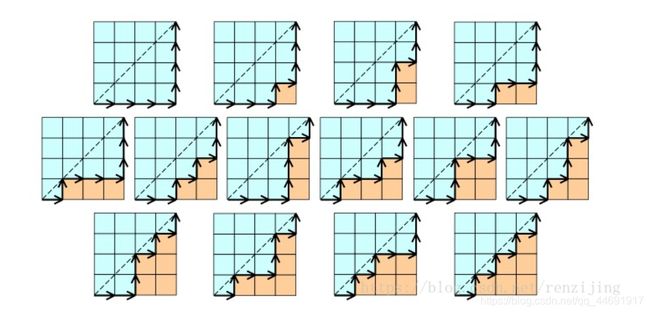
如上图斜着旋转，再将$y=x$这条线放在$x$轴上，就是卡特兰数。

但是我们还需要考虑直线向右走的情况，不难发现一次直线向右走就相当于一次右上和一次右下，因为二者的组合方式互相无影响，那么我们就考虑有多少种组合方式，此处需要用到乘法原理，加法原理等（紫书P318）

假设我们向右直线走了$i$步，走过的距离为$2\ast i$，那么右上和右下分别需要走$n-i$步。总的来说$2\ast n$的距离共需要走$2n-i$步。根据组合数学的知识以及乘法原理，二者组合的次数等于$\frac{总步数的阶乘}{分布阶乘的乘积}$

而右上和右下必须是成对出现的，均需要走$n-i$次，根据加法原理，应该是$((n-i)+(n-i))!=(2\ast (n-i))!$

然后右上和右下的走法满足卡特兰数，设第$i$个卡特兰数为$f(i)$，则最后的答案为${\sum }_{i=0}^{n}f(i)\ast \frac{(2\ast n-i)!}{i!\ast (2n-2i)!}$

然后就是线性求卡特兰数，求线性逆元，求阶乘逆元此类的板子活儿了

#include <set>
#include <map>
#include <stack>
#include <queue>
#include <math.h>
#include <cstdio>
#include <string>
#include <bitset>
#include <cstring>
#include <sstream>
#include <iostream>
#include <algorithm>
#include <unordered_map>
using namespace std;
#define fi first
#define se second
#define pb push_back
#define ins insert
#define lowbit(x) (x&(-x))
#define mkp(x,y) make_pair(x,y)
#define mem(a,x) memset(a,x,sizeof a);
typedef long long ll;
typedef long double ld;
typedef unsigned long long ull;
typedef pair<int,int> P;
const double eps=1e-8;
const double pi=acos(-1.0);
const int inf=0x3f3f3f3f;
const ll INF=1e18;
const int Mod=998244353;
const int maxn=2e5+100;

ll f[maxn],inv1[maxn],inv2[maxn];
ll fac[maxn];

ll quick_mod(ll x,ll n,ll p){
	ll ans=1;
    while(n){
        if(n&1) ans=ans*x%p;
        x=x*x%p;
        n>>=1;
    }
	return ans;
}

void solve(int n,ll p){
	fac[0]=1;

	for (int i=1;i<=n; i++) {
		fac[i]=fac[i-1]*i%p;
	}

	inv2[n]=quick_mod(fac[n],p-2,p);
	for (int i=n-1;i>=0;i--) {
		inv2[i]=inv2[i+1]*(i+1)%p;
	}
}

void katelan(int n,int p){  //p是取模的数
    f[2]=inv1[1]=1LL;

	for(int i=2;i<=n+2;i++)  //线性求i的逆元
    	inv1[i]=(p-p/i)*inv1[p%i]%p;

    for(int i=2;i<=n+2;i++)
        f[i+1]=(4*i-6)*f[i]%p*inv1[i]%p;
}


int main(){
    //freopen("in.txt","r",stdin);
    //freopen("out.txt","w",stdout);
    ios::sync_with_stdio(0),cin.tie(0),cout.tie(0);
    int n;
    solve(maxn-10,Mod);
    katelan(maxn-10,Mod);
    cin>>n;
    ll ans=0;
    for(int i=0;i<=n;i++){
        ans+=f[n-i+2]*fac[2*n-i]%Mod*inv2[i]%Mod*inv2[2*(n-i)]%Mod;
        ans%=Mod;
    }
    cout<<ans<<endl;
    return 0;
}