- 左神算法之有序二维矩阵中的目标值查找
岳轩子
左神算法算法矩阵线性代数
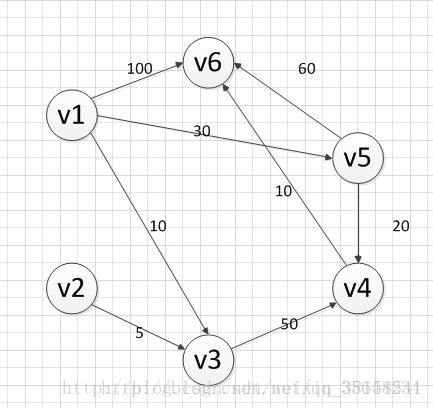
有序二维矩阵中的目标值查找目录有序二维矩阵中的目标值查找1.题目描述2.问题解释3.解决思路方法一:逐行二分查找(适合行数较少的情况)方法二:利用行列有序特性(最优解)4.代码实现5.总结1.题目描述给定一个元素为非负整数的二维数组matrix,其中:每一行按照从左到右递增的顺序排列每一列按照从上到下递增的顺序排列再给定一个非负整数aim,请判断aim是否存在于matrix中。示例:int[][]
- 技术开发全流程管理:涵盖天线系统的仿真建模(HFSS/CST等)、原型设计、调试优化(网络分析仪/暗室测试)到量产导入(LDS工艺识别),需主导技术文档编写(设计规范/测试报告)。
百态老人
网络设计规范
以下是针对天线系统技术开发全流程管理的完整解析,涵盖仿真建模、原型设计、调试优化、量产导入及技术文档编写五大环节,结合行业实践与资料核心信息进行系统阐述:一、仿真建模(HFSS/CST)1.软件选择与算法差异HFSS:基于有限元法(FEM),擅长电小尺寸、窄带天线设计(如微带天线、滤波电路),可精确计算辐射方向图、增益、S参数等。其自适应网格技术确保高精度,但计算资源消耗大,不适于电大尺寸模型。C
- 基于级联深度学习算法在双参数MRI中检测前列腺病变的评估| 文献速递-AI辅助的放射影像疾病诊断
有Li
人工智能深度学习算法
Title题目EvaluationofaCascadedDeepLearning–basedAlgorithmforProstateLesionDetectionatBiparametricMRI基于级联深度学习算法在双参数MRI中检测前列腺病变的评估Background背景MultiparametricMRI(mpMRI)improvesprostatecancer(PCa)detectionc
- 常见的强化学习算法分类及其特点
ywfwyht
人工智能算法分类人工智能
强化学习(ReinforcementLearning,RL)是一种机器学习方法,通过智能体(Agent)与环境(Environment)的交互来学习如何采取行动以最大化累积奖励。以下是一些常见的强化学习算法分类及其特点:1.基于值函数的算法这些算法通过估计状态或状态-动作对的价值来指导决策。Q-Learning无模型的离线学习算法。通过更新Q值表来学习最优策略。更新公式:Q(s,a)←Q(s,a)
- 【Python】PyRoboPath:Python机器人路径规划的终极指南
宅男很神经
python开发语言
PyRoboPath:Python机器人路径规划的终极指南第1部分:PyRoboPath与路径规划基础第1章:PyRoboPath概览与核心理念1.1什么是PyRoboPath?PyRoboPath是一个先进的、开源的Python库,致力于为学术研究人员、行业工程师以及机器人爱好者提供一套完整、高效、易用且可扩展的机器人路径规划解决方案。它不仅仅是一个算法的集合,更是一个集成了机器人建模、环境表示
- 最新 抖音 iOS 设备注册算法(配合心跳做不上榜人气用)
qq_1771238069
ios算法cocoa
最新业务需要研究了一周时间做出来了可以配合心跳包做抖音人气用一下部分代码#-*-encoding:utf-8-*-importjson,random,time,sysimportrequestsfromurllib.parseimporturlparse,parse_qsimportratelimitfromloguruimportloggerfromspiders.reg.confimportm
- Scikit-learn:机器学习的「万能工具箱」
科技林总
DeepSeek学AI人工智能
——三行代码构建AI模型的全栈指南**###**一、诞生背景:让机器学习从实验室走向大众****2010年前的AI困境**:-学术界模型难以工程化-算法实现碎片化(MATLAB/C++主导)-企业应用门槛极高>**破局者**:DavidCournapeau发起*Scikit-learn*项目,**统一算法接口**+**Python简易语法**=机器学习民主化革命---###**二、设计哲学:一致性
- Serverless架构下的持续交付实践
软件工程实践
软件工程最佳实践AI软件构建大数据系统架构serverless架构运维ai
Serverless架构下的持续交付实践关键词:Serverless架构、持续交付、DevOps、无服务器计算、自动化部署摘要:本文深入探讨了Serverless架构下的持续交付实践。首先介绍了Serverless架构和持续交付的背景知识,接着解释了相关核心概念及其关系,详细阐述了核心算法原理与操作步骤,通过数学模型加深理解,结合实际项目案例展示了代码实现与解读,探讨了实际应用场景,推荐了相关工具
- 海思Hi3519DV500方案1200万无人机吊舱套板
weixin_Todd_Wong2010
嵌入式硬件AI前端边缘计算图像处理
海思Hi3519DV500方案1200万无人机吊舱套板Hi3519DV500是一颗面向行业市场推出的超高清智能网络摄像头SoC。该芯片最高支持四路sensor输入,支持最高4K@30fps的ISP图像处理能力,支持2FWDR、多级降噪、六轴防抖、全景拼接、多光谱融合等多种传统图像增强和处理算法,支持通过AI算法对输入图像进行实时降躁等处理,为用户提供了卓越的图像处理能力,集成了高效的神经网络推理引
- 飞算 JavaAI 2.0.0和 AI 编程技术设计的 120 章 Java 系统教程
AI编程员
001AI传统&编程语言002AI编程工具汇总003AI编程作品汇总开发语言深度学习pillowAI编程人工智能
以下是基于飞算JavaAI2.0.0和AI编程技术设计的120章Java系统教程,涵盖从基础到高阶、理论到实践的全栈知识体系,结合经典案例与企业级项目实战,适合零基础到架构师的学习路径:第一部分:基础入门(第1-30章)Java开发环境配置JDK21+IntelliJIDEA+飞算AI插件安装第一个AI生成的HelloWorld程序基础语法与AI辅助编程数据类型、变量、运算符飞算AI:自动生成算法
- 算法大厨日记:猫猫狐狐带你用代码做一锅香喷喷的“预测汤”
Gyoku Mint
AI修炼日记猫猫狐狐的小世界人工智能人工智能机器学习python算法database深度学习数据挖掘
️【开场·今天的料理名叫“预测炖汤”】猫猫:“咱今天突发奇想,决定用机器学习代码给你炖一锅‘预测汤’喵!这不是教你代码,是要告诉你怎么把‘算法’吃进肚子里~”狐狐:“别急,她又在打比方了。这锅汤从数据准备到调参优化,就跟你平常做饭的过程没两样,只不过食材都被咱们用代码换了一遍。”【第一步·数据准备,就是挑菜啦】猫猫:“首先是挑菜(数据预处理),不能什么菜都扔进去锅里吧?要洗干净去皮(数据清洗),再
- Python实例题:基于 KNN 算法的手写数字识别
目录Python实例题题目要求:解题思路:代码实现:Python实例题题目基于KNN算法的手写数字识别要求:实现一个基于K-NearestNeighbors(KNN)算法的手写数字识别系统。支持以下功能:使用MNIST数据集训练和测试模型实现KNN分类算法可视化手写数字样本评估模型性能(准确率、混淆矩阵等)添加用户交互界面,允许用户绘制数字并进行识别。解题思路:使用sklearn加载MNIST数据
- Python实例题:基于遗传算法的旅行商问题求解
狐凄
实例python开发语言
目录Python实例题题目要求:解题思路:代码实现:Python实例题题目基于遗传算法的旅行商问题求解要求:使用遗传算法解决旅行商问题(TSP)。支持以下功能:随机生成城市坐标或导入预定义城市实现遗传算法的基本操作(选择、交叉、变异)可视化进化过程和最终路径统计进化过程中的适应度变化允许用户调整遗传算法参数(种群大小、迭代次数、交叉率、变异率等)。解题思路:用列表表示城市访问顺序作为染色体。使用欧
- 【算法笔记】红黑树插入操作
PXM的算法星球
算法笔记算法笔记
红黑树插入与调整详解一、红黑树的五大性质红黑树是一种自平衡的二叉搜索树(BST),其核心特性如下:颜色属性:每个节点非红即黑根属性:根节点必须为黑色叶子属性:所有的NIL叶子节点都是黑色红节点约束:红色节点的子节点必须为黑色(即无连续红节点)黑高平衡:从任一节点到其所有后代叶子节点的路径中,黑色节点数量相等二、插入操作流程阶段1:标准BST插入从根节点开始查找插入位置新节点总是红色按照BST规则插
- 什么是Sentinel? 以及优点
肘击鸣的百k路
sentinel
Sentinel是阿里巴巴开源的轻量级流量治理与系统保护组件,专注于微服务架构下的实时流量控制、熔断降级和系统稳定性保障。其核心目标是通过动态规则管理防止服务因高并发、突发流量或依赖故障导致雪崩崩溃。⚙️Sentinel的核心功能流量控制基于QPS(每秒请求数)或并发线程数限制资源访问,支持直接拒绝、匀速排队(漏桶算法)、慢启动(令牌桶算法)等策略。细粒度控制:可针对特定接口、方法甚至热点参数(如
- 用AI给AR加“智慧”:揭秘增强现实智能互动的优化秘密
Echo_Wish
人工智能前沿技术人工智能ar
用AI给AR加“智慧”:揭秘增强现实智能互动的优化秘密引子:增强现实,到底还能怎么更聪明?还记得当年PokémonGO火爆全球的场景吗?玩家们手机对准街头,虚拟小精灵活灵活现地跳出来,那就是增强现实(AR)最经典的应用之一。随着硬件发展和算法进步,AR正逐步从“炫酷玩具”变成生产力工具、教育助手、零售新体验。但AR想要更“聪明”,不是简单把虚拟物放到现实里那么简单,而是让虚拟世界和现实环境更自然地
- 推荐算法特征工程实战:用户与物料动态画像构建指南
Jay Kay
推荐算法推荐算法算法机器学习
在推荐系统的特征工程中,动态画像是提升推荐精准性的核心武器。通过捕捉用户行为偏好和物料热度变化,算法能实现千人千面的精准推荐。本文结合两张关键图表,深入解析动态画像的构建方法与工程实践。一、用户动态画像:六大维度精准刻画兴趣偏好用户动态画像基于六个关键维度构建(如表2-1所示),形成"6W"行为模型:用户粒度物料属性时间粒度动作类型统计对象统计方法1.核心维度解析(附典型场景)维度可选值应用场景用
- 非关系型数据库在数据库领域的崛起与应用
数据库管理艺术
数据库专家之路大数据AI人工智能MCP&AgentSQL实战数据库nosql网络ai
非关系型数据库在数据库领域的崛起与应用关键词:非关系型数据库、关系型数据库、崛起原因、应用场景、数据库领域摘要:本文主要探讨了非关系型数据库在数据库领域的崛起与应用。首先介绍了非关系型数据库的背景,包括目的、预期读者等内容。接着详细解释了非关系型数据库、关系型数据库等核心概念,并阐述了它们之间的关系。然后深入讲解了非关系型数据库的核心算法原理、数学模型和公式。通过项目实战展示了非关系型数据库的实际
- 搜索领域知识图谱的知识推理算法研究
搜索引擎技术
知识图谱算法人工智能ai
搜索领域知识图谱的知识推理算法研究关键词:知识图谱、知识推理、搜索算法、图神经网络、路径推理、规则推理、表示学习摘要:本文深入探讨搜索领域中知识图谱的知识推理算法。我们将从知识图谱的基本概念出发,分析不同类型的知识推理算法原理,包括基于规则的推理、基于表示的推理和基于路径的推理。通过实际案例和代码实现,展示这些算法如何提升搜索效果,最后讨论该领域的未来发展趋势和挑战。背景介绍目的和范围本文旨在系统
- 数据结构与算法中单调栈的常见误区
数据结构与算法学习
服务器运维ai
数据结构与算法中单调栈的常见误区关键词:单调栈、数据结构、算法、误区、栈、时间复杂度、应用场景摘要:单调栈是一种特殊的数据结构,它在解决某些特定问题时非常高效。然而,许多初学者在使用单调栈时容易陷入一些常见的误区。本文将详细介绍单调栈的概念、原理和应用,重点分析使用单调栈时的常见误区,并通过实际代码示例展示如何正确使用单调栈解决问题。背景介绍目的和范围本文旨在帮助读者深入理解单调栈的概念和工作原理
- AI优化算法实战:使用粒子群优化求解复杂工程问题
AI学长带你学AI
ai
AI优化算法实战:使用粒子群优化求解复杂工程问题关键词:粒子群优化(PSO)、全局优化、工程问题、智能算法、参数调优摘要:本文以“鸟群觅食”为灵感来源,深入浅出地讲解粒子群优化(ParticleSwarmOptimization,PSO)算法的核心原理,并通过机械结构轻量化设计的实战案例,展示其在复杂工程问题中的应用。文章从算法起源到数学模型,从代码实现到工程落地,层层拆解技术细节,帮助读者快速掌
- open3d 使用 RANSAC 算法拟合平面
扶子
python点云处理平面pythonopen3d经验分享点云拟合平面
1、功能介绍:一个python代码演示了如何使用open3d和numpy来完成一个完整的点云平面拟合任务。它包括以下几个主要部分:生成符合某一平面方程的随机点云数据、使用RANSAC算法对这些点云进行平面拟合、可视化原始点云和平面拟合结果2、代码部分:importnumpyasnpimportopen3daso3d#生成随机点云np.random.seed(42)n_points=100#假设这些
- 深度剖析AI人工智能在自动驾驶中的系统优化
AI云原生与云计算技术学院
人工智能自动驾驶机器学习ai
深度剖析AI人工智能在自动驾驶中的系统优化关键词:AI人工智能、自动驾驶、系统优化、传感器融合、决策算法摘要:本文深入探讨了AI人工智能在自动驾驶系统中的优化问题。从自动驾驶的背景入手,详细解释了相关核心概念,如传感器、决策算法等。阐述了这些核心概念之间的关系,介绍了核心算法原理和具体操作步骤,还通过数学模型和公式进行了理论支持。给出了项目实战案例,分析了实际应用场景,推荐了相关工具和资源,最后探
- CRC3校验算法
安庆平.Я
C/C++语言总结java前端服务器c语言unixlinux算法
C在线工具|菜鸟工具CRC3,16位数据校验使用,多项式g(x)=x3+x+1->0b1011#include#includeuint8_tCrc3(constuint32_tdata,uint8_tlen){uint8_tchk=0x08;uint8_tpoly=0x03;/*多顶式1011*/uint8_tpoly_len=4;uint8_talu=0x00;alu=(data>>len-po
- C++分发器
IT灰猫
c++开发语言
以调用某个算法为例,该算法有一个确定的函数Process,其参数不确定,返回值确定为bool类型,当然Process的返回值也可用模板进行替换,实现更灵活的返回值。#pragmaonce#include#include#include#include#include#includeclassAlgorithmDispatch{public:templatestd::shared_ptralgori
- day043-负载均衡算法与高可用keepalived
孙克旭
老男孩教育Linux运维99期负载均衡算法运维linux
文章目录0.老男孩思想-运维能为公司创造的价值1.负载均衡轮询算法1.1加权轮询1.2ip哈希1.3url哈希2.负载均衡模块指令补充3.高可用4.keepalived4.1部署keepalived服务4.2脑裂故障4.2.1脑裂故障常见原因4.2.2脑裂故障解决方法5.思维导图0.老男孩思想-运维能为公司创造的价值省钱:服务器设备、机房带宽、云主机云服务减少CDN流量优化、架构改造,当流量增加时
- 模拟工作队列 - 华为OD机试真题(JavaScript卷)
什码情况
算法面试javascript数据结构华为od
华为OD机试题库《C++》限时优惠9.9华为OD机试题库《Python》限时优惠9.9华为OD机试题库《JavaScript》限时优惠9.9针对刷题难,效率慢,我们提供一对一算法辅导,针对个人情况定制化的提高计划(全称1V1效率更高)。看不懂有疑问需要答疑辅导欢迎私VX:code5bug题目描述让我们来模拟一个工作队列的运作,有一个任务提交者和若干任务执行者,执行者从1开始编号。提交者会在给定的时
- 数据分类 - 华为OD机试真题(JavaScript 题解)
什码情况
华为odjavascript开发语言数据结构算法机试
华为OD机试题库《C++》限时优惠9.9华为OD机试题库《Python》限时优惠9.9华为OD机试题库《JavaScript》限时优惠9.9针对刷题难,效率慢,我们提供一对一算法辅导,针对个人情况定制化的提高计划(全称1V1效率更高)。看不懂有疑问需要答疑辅导欢迎私VX:code5bug题目描述对一个数据a进行分类,分类方法为:此数据a(四个字节大小)的四个字节相加对一个给定的值b取模,如果得到的
- GESP认证C++编程真题解析 | GESP202409 三级 单选题和判断题
热爱编程的通信人
历年GESPCSP-JCSP-S真题解析c++开发语言
欢迎大家订阅我的专栏:算法题解:C++与Python实现!本专栏旨在帮助大家从基础到进阶,逐步提升编程能力,助力信息学竞赛备战!专栏特色1.经典算法练习:根据信息学竞赛大纲,精心挑选经典算法题目,提供清晰的代码实现与详细指导,帮助您夯实算法基础。2.系统化学习路径:按照算法类别和难度分级,从基础到进阶,循序渐进,帮助您全面提升编程能力与算法思维。适合人群:准备参加蓝桥杯、GESP、CSP-J、CS
- LeetCode 学习day3 不喜勿喷
小小小新人12123
leetcode学习算法python
题目:给定一个数组prices,它的第i个元素prices[i]表示一支给定股票第i天的价格。你只能选择某一天买入这只股票,并选择在未来的某一个不同的日子卖出该股票。设计一个算法来计算你所能获取的最大利润。返回你可以从这笔交易中获取的最大利润。如果你不能获取任何利润,返回0。(LeetCode121.买卖股票的最佳时机)问题分析:简而言之为求最大差Python代码:importnumpyasnpc
- jsonp 常用util方法
hw1287789687
jsonpjsonp常用方法jsonp callback
jsonp 常用java方法
(1)以jsonp的形式返回:函数名(json字符串)
/***
* 用于jsonp调用
* @param map : 用于构造json数据
* @param callback : 回调的javascript方法名
* @param filters : <code>SimpleBeanPropertyFilter theFilt
- 多线程场景
alafqq
多线程
0
能不能简单描述一下你在java web开发中需要用到多线程编程的场景?0
对多线程有些了解,但是不太清楚具体的应用场景,能简单说一下你遇到的多线程编程的场景吗?
Java多线程
2012年11月23日 15:41 Young9007 Young9007
4
0 0 4
Comment添加评论关注(2)
3个答案 按时间排序 按投票排序
0
0
最典型的如:
1、
- Maven学习——修改Maven的本地仓库路径
Kai_Ge
maven
安装Maven后我们会在用户目录下发现.m2 文件夹。默认情况下,该文件夹下放置了Maven本地仓库.m2/repository。所有的Maven构件(artifact)都被存储到该仓库中,以方便重用。但是windows用户的操作系统都安装在C盘,把Maven仓库放到C盘是很危险的,为此我们需要修改Maven的本地仓库路径。
- placeholder的浏览器兼容
120153216
placeholder
【前言】
自从html5引入placeholder后,问题就来了,
不支持html5的浏览器也先有这样的效果,
各种兼容,之前考虑,今天测试人员逮住不放,
想了个解决办法,看样子还行,记录一下。
【原理】
不使用placeholder,而是模拟placeholder的效果,
大概就是用focus和focusout效果。
【代码】
<scrip
- debian_用iso文件创建本地apt源
2002wmj
Debian
1.将N个debian-506-amd64-DVD-N.iso存放于本地或其他媒介内,本例是放在本机/iso/目录下
2.创建N个挂载点目录
如下:
debian:~#mkdir –r /media/dvd1
debian:~#mkdir –r /media/dvd2
debian:~#mkdir –r /media/dvd3
….
debian:~#mkdir –r /media
- SQLSERVER耗时最长的SQL
357029540
SQL Server
对于DBA来说,经常要知道存储过程的某些信息:
1. 执行了多少次
2. 执行的执行计划如何
3. 执行的平均读写如何
4. 执行平均需要多少时间
列名 &
- com/genuitec/eclipse/j2eedt/core/J2EEProjectUtil
7454103
eclipse
今天eclipse突然报了com/genuitec/eclipse/j2eedt/core/J2EEProjectUtil 错误,并且工程文件打不开了,在网上找了一下资料,然后按照方法操作了一遍,好了,解决方法如下:
错误提示信息:
An error has occurred.See error log for more details.
Reason:
com/genuitec/
- 用正则删除文本中的html标签
adminjun
javahtml正则表达式去掉html标签
使用文本编辑器录入文章存入数据中的文本是HTML标签格式,由于业务需要对HTML标签进行去除只保留纯净的文本内容,于是乎Java实现自动过滤。
如下:
public static String Html2Text(String inputString) {
String htmlStr = inputString; // 含html标签的字符串
String textSt
- 嵌入式系统设计中常用总线和接口
aijuans
linux 基础
嵌入式系统设计中常用总线和接口
任何一个微处理器都要与一定数量的部件和外围设备连接,但如果将各部件和每一种外围设备都分别用一组线路与CPU直接连接,那么连线
- Java函数调用方式——按值传递
ayaoxinchao
java按值传递对象基础数据类型
Java使用按值传递的函数调用方式,这往往使我感到迷惑。因为在基础数据类型和对象的传递上,我就会纠结于到底是按值传递,还是按引用传递。其实经过学习,Java在任何地方,都一直发挥着按值传递的本色。
首先,让我们看一看基础数据类型是如何按值传递的。
public static void main(String[] args) {
int a = 2;
- ios音量线性下降
bewithme
ios音量
直接上代码吧
//second 几秒内下降为0
- (void)reduceVolume:(int)second {
KGVoicePlayer *player = [KGVoicePlayer defaultPlayer];
if (!_flag) {
_tempVolume = player.volume;
- 与其怨它不如爱它
bijian1013
选择理想职业规划
抱怨工作是年轻人的常态,但爱工作才是积极的心态,与其怨它不如爱它。
一般来说,在公司干了一两年后,不少年轻人容易产生怨言,除了具体的埋怨公司“扭门”,埋怨上司无能以外,也有许多人是因为根本不爱自已的那份工作,工作完全成了谋生的手段,跟自已的性格、专业、爱好都相差甚远。
- 一边时间不够用一边浪费时间
bingyingao
工作时间浪费
一方面感觉时间严重不够用,另一方面又在不停的浪费时间。
每一个周末,晚上熬夜看电影到凌晨一点,早上起不来一直睡到10点钟,10点钟起床,吃饭后玩手机到下午一点。
精神还是很差,下午像一直野鬼在城市里晃荡。
为何不尝试晚上10点钟就睡,早上7点就起,时间完全是一样的,把看电影的时间换到早上,精神好,气色好,一天好状态。
控制让自己周末早睡早起,你就成功了一半。
有多少个工作
- 【Scala八】Scala核心二:隐式转换
bit1129
scala
Implicits work like this: if you call a method on a Scala object, and the Scala compiler does not see a definition for that method in the class definition for that object, the compiler will try to con
- sudoku slover in Haskell (2)
bookjovi
haskellsudoku
继续精简haskell版的sudoku程序,稍微改了一下,这次用了8行,同时性能也提高了很多,对每个空格的所有解不是通过尝试算出来的,而是直接得出。
board = [0,3,4,1,7,0,5,0,0,
0,6,0,0,0,8,3,0,1,
7,0,0,3,0,0,0,0,6,
5,0,0,6,4,0,8,0,7,
- Java-Collections Framework学习与总结-HashSet和LinkedHashSet
BrokenDreams
linkedhashset
本篇总结一下两个常用的集合类HashSet和LinkedHashSet。
它们都实现了相同接口java.util.Set。Set表示一种元素无序且不可重复的集合;之前总结过的java.util.List表示一种元素可重复且有序
- 读《研磨设计模式》-代码笔记-备忘录模式-Memento
bylijinnan
java设计模式
声明: 本文只为方便我个人查阅和理解,详细的分析以及源代码请移步 原作者的博客http://chjavach.iteye.com/
import java.util.ArrayList;
import java.util.List;
/*
* 备忘录模式的功能是,在不破坏封装性的前提下,捕获一个对象的内部状态,并在对象之外保存这个状态,为以后的状态恢复作“备忘”
- 《RAW格式照片处理专业技法》笔记
cherishLC
PS
注意,这不是教程!仅记录楼主之前不太了解的
一、色彩(空间)管理
作者建议采用ProRGB(色域最广),但camera raw中设为ProRGB,而PS中则在ProRGB的基础上,将gamma值设为了1.8(更符合人眼)
注意:bridge、camera raw怎么设置显示、输出的颜色都是正确的(会读取文件内的颜色配置文件),但用PS输出jpg文件时,必须先用Edit->conv
- 使用 Git 下载 Spring 源码 编译 for Eclipse
crabdave
eclipse
使用 Git 下载 Spring 源码 编译 for Eclipse
1、安装gradle,下载 http://www.gradle.org/downloads
配置环境变量GRADLE_HOME,配置PATH %GRADLE_HOME%/bin,cmd,gradle -v
2、spring4 用jdk8 下载 https://jdk8.java.
- mysql连接拒绝问题
daizj
mysql登录权限
mysql中在其它机器连接mysql服务器时报错问题汇总
一、[running]
[email protected]:~$mysql -uroot -h 192.168.9.108 -p //带-p参数,在下一步进行密码输入
Enter password: //无字符串输入
ERROR 1045 (28000): Access
- Google Chrome 为何打压 H.264
dsjt
applehtml5chromeGoogle
Google 今天在 Chromium 官方博客宣布由于 H.264 编解码器并非开放标准,Chrome 将在几个月后正式停止对 H.264 视频解码的支持,全面采用开放的 WebM 和 Theora 格式。
Google 在博客上表示,自从 WebM 视频编解码器推出以后,在性能、厂商支持以及独立性方面已经取得了很大的进步,为了与 Chromium 现有支持的編解码器保持一致,Chrome
- yii 获取控制器名 和方法名
dcj3sjt126com
yiiframework
1. 获取控制器名
在控制器中获取控制器名: $name = $this->getId();
在视图中获取控制器名: $name = Yii::app()->controller->id;
2. 获取动作名
在控制器beforeAction()回调函数中获取动作名: $name =
- Android知识总结(二)
come_for_dream
android
明天要考试了,速速总结如下
1、Activity的启动模式
standard:每次调用Activity的时候都创建一个(可以有多个相同的实例,也允许多个相同Activity叠加。)
singleTop:可以有多个实例,但是不允许多个相同Activity叠加。即,如果Ac
- 高洛峰收徒第二期:寻找未来的“技术大牛” ——折腾一年,奖励20万元
gcq511120594
工作项目管理
高洛峰,兄弟连IT教育合伙人、猿代码创始人、PHP培训第一人、《细说PHP》作者、软件开发工程师、《IT峰播》主创人、PHP讲师的鼻祖!
首期现在的进程刚刚过半,徒弟们真的很棒,人品都没的说,团结互助,学习刻苦,工作认真积极,灵活上进。我几乎会把他们全部留下来,现在已有一多半安排了实际的工作,并取得了很好的成绩。等他们出徒之日,凭他们的能力一定能够拿到高薪,而且我还承诺过一个徒弟,当他拿到大学毕
- linux expect
heipark
expect
1. 创建、编辑文件go.sh
#!/usr/bin/expect
spawn sudo su admin
expect "*password*" { send "13456\r\n" }
interact
2. 设置权限
chmod u+x go.sh 3.
- Spring4.1新特性——静态资源处理增强
jinnianshilongnian
spring 4.1
目录
Spring4.1新特性——综述
Spring4.1新特性——Spring核心部分及其他
Spring4.1新特性——Spring缓存框架增强
Spring4.1新特性——异步调用和事件机制的异常处理
Spring4.1新特性——数据库集成测试脚本初始化
Spring4.1新特性——Spring MVC增强
Spring4.1新特性——页面自动化测试框架Spring MVC T
- idea ubuntuxia 乱码
liyonghui160com
1.首先需要在windows字体目录下或者其它地方找到simsun.ttf 这个 字体文件。
2.在ubuntu 下可以执行下面操作安装该字体:
sudo mkdir /usr/share/fonts/truetype/simsun
sudo cp simsun.ttf /usr/share/fonts/truetype/simsun
fc-cache -f -v
- 改良程序的11技巧
pda158
技巧
有很多理由都能说明为什么我们应该写出清晰、可读性好的程序。最重要的一点,程序你只写一次,但以后会无数次的阅读。当你第二天回头来看你的代码 时,你就要开始阅读它了。当你把代码拿给其他人看时,他必须阅读你的代码。因此,在编写时多花一点时间,你会在阅读它时节省大量的时间。
让我们看一些基本的编程技巧:
尽量保持方法简短
永远永远不要把同一个变量用于多个不同的
- 300个涵盖IT各方面的免费资源(下)——工作与学习篇
shoothao
创业免费资源学习课程远程工作
工作与生产效率:
A. 背景声音
Noisli:背景噪音与颜色生成器。
Noizio:环境声均衡器。
Defonic:世界上任何的声响都可混合成美丽的旋律。
Designers.mx:设计者为设计者所准备的播放列表。
Coffitivity:这里的声音就像咖啡馆里放的一样。
B. 避免注意力分散
Self Co
- 深入浅出RPC
uule
rpc
深入浅出RPC-浅出篇
深入浅出RPC-深入篇
RPC
Remote Procedure Call Protocol
远程过程调用协议
它是一种通过网络从远程计算机程序上请求服务,而不需要了解底层网络技术的协议。RPC协议假定某些传输协议的存在,如TCP或UDP,为通信程序之间携带信息数据。在OSI网络通信模型中,RPC跨越了传输层和应用层。RPC使得开发