算法 | 一周刷完《剑指Offer》 Day5：第50~60题

写在前面

• 本系列包含《剑指Offer》66道算法题，预计一周刷完，这是第五篇。
  系列汇总：剑指Offer 66题 Java 刷题笔记汇总
• 所有题目均可在牛客网在线编程平台进行调试。
  网址：https://www.nowcoder.com/ta/coding-interviews
• 本系列包含题目，解题思路及代码（Java）。
  代码同步发布在GitHub：https://github.com/JohnnyJYWu/offer-Java

上一篇：算法 | 一周刷完《剑指Offer》 Day4：第38~49题
下一篇：算法 | 一周刷完《剑指Offer》 Day6：第61~66题

---

Day5：第50~60题

难度都下来了。。。好像6天就能结束？

• T50. 二叉树的深度
• T51. 构建乘积数组
• T52. 正则表达式匹配
• T53. 表示数值的字符串
• T54. 字符流中第一个不重复的字符
• T55. 链表中环的入口结点
• T56. 删除链表中重复的结点
• T57. 二叉树的下一个结点
• T58. 对称的二叉树
• T59. 按之字形顺序打印二叉树
• T60. 把二叉树打印成多行

---

T50. 数组中重复的数字

题目描述

在一个长度为n的数组里的所有数字都在0到n-1的范围内。 数组中某些数字是重复的，但不知道有几个数字是重复的。也不知道每个数字重复几次。请找出数组中任意一个重复的数字。 例如，如果输入长度为7的数组{2,3,1,0,2,5,3}，那么对应的输出是第一个重复的数字2。

解题思路

交换思想。由于数字范围为0 ~ n-1，将数组的值进行交换使值与下标对应相等，即numbers[x] = x。这样若numbers[x]的值已经为x了，在下次又碰到要交换numbers[x]时即可判断是否出现重复数字。

	public boolean duplicate(int[] numbers, int length, int[] duplication) {//算法，交换思想
    	int index = 0;
    	
    	while(index < length) {
    		if(numbers[index] == index) {
    			index ++;
    		} else {
    			int num = numbers[index];
    			if(numbers[num] == num) {//存在重复
    				duplication[0] = num;
    				return true;
    			} else {//不重复则将num交换到numbers[num]上
    				int tmp = numbers[num];
    				numbers[num] = num;
    				numbers[index] = tmp;
    			}
    		}
    	}
    	
    	return false;
    }

排序，找重复。

	public boolean duplicate(int[] numbers, int length, int[] duplication) {//排序，找重复
    	if(numbers == null || numbers.length == 0) {
    		return false;
    	}
    	
    	Arrays.sort(numbers);
    	
    	for(int i = 0; i < length - 1; i ++) {
    		if(numbers[i + 1] == numbers[i]) {
				duplication[0] = numbers[i];
    			return true;
    		}
    	}
    	
    	return false;
    }

哈希表，找重复。

	public boolean duplicate(int[] numbers, int length, int[] duplication) {//哈希表，找重复
    	if(numbers == null || numbers.length == 0) {
    		return false;
    	}
    	
    	Set<Integer> set = new HashSet<>();
    	
    	for(int val: numbers) {
    		if(set.contains(val)) {
				duplication[0] = val;
    			return true;
    		} else {
    			set.add(val);
    		}
    	}
    	
    	return false;
    }

T51. 构建乘积数组

题目描述

给定一个数组A[0,1,…,n-1],请构建一个数组B[0,1,…,n-1],其中B中的元素B[i]=A[0] * A[1] * … * A[i-1] * A[i+1] * … * A[n-1]。不能使用除法。

解题思路

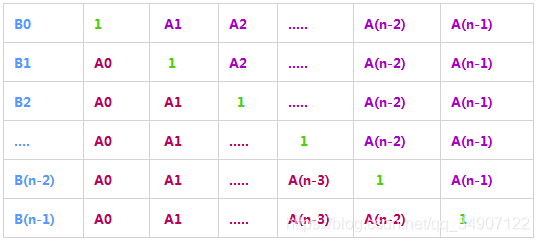
分三步走。如图，构建左下部分的前向乘积数组和右上部分的后向乘积数组。

step1：构建前向乘积数组C[i] = A[0] * A[1] * … * A[i-1]，即C[i] = C[i-1] * A[i-1]

step2：构建后向乘积数组D[i] = A[n-1] * A[n-2] * …A[n-i+1]，即D[i] = D[i+1] * A[i+1]

step3：B[i] = C[i] * D[i]

	public int[] multiply(int[] A) {//矩阵，详见图片
    	int len = A.length;
    	int[] B = new int[len];//乘积数组
    	int[] C = new int[len];
    	int[] D = new int[len];
    	
    	//构建前向乘积数组C[i]=A[0]*A[1]*...*A[i-1]，即C[i]=C[i-1]*A[i-1]
    	C[0] = 1;
    	for(int i = 1; i < len; i ++) {
    		C[i] = C[i - 1] * A[i - 1];
    	}
    	
    	//构建后向乘积数组D[i]=A[n-1]*A[n-2]*...A[n-i+1]，即D[i]=D[i+1]*A[i+1]
    	D[len - 1] = 1;
    	for(int i = len - 2; i >= 0; i --) {
    		D[i] = D[i + 1] * A[i + 1];
    	}
    	
    	//B[i]=C[i]*D[i]
    	for(int i = 0; i < len; i ++) {
    		B[i] = C[i] * D[i];
    	}
    	
    	return B;
    }

T52. 正则表达式匹配

题目描述

请实现一个函数用来匹配包括’.‘和’ * ‘的正则表达式。模式中的字符’.‘表示任意一个字符，而’ * '表示它前面的字符可以出现任意次（包含0次）。 在本题中，匹配是指字符串的所有字符匹配整个模式。例如，字符串"aaa"与模式"a.a"和"abaca"匹配，但是与"aa.a"和"ab*a"均不匹配

解题思路

这题没什么可说的，细心点，慢慢来，多调几遍bug。。。

	public boolean match(char[] str, char[] pattern) {
    	if(str == null || pattern == null) return false;
        if(str.length == 0 && pattern.length == 0) return true;//都空，匹配成功
        if(str.length != 0 && pattern.length == 0) return false;//第一个非空，第二个空，匹配失败（相反是有可能成功的，例如"a*a*"可匹配成""）
        if(str.length == 0){
            if((pattern.length == 2 && pattern[1] == '*')){//特殊情况
                return true;
            }else {
                return false;
            }
        }
        
        return matchCore(str, 0, pattern, 0);
    }
    
    private boolean matchCore(char[] str, int sIndex, char[] pattern, int pIndex) {
        if(sIndex == str.length && pIndex == pattern.length) return true;
        
        if(sIndex != str.length && pIndex == pattern.length) return false;
        
        if((pIndex + 1) < pattern.length && pattern[pIndex + 1] == '*') {
            if((sIndex < str.length && str[sIndex] == pattern[pIndex]) 
            		|| (pattern[pIndex] == '.' && sIndex < str.length)) {
            	
                return matchCore(str, sIndex + 1, pattern, pIndex + 2) 
                		|| matchCore(str, sIndex + 1, pattern, pIndex)
                		|| matchCore(str, sIndex, pattern, pIndex + 2);
            }else{
                return matchCore(str, sIndex, pattern, pIndex + 2);
            }
        }
        if((sIndex < str.length && str[sIndex] == pattern[pIndex])
        		|| (pattern[pIndex] == '.' && sIndex < str.length)) {
        	
            return matchCore(str, sIndex + 1, pattern, pIndex + 1);
        }else{
            return false;
        }
    }

T53. 表示数值的字符串

题目描述

请实现一个函数用来判断字符串是否表示数值（包括整数和小数）。例如，字符串"+100",“5e2”,"-123",“3.1416"和”-1E-16"都表示数值。 但是"12e",“1a3.14”,“1.2.3”,"±5"和"12e+4.3"都不是。

解题思路

又是一道考察细致的题。注意可能出现的多种情况。

	public boolean isNumeric(char[] str) {
		//+-符号，小数点，e是否出现过
        boolean hasSign = false, hasDecimal = false, hasE = false;
        
        for(int i = 0; i < str.length; i ++) {
            if(str[i] == 'e' || str[i] == 'E') {
            	//e后面一定要接数字
                if(i == str.length - 1) return false; 
                //不能同时存在两个e
                if(hasE) return false; 
                //出现e
                hasE = true;
            } else if(str[i] == '+' || str[i] == '-') {
                //第二次出现+-符号，则必须紧接在e之后
                if(hasSign && str[i - 1] != 'e' && str[i - 1] != 'E') return false;
                //第一次出现+-符号，且不是在字符串开头，则也必须紧接在e之后
                if(!hasSign && i > 0 && str[i - 1] != 'e' && str[i - 1] != 'E') return false;
                //出现+-符号
                hasSign = true;
            } else if(str[i] == '.') {
                //e后面不能接小数点，小数点不能出现两次
                if(hasE || hasDecimal) return false;
                //出现小数点
                hasDecimal = true;
            } else if(str[i] < '0' || str[i] > '9') // 不合法字符
                return false;
        }
        return true;
    }

或者，直接正则表达式匹配。（还是正则看着舒服）

	/**
     * 利用正则表达式
     * [\\+\\-]?             正或负符号出现与否
     * \\d*                  整数部分是否出现，如-.34 或 +3.34均符合
     * (\\.\\d+)?            如果出现小数点，那么小数点后面必须有数字；否则一起不出现
     * ([eE][\\+\\-]?\\d+)?  如果存在指数部分，那么e或E肯定出现，+或-可以不出现，紧接着必须跟着整数；或者整个部分都不出现
     */
    public boolean isNumeric(char[] str) {
        return new String(str).matches("[\\+\\-]?\\d*(\\.\\d+)?([eE][\\+\\-]?\\d+)?");
    }

T54. 字符流中第一个不重复的字符

题目描述

请实现一个函数用来找出字符流中第一个只出现一次的字符。例如，当从字符流中只读出前两个字符"go"时，第一个只出现一次的字符是"g"。当从该字符流中读出前六个字符“google"时，第一个只出现一次的字符是"l"。
（如果当前字符流没有存在出现一次的字符，返回#字符。）

解题思路

原理同T34，定义下标计数数组，插入字符时计数，同时记录字符串。输出时按照字符串中字符顺序找到第一个计数为1的即可。

	private String str = "";
	private int[] count = new int[256];//计数数组
    //Insert one char from stringstream
    public void Insert(char ch) {
        str += ch;
        count[ch] ++;
    }
	//return the first appearence once char in current stringstream
    public char FirstAppearingOnce() {
    	for(int i = 0; i < str.length(); i ++) {
    		if(count[str.charAt(i)] == 1) return str.charAt(i);
    	}
    	
    	return '#';
    }

T55. 链表中环的入口结点

题目描述

给一个链表，若其中包含环，请找出该链表的环的入口结点，否则，输出null。

解题思路

首先判断是否有环。定义两个指针，一个一次一步，一个一次两步，若能相遇则有环。

如果链表存在环，在相遇时，让一个指针在相遇点出发，另一个指针在链表首部出发。然后两个指针同步每次走一步，当它们相遇时，就是环的入口处。（原理画图列个公式推导一下）

	public ListNode EntryNodeOfLoop(ListNode pHead) {
    	if(pHead == null || pHead.next == null) return null;
    	
    	ListNode node1 = pHead;
    	ListNode node2 = pHead;
    	
    	while(node1 != null && node2 != null) {
    		node1 = node1.next;
    		node2 = node2.next.next;
    		
    		if(node1 == node2) break;
    	}
    	
    	if(node1 != null && node2 != null) {
        	node1 = pHead;
        	while(node1 != node2) {
            	node1 = node1.next;
            	node2 = node2.next;
        	}
        	return node1;
    	}
    	
    	return null;
    }

或者使用哈希表，重复的第一个结点即为环入口。

	public ListNode EntryNodeOfLoop(ListNode pHead) {
    	if(pHead == null || pHead.next == null) return null;
    	
    	ListNode node = pHead;
    	Set<ListNode> set = new HashSet<>();
    	
    	while(node != null) {
    		if(set.contains(node)) {
    			return node;
    		} else {
    			set.add(node);
    			node = node.next;
    		}
    	}
    	
    	return null;

T56. 删除链表中重复的结点

题目描述

在一个排序的链表中，存在重复的结点，请删除该链表中重复的结点，重复的结点不保留，返回链表头指针。 例如，链表1->2->3->3->4->4->5 处理后为 1->2->5

解题思路

需要定义一个指针preNode记录前一个不重复结点，之后改指针即可。

注意：注意头结点是否重复。

	public ListNode deleteDuplication(ListNode pHead) {
    	if(pHead == null) return null;
    	
    	ListNode preNode = null;
    	ListNode node = pHead;
    	
    	while(node != null) {
    		if(node.next != null && node.val == node.next.val) {
    			int val = node.val;
    			while(node.next != null && node.next.val == val) {
    				node = node.next;
    			}
    			
    			if(preNode == null) {
    				//能执行到此步说明此时头结点重复了
    				//改变pHead使其指向第一个不重复的结点
    				pHead = node.next;
    			} else {
    				//改指针，去掉中间重复的链
    				preNode.next = node.next;
    			}
    		} else {
    			preNode = node;
    		}
    		node = node.next;
    	}
    	
    	return pHead;
    }

T57. 二叉树的下一个结点

题目描述

给定一个二叉树和其中的一个结点，请找出中序遍历顺序的下一个结点并且返回。注意，树中的结点不仅包含左右子结点，同时包含指向父结点的指针。

解题思路

分情况讨论。中序遍历：左 -> 根 -> 右

情况一：该结点有右子树，直接进行中序遍历即可。

情况二：该结点无右子树，则需访问其父结点。此时又要分两种情况：

1. 若该结点是父结点的左子结点，继续访问父结点即可
2. 若是右子结点，则指针向上移动到其父结点，继续判断。

	public TreeLinkNode GetNext(TreeLinkNode pNode) {
        if(pNode == null) return null;
        
        if(pNode.right != null) {//该结点有右子树，进行中序遍历即可
        	pNode = pNode.right;
        	while(pNode.left != null) {
        		pNode = pNode.left;
        	}
        	return pNode;
        } else {//该节结点无右子树，则需返回
        	while(pNode.next != null) {
        		if(pNode.next.left == pNode) {//若该结点是父结点的左孩子
        			return pNode.next;
        		} else {//若是右孩子,则向上移动
        			pNode = pNode.next;
        		}
        	}
        }
        
        return null;
    }

T58. 对称的二叉树

题目描述

请实现一个函数，用来判断一颗二叉树是不是对称的。注意，如果一个二叉树同此二叉树的镜像是同样的，定义其为对称的。

解题思路

对左右子结点进行判断，同时由于需要镜像对称，则需将【左子结点的左子结点点和右子结点的右子结点】，【左子结点的右子节点和右子结点的左子结点】分别进行此操作进行判断，递归进行即可。

	public boolean isSymmetrical(TreeNode pRoot) {
        if(pRoot == null) return true;
        
        return isSymmetricalTree(pRoot.left, pRoot.right);
    }
	
	private boolean isSymmetricalTree(TreeNode left, TreeNode right) {
		if(left == null && right == null) return true;
		if(left == null) return false;
		if(right == null) return false;
		
		return left.val == right.val
				&& isSymmetricalTree(left.left, right.right)
				&& isSymmetricalTree(left.right, right.left);
	}

T59. 按之字形顺序打印二叉树

题目描述

请实现一个函数按照之字形打印二叉树，即第一行按照从左到右的顺序打印，第二层按照从右至左的顺序打印，第三行按照从左到右的顺序打印，其他行以此类推。

解题思路

二叉树层输出的变种。为了能够按之字形打印，则需考虑怎样能倒着输出。因此，使用两个栈来代替原来的两个队列进行广度优先搜索（BFS），即可实现。

	public ArrayList<ArrayList<Integer>> Print(TreeNode pRoot) {
		ArrayList<ArrayList<Integer>> result = new ArrayList<>();
		
		if(pRoot == null) return result;
		
		Stack<TreeNode> stack1 = new Stack<>();//存奇数行
		Stack<TreeNode> stack2 = new Stack<>();//存偶数行
		
		stack1.push(pRoot);
		
		while(!stack1.isEmpty() || !stack2.isEmpty()) {
			if(!stack1.isEmpty()) {
				ArrayList<Integer> list = new ArrayList<>();
				while(!stack1.isEmpty()) {
					TreeNode node = stack1.pop();
					list.add(node.val);
					
					if(node.left != null) stack2.push(node.left);
					if(node.right != null) stack2.push(node.right);
				}
				result.add(list);
			} else {
				ArrayList<Integer> list = new ArrayList<>();
				while(!stack2.isEmpty()) {
					TreeNode node = stack2.pop();
					list.add(node.val);

					if(node.right != null) stack1.push(node.right);
					if(node.left != null) stack1.push(node.left);
				}
				result.add(list);
			}
		}
		
		return result;
    }

T60. 把二叉树打印成多行

题目描述

从上到下按层打印二叉树，同一层结点从左至右输出。每一层输出一行。

解题思路

即二叉树层输出。使用两个队列进行广度优先搜索（BFS）。

	public ArrayList<ArrayList<Integer>> Print(TreeNode pRoot) {
		ArrayList<ArrayList<Integer>> result = new ArrayList<>();
		
		if(pRoot == null) return result;
		
		//使用队列实现，不断按层压入及输出
		Queue<TreeNode> queue1 = new LinkedList<>();
		Queue<TreeNode> queue2 = new LinkedList<>();
		
		queue1.add(pRoot);
		
		while(!queue1.isEmpty() || !queue2.isEmpty()) {
			if(!queue1.isEmpty()) {
				ArrayList<Integer> list = new ArrayList<>();
				while(!queue1.isEmpty()) {
					TreeNode node = queue1.poll();
					list.add(node.val);
					
					if(node.left != null) queue2.add(node.left);
					if(node.right != null) queue2.add(node.right);
				}
				result.add(list);
			} else {
				ArrayList<Integer> list = new ArrayList<>();
				while(!queue2.isEmpty()) {
					TreeNode node = queue2.poll();
					list.add(node.val);
					
					if(node.left != null) queue1.add(node.left);
					if(node.right != null) queue1.add(node.right);
				}
				result.add(list);
			}
		}
		
		return result;
    }

---

项目地址：https://github.com/JohnnyJYWu/offer-Java

上一篇：算法 | 一周刷完《剑指Offer》 Day4：第38~49题
下一篇：算法 | 一周刷完《剑指Offer》 Day6：第61~66题

希望这篇文章对你有帮助~