145. Binary Tree Postorder Traversal（二叉树的后序遍历）两种解法（C++ & 注释）

1. 题目描述

给定一个二叉树，返回它的 后序 遍历。

示例:

进阶: 递归算法很简单，你可以通过迭代算法完成吗？

题目链接：中文题目；英文题目

2. 递归（Recursion）

2.1 解题思路

我推荐大家把二叉树的三种遍历方式一起看了，对比理解，加深记忆（链接更新中，可先到个人主页查看）：

1. 二叉树三种遍历实现串讲（前中后序遍历） - 推荐；
2. Binary Tree Preorder Traversal（二叉树的前序遍历）；
3. Binary Tree Inorder Traversal（二叉树的中序遍历）；

递归方法非常的简单，首先我们先来看看后序遍历的顺序：

后序遍历和中序遍历比较相似，不同的是后序遍历走完左边，再走右边，最后才打印当前节点，所以整个递归访问顺序为：

postorderRecursion(root->left); // 往左走
postorderRecursion(root->right); // 往右走
ans.push_back(root->val); // 添加当前节点

2.2 实例代码

class Solution {
    vector<int> ans;

    void postorderRecursion(TreeNode* root) {
        if (!root) return;

        postorderRecursion(root->left);
        postorderRecursion(root->right);
        ans.push_back(root->val);
    }

public:
    vector<int> postorderTraversal(TreeNode* root) {
        postorderRecursion(root);
        return ans;
    }
};

3. 迭代（Iteration）

3.1 解题思路

使用列队（Queue）实现迭代的方法和中序遍历是一样的，只是添加节点的顺序稍微有点区别（和递归保持一致），这里就不再赘述了，大家可以参考这里哒：Binary Tree Inorder Traversal（二叉树的中序遍历）；

其实后序遍历的迭代使用stack会更加简单，这是因为后序遍历遵循“先进后得”的特点（先右后左添加节点），和stack“先进后出”的特点相匹配，使用stack的思路流程图如下，大家看一下就明白了啦，不难哒：

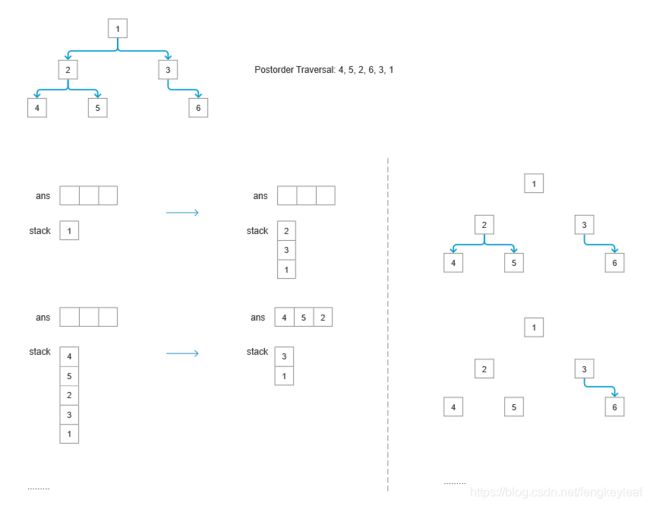
3.2 实例代码

class Solution {
    vector<int> ans;
    
    // 1. 使用queue的迭代
    void iterationUsingQueue(TreeNode* root) {
        queue<TreeNode*> q;
        q.push(root);

        while (q.size()) {
            TreeNode* temp = q.front();
            q.pop();
            int len = q.size();

            if (!temp->left && !temp->right) { ans.push_back(temp->val); continue; }

            // 后序遍历顺序重组
            if (temp->left) { q.push(temp->left); temp->left = nullptr; }
            if (temp->right) { q.push(temp->right); temp->right = nullptr; }
            q.push(temp);

            for (int i = 0; i < len; i++) { q.push(q.front()); q.pop(); }
        }

    }

    // 2. 使用stack的迭代
    void iterationUsingStack(TreeNode* root) {
        stack<TreeNode*> s;
        s.push(root);

        while (s.size()) {
            TreeNode* temp = s.top();
            
            if (!temp->left && !temp->right) { ans.push_back(temp->val); s.pop(); continue; }

            if (temp->right) { s.push(temp->right); temp->right = nullptr; }
            if (temp->left) { s.push(temp->left); temp->left = nullptr; }
        }
    }

public:
    vector<int> postorderTraversal(TreeNode* root) {
        if (!root) return ans;
        //iterationUsingQueue(root); 
        iterationUsingStack(root); 

        return ans;
    }
};