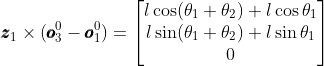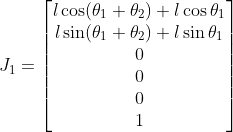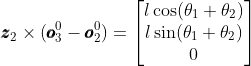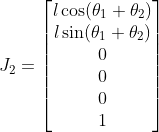- matlab卷积矩阵绝对值,MATLAB矩阵分析和计算
weixin_39928736
matlab卷积矩阵绝对值
MATLAB矩阵分析和计算编辑锁定讨论上传视频本词条缺少概述图,补充相关内容使词条更完整,还能快速升级,赶紧来编辑吧!《MATLAB矩阵分析和计算》是清华大学出版社出版的一本图书。[1]书名MATLAB矩阵分析和计算作者杜树春出版社清华大学出版社出版时间2019年6月1日定价59元ISBN9787302524816印次1-1印刷日期2019.04.23MATLAB矩阵分析和计算图书内容编辑本书侧重
- Matlab裁剪降水数据:1km掩膜制作实战
咋(za)说
matlab降水数据处理裁剪掩膜制作降水数据裁剪China_Pre
1km降水数据处理-制作数据裁剪掩膜1.数据概述2掩膜文件制作示例2.1数据准备2.2matlab掩膜制作示例代码3结语 中国1km分辨率逐月降水量数据集(1901-2024)是高精度、长时间序列的气候数据产品,广泛应用于水文、生态、农业等领域的研究。本篇基于应用需要,以该数据集为输入,结合研究区shp边界文件,制作用于数据提取/裁剪的掩膜文件。下面为具体内容。1.数据概述 中国1km分辨率逐
- MATLAB实现快速非局部均值图像去噪方法
一只爪子
本文还有配套的精品资源,点击获取简介:非局部均值滤波是一种先进的图像去噪技术,与传统方法相比,它利用图像的全局信息来去除噪声,同时保持图像细节。该算法通过搜索和利用整个图像中相似的像素块,对每个像素点进行去噪处理。本文提供的MATLAB代码FAST_NLM_II.m实现此算法,并包含必要的参数设置、相似性计算、加权平均和图像更新步骤。了解并应用此代码是学习和进一步改进非局部均值滤波技术的基础。1.
- matlab画信号图方法,献给初学者:手把手教你绘制信号通路图
信号通路是指能将细胞外的分子信号经细胞膜传入细胞内发挥效应的一系列酶促反应通路。细胞信号通路图是科研研究过程中最常见也是最常用到的,如何绘制适合我们自己科研课题的信号通路图呢?可以试试pathwaybuildertool软件。这款软件简单易学,即便是零基础的同学,也可以做出漂亮的信号通路。1.首先,打开PathwayBuilderTool2.0软件,软件自带分子生物学会用到的基本元素,如不同的细胞
- Octo:伯克利开源机器人开发框架
【摘要】在各种机器人数据集上预先训练的大型策略有可能改变机器人学习:这种通用机器人策略无需从头开始训练新策略,只需使用少量领域内数据即可进行微调,但具有广泛的泛化能力。然而,为了广泛应用于各种机器人学习场景、环境和任务,这些策略需要处理不同的传感器和动作空间,适应各种常用的机器人平台,并轻松高效地微调到新领域。在这项工作中,我们旨在为开发开源、广泛适用的通用机器人操作策略奠定基础。作为第一步,我们
- 【论文复现】Taylor算法用于TOA(到达时间)的三维标签位置解算,360个标签、12个基站的环境作为验证,附MATLAB例程
MATLAB卡尔曼
论文复现算法matlab开发语言
本文给出论文《基于Taylor-Chan算法的改进UWB室内三维定位方法》中的Taylor算法来解算TOA的复现程序(MATLAB)。使用论文中给定的12个锚点/360个测试的标签用来测试算法性能文章目录运行结果程序介绍核心功能概述结果输出应用场景MATLAB源代码运行结果误差输出:程序介绍本程序基于Taylor迭代算法,实现了对三维空间内360个目标点的TOA(TimeofArrival)定位解
- matlab计算转子系统的固有频率、振型、不平衡响应
可以计算转子系统的固有频率、振型、不平衡响应MatrixRiccati/code/Dichotomy_1(2).m,2210MatrixRiccati/code/Dichotomy_1.m,2210MatrixRiccati/code/RiccatiSY_1.m,2756MatrixRiccati/code/Trans1x(2).m,451MatrixRiccati/code/Trans1x.m,
- 基于MATLAB的语音信号预处理
3.1.语音信号的预加重处理对语音的的高频部分进行加重以去除口唇部分的影响,就必须要对输入的数字语音信号进行预加重处理,以此来增加语音的高频分辨率。通常通过传递函数为的一阶FIR高通数字滤波器来实现预加重,其中为预加重系数,0.9<<1.0。设n时刻的语音采样值为X(n),经过预加重处理的结果为,这里取=0.98。图3.1为该高通滤波器的幅频特性及相频特性。图3.2中分别给出了预加重前和预加重后的
- 【大数据】FP-growth算法
大雨淅淅
大数据算法人工智能大数据
目录一、FP-growth算法概述二、FP-growth算法代码实现2.1FP-growth算法matlab实现2.2FP-growth算法python实现三、FP-growth算法应用四、FP-growth算法发展趋势一、FP-growth算法概述FP-growth算法是一种用于发现数据集中频繁项集的高效算法。它由JiaweiHan等人提出,旨在解决Apriori算法在大数据集上效率低下的问题。
- MATLAB随机模拟技术在气候模型中的应用
本文还有配套的精品资源,点击获取简介:MATLAB是科学研究和工程领域中广泛使用的一款数学计算与编程软件,尤其在气象学和气候模拟方面有着重要的应用。’Fletcher_2019_Learning_Climate’项目通过MATLAB实现的随机模拟方法帮助理解气候变化。本文将详细探讨该项目的关键内容,包括气候模型的构成、随机过程与统计方法的运用、MATLAB编程技能、气候数据处理与分析、结果可视化以
- 数据可视化5:MATLAB绘制单组箱线图
箱线图的作用箱形图(又称为「盒须图」或「箱线图」)能方便显示数字数据组的四分位数。箱形图通常用于描述性统计,是以图形方式快速查看一个或多个数据集的好方法。虽然与直方图或密度图相比似乎有点原始,但它们占用较少空间,当要比较很多组或数据集之间的分布时便相当有用。箱线图基本描述该图展示的是一个箱线图(BoxPlot)的主要组成部分及其含义。箱线图是一种用于展示数据分布情况的统计图表,能够直观地反映数据的
- python 科研作图_Origin科研绘图
weixin_39525933
python科研作图
前言入了生物学的坑,狗狗们需要时不时的画一些图,看着别人高大上的图片,大家有没有好奇这些图片是怎么做出来的呢?就本狗狗来看(狗狗可能来自农村-_-,),现在铺天盖地的paper里的图,有些,当然本身就是照片啦,比如跑胶啊WB啊,有些是用R、python、或者matlab做的,那么对于不懂编程的狗狗来说,就需要利用一些趁手作图软件,也可以做出毫不逊色于前者的美图,常见的这类软件有origin,gra
- MATLAB 实现 SRCNN 图像超分辨率重建
leo__520
matlab超分辨率重建开发语言
SRCNN代码实现。该代码使用三层卷积神经网络,进行图像的超分辨率重建,效果比双三次插值好很多SRCNN/Readme.txt,1494SRCNN/SRCNN.m,1267SRCNN/Set14/baboon.bmp,720054SRCNN/Set14/barbara.bmp,1244214SRCNN/Set14/bridge.bmp,263222SRCNN/Set14/coastguard.bm
- Matlab实现特征选择算法中Relief-F算法
guygg88
大数据
特征选择算法中Relief-F算法使用Matlab的实现GetRandSamples.m,1719ReliefF.m,1034Untitled.m,1238data.txt,23637dataregress.m,210
- 基于matlab的二连杆机械臂PD控制的仿真
bubiyoushang888
matlab开发语言
基于matlab的二连杆机械臂PD控制的仿真。。。chap3_5input.m,1206d2plant1.m,1364hs_err_pid2808.log,15398hs_err_pid4008.log,15494lx_plot.m,885PD_Control.mdl,35066tiaojie.m,737chap2_1ctrl.asv,988chap2_1ctrl.m,905
- 2025——》import matplotlib.pyplot as plt/如何在Jupyter Notebook中使用Matplotlib库?
明—猿
NumPyjupytermatplotlibnumpyjupyter
importmatplotlib.pyplotasplt是Python中用于导入Matplotlib库的绘图模块pyplot并设置别名为plt的标准语句。Matplotlib是一个强大的可视化库,广泛用于数据可视化、图表绘制和科学绘图。以下是关于该语句的详细说明:一、语句解析importmatplotlib.pyplotmatplotlib是基础库,pyplot是其子模块,提供类似MATLAB的绘
- 用matlab实现随机森林算法
showmethetime
算法matlab随机森林
用matlab实现随机森林算法,里面附有说明文档,参数可调节RandomForest_matlab/RandomForests/RF.mexw32,81920RandomForest_matlab/RandomForests/RF_demo.m,2536RandomForest_matlab/RandomForests/runRF.m,2616RandomForest_matlab/RandomF
- 231转序和321转序的姿态角与四元数的变换关系(文末附VC++代码和Matlab验证代码)
小亨GNC颐园
matlabVC++运载火箭321转序231转序导弹导航初始化
近程战术导弹的转序一般采用231的顺序,先偏航、后俯仰、再滚转。远程导弹、运载火箭、某些垂直发射拦截导弹的初制导段会采用321的转序,先俯仰、后偏航、再滚转。这两种转下的姿态角与四元数的转换关系如下:321转序//--------惯性坐标系到箭体系的四元数--------------------//doublesic_T=sin(Theta_T_rad/2.0);余下的VC++代码和Matlab代
- matlab教程pdf,Matlab2010经典超强教程(清晰、版).pdf
malartinla
matlab教程pdf
第1章基础准备及入门本章有三个目的:一是讲述MATLAB正常运行所必须具备的基础条件;二是简明地介绍MATLAB及其操作桌面Desktop的基本使用方法;三是全面介绍MATLAB的帮助系统。本章的前两节讲述:MATLAB的正确安装方法和MATLAB环境的启动。因为指令窗是MATLAB最重要的操作界面,所以本章用第1.3、1.4两节以最简单通俗的叙述、算例讲述指令窗的基本操作方法和规则。这部分内容几
- matlab教程r2018a教材,MATLAB R2018a从入门到精通(升级版)
知外君
章MATLAB入门11.1MATLAB概述21.2MATLAB工作环境41.3MATLAB帮助61.4MATLAB操作实例91.5本章小结11第2章MATLAB界面122.1MATLAB搜索路径132.2MATLAB工作区142.3格式显示162.4本章小结17第3章MATLAB基本功能183.1命令行窗口193.2数据类型233.3初等函数运算31章MATLAB入门11.1MATLAB概述21.
- 【优秀文章】7月优秀文章推荐
优秀文章智能自主运动体与人工智能技术——环境感知、SLAM定位、路径规划、运动控制、多智能体协同作者:fpga和matlabC++之红黑树认识与实现作者:zzh_zao【手把手带你刷好题】–C语言基础编程题(十)作者:草莓熊Lotso飞算JavaAI:从“码农”到“代码指挥官”的终极进化论作者:可涵不会debug前端网页开发学习(HTML+CSS+JS)有这一篇就够!作者:一颗小谷粒
- 【NWFSP问题】基于中华穿山甲算法CPO求解零等待流水车间调度问题NWFSP研究(Matlab代码实现)
欢迎来到本博客❤️❤️博主优势:博客内容尽量做到思维缜密,逻辑清晰,为了方便读者。⛳️座右铭:行百里者,半于九十。本文目录如下:目录⛳️赠与读者1概述1.引言2.理论基础2.1中华穿山甲算法(CPO)核心原理2.2NWFSP数学模型3.CPO-NWFSP求解框架设计3.1编码与解码3.2离散化位置更新3.3目标函数适配4.实验设计与性能分析4.1实验设置4.2结果分析4.3敏感性分析5.结论与展望
- 计算三维空间中AOA定位的 CRLB(Cramér–Rao 下界,克拉美罗下界)公式与MATLAB例程
MATLAB卡尔曼
MATLAB定位程序与详解matlab机器学习定位导航
文章目录适用条件✅符号定义✅CRLB计算基本框架1.方向向量定义2.雅可比矩阵(Jacobian)3.Fisher信息矩阵(FIM)4.Cramér–RaoLowerBound✅例程中文注释版`aoa_crlb_3d_demo.m`✅运行输出结果在三维空间中,利用AOA(AngleofArrival,到达角度)测量信息进行目标定位时,CRLB(Cramér–RaoLowerBound)表示该测量系
- 基于条件风险价值CVaR的微网动态定价与调度策略(Matlab代码实现)
Ps.729
matlab开发语言
个人主页欢迎来到本博客❤️❤️博主优势:博客内容尽量做到思维缜密,逻辑清晰,为了方便读者。⛳️座右铭:行百里者,半于九十。本文目录如下:目录1概述一、CVaR的理论基础及其在微网中的适用性1.CVaR的定义与优势2.微网应用场景适配性二、动态定价与调度模型的联合优化框架1.目标函数设计2.动态定价机制3.不确定性处理方法三、关键算法与求解策略1.随机规划与CVaR集成2.智能优化算法对比四、实证
- 线性代数在图像处理中的应用 --- 纳尼? 2D的高斯核可以通过1D的高斯核直接生成?(秩为1的矩阵)
松下J27
LinearAlgebra线性代数图像处理人工智能
二维高斯核,Rank秩等于一的矩阵之前,我在学习图像处理的时候,会经常用到Gaussianblur,也就是二维高斯低通滤波。当时用的都是Matlab中,现成的图像处理库。只需要输入sigma和kernelsize这些参数就行了,完全不需要考虑高斯核中的每个点长啥样。虽然教科书里面也会有一些配图,例如:直到后来,我学习高斯图像金字塔的时候发现,在别人的代码里面,他在生成二维高斯核的时候,并不是直接写
- 【极光优化算法+分解对比】VMD-PLO-Transformer-LSTM多变量时间序列光伏功率预测Matlab代码
matlab科研助手
算法transformerlstm
✅作者简介:热爱数据处理、建模、算法设计的Matlab仿真开发者。更多Matlab代码及仿真咨询内容点击:Matlab科研工作室个人信条:格物致知。内容介绍光伏发电作为一种清洁能源,其功率预测对于电网稳定运行和电力系统调度至关重要。然而,光伏功率具有高度的非线性和波动性,传统的预测方法难以准确捕捉其动态特性。近年来,深度学习技术在时间序列预测领域取得了显著进展,为提高光伏功率预测精度提供了新的途径
- 用Python解锁图像处理之力:从基础到智能应用的深度探索
熊猫钓鱼>_>
python图像处理开发语言
在像素构成的数字世界里,Python已成为解码图像奥秘的核心引擎。一、为何选择Python处理图像?超越工具的本质思考当人们谈论图像处理时,往往会陷入工具对比的漩涡(PythonvsMATLABvsC++)。但Python的真正价值在于其构建的完整生态闭环:科学计算基石:NumPy的ndarray结构完美对应图像的多维矩阵本质算法实现自由:从传统算子到深度学习模型的无缝衔接可视化即战力:Matpl
- 【心电信号ECG去噪】小波变换(heursure规则阈值+Minimax规则阈值)心电信号去噪【含Matlab源码 3402期】
Matlab领域
matlab
Matlab领域博客之家
- 【信号去噪】基于NLM时间序列心电信号去噪附matlab代码
天天Matlab科研工作室
信号处理Matlab各类代码matlab开发语言fpga开发
1简介作为一种信号预处理手段,信号去噪在众多信号处理应用中发挥着重要的作用.到目前为止,信号去噪问题被大量研究,并取得了许多重要成果,涌现出了包括非局部均值(NLM)去噪算法在内的一批优秀的去噪方法.值得一提的是,相比于传统的局部去噪算法,非局部均值去噪算法有着更好的去噪性能和更好的信号细节保留能力.2部分代码function[denoisedSig,debug]=NLM_1dDarbon(sig
- 基于 Matlab 的小波变换方法对心电信号进行去噪
CodeWG
matlab数学建模开发语言
基于Matlab的小波变换方法对心电信号进行去噪心电信号是医学上常用的一种生物信号,可以反映人体心脏的电活动。然而,由于受到许多因素的干扰,如肌肉运动、电源杂波等,获取到的心电信号往往包含大量的噪声。因此,对心电信号进行准确的分析和诊断前,通常需要先对信号进行去噪处理。小波变换是一种常见的信号处理方法,在心电信号去噪方面也得到了广泛应用。它通过将信号分解成不同频率的子带,根据子带中的能量大小来进行
- 分享100个最新免费的高匿HTTP代理IP
mcj8089
代理IP代理服务器匿名代理免费代理IP最新代理IP
推荐两个代理IP网站:
1. 全网代理IP:http://proxy.goubanjia.com/
2. 敲代码免费IP:http://ip.qiaodm.com/
120.198.243.130:80,中国/广东省
58.251.78.71:8088,中国/广东省
183.207.228.22:83,中国/
- mysql高级特性之数据分区
annan211
java数据结构mongodb分区mysql
mysql高级特性
1 以存储引擎的角度分析,分区表和物理表没有区别。是按照一定的规则将数据分别存储的逻辑设计。器底层是由多个物理字表组成。
2 分区的原理
分区表由多个相关的底层表实现,这些底层表也是由句柄对象表示,所以我们可以直接访问各个分区。存储引擎管理分区的各个底层
表和管理普通表一样(所有底层表都必须使用相同的存储引擎),分区表的索引只是
- JS采用正则表达式简单获取URL地址栏参数
chiangfai
js地址栏参数获取
GetUrlParam:function GetUrlParam(param){
var reg = new RegExp("(^|&)"+ param +"=([^&]*)(&|$)");
var r = window.location.search.substr(1).match(reg);
if(r!=null
- 怎样将数据表拷贝到powerdesigner (本地数据库表)
Array_06
powerDesigner
==================================================
1、打开PowerDesigner12,在菜单中按照如下方式进行操作
file->Reverse Engineer->DataBase
点击后,弹出 New Physical Data Model 的对话框
2、在General选项卡中
Model name:模板名字,自
- logbackのhelloworld
飞翔的马甲
日志logback
一、概述
1.日志是啥?
当我是个逗比的时候我是这么理解的:log.debug()代替了system.out.print();
当我项目工作时,以为是一堆得.log文件。
这两天项目发布新版本,比较轻松,决定好好地研究下日志以及logback。
传送门1:日志的作用与方法:
http://www.infoq.com/cn/articles/why-and-how-log
上面的作
- 新浪微博爬虫模拟登陆
随意而生
新浪微博
转载自:http://hi.baidu.com/erliang20088/item/251db4b040b8ce58ba0e1235
近来由于毕设需要,重新修改了新浪微博爬虫废了不少劲,希望下边的总结能够帮助后来的同学们。
现行版的模拟登陆与以前相比,最大的改动在于cookie获取时候的模拟url的请求
- synchronized
香水浓
javathread
Java语言的关键字,可用来给对象和方法或者代码块加锁,当它锁定一个方法或者一个代码块的时候,同一时刻最多只有一个线程执行这段代码。当两个并发线程访问同一个对象object中的这个加锁同步代码块时,一个时间内只能有一个线程得到执行。另一个线程必须等待当前线程执行完这个代码块以后才能执行该代码块。然而,当一个线程访问object的一个加锁代码块时,另一个线程仍然
- maven 简单实用教程
AdyZhang
maven
1. Maven介绍 1.1. 简介 java编写的用于构建系统的自动化工具。目前版本是2.0.9,注意maven2和maven1有很大区别,阅读第三方文档时需要区分版本。 1.2. Maven资源 见官方网站;The 5 minute test,官方简易入门文档;Getting Started Tutorial,官方入门文档;Build Coo
- Android 通过 intent传值获得null
aijuans
android
我在通过intent 获得传递兑现过的时候报错,空指针,我是getMap方法进行传值,代码如下 1 2 3 4 5 6 7 8 9
public
void
getMap(View view){
Intent i =
- apache 做代理 报如下错误:The proxy server received an invalid response from an upstream
baalwolf
response
网站配置是apache+tomcat,tomcat没有报错,apache报错是:
The proxy server received an invalid response from an upstream server. The proxy server could not handle the request GET /. Reason: Error reading fr
- Tomcat6 内存和线程配置
BigBird2012
tomcat6
1、修改启动时内存参数、并指定JVM时区 (在windows server 2008 下时间少了8个小时)
在Tomcat上运行j2ee项目代码时,经常会出现内存溢出的情况,解决办法是在系统参数中增加系统参数:
window下, 在catalina.bat最前面
set JAVA_OPTS=-XX:PermSize=64M -XX:MaxPermSize=128m -Xms5
- Karam与TDD
bijian1013
KaramTDD
一.TDD
测试驱动开发(Test-Driven Development,TDD)是一种敏捷(AGILE)开发方法论,它把开发流程倒转了过来,在进行代码实现之前,首先保证编写测试用例,从而用测试来驱动开发(而不是把测试作为一项验证工具来使用)。
TDD的原则很简单:
a.只有当某个
- [Zookeeper学习笔记之七]Zookeeper源代码分析之Zookeeper.States
bit1129
zookeeper
public enum States {
CONNECTING, //Zookeeper服务器不可用,客户端处于尝试链接状态
ASSOCIATING, //???
CONNECTED, //链接建立,可以与Zookeeper服务器正常通信
CONNECTEDREADONLY, //处于只读状态的链接状态,只读模式可以在
- 【Scala十四】Scala核心八:闭包
bit1129
scala
Free variable A free variable of an expression is a variable that’s used inside the expression but not defined inside the expression. For instance, in the function literal expression (x: Int) => (x
- android发送json并解析返回json
ronin47
android
package com.http.test;
import org.apache.http.HttpResponse;
import org.apache.http.HttpStatus;
import org.apache.http.client.HttpClient;
import org.apache.http.client.methods.HttpGet;
import
- 一份IT实习生的总结
brotherlamp
PHPphp资料php教程php培训php视频
今天突然发现在不知不觉中自己已经实习了 3 个月了,现在可能不算是真正意义上的实习吧,因为现在自己才大三,在这边撸代码的同时还要考虑到学校的功课跟期末考试。让我震惊的是,我完全想不到在这 3 个月里我到底学到了什么,这是一件多么悲催的事情啊。同时我对我应该 get 到什么新技能也很迷茫。所以今晚还是总结下把,让自己在接下来的实习生活有更加明确的方向。最后感谢工作室给我们几个人这个机会让我们提前出来
- 据说是2012年10月人人网校招的一道笔试题-给出一个重物重量为X,另外提供的小砝码重量分别为1,3,9。。。3^N。 将重物放到天平左侧,问在两边如何添加砝码
bylijinnan
java
public class ScalesBalance {
/**
* 题目:
* 给出一个重物重量为X,另外提供的小砝码重量分别为1,3,9。。。3^N。 (假设N无限大,但一种重量的砝码只有一个)
* 将重物放到天平左侧,问在两边如何添加砝码使两边平衡
*
* 分析:
* 三进制
* 我们约定括号表示里面的数是三进制,例如 47=(1202
- dom4j最常用最简单的方法
chiangfai
dom4j
要使用dom4j读写XML文档,需要先下载dom4j包,dom4j官方网站在 http://www.dom4j.org/目前最新dom4j包下载地址:http://nchc.dl.sourceforge.net/sourceforge/dom4j/dom4j-1.6.1.zip
解开后有两个包,仅操作XML文档的话把dom4j-1.6.1.jar加入工程就可以了,如果需要使用XPath的话还需要
- 简单HBase笔记
chenchao051
hbase
一、Client-side write buffer 客户端缓存请求 描述:可以缓存客户端的请求,以此来减少RPC的次数,但是缓存只是被存在一个ArrayList中,所以多线程访问时不安全的。 可以使用getWriteBuffer()方法来取得客户端缓存中的数据。 默认关闭。 二、Scan的Caching 描述: next( )方法请求一行就要使用一次RPC,即使
- mysqldump导出时出现when doing LOCK TABLES
daizj
mysqlmysqdump导数据
执行 mysqldump -uxxx -pxxx -hxxx -Pxxxx database tablename > tablename.sql
导出表时,会报
mysqldump: Got error: 1044: Access denied for user 'xxx'@'xxx' to database 'xxx' when doing LOCK TABLES
解决
- CSS渲染原理
dcj3sjt126com
Web
从事Web前端开发的人都与CSS打交道很多,有的人也许不知道css是怎么去工作的,写出来的css浏览器是怎么样去解析的呢?当这个成为我们提高css水平的一个瓶颈时,是否应该多了解一下呢?
一、浏览器的发展与CSS
- 《阿甘正传》台词
dcj3sjt126com
Part Ⅰ:
《阿甘正传》Forrest Gump经典中英文对白
Forrest: Hello! My names Forrest. Forrest Gump. You wanna Chocolate? I could eat about a million and a half othese. My momma always said life was like a box ochocol
- Java处理JSON
dyy_gusi
json
Json在数据传输中很好用,原因是JSON 比 XML 更小、更快,更易解析。
在Java程序中,如何使用处理JSON,现在有很多工具可以处理,比较流行常用的是google的gson和alibaba的fastjson,具体使用如下:
1、读取json然后处理
class ReadJSON
{
public static void main(String[] args)
- win7下nginx和php的配置
geeksun
nginx
1. 安装包准备
nginx : 从nginx.org下载nginx-1.8.0.zip
php: 从php.net下载php-5.6.10-Win32-VC11-x64.zip, php是免安装文件。
RunHiddenConsole: 用于隐藏命令行窗口
2. 配置
# java用8080端口做应用服务器,nginx反向代理到这个端口即可
p
- 基于2.8版本redis配置文件中文解释
hongtoushizi
redis
转载自: http://wangwei007.blog.51cto.com/68019/1548167
在Redis中直接启动redis-server服务时, 采用的是默认的配置文件。采用redis-server xxx.conf 这样的方式可以按照指定的配置文件来运行Redis服务。下面是Redis2.8.9的配置文
- 第五章 常用Lua开发库3-模板渲染
jinnianshilongnian
nginxlua
动态web网页开发是Web开发中一个常见的场景,比如像京东商品详情页,其页面逻辑是非常复杂的,需要使用模板技术来实现。而Lua中也有许多模板引擎,如目前我在使用的lua-resty-template,可以渲染很复杂的页面,借助LuaJIT其性能也是可以接受的。
如果学习过JavaEE中的servlet和JSP的话,应该知道JSP模板最终会被翻译成Servlet来执行;而lua-r
- JZSearch大数据搜索引擎
颠覆者
JavaScript
系统简介:
大数据的特点有四个层面:第一,数据体量巨大。从TB级别,跃升到PB级别;第二,数据类型繁多。网络日志、视频、图片、地理位置信息等等。第三,价值密度低。以视频为例,连续不间断监控过程中,可能有用的数据仅仅有一两秒。第四,处理速度快。最后这一点也是和传统的数据挖掘技术有着本质的不同。业界将其归纳为4个“V”——Volume,Variety,Value,Velocity。大数据搜索引
- 10招让你成为杰出的Java程序员
pda158
java编程框架
如果你是一个热衷于技术的
Java 程序员, 那么下面的 10 个要点可以让你在众多 Java 开发人员中脱颖而出。
1. 拥有扎实的基础和深刻理解 OO 原则 对于 Java 程序员,深刻理解 Object Oriented Programming(面向对象编程)这一概念是必须的。没有 OOPS 的坚实基础,就领会不了像 Java 这些面向对象编程语言
- tomcat之oracle连接池配置
小网客
oracle
tomcat版本7.0
配置oracle连接池方式:
修改tomcat的server.xml配置文件:
<GlobalNamingResources>
<Resource name="utermdatasource" auth="Container"
type="javax.sql.DataSou
- Oracle 分页算法汇总
vipbooks
oraclesql算法.net
这是我找到的一些关于Oracle分页的算法,大家那里还有没有其他好的算法没?我们大家一起分享一下!
-- Oracle 分页算法一
select * from (
select page.*,rownum rn from (select * from help) page
-- 20 = (currentPag
![]() 的坐标表示为
的坐标表示为 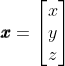 ,则有末端操作速度
,则有末端操作速度  。各关节速度组成矢量
。各关节速度组成矢量 ![]() ,有如下关系:
,有如下关系:![]()
。
。
![]() 求导,则得到操作速度和关节速度之间的关系。
求导,则得到操作速度和关节速度之间的关系。