【Java数据结构】集合PriorityQueue及其背后的数据结构堆（优先级队列）

作者：渴望力量的土狗

博客主页：渴望力量的土狗的博客主页

专栏：数据结构与算法

工欲善其事必先利其器，给大家介绍一款超牛的斩获大厂offer利器——牛客网

点击免费注册和我一起刷题吧

目录

优先级队列（PriorityQueue）

优先级队列的概念 

堆（Heap） 

堆的概念 

堆的性质 

堆的存储方式 

堆的创建：

调整方式： 

大根堆实现代码： 

小根堆实现代码：

建堆的时间复杂度 

堆的插入与删除

堆的插入 

 堆的删除

 用堆模拟实现优先级队列（完整代码）

 常用接口介绍

PriorityQueue常用接口介绍

---

优先级队列（PriorityQueue）

优先级队列的概念 

前面介绍过队列，队列是一种先进先出(FIFO)的数据结构，但有些情况下，操作的数据可能带有优先级，一般出队列时，可能需要优先级高的元素先出队列，该中场景下，使用队列显然不合适。在这种情况下，我们的数据结构应该提供两个最基本的操作，一个是返回最高优先级对象，一个是添加新的对象。这种数据结构就是优先级队列(Priority Queue)
JDK1.8中的PriorityQueue底层使用了堆的数据结构，而堆实际就是在完全二叉树的基础之上进行了一些元素的调整。

堆（Heap） 

堆的概念 

如果有一个关键码的集合K = {k0，k1， k2，…，kn-1}，把它的所有元素按完全二叉树的顺序存储方式存储 在一个一维数组中，并满足：Ki <= K2i+1 且 Ki<= K2i+2 (Ki >= K2i+1 且 Ki >= K2i+2) i = 0，1，2…，则称为 小堆(或大堆)。将根节点最大的堆叫做最大堆或大根堆，根节点最小的堆叫做最小堆或小根堆。  

堆的性质 

堆中某个节点的值总是不大于或不小于其父节点的值；
堆总是一棵完全二叉树。

堆的存储方式 

从堆的概念可知，堆是一棵完全二叉树，因此可以层序的规则采用顺序的方式来高效存储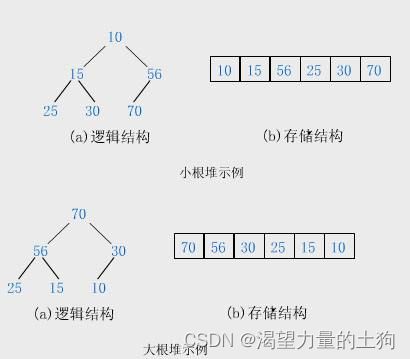

 注意：对于非完全二叉树，则不适合使用顺序方式进行存储，因为为了能够还原二叉树，空间中必须要存储空节点，就会导致空间利用率比较低

堆的创建：

调整方式： 

向下调整 是让调整的结点与其孩子节点进行比较
向上调整 是让调整的结点与其父亲结点进行比较
已知双亲的下标，则左孩子的下标为：left=2parent+1；
则右孩子的下标为：left=2parent+2；
已知孩子结点（不区分左右）的下标，则双亲的下标为：（child-1）/2

大根堆实现代码： 

public class TestHeap {

    public int[]elem;
    public int usedSize;

    public static final int DEFAULT_SIZE=10;

    public TestHeap() {
        elem=new int[DEFAULT_SIZE];
    }

    public void initElem(int[]arr){

        for(int i=0;i=0;parent--){
            //进行调整
            shiftDown(parent,usedSize);
        }

    }

    /**
     *
     * @param root 每颗子树的根
     * @param len   每颗子树结束的位置
     */

    public void shiftDown(int root,int len){
        int child=2*root+1;
        //保证有左孩子
        while (childelem[root]){
                int temp=elem[child];
                elem[child]=elem[root];
                elem[root]=temp;
                root=child;
                child=2*root+1;
            }else{
                break;
            }

        }


    }
}

小根堆实现代码：

public class TestHeap {

    public int[]elem;
    public int usedSize;

    public static final int DEFAULT_SIZE=10;

    public TestHeap() {
        elem=new int[DEFAULT_SIZE];
    }

    public void initElem(int[]arr){

        for(int i=0;i=0;parent--){
            //进行调整
            shiftDown(parent,usedSize);
        }

    }

    /**
     *
     * @param root 每颗子树的根
     * @param len   每颗子树结束的位置
     */

    public void shiftDown(int root,int len){
        int child=2*root+1;
        //保证有左孩子
        while (childelem[child+1]){
                //特别注意这个判断的条件（保证有右孩子才能执行下面的操作）

                child++;//右移
            }
            //现在child下标一定是左右孩子的最小值的下标

                //判断root下标的值和孩子的最大值
//            if(elem[child]>elem[root]){
//                int temp=elem[child];
//                elem[child]=elem[root];
//                elem[root]=temp;
//                root=child;
//                child=2*root+1;
//            }else{
//                break;
//            }

            //判断root下标的值和孩子的最小值
            if(elem[child]

建堆的时间复杂度 

因为堆是完全二叉树，而满二叉树也是完全二叉树，此处为了简化使用满二叉树来证明(时间复杂度本来看的就是近似值，多几个节点不影响最终结果)：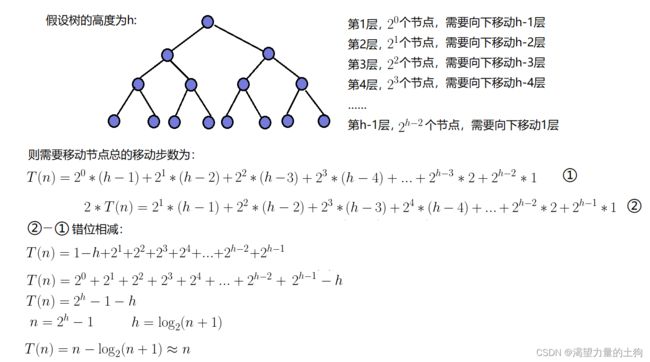
 因此：建堆的时间复杂度为O(N)

堆的插入与删除

堆的插入 

堆的插入总共需要两个步骤：
1. 先将元素放入到底层空间中(注意：空间不够时需要扩容)
2. 将最后新插入的节点向上调整，直到满足堆的性质

    /**
     * 入队：仍然要保持是大根堆
     * @param val
     */
    public void push(int val) {
        if(isFull()){
            elem= Arrays.copyOf(this.elem,2*this.elem.length);
        }
        elem[usedSize]=val;
        usedSize++;
        shiftUp(usedSize-1);

    }

    private void shiftUp(int child) {

        int parent=(child-1)/2;

        while(child>0)
        if(elem[child]>elem[parent]){

            int temp=elem[child];
            elem[child]=elem[parent];
            elem[parent]=temp;
            child=parent;
            parent=(child-1)/2;
        }else{
            break;
        }
    }

 堆的删除

注意：堆的删除一定删除的是堆顶元素。具体如下：
1. 将堆顶元素对堆中最后一个元素交换
2. 将堆中有效数据个数减少一个
3. 对堆顶元素进行向下调整

    public void pollHeap() {
        if(isEmpty()){
            throw new RuntimeException();
        }
        int temp=elem[0];
        elem[0]=elem[usedSize-1];
        elem[usedSize-1]=temp;
        usedSize--;
        //保证依然是大根堆
        shiftDown(0,usedSize);

    }

    public boolean isEmpty() {
        return usedSize==0;
    }

    /**
     *
     * @param root 是每棵子树的根节点的下标
     * @param len  是每棵子树调整结束的结束条件
     * 向下调整的时间复杂度：O(logn)
     */
    private void shiftDown(int root,int len) {

        int child=2*root+1;

        while(child

 用堆模拟实现优先级队列（完整代码）

import java.util.Arrays;

public class PriorityQueue {
    public int[] elem;
    public int usedSize;

    public static final int DEFAULT_INIT_USESZIE=10;
    public PriorityQueue() {
        elem=new int[DEFAULT_INIT_USESZIE];
    }

    /**
     * 建堆的时间复杂度：O(n)
     *
     * @param array
     */
    public void createHeap(int[] array) {



        for(int i=0;i=0;parent--){

            shiftDown(parent,usedSize);
        }

    }

    /**
     *
     * @param root 是每棵子树的根节点的下标
     * @param len  是每棵子树调整结束的结束条件
     * 向下调整的时间复杂度：O(logn)
     */
    private void shiftDown(int root,int len) {

        int child=2*root+1;

        while(child0)
        if(elem[child]>elem[parent]){

            int temp=elem[child];
            elem[child]=elem[parent];
            elem[parent]=temp;
            child=parent;
            parent=(child-1)/2;
        }else{
            break;
        }
    }

    public boolean isFull() {
        return usedSize== elem.length;
    }

    /**
     * 出队【删除】：每次删除的都是优先级高的元素
     * 仍然要保持是大根堆
     */
    public void pollHeap() {
        if(isEmpty()){
            throw new RuntimeException();
        }
        int temp=elem[0];
        elem[0]=elem[usedSize-1];
        elem[usedSize-1]=temp;
        usedSize--;
        //保证依然是大根堆
        shiftDown(0,usedSize);

    }

    public boolean isEmpty() {
        return usedSize==0;
    }

    /**
     * 获取堆顶元素
     * @return
     */
    public int peekHeap() {
        if(isEmpty()){
            return -1;
        }
        return elem[0];
    }
}

 常用接口介绍

Java集合框架中提供了PriorityQueue和PriorityBlockingQueue两种类型的优先级队列，PriorityQueue是线程不安全的，PriorityBlockingQueue是线程安全的，本文主要介绍PriorityQueue。 

关于PriorityQueue的使用要注意：
1. 使用时必须导入PriorityQueue所在的包，即：

import java.util.PriorityQueue;

2. PriorityQueue中放置的元素必须要能够比较大小，不能插入无法比较大小的对象，否则会抛出
ClassCastException异常
3. 不能插入null对象，否则会抛出NullPointerException
4. 没有容量限制，可以插入任意多个元素，其内部可以自动扩容
5. 插入和删除元素的时间复杂度为
6. PriorityQueue底层使用了堆数据结构, (注意：此处大家可以不用管什么是堆，后文中有介绍)
7. PriorityQueue默认情况下是小堆---即每次获取到的元素都是最小的元素

PriorityQueue常用接口介绍

构造器	功能介绍
PriorityQueue()	创建一个空的优先级队列，默认容量是11
PriorityQueue(int initialCapacity)	创建一个初始容量为initialCapacity的优先级队列，注意： initialCapacity不能小于1，否则会抛IllegalArgumentException异 常
PriorityQueue(Collection extends E> c)	用一个集合来创建优先级队列

注意：默认情况下，PriorityQueue队列是小堆，如果需要大堆需要用户提供比较器

// 用户自己定义的比较器：直接实现Comparator接口，然后重写该接口中的compare方法即可
class IntCmp implements Comparator{
@Override
public int compare(Integer o1, Integer o2) {
return o2-o1;
}
}
public class TestPriorityQueue {
public static void main(String[] args) {
PriorityQueue p = new PriorityQueue<>(new IntCmp());

插入/删除/获取优先级最高的元素

函数名	功能介绍
boolean offer(E e)	插入元素e，插入成功返回true，如果e对象为空，抛出NullPointerException异常，时 间复杂度 ，注意：空间不够时候会进行扩容
E peek()	获取优先级最高的元素，如果优先级队列为空，返回null
E poll()	移除优先级最高的元素并返回，如果优先级队列为空，返回null
int size()	获取有效元素的个数
void clear()	清空
boolean isEmpty()	检测优先级队列是否为空，空返回true