最短路径三大算法——2,迪杰斯特拉算法dijkstra (复杂度O（n^2))

目前已经更新：

1，弗洛伊德算法floyd(复杂度O（n^3))

2,迪杰斯特拉算法dijkstra (复杂度O（最坏n^2))

3，SPFA算法（复杂度O（n*m））

当然，最短路径有通用的bfs，dfs大神，但是我们这里特别介绍floyd，dijkstra还有spfa，本系列分为三篇

---

思想

dijkstra本质是贪心，即要求我走的每一步是局部最优就好，什么叫局部最优——我每次都去离我(起点）最近的点，然后更新其他点到起点的距离，当然，原来走过的点要标记一下，不能回去

为什么可以这么贪心呢：当我们每次选择dis[i]最小的点时，我们保证，因为其他点x离起点较远，即使通过i点可以缩小到起点的距离，那么仍然dis[x]>dis[i]+arr[i][x]>dis[i],即更新前后在所有未标记的点中dis[i]离起点更近成立，不会有后效性。

但是dijkstra也有缺点，无法处理负值点（需要用到spfa），但是对于全为正边，他无疑是相当优秀的

 

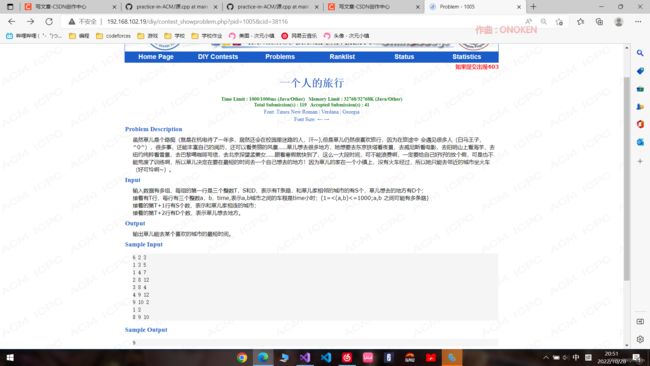
HDU一道例题

 多个起点，多个终点，但是不用害怕，聪明的你一定会联想到，我们可以设置一个超级起点（各个起点到他距离为0）和一个超级终点（各个终点到他距离为0），这样只需要跑一遍dijkstra

我们每次都求每个更新后的点中到达起点最近的点，一直到终点，这样我们最后得到的就是终点到起点最近

ACcode

#include 
using namespace std;
#define ll     long long
const int INF = 0x3f3f3f3f;
const int N = 1100;

struct city
{
	int cit, p;//cit表示指向的城市，p表示路程
};

vectorarr[N];

bool vis[N];//标记是否访问过
ll dis[N];//dis[x]表示x到超级起点距离
void dijkstra()
{
	memset(vis, 0, sizeof(vis));
	memset(dis, 0x3f, sizeof(dis));
	priority_queue < pair, vector< pair >, greater>>q;
	dis[0] = 0;
	q.push({0, 0});//第一个是距离（用于排序构建最小堆，第二个是城市
	while (!q.empty())
		{
			int tmp = q.top().second;//存入城市
			q.pop();
			if (vis[tmp])continue;
			vis[tmp] = 1;
			for (int i = 0; i < (int)arr[tmp].size(); ++i)//只对跟我邻接的边操作，其余操作也是无意义
				{
					if (dis[arr[tmp][i].cit] > dis[tmp] + arr[tmp][i].p)
						{
							dis[arr[tmp][i].cit] = dis[tmp] + arr[tmp][i].p;
							if (!vis[arr[tmp][i].cit])//避免重复标记
								q.push({dis[arr[tmp][i].cit], arr[tmp][i].cit});
						}
				}

		}
}

int main()
{
	int t, s, d;

	while (cin >> t >> s >> d)
		{
			int maxn = -1;
			int a, b, time;

			for (int i = 1; i <= t; ++i)
				{
					cin >> a >> b >> time;
					maxn = max(maxn, max(a, b));
					arr[a].push_back({b, time});
					arr[b].push_back({a, time});
				}
			for (int i = 1; i <= s; ++i)
				{
					cin >> a;
					arr[0].push_back({a, 0});
				}
			for (int i = 1; i <= d; ++i)
				{
					cin >> a;
					arr[a].push_back({maxn + 1, 0});
				}

			dijkstra();
			cout << dis[maxn + 1] << endl;
			for (int i = 0; i <= maxn + 1; ++i)arr[i].clear(); //多组数据不要忘记清空数组
		}

}

聪明的你可能会想，为什么我一定要跑最坏复杂度呢，对啊，所以明显可以用优先队列来优化（这样每次筛选最小也比上面方法快）

优先队列的使用可以参考优先队列priority_queue

#include 
using namespace std;
#define ll     long long
const int INF = 0x3f3f3f3f;
const int N = 1100;

struct city
{
	int cit, p;//cit表示指向的城市，p表示路程
};

vectorarr[N];

bool vis[N];//标记是否访问过
ll dis[N];//dis[x]表示x到超级起点距离
void dijkstra()
{
	memset(vis, 0, sizeof(vis));
	memset(dis, 0x3f, sizeof(dis));
	priority_queue < pair, vector< pair >, greater>>q;
	dis[0] = 0;
	q.push({dis[0], 0});//第一个是距离（用于排序构建最小堆，第二个是城市
	while (!q.empty())
		{
			int tmp = q.top().second;//存入城市
			q.pop();
			if (vis[tmp])continue;
			vis[tmp] = 1;//我们在进来是标记访问，而不是在更新点时标记访问，因为我们有可能在tmp点还未进入while时，被更新了几次，那么我们必须一直vis[tmp]=0才可以存入这些更新的值
			//因为优先队列保证同一个点，在多个更新的值中，肯定是离起点最近的最先访问，所以当他进来时，我们才标记访问，后续那些点进来，也会因为已经进来过一次直接跳过
			for (int i = 0; i < (int)arr[tmp].size(); ++i)//只对跟我邻接的边操作，其余操作也是无意义
				{
					if (dis[arr[tmp][i].cit] > dis[tmp] + arr[tmp][i].p)
						{
							dis[arr[tmp][i].cit] = dis[tmp] + arr[tmp][i].p;
							if (!vis[arr[tmp][i].cit])//避免重复标记
								q.push({dis[arr[tmp][i].cit], arr[tmp][i].cit});
						}
				}

		}
}

int main()
{
	int t, s, d;

	while (cin >> t >> s >> d)
		{
			int maxn = -1;
			int a, b, time;

			for (int i = 1; i <= t; ++i)
				{
					cin >> a >> b >> time;
					maxn = max(maxn, max(a, b));
					arr[a].push_back({b, time});
					arr[b].push_back({a, time});
				}
			for (int i = 1; i <= s; ++i)
				{
					cin >> a;
					arr[0].push_back({a, 0});
				}
			for (int i = 1; i <= d; ++i)
				{
					cin >> a;
					arr[a].push_back({maxn + 1, 0});
				}
			dijkstra();
			cout << dis[maxn + 1] << endl;
			for (int i = 0; i <= maxn + 1; ++i)arr[i].clear(); //多组数据不要忘记清空数组
		}

}