数据结构头歌实验梳理
数据结构头歌实验梳理
- 实验一 算法表示与实现基础
-
- 1 数据交换
- 2 最大最小值问题
- 3 ADT-Complex
- 数据结构与算法 - 线性表
-
- 1 实现一个顺序存储的线性表
- 2 实现一个链接存储的线性表
- 3 就地归并两个有序表
-
- 总结:
- 4 两个一元多项式异地相加
- 5 约瑟夫环问题
- 实验三 栈之基础
-
- 1 顺序存储的栈
- 2 实现一个链接存储的栈
- 实验三 栈之应用
-
- 第1关:利用栈实现整数的十进制转八进制
- 第2关:利用栈判断字符串括号是否匹配
- 第3关:利用栈判断字符串是否为回文串
- 队列之基础
-
- 第1关:实现一个顺序存储的队列
- 第2关:实现一个链接存储的队列
实验一 算法表示与实现基础
1 数据交换
#include 2 最大最小值问题
#include3 ADT-Complex
#include数据结构与算法 - 线性表
1 实现一个顺序存储的线性表
#include 2 实现一个链接存储的线性表
PS:该代码块表明了链接存储线性表所有常见相关用法及性质。对于初学者需要分成一小块一小块食用
特别说明:
- “当前位置”:当前位置由curr指针给出,当前位置的前一个位置由pre指针给出,当前位置的编号由position给出。后面将定义的若干操作与当前位置有关,例如:在当前位置结点之前插入结点,在当前位置结点之后插入结点,等等。当为空链表时,curr和pre都为空指针,position为0。当前位置在非空链表的最左端时,pre为空指针,curr指向头结点,position=0。当前位置在非空链表的最右端时,pre指向尾结点,curr为空指针,position等于len。
- 要好好理解一下下面几个指针
struct LinkNode {
T data;
LinkNode* next;
};
struct LinkList {
LinkNode* front; // 指向头结点。
LinkNode* rear; // 指向尾结点。
LinkNode* pre; // 指向当前位置结点的前一个结点。
LinkNode* curr; // 指向当前位置结点。
int position; // 当前位子结点的编号。
int len; // 线性表的大小(链表结点数)。
};
针对链表数据,我们定义如下操作:
- 创建空线性表:创建一个链接存储的线性表,初始为空表,返回llist指针。具体操作函数定义如下:
LinkList* LL_Create()
// 1)
LinkList* LL_Create()
// 创建一个链接存储的线性表,初始为空表,返回llist指针。
{
LinkList* llist=(LinkList*)malloc(sizeof(LinkList));
llist->front=NULL;
llist->rear=NULL;
llist->pre=NULL;
llist->curr=NULL;
llist->position=0;
llist->len=0;
return llist;
}
- 释放线性表结点:释放链表的结点,然后释放llist所指向的结构。具体操作函数定义如下:
void LL_Free(LinkList* llist)
// 2)
void LL_Free(LinkList* llist)
// 释放链表的结点,然后释放llist所指向的结构。
{
LinkNode* node=llist->front;
LinkNode* nextnode;
while(node){
nextnode=node->next;
free(node);
node=nextnode;
}
free(llist);
}
- 置空线性表:释放链表的所有结点,将当前线性表变为一个空表。具体操作函数定义如下:
void LL_MakeEmpty(LinkList* llist)
// 3)
void LL_MakeEmpty(LinkList* llist)
// 将当前线性表变为一个空表,因此需要释放所有结点。
{
LinkNode* node=llist->front;
LinkNode* nextnode;
while(node){
nextnode=node->next;
free(node);
node=nextnode;
}
llist->front=NULL;
llist->rear=NULL;
llist->pre=NULL;
llist->curr=NULL;
llist->position=0;
llist->len=0;
}
- 获取线性表当前长度: 返回线性表的当前长度。具体操作函数定义如下:
int LL_Length(LinkList* llist)
// 4)
int LL_Length(LinkList* llist)
// 返回线性表的当前长度。
{
return llist->len;
}
- 判断线性表是否为空: 若当前线性表是空表,则返回true,否则返回false。具体操作函数定义如下:
bool LL_IsEmpty(LinkList* llist)
// 5)
bool LL_IsEmpty(LinkList* llist)
// 若当前线性表是空表,则返回true,否则返回TRUE。
{
return llist->len==0;
}
- 设置线性表当前位置: 设置线性表的当前位置为i号位置。设置成功,则返回true,否则返回false(线性表为空,或i不在有效范围)。假设线性表当前长度为len,那么i的有效范围为[0,len]。具体操作函数定义如下:
bool LL_SetPosition(LinkList* llist, int i)
// 6)
bool LL_SetPosition(LinkList* llist, int i)
// 设置线性表的当前位置为i号位置。
// 设置成功,则返回true,否则返回false(线性表为空,或i不在有效的返回)。
// 假设线性表当前长度为len,那么i的有效范围为[0,len]。
{
int k;
/* 若链表为空,则返回*/
if (llist->len==0) return false;
/*若位置越界*/
if( i < 0 || i > llist->len)
{ printf("LL_SetPosition(): position error");
return false;
}
/* 寻找对应结点*/
llist->curr = llist->front;
llist->pre = NULL;
llist->position = 0;
for ( k = 0; k < i; k++) {
llist->position++;
llist->pre = llist->curr;
llist->curr = (llist->curr)->next;
}
/* 返回当前结点位置*/
return true;
}
- 获取线性表当前位置结点编号: 获取线性表的当前位置结点的编号。具体操作函数定义如下:
int LL_GetPosition(LinkList* llist)
// 7)
int LL_GetPosition(LinkList* llist)
// 获取线性表的当前位置结点的编号。
{
return llist->position;
}
- 设置线性表当前位置的下一位置为当前位置: 设置成功,则返回true,否则返回false(线性表为空,或当前位置为表尾)。具体操作函数定义如下:
bool LL_NextPosition(LinkList* llist)
// 8)
bool LL_NextPosition(LinkList* llist)
// 设置线性表的当前位置的下一个位置为当前位置。
// 设置成功,则返回true,否则返回false(线性表为空,或当前位置为表尾)。
{
if (llist->position >= 0 && llist->position < llist->len)
/* 若当前结点存在,则将其后继结点设置为当前结点*/
{
llist->position++;
llist->pre = llist->curr;
llist->curr = llist->curr->next;
return true;
}
else
return false;
}
- 获取线性表当前位置的数据元素的值: 具体操作函数定义如下:
T LL_GetAt(LinkList* llist)
// 9)
T LL_GetAt(LinkList* llist)
// 返回线性表的当前位置的数据元素的值。
{
if(llist->curr==NULL)
{
printf("LL_GetAt(): Empty list, or End of the List.\n");
LL_Free(llist);
exit(1);
}
return llist->curr->data;
}
- 修改线性表当前位置数据元素的值: 将线性表的当前位置的数据元素的值修改为x。具体操作函数定义如下:
void LL_SetAt(LinkList* llist, T x)
// 10)
void LL_SetAt(LinkList* llist, T x)
// 将线性表的当前位置的数据元素的值修改为x。
{
if(llist->curr==NULL)
{
printf("LL_SetAt(): Empty list, or End of the List.\n");
LL_Free(llist);
exit(1);
}
llist->curr->data=x;
}
- 在线性表当前位置之前插入数据元素: 在线性表的当前位置之前插入数据元素x。空表允许插入,当前位置指针将指向新结点。若插入失败,返回false,否则返回true。具体操作函数定义如下:
bool LL_InsAt(LinkList* llist, T x)
// 11)
bool LL_InsAt(LinkList* llist, T x)
// 在线性表的当前位置之前插入数据元素x。当前位置指针指向新数据元素结点。
// 若插入失败,返回false,否则返回true。
{
LinkNode *newNode=(LinkNode*)malloc(sizeof(LinkNode));
if (newNode==NULL) return false;
newNode->data=x;
if (llist->len==0){
/* 在空表中插入*/
newNode->next=NULL;
llist->front = llist->rear = newNode;
}
//当前位置为表头。
else if (llist->pre==NULL)
{
/* 在表头结点处插入*/
newNode->next = llist->front;
llist->front = newNode;
}
else {
/* 在链表的中间位置或表尾后的位置插入*/
newNode->next = llist->curr;
llist->pre->next=newNode;
}
//插入在表尾后。
if (llist->pre==llist->rear)
llist->rear=newNode;
/* 增加链表的大小*/
llist->len++;
/* 新插入的结点为当前结点*/
llist->curr = newNode;
return true;
}
- 在线性表当前位置之后插入数据元素: 在线性表的当前位置之后插入数据元素x。空表允许插入,当前位置指针将指向新结点。若插入失败,返回false,否则返回true。具体操作函数定义如下:
bool LL_InsAfter(LinkList* llist, T x)
// 12)
bool LL_InsAfter(LinkList* llist, T x)
// 在线性表的当前位置之后插入数据元素x。空表允许插入。当前位置指针将指向新结点。
// 若插入失败,返回false,否则返回true。
{
// 请在Begin-End之间补充代码,实现结点插入。
/********** Begin *********/
LinkNode *newNode=(LinkNode*)malloc(sizeof(LinkNode));
if (newNode==NULL) return false;
newNode->data=x;
if (llist->len==0){
newNode->next=NULL;
llist->front = llist->rear = newNode;
llist->curr = newNode;
}
else if (llist->curr==llist->rear)
{
newNode->next=NULL;
llist->rear->next=newNode;
llist->pre=llist->rear;
llist->rear=newNode;
llist->curr = newNode;
llist->position=llist->len;
}
else{
newNode->next=llist->curr->next;
llist->curr->next=newNode;
llist->pre=llist->curr;
llist->curr=newNode;
llist->position++;
}
llist->len++;
return true;
/********** End **********/
}
- 删除线性表当前位置数据元素结点: 若删除失败(为空表),则返回false,否则返回true。具体操作函数定义如下:
bool LL_DelAt(LinkList* llist)
// 13)
bool LL_DelAt(LinkList* llist)
// 删除线性表的当前位置的数据元素结点。
// 若删除失败(为空表,或当前位置为尾结点之后),则返回false,否则返回true。
{
LinkNode *oldNode;
/* 若表为空或已到表尾之后,则给出错误提示并返回*/
if (llist->curr==NULL)
{
printf("LL_DelAt(): delete a node that does not exist.\n");
return false;
}
oldNode=llist->curr;
/* 删除的是表头结点*/
if (llist->pre==NULL)
{
llist->front = oldNode->next;
}
/* 删除的是表中或表尾结点*/
else if(llist->curr!=NULL){
llist->pre->next = oldNode->next;
}
if (oldNode == llist->rear) {
/* 删除的是表尾结点,则修改表尾指针和当前结点位置值*/
llist->rear = llist->pre;
}
/* 后继结点作为新的当前结点*/
llist->curr = oldNode->next;
/* 释放原当前结点*/
free(oldNode);
/* 链表大小减*/
llist->len --;
return true;
}
- 删除线性表当前位置后面的那个数据元素结点: 若删除失败(为空表,或当前位置是表尾),则返回false,否则返回true。具体操作函数定义如下:
bool LL_DelAfter(LinkList* llist)
// 14)
bool LL_DelAfter(LinkList* llist)
// 删除线性表的当前位置的后面那个数据元素。
// 若删除失败(为空表,或当前位置时表尾),则返回false,否则返回true。
{
LinkNode *oldNode;
/* 若表为空或已到表尾,则给出错误提示并返回*/
if (llist->curr==NULL || llist->curr== llist->rear)
{
printf("LL_DelAfter(): delete a node that does not exist.\n");
return false;
}
/* 保存被删除结点的指针并从链表中删除该结点*/
oldNode = llist->curr->next;
llist->curr->next=oldNode->next;
if (oldNode == llist->rear)
/* 删除的是表尾结点*/
llist->rear = llist->curr;
/* 释放被删除结点*/
free(oldNode);
/* 链表大小减*/
llist->len --;
return true;
}
- 查找线性表中第一个值为x的数据元素的编号: 返回值-1表示没有找到,返回值>=0表示编号。具体操作函数定义如下:
int LL_FindValue(LinkList* llist, T x)
// 15)
int LL_FindValue(LinkList* llist, T x)
// 找到线性表中第一个值为x的数据元素的编号。
// 返回值-1表示没有找到,返回值>=0表示编号。
{
LinkNode* p=llist->front;
int idx=0;
while(p!=NULL && p->data!=x) {
idx++;
p = p->next;
}
if (idx>=llist->len) return -1;
else return idx;
}
- 删除线性表中第一个值为x的数据元素: 删除第一个值为x的数据元素,返回该数据元素的编号。如果不存在值为x的数据元素,则返回-1。具体操作函数定义如下:
int LL_DelValue(LinkList* llist, T x)
// 16)
int LL_DelValue(LinkList* llist, T x)
// 删除第一个值为x的数据元素,返回该数据元素的编号。如果不存在值为x的数据元素,则返回-1。
{
int idx=LL_FindValue(llist, x);
if (idx<0) return -1;
LL_SetPosition(llist, idx);
LL_DelAt(llist);
return idx;
}
- 打印整个线性表: 具体操作函数定义如下:
void LL_Print(LinkList* llist)
// 17)
void LL_Print(LinkList* llist)
// 打印整个线性表。
{
LinkNode* node=llist->front;
while (node) {
printf("%d ", node->data);
node=node->next;
}
printf("\n");
}
"LinkList.h"头文件是自己创建的
这个文件里包含:
- 创建头结点LNode
- 创建链表LinkList
- 函数声明
// 单链表 头文件
///
#if !defined(LINKED_LIST_H_LIELJE7398CNHD_INCLUDE_)
#define LINKED_LIST_H_LIELJE7398CNHD_INCLUDE_
typedef int T;
struct LinkNode {
T data;
LinkNode* next;
};
struct LinkList {
LinkNode* front; // 指向头结点。
LinkNode* rear; // 指向尾结点。
LinkNode* pre; // 指向当前位置结点的前一个结点。
LinkNode* curr; // 指向当前位置结点。
int position; // 当前位子结点的编号。
int len; // 线性表的大小(链表结点数)。
};
// 1)
LinkList* LL_Create();
// 创建一个链接存储的线性表,初始为空表,返回llist指针。
// 2)
void LL_Free(LinkList* llist);
// 释放链表的结点,然后释放llist所指向的结构。
// 3)
void LL_MakeEmpty(LinkList* llist);
// 将当前线性表变为一个空表,因此需要释放所有结点。
// 4)
int LL_Length(LinkList* llist);
// 返回线性表的当前长度。
// 5)
bool LL_IsEmpty(LinkList* llist);
// 若当前线性表是空表,则返回true,否则返回TRUE。
// 6)
bool LL_SetPosition(LinkList* llist, int i);
// 设置线性表的当前位置为i号位置。
// 设置成功,则返回true,否则返回false(线性表为空,或i不在有效的返回)。
// 假设线性表当前长度为len,那么i的有效范围为[0,len]。
// 7)
int LL_GetPosition(LinkList* llist);
// 获取线性表的当前位置结点的编号。
// 8)
bool LL_NextPosition(LinkList* llist);
// 设置线性表的当前位置的下一个位置为当前位置。
// 设置成功,则返回true,否则返回false(线性表为空,或当前位置为表尾)。
// 9)
T LL_GetAt(LinkList* llist);
// 返回线性表的当前位置的数据元素的值。
// 10)
void LL_SetAt(LinkList* llist, T x);
// 将线性表的当前位置的数据元素的值修改为x。
// 11)
bool LL_InsAt(LinkList* llist, T x);
// 在线性表的当前位置之前插入数据元素x。空表允许插入。当前位置指针将指向新结点。
// 若插入失败,返回false,否则返回true。
// 12)
bool LL_InsAfter(LinkList* llist, T x);
// 在线性表的当前位置之后插入数据元素x。空表允许插入。当前位置指针将指向新结点。
// 若插入失败,返回false,否则返回true。
// 13)
bool LL_DelAt(LinkList* llist);
// 删除线性表的当前位置的数据元素结点。
// 若删除失败(为空表),则返回false,否则返回true。
// 14)
bool LL_DelAfter(LinkList* llist);
// 删除线性表的当前位置的后面那个数据元素。
// 若删除失败(为空表,或当前位置时表尾),则返回false,否则返回true。
// 15)
int LL_FindValue(LinkList* llist, T x);
// 找到线性表中第一个值为x的数据元素的编号。
// 返回值-1表示没有找到,返回值>=0表示编号。
// 16)
int LL_DelValue(LinkList* llist, T x);
// 删除第一个值为x的数据元素,返回该数据元素的编号。如果不存在值为x的数据元素,则返回-1。
// 17)
void LL_Print(LinkList* llist);
// 打印整个线性表。
#endif // LINKED_LIST_H_LIELJE7398CNHD_INCLUDE_
// 单链表实现文件
#include 3 就地归并两个有序表
编程要求
首先建立两个有序单链表,就地归并后输出。
测试说明
平台会对你编写的代码进行测试:
7 //输入第一个表的长度n1
2 4 7 8 10 13 18 //依次输入n1个有序的元素
5 /输入第一个表的长度n2
3 4 5 6 9 //依次输入n2个有序的元素
预期输出:
归并表为:2 3 4 5 6 7 8 9 10 13 18
//有序表就地归并
#include总结:
- 注意题目是说:有序链表(测试的时候千万不要乱输入,否则输出结果就是将两个表拼接)
- 当*pa==*pb时要单独考虑
- pa本身是一个数据指针,有对应的地址,不加*使用就是指针,加上使用就代表对应地址的值
- &L.elem[i]对他取地址,是对应元素又有一个地址,而本身L.elem是一个指针。相当于它又构成了一个指针域。
- while(pb<=pb_last) *pc++=*pb++;
while(pa<=pa_last) *pc++=*pa++;
这里千万不要写反了
4 两个一元多项式异地相加
#include 5 约瑟夫环问题
#include实验三 栈之基础
1 顺序存储的栈
输入格式:
首先输入一个正整数max,创建一个最多可存储max个元素的栈。然后输入多个操作:如果输入”push”,则后面跟一个数x,表示x进栈;如果输入”pop”,表示出栈操作;如果输入”end”,表示输入结束。
输出格式:
输出栈的长度,然后从栈底到栈顶依次输出各元素。
样例输入:
6
push 56
push 15
push 12
push 13
pop
end
样例输出
Stack length: 3
stack data (from bottom to top): 56 15 12
SeqStack.h
typedef int T;
struct SeqStack{
T* data; // 数据元素指针
int top; // 栈顶元素编号
int max; // 最大节点数
};
SeqStack* SS_Create(int maxlen);
void SS_Free(SeqStack* ss);
void SS_MakeEmpty(SeqStack* ss);
bool SS_IsFull(SeqStack* ss);
bool SS_IsEmpty(SeqStack* ss);
int SS_Length(SeqStack* ss);
bool SS_Push(SeqStack* ss, T x);
bool SS_Pop(SeqStack* ss, T& item);
bool SS_Top(SeqStack* ss, T& item);
void SS_Print(SeqStack* ss);
//顺序存储的栈 实现文件
/
#include 2 实现一个链接存储的栈
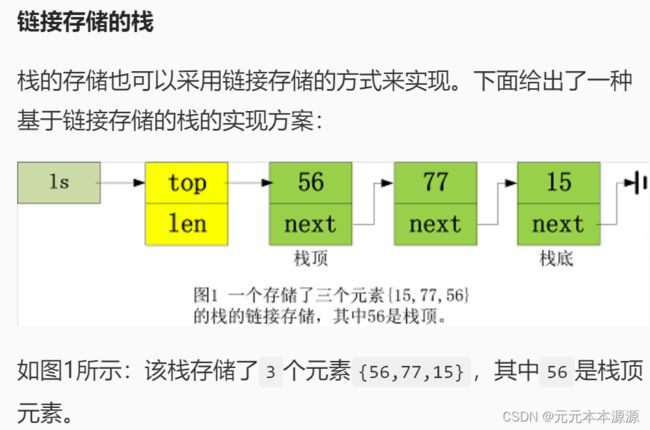
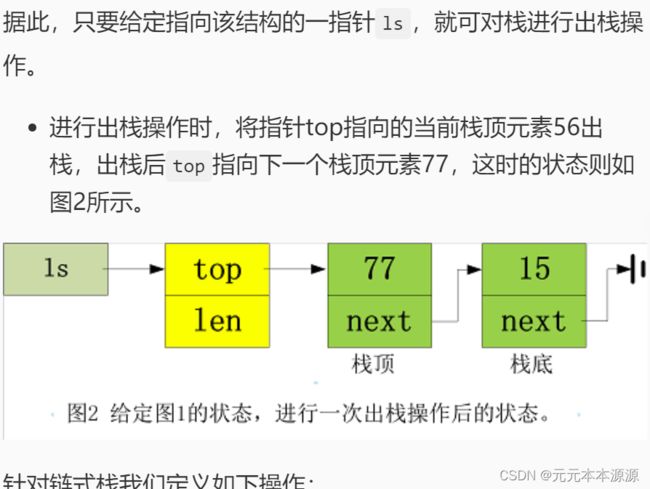
这种实现方案中与栈相关的两个属性元素top和len介绍如下:
编程要求
本关任务是实现step2/LnkStack.cpp中的LS_IsEmpty、LS_Length、LS_Push、LS_Pop和LS_Top五个操作函数,以实现判断栈是否为空、求栈的长度、进栈、出栈以及获取栈顶元素等功能。具体要求如下:
输入输出格式请参见后续说明及测试样例。
本关任务是实现step2/LnkStack.cpp中的LS_IsEmpty、LS_Length、LS_Push、LS_Pop和LS_Top五个操作函数,以实现判断栈是否为空、求栈的长度、进栈、出栈以及获取栈顶元素等功能。具体要求如下:
-
LS_IsEmpty:判断栈是否为空,若栈为空,则返回true,否则返回false。
-
LS_Length:获取链式栈的长度。
-
LS_Push:将元素x进栈,若满栈则无法进栈,返回false,否则返回true。
-
LS_Pop:若出栈成功(栈不为空),则返回true;否则(空栈),返回false。
-
LS_Top:获取栈顶元素。
// 栈的链接存储 头文件
#if !defined(LINKED_STACK_H_985552)
#define LINKED_STACK_H_985552
typedef int T; //数据元素类型
struct LNode {
T data;
LNode* next;
};
struct LinkStack {
LNode* top; // 栈顶指针
int len; // 栈的长度
};
LinkStack* LS_Create();
void LS_Free(LinkStack* ls);
void LS_MakeEmpty(LinkStack* ls);
bool LS_IsEmpty(LinkStack* ls);
int LS_Length(LinkStack* ls);
void LS_Push(LinkStack* ls, T x);
bool LS_Pop(LinkStack* ls, T& item);
bool LS_Top(LinkStack* ls, T& item);
void LS_Print(LinkStack* ls);
#endif
//
// 链接存储的栈实现文件
#include 实验三 栈之应用
第1关:利用栈实现整数的十进制转八进制
样例一:
测试输入:71
预期输出:107
样例二:
测试输入:8
预期输出:10
#ifndef stack__h
#define stack__h
#endif /* stack__h */
#include 第2关:利用栈判断字符串括号是否匹配
编程要求:基于栈stack数据结构判断字符串中的括号是否匹配,字符串中仅包含如下字符:( ) [ ] { }。
样例一:
测试输入:
6
{[()]}
预期输出:
YES
样例二:
测试输入:
4
[(])
预期输出:
NO
Stack* Stack_Create(int maxlen)
// 创建栈
{
Stack* stk = (Stack*)malloc(sizeof(Stack));
stk->data = (T*)malloc(sizeof(T)*maxlen);
stk->max = maxlen;
stk->top = -1;
return stk;
}
void Stack_Free(Stack* stk)
// 释放栈
{
free(stk->data);
free(stk);
}
void Stack_MakeEmpty(Stack* stk)
// 置为空栈
{
stk->top = -1;
}
bool Stack_IsEmpty(Stack* stk)
// 判断栈是否空
{
return -1 == stk->top;
}
bool Stack_IsFull(Stack* stk)
// 判断栈是否满
{
return stk->top == stk->max-1;
}
T Stack_Top(Stack* stk)
// 获取当前栈顶元素
{
return stk->data[stk->top];
}
T Stack_Push(Stack* stk, T e)
// 将元素e压入栈顶
// 返回栈顶点元素
{
if(Stack_IsFull(stk)) {
printf("Stack_IsFull(): stack full error when push element to the stack!\n");
Stack_Free(stk);
exit(0);
}
else{
stk->top += 1;
stk->data[stk->top] = e;
return Stack_Top(stk);
}
}
T Stack_Pop(Stack* stk)
// 将栈顶元素出栈
// 返回栈顶元素
{
if(Stack_IsEmpty(stk)) {
printf("Stack_IsEmpty(): stack empty error when pop element of the stack top!\n");
Stack_Free(stk);
exit(0);
}
else{
T topE = Stack_Top(stk);
stk->top -= 1;
return topE;
}
}
void Stack_Print(Stack* stk)
// 打印栈顶到栈低的元素
{
if (Stack_IsEmpty(stk)) {
printf("The stack is empty.\n");
return;
}
//printf("The stack contains: ");
for (int i=stk->top; i>=0; i--) {
printf("%d", stk->data[i]);
}
printf("\n");
}
void Bracket_Match(T* str, int len)
// 利用stack栈判断括号是否匹配
// 输入参数:字符串序列,字符串长度
// 若匹配输出YES,否则输出NO,末尾换行
{
// 请在这里补充代码,完成本关任务
/********** Begin *********/
Stack *stk=Stack_Create(len);
int i;
char ch;
bool flag=1;
//判断栈是否满
Stack_IsFull(stk);
for(i=0;i<len;++i)
{
switch(str[i])
{
case'(':
case'[':
case'{':Stack_Push(stk,str[i]); break;//入栈
case')':
case']':
case'}':Stack_Top(stk);// 获取当前栈顶元素
ch=stk->data[stk->top];//赋值给ch
if((ch=='('&&str[i]==')')||(ch=='['&&str[i]==']')||(ch=='{'&&str[i]=='}')){
Stack_Pop(stk);// 将栈顶元素出栈
}
else {
flag=0;
}
}
}
//判断栈顶指针是否为空,不为空则说明还没判断完成
if (stk->top!=-1)
flag = 0;
if (flag==1)
printf("YES\n");
else
printf("NO\n");
/********** End **********/
}
第3关:利用栈判断字符串是否为回文串
样例一:
测试输入:
4
1221
预期输出:
YES
样例二:
测试输入:
7
abababa
预期输出:
YES
Stack* Stack_Create(int maxlen)
// 创建栈
{
Stack* stk = (Stack*)malloc(sizeof(Stack));
stk->data = (T*)malloc(sizeof(T)*maxlen);
stk->max = maxlen;
stk->top = -1;
return stk;
}
void Stack_Free(Stack* stk)
// 释放栈
{
free(stk->data);
free(stk);
}
void Stack_MakeEmpty(Stack* stk)
// 置为空栈
{
stk->top = -1;
}
bool Stack_IsEmpty(Stack* stk)
// 判断栈是否空
{
return -1 == stk->top;
}
bool Stack_IsFull(Stack* stk)
// 判断栈是否满
{
return stk->top == stk->max-1;
}
T Stack_Top(Stack* stk)
// 获取当前栈顶元素
{
return stk->data[stk->top];
}
T Stack_Push(Stack* stk, T e)
// 将元素e压入栈顶
// 返回栈顶点元素
{
if(Stack_IsFull(stk)) {
printf("Stack_IsFull(): stack full error when push element to the stack!\n");
Stack_Free(stk);
exit(0);
}
else{
stk->top += 1;
stk->data[stk->top] = e;
return Stack_Top(stk);
}
}
T Stack_Pop(Stack* stk)
// 将栈顶元素出栈
// 返回栈顶元素
{
if(Stack_IsEmpty(stk)) {
printf("Stack_IsEmpty(): stack empty error when pop element of the stack top!\n");
Stack_Free(stk);
exit(0);
}
else{
T topE = Stack_Top(stk);
stk->top -= 1;
return topE;
}
}
void Stack_Print(Stack* stk)
// 打印栈顶到栈低的元素
{
if (Stack_IsEmpty(stk)) {
printf("The stack is empty.\n");
return;
}
//printf("The stack contains: ");
for (int i=stk->top; i>=0; i--) {
printf("%d", stk->data[i]);
}
printf("\n");
}
void Palindrome(T* str, int len)
// 利用stack栈判断字符串是否为回文串
// 输入参数:字符串序列,字符串长度
// 若是回文串输出YES,否则输出NO,末尾换行
{
// 请在这里补充代码,完成本关任务
/********** Begin *********/
if(len==1){
printf("YES\n");
}else{
//创建一个栈
Stack *s=Stack_Create(100);
//判断长度为奇偶
if(len%2!=0){
for(int i=len/2;i<len;i++){
str[i]=str[i+1];
}
for(int i=0;i<len-1;i++){
//将当前栈顶元素赋给st
char st=Stack_Top(s);
if(st==str[i]){
//匹配后将栈顶元素出栈,返回栈顶元素
Stack_Pop(s);
}else{
//将元素str[i]压入新栈s
Stack_Push(s,str[i]);
}
}
if(Stack_IsEmpty(s)){
printf("YES\n");
}else{
printf("NO\n");
}
}else{
for(int i=0;i<len;i++){
char st=Stack_Top(s);
if(st==str[i]){
Stack_Pop(s);
}else{
Stack_Push(s,str[i]);
}
}
if(Stack_IsEmpty(s)){
printf("YES\n");
}else{
printf("NO\n");
}
}
}
/********** End **********/
}
队列之基础
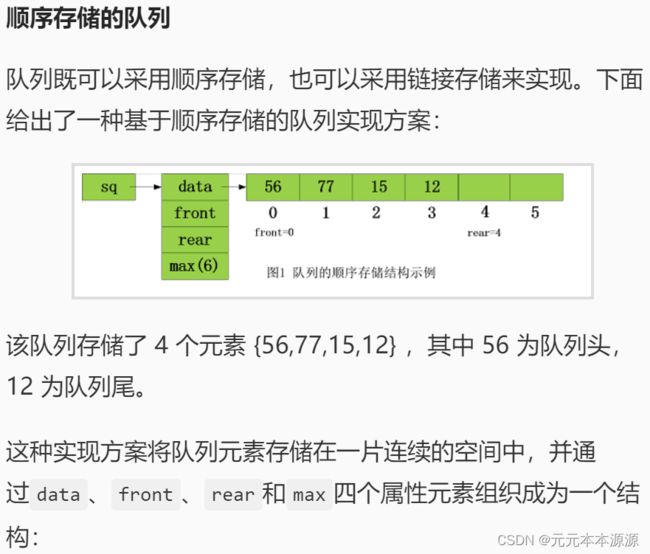
第1关:实现一个顺序存储的队列
#if !defined(SEQUENCE_QUEUE_H_LIELJE7398CNHD_INCLUDE_)
#define SEQUENCE_QUEUE_H_LIELJE7398CNHD_INCLUDE_
/
typedef int T;
struct SeqQueue {
T* data; // 指向数据元素数组的指针。
int front; // 下一个出队元素的数组下标。
int rear; // 下一个入队元素应该存放的单元的数组下标。
int max; // 队列中最多可放max-1个数据元素,留一个空数据单元以区分空和满。
};
SeqQueue* SQ_Create(int maxlen);
void SQ_Free(SeqQueue* sq);
void SQ_MakeEmpty(SeqQueue* sq);
bool SQ_IsEmpty(SeqQueue* sq);
bool SQ_IsFull(SeqQueue* sq);
int SQ_Length(SeqQueue* sq);
bool SQ_In(SeqQueue* sq, T x);
bool SQ_Out(SeqQueue* sq, T& item);
bool SQ_Head(SeqQueue* sq, T& head);
void SQ_Print(SeqQueue* sq);
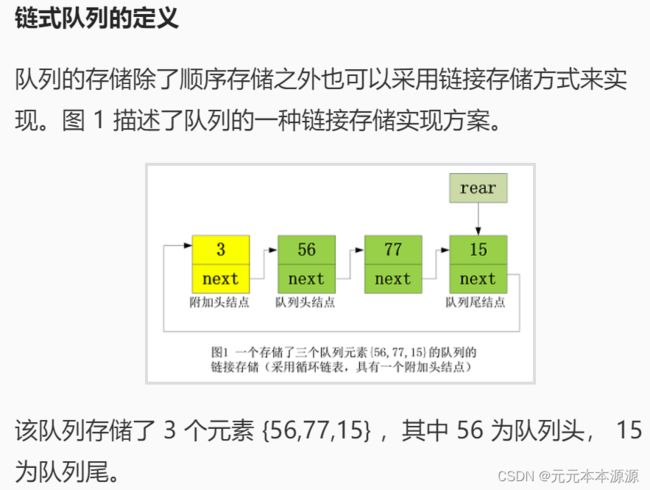
#include #include 第2关:实现一个链接存储的队列
-
输入格式:
输入多个操作:如果输入 “in” ,则后面跟一个数 x ,表示 x 入队列;如果输入 “out” ,表示出队列操作;如果输入 “end” ,表示输入结束。 -
输出格式:
输出队列长度,然后从队头到队尾依次输出队列的各元素。
typedef int T;
struct LNode {
T data;
LNode* next;
};
#include #include