- java并发编程LockSupport之park/unpark
jmysql
javajava
【尚学堂】Java300集零基础适合初学者视频教程_Java300集零基础教程_Java初学入门视频基础巩固教程_Java语言入门到精通_哔哩哔哩_bilibili一、简介1.1主要方法Park/UnPark方法是LockSupport当中的方法。其常用方法有如下:park():暂停当前线程。park(Objectblocker):暂停当前线程,并指定负责此线程停放的同步对像。parkNanos(
- tailwindcss报错
于慨
javascript
warn-The`content`optioninyourTailwindCSSconfigurationismissingorempty.warn-ConfigureyourcontentsourcesoryourgeneratedCSSwillbemissingstyles.warn-https://tailwindcss.com/docs/content-configurationtrans
- 那个情人节送Harry Winston钻戒的土豪程序猿
程序员1024故事集
情人节那天A在群里发了张F君的朋友圈,大呼这是今天见过的朋友圈里最土豪的礼物了!只见在法拉利的车里,娇艳的红玫瑰簇拥着一个HarryWinston的心形钻戒!原本只知道F君有钱,可没想到这么有钱。以前看GossipGirls里一众名媛的唯一选择就是HarryWinston,据说号称是钻石之王。以下是HarryWinston的百科资料:享誉全球超过百年的超级珠宝品牌,在切割钻石上的精湛工艺与周密谨慎
- 网易云音乐会员优惠大揭秘,网友:太值了!
氧惠佣金真的高
在数字音乐时代,拥有一款高品质的音乐APP是音乐爱好者的必备之选。作为中国音乐市场的佼佼者,网易云音乐凭借其丰富的曲库、出色的推荐算法以及浓厚的社区氛围,吸引了大量用户。近日,网易云音乐推出了一系列会员优惠活动,让我们一起来了解一下吧!大家好,我是氧惠联合创始人七言导师,给大家推荐一款省钱更加赚钱的app——氧惠。氧惠是与以往完全不同的抖客+淘客app!2023全新模式,我的直推也会放到你下面。主
- Java反射获取Class的三种方式
喵星人ZC
packagecom.sou.reflection.classpkg;importorg.junit.Test;importjava.lang.reflect.Method;/***反射获取Class的三种方式*1)Class.forName()*2)obj.getClass*3)class.class*/publicclassReflectionApp{//Class.forName()@Tes
- 微算法科技基于格密码的量子加密技术,融入LSQb算法的信息隐藏与传输过程中,实现抗量子攻击策略强化
MicroTech2025
量子计算区块链
随着量子计算技术的发展,传统加密算法面临被量子计算机破解的风险,LSQb算法也需考虑应对未来可能的量子攻击。微算法科技基于格密码的量子加密技术,融入LSQb算法的信息隐藏与传输过程中,实现抗量子攻击策略强化。格密码在面对量子攻击时具有较高的安全性,通过这种融合,能为LSQb算法提供更强大的抗攻击能力,确保信息在复杂的量子计算环境下的安全性。格密码是一种基于数学格结构的密码学方法,具有在量子计算环境
- 微算法科技技术突破:用于前馈神经网络的量子算法技术助力神经网络变革
MicroTech2025
量子计算算法神经网络
随着量子计算和机器学习的迅猛发展,企业界正逐步迈向融合这两大领域的新时代。在这一背景下,微算法科技(NASDAQ:MLGO)成功研发出一套用于前馈神经网络的量子算法,突破了传统神经网络在训练和评估中的性能瓶颈。这一创新性的量子算法以经典的前馈和反向传播算法为基础,借助量子计算的强大算力,极大提升了网络训练和评估效率,并带来了对过拟合的天然抗性。前馈神经网络是深度学习的核心架构,广泛应用于图像分类、
- 微算法科技研究量子视觉计算,利用量子力学原理提升传统计算机视觉任务的性能
计算机视觉,作为人工智能领域的一个重要分支,致力于模拟人类视觉系统对图像或视频等视觉数据的理解与分析能力。它涵盖了图像识别、目标检测、图像分割等一系列复杂任务,广泛应用于自动驾驶、医疗影像分析、安防监控等多个领域。然而,随着数据规模的不断膨胀和任务复杂度的日益提升,传统计算机视觉算法在处理大规模、高维度数据时遇到了性能瓶颈。微算法科技(NASDAQ:MLGO)研究量子视觉计算,探索量子计算与经典卷
- 图机器学习(13)——图相似性检测
图机器学习(13)——图相似性检测0.前言1.基于图嵌入的方法2.基于图核的方法3.基于GNN的方法4.应用0.前言图机器学习(machinelearning,ML)方法能广泛应用于各类任务,其应用场景涵盖从药物设计到社交网络推荐系统等多个领域。值得注意的是,由于这类方法在设计上具有通用性,同一算法可用于解决不同问题。学习图之间相似性的定量度量是一个关键问题。事实上,这是网络分析的重要步骤,同时也
- linux安装Node.js 环境,Docker 环境,Ruby 环境,MongoDB 环境,PostgreSQL 数据库,Go 开发环境,Python 虚拟环境
2401_87017622
数据库linuxnode.js
在Linux上安装其他常见的开发环境可以根据具体需求而定,以下是一些常见的安装步骤:1.Node.js环境Node.js是一个基于ChromeV8引擎的JavaScript运行环境,适用于服务器端开发。安装Node.js:通过包管理器安装:sudoyuminstall-ygcc-c++makecurl-sLhttps://rpm.nodesource.com/setup_14.x|sudo-Eba
- 详解NIO Channel类
沧澜sincerely
Java高并发nio
目录什么是NIOChannel?为什么要学习NIOChannel?FileChannelSocketChannelDatagramChannel本篇文章内容的前置知识为NIOBuffer类,如果不了解,可点击链接学习详解NIOBuffer类及其属性和方法-CSDN博客什么是NIOChannel?在JavaNIO中,Channel(通道)是一种广义的I/O抽象,用于表示与数据源或数据目的地之间的连接
- 常用的折叠展开过渡动画效果css
如何实现优雅的折叠展开动画效果在现代Web设计中,折叠展开动画是一种常见且实用的交互方式,它可以帮助用户在保持界面简洁的同时,灵活控制内容的显示与隐藏。本文将分享如何使用HTML、CSS和JavaScript实现一个平滑流畅的折叠展开动画效果。基本原理折叠展开动画的核心原理是通过CSS过渡(transition)来控制元素的高度、内边距和其他样式属性的变化。当用户点击触发按钮时,JavaScrip
- Redux架构解析:状态管理的核心原理
止观止
架构前端react.jsredux
Redux作为JavaScript应用的状态管理库,其技术架构与核心原理围绕可预测的状态管理设计,通过严格的单向数据流和函数式编程理念实现复杂应用的状态控制。以下从设计理念、核心架构、工作流程、源码实现等角度进行系统性剖析:一、设计理念与原则单一数据源(SingleSourceofTruth)整个应用的状态存储在一个全局Store对象中,形成唯一的状态树(StateTree)。优势:简化状态共享和
- Kafka 时间轮深度解析:如何O(1)处理定时任务
lifallen
KafkaJavakafkalinq分布式java数据库数据结构apache
TimingWheel(时间轮)TimingWheel是一种高效的、用于实现大量定时任务调度的算法结构。相比于传统的基于优先队列(PriorityQueue)的定时器(其添加/删除操作的时间复杂度为O(logn)),时间轮可以实现近乎O(1)的添加和删除操作,这在需要管理成千上万个定时任务的场景下(例如Kafka中的请求超时、延迟操作等)具有巨大的性能优势。可以把一个TimingWheel想象成一
- 一天学会超级玛丽小游戏_手把手教学_Java小游戏
62f5ecb72f71
超级玛丽是任天堂制作的一款小游戏,在的童年里一起玩这个游戏,大胡子,背带裤的马里奥,每关以马里奥在走到重点的前提下尽可能地收集金币。他在闯关过程中,会遇到怪物,可以通过踩死或者跳过。也会遇到深坑。给游戏增加了一定的难度。今天带大家用java制作制作这款小游戏,下面是课程介绍.课程介绍:在你的童年记忆里,是否有一个会蹦跳,会吃蘑菇的小人?超级玛丽是一款经典并且流行的小游戏,通过键盘来控制马里奥的移动
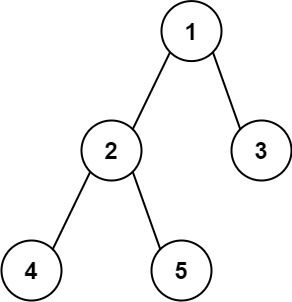
- 【算法训练营Day12】二叉树part2
十八岁讨厌编程
算法训练营算法
文章目录翻转二叉树对称二叉树二叉树的最大深度二叉树的最小深度翻转二叉树题目链接:226.翻转二叉树解题逻辑:翻转二叉树也就是将所有非叶节点的左右孩子相互交换,那么我们就可以采用层序遍历判断非叶节点进行翻转:初始化一个辅助队列将根节点添加到队列中去弹出队头元素如果该元素的两个子节点均不为null则翻转两个子节点然后将子节点入队如此循环往复直到队列为空代码如下:classSolution{public
- java LockSupport park() unpark() 的用法&和wait() notify()的区别
javaLockSupportpark()unpark()的用法ockSupport类是Java并发包中的一个工具类,提供了一些基本的线程阻塞和唤醒操作。其中,unpark(Threadthread)方法是用来唤醒指定线程的关键方法。下面详细解释一下unpark方法:unpark方法的作用:唤醒线程:unpark方法可以解除指定线程的阻塞状态,使其有机会继续执行。如果线程在调用park()之前被u
- 3步搞定Java漏洞修复?别再让黑客当“家”!
关注墨瑾轩,带你探索编程的奥秘!超萌技术攻略,轻松晋级编程高手技术宝库已备好,就等你来挖掘订阅墨瑾轩,智趣学习不孤单即刻启航,编程之旅更有趣Java城堡的“裂缝”与程序员的救赎想象一下:你的Java应用是一座巍峨的城堡,而安全漏洞就是那些悄悄蔓延的裂缝。SQL注入:像是小偷从窗户溜进来,偷偷改写数据库的账本。XSS攻击:像在城堡里偷偷放了一张带毒的地毯,路过的人会被“刺”伤。SSRF漏洞:像让城堡
- 5大核心技术+3大交互革命!Java如何让虚拟世界‘活过来’?——附代码实战+防坑指南!
墨瑾轩
Java乐园交互java开发语言
关注墨瑾轩,带你探索编程的奥秘!超萌技术攻略,轻松晋级编程高手技术宝库已备好,就等你来挖掘订阅墨瑾轩,智趣学习不孤单即刻启航,编程之旅更有趣从“木头人”到“交互大师”的Java魔法之旅一、Java的“五大核心技术”——虚拟世界的“五感开关”1.1核心技术1:JOGL渲染引擎——“视觉中枢”作用:用OpenGL实现3D场景渲染代码示例://JOGL渲染循环:画一个旋转的立方体importjavax.
- 高通camera结构(第五天)
一、摄像头的结构和工作原理镜头用来拍摄景物,拍摄的图片在传感器上将光信号转换成了电信号,电信号经过AD转换器(模数转换器)转换成了数字信号,数字信号经过DSP(数字信号处理器)进行加工处理,再被送到电脑中进行处理,最终转换成了手机屏幕上我们可以看到的图像。数字信号处理器芯片(DSP)功能:主要是通过一系列数学的算法运算,对数字图像信号进行优化处理,并把处理过的信号通过USB等接口传到PC等设备。D
- Java多线程、锁、线程池详解
Java多线程、锁、线程池详解在现代软件开发中,多线程编程是提高程序性能和响应能力的重要手段。Java提供了丰富的多线程支持,包括线程的创建、同步、通信以及线程池管理等。本文将深入探讨Java中的多线程、锁机制、线程池的原理和应用,并涵盖成员方法、并行、调度、同步、死锁、睡眠、唤醒以及线程状态等知识。一、多线程基础1.多线程的概念多线程允许程序同时执行多个任务,从而提高程序的执行效率。2.多线程的
- 打球心得
经书
今年开始与金沙洲医院的医生一起打羽毛球了,我们每周五晚上6-8点打两个小时。刚开始时,参加的人数不少,后来慢慢的去的人也开始固定了,就那么七八个人,然后双打轮流上。这几个月的时间,是我自从毕业后运动最多的日子,以前在昆明和来广州的一年都没有持续的锻炼,只有隔三差五的运动,所以身体体质也不是很好。羽毛球是我一直在玩的运动,从小时候到现在,一直没间断过。羽毛球是全民运动,大家都会打几下,但是业务与专业
- 如何使用Google AdSense实现H5小游戏变现(二)
Louisa的工作日记
上一篇内容主要介绍了H5小游戏的市场情况洞察、GoogleAdSense介绍、收入核心指标,本篇文章将介绍如何改善展示次数、eCPM,以及如何使用GA来优化网站质量。01.如何改善展示次数1、网站DAU网站DAU和你的渠道息息相关,这里作为H5小游戏网站常常容易遇到的问题:服务器承载压力过大崩溃。需要时刻监控服务器情况,海外推荐使用亚马逊相关服务,国内服务提供商均存在不稳定情况。此外,必须支持ht
- 推荐算法召回:架构理解
Jay Kay
c++推荐算法推荐算法架构算法
一、召回服务的定位与挑战召回层是推荐系统的第一道漏斗,负责从亿级候选集中筛选出千级别的相关项,其效果直接决定推荐效果的天花板。核心挑战包括:低延迟约束:需在50ms内完成海量候选检索;高召回率要求:98%的召回率需覆盖用户多样化兴趣;数据漂移应对:实时用户行为分布变化需动态适应;误杀控制:避免优质内容被过度过滤引发用户投诉。⚙️二、召回服务核心架构1.多路召回并行召回策略实现方式适用场景规则召回基
- A*算法详解
A*算法详解一、A*算法基础概念1.1算法定位1.2核心评估函数1.3关键数据结构二、A*算法的核心步骤三、启发函数设计3.1网格地图中的启发函数3.2启发函数的选择原则三、Java代码实现四、启发函数的设计与优化4.1启发函数的可采纳性4.2启发函数的效率影响4.3常见启发函数对比五、A*算法的应用场景与拓展5.1典型应用5.2算法拓展六、A*算法的优缺点优点缺点从游戏中的角色寻路到机器人导航,
- 分层图最短路径算法详解
GG不是gg
数据结构与算法分析#算法分析与设计图搜索算法
分层图最短路径算法详解一、分层图算法的核心思想1.1问题引入:带约束的最短路径1.2分层图的核心思路二、分层图的构建方法2.1分层图的结构定义2.2构建步骤(以“最多k次边权改为0”为例)三、分层图最短路径的求解3.1算法步骤3.2Java代码实现(以Dijkstra为例)四、分层图算法的关键细节4.1状态表示与空间优化4.2边的处理4.3复杂度分析五、典型应用场景5.1带次数约束的路径优化5.2
- Tomcat生命周期原理深度剖析
Tomcat生命周期原理深度剖析本文围绕Tomcat生命周期机制,结合架构图、源码精讲、设计思想、实际案例、调优技巧等全方位解读,帮助读者系统掌握Tomcat生命周期的本质与应用。一、Tomcat生命周期概述Tomcat作为JavaWeb服务器的代表,其架构中每个核心组件(如Server、Service、Engine、Host、Context、Wrapper等)都拥有独立的生命周期。Tomcat通
- 信息学奥赛-一本通-第二部分 基础算法 --> 第五章 搜索与回溯算法
攻城丶狮
C++比赛信息算法深度优先图论c++青少年编程
1317:【例5.2】组合的输出【题目描述】排列与组合是常用的数学方法,其中组合就是从n个元素中抽出r个元素(不分顺序且r≤n),我们可以简单地将n个元素理解为自然数1,2,…,n,从中任取r个数。现要求你用递归的方法输出所有组合。例如n=5,r=3,所有组合为:123124125134135145234235245345【题目分析】1.搜索函数参数:上一次搜索的数字i(i(n)>=i(n-1))
- Java使用Langchai4j接入AI大模型的简单使用(四)--整合Springboot
moxiaoran5753
javaspringbootspring
一、在Maven中引入依赖核心配置如下,这里使用的是Springboog3,jdk17,注意版本,如果启动不起来很有可能是版本冲突:17UTF-8UTF-83.4.31.0.0-beta1dev.langchain4jlangchain4j-community-dashscope-spring-boot-starterorg.springframework.bootspring-boot-star
- gin数据解析和绑定
rit8432499
giniphoneios
go代码packagemainimport("net/http""github.com/gin-gonic/gin")//定义接收JSON数据的结构体typeLoginstruct{Usernamestring`form:"username"json:"username"uri:"username"xml:"username"binding:"required"`Passwordstring`fo
- linux系统服务器下jsp传参数乱码
3213213333332132
javajsplinuxwindowsxml
在一次解决乱码问题中, 发现jsp在windows下用js原生的方法进行编码没有问题,但是到了linux下就有问题, escape,encodeURI,encodeURIComponent等都解决不了问题
但是我想了下既然原生的方法不行,我用el标签的方式对中文参数进行加密解密总该可以吧。于是用了java的java.net.URLDecoder,结果还是乱码,最后在绝望之际,用了下面的方法解决了
- Spring 注解区别以及应用
BlueSkator
spring
1. @Autowired
@Autowired是根据类型进行自动装配的。如果当Spring上下文中存在不止一个UserDao类型的bean,或者不存在UserDao类型的bean,会抛出 BeanCreationException异常,这时可以通过在该属性上再加一个@Qualifier注解来声明唯一的id解决问题。
2. @Qualifier
当spring中存在至少一个匹
- printf和sprintf的应用
dcj3sjt126com
PHPsprintfprintf
<?php
printf('b: %b <br>c: %c <br>d: %d <bf>f: %f', 80,80, 80, 80);
echo '<br />';
printf('%0.2f <br>%+d <br>%0.2f <br>', 8, 8, 1235.456);
printf('th
- config.getInitParameter
171815164
parameter
web.xml
<servlet>
<servlet-name>servlet1</servlet-name>
<jsp-file>/index.jsp</jsp-file>
<init-param>
<param-name>str</param-name>
- Ant标签详解--基础操作
g21121
ant
Ant的一些核心概念:
build.xml:构建文件是以XML 文件来描述的,默认构建文件名为build.xml。 project:每个构建文
- [简单]代码片段_数据合并
53873039oycg
代码
合并规则:删除家长phone为空的记录,若一个家长对应多个孩子,保留一条家长记录,家长id修改为phone,对应关系也要修改。
代码如下:
- java 通信技术
云端月影
Java 远程通信技术
在分布式服务框架中,一个最基础的问题就是远程服务是怎么通讯的,在Java领域中有很多可实现远程通讯的技术,例如:RMI、MINA、ESB、Burlap、Hessian、SOAP、EJB和JMS等,这些名词之间到底是些什么关系呢,它们背后到底是基于什么原理实现的呢,了解这些是实现分布式服务框架的基础知识,而如果在性能上有高的要求的话,那深入了解这些技术背后的机制就是必须的了,在这篇blog中我们将来
- string与StringBuilder 性能差距到底有多大
aijuans
之前也看过一些对string与StringBuilder的性能分析,总感觉这个应该对整体性能不会产生多大的影响,所以就一直没有关注这块!
由于学程序初期最先接触的string拼接,所以就一直没改变过自己的习惯!
- 今天碰到 java.util.ConcurrentModificationException 异常
antonyup_2006
java多线程工作IBM
今天改bug,其中有个实现是要对map进行循环,然后有删除操作,代码如下:
Iterator<ListItem> iter = ItemMap.keySet.iterator();
while(iter.hasNext()){
ListItem it = iter.next();
//...一些逻辑操作
ItemMap.remove(it);
}
结果运行报Con
- PL/SQL的类型和JDBC操作数据库
百合不是茶
PL/SQL表标量类型游标PL/SQL记录
PL/SQL的标量类型:
字符,数字,时间,布尔,%type五中类型的
--标量:数据库中预定义类型的变量
--定义一个变长字符串
v_ename varchar2(10);
--定义一个小数,范围 -9999.99~9999.99
v_sal number(6,2);
--定义一个小数并给一个初始值为5.4 :=是pl/sql的赋值号
- Mockito:一个强大的用于 Java 开发的模拟测试框架实例
bijian1013
mockito单元测试
Mockito框架:
Mockito是一个基于MIT协议的开源java测试框架。 Mockito区别于其他模拟框架的地方主要是允许开发者在没有建立“预期”时验证被测系统的行为。对于mock对象的一个评价是测试系统的测
- 精通Oracle10编程SQL(10)处理例外
bijian1013
oracle数据库plsql
/*
*处理例外
*/
--例外简介
--处理例外-传递例外
declare
v_ename emp.ename%TYPE;
begin
SELECT ename INTO v_ename FROM emp
where empno=&no;
dbms_output.put_line('雇员名:'||v_ename);
exceptio
- 【Java】Java执行远程机器上Linux命令
bit1129
linux命令
Java使用ethz通过ssh2执行远程机器Linux上命令,
封装定义Linux机器的环境信息
package com.tom;
import java.io.File;
public class Env {
private String hostaddr; //Linux机器的IP地址
private Integer po
- java通信之Socket通信基础
白糖_
javasocket网络协议
正处于网络环境下的两个程序,它们之间通过一个交互的连接来实现数据通信。每一个连接的通信端叫做一个Socket。一个完整的Socket通信程序应该包含以下几个步骤:
①创建Socket;
②打开连接到Socket的输入输出流;
④按照一定的协议对Socket进行读写操作;
④关闭Socket。
Socket通信分两部分:服务器端和客户端。服务器端必须优先启动,然后等待soc
- angular.bind
boyitech
AngularJSangular.bindAngularJS APIbind
angular.bind 描述: 上下文,函数以及参数动态绑定,返回值为绑定之后的函数. 其中args是可选的动态参数,self在fn中使用this调用。 使用方法: angular.bind(se
- java-13个坏人和13个好人站成一圈,数到7就从圈里面踢出一个来,要求把所有坏人都给踢出来,所有好人都留在圈里。请找出初始时坏人站的位置。
bylijinnan
java
import java.util.ArrayList;
import java.util.List;
public class KickOutBadGuys {
/**
* 题目:13个坏人和13个好人站成一圈,数到7就从圈里面踢出一个来,要求把所有坏人都给踢出来,所有好人都留在圈里。请找出初始时坏人站的位置。
* Maybe you can find out
- Redis.conf配置文件及相关项说明(自查备用)
Kai_Ge
redis
Redis.conf配置文件及相关项说明
# Redis configuration file example
# Note on units: when memory size is needed, it is possible to specifiy
# it in the usual form of 1k 5GB 4M and so forth:
#
- [强人工智能]实现大规模拓扑分析是实现强人工智能的前奏
comsci
人工智能
真不好意思,各位朋友...博客再次更新...
节点数量太少,网络的分析和处理能力肯定不足,在面对机器人控制的需求方面,显得力不从心....
但是,节点数太多,对拓扑数据处理的要求又很高,设计目标也很高,实现起来难度颇大...
- 记录一些常用的函数
dai_lm
java
public static String convertInputStreamToString(InputStream is) {
StringBuilder result = new StringBuilder();
if (is != null)
try {
InputStreamReader inputReader = new InputStreamRead
- Hadoop中小规模集群的并行计算缺陷
datamachine
mapreducehadoop并行计算
注:写这篇文章的初衷是因为Hadoop炒得有点太热,很多用户现有数据规模并不适用于Hadoop,但迫于扩容压力和去IOE(Hadoop的廉价扩展的确非常有吸引力)而尝试。尝试永远是件正确的事儿,但有时候不用太突进,可以调优或调需求,发挥现有系统的最大效用为上策。
-----------------------------------------------------------------
- 小学4年级英语单词背诵第二课
dcj3sjt126com
englishword
egg 蛋
twenty 二十
any 任何
well 健康的,好
twelve 十二
farm 农场
every 每一个
back 向后,回
fast 快速的
whose 谁的
much 许多
flower 花
watch 手表
very 非常,很
sport 运动
Chinese 中国的
- 自己实践了github的webhooks, linux上面的权限需要注意
dcj3sjt126com
githubwebhook
环境, 阿里云服务器
1. 本地创建项目, push到github服务器上面
2. 生成www用户的密钥
sudo -u www ssh-keygen -t rsa -C "
[email protected]"
3. 将密钥添加到github帐号的SSH_KEYS里面
3. 用www用户执行克隆, 源使
- Java冒泡排序
蕃薯耀
冒泡排序Java冒泡排序Java排序
冒泡排序
>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>
蕃薯耀 2015年6月23日 10:40:14 星期二
http://fanshuyao.iteye.com/
- Excle读取数据转换为实体List【基于apache-poi】
hanqunfeng
apache
1.依赖apache-poi
2.支持xls和xlsx
3.支持按属性名称绑定数据值
4.支持从指定行、列开始读取
5.支持同时读取多个sheet
6.具体使用方式参见org.cpframework.utils.excelreader.CP_ExcelReaderUtilTest.java
比如:
Str
- 3个处于草稿阶段的Javascript API介绍
jackyrong
JavaScript
原文:
http://www.sitepoint.com/3-new-javascript-apis-may-want-follow/?utm_source=html5weekly&utm_medium=email
本文中,介绍3个仍然处于草稿阶段,但应该值得关注的Javascript API.
1) Web Alarm API
&
- 6个创建Web应用程序的高效PHP框架
lampcy
Web框架PHP
以下是创建Web应用程序的PHP框架,有coder bay网站整理推荐:
1. CakePHP
CakePHP是一个PHP快速开发框架,它提供了一个用于开发、维护和部署应用程序的可扩展体系。CakePHP使用了众所周知的设计模式,如MVC和ORM,降低了开发成本,并减少了开发人员写代码的工作量。
2. CodeIgniter
CodeIgniter是一个非常小且功能强大的PHP框架,适合需
- 评"救市后中国股市新乱象泛起"谣言
nannan408
首先来看百度百家一位易姓作者的新闻:
三个多星期来股市持续暴跌,跌得投资者及上市公司都处于极度的恐慌和焦虑中,都要寻找自保及规避风险的方式。面对股市之危机,政府突然进入市场救市,希望以此来重建市场信心,以此来扭转股市持续暴跌的预期。而政府进入市场后,由于市场运作方式发生了巨大变化,投资者及上市公司为了自保及为了应对这种变化,中国股市新的乱象也自然产生。
首先,中国股市这两天
- 页面全屏遮罩的实现 方式
Rainbow702
htmlcss遮罩mask
之前做了一个页面,在点击了某个按钮之后,要求页面出现一个全屏遮罩,一开始使用了position:absolute来实现的。当时因为画面大小是固定的,不可以resize的,所以,没有发现问题。
最近用了同样的做法做了一个遮罩,但是画面是可以进行resize的,所以就发现了一个问题,当画面被reisze到浏览器出现了滚动条的时候,就发现,用absolute 的做法是有问题的。后来改成fixed定位就
- 关于angularjs的点滴
tntxia
AngularJS
angular是一个新兴的JS框架,和以往的框架不同的事,Angularjs更注重于js的建模,管理,同时也提供大量的组件帮助用户组建商业化程序,是一种值得研究的JS框架。
Angularjs使我们可以使用MVC的模式来写JS。Angularjs现在由谷歌来维护。
这里我们来简单的探讨一下它的应用。
首先使用Angularjs我
- Nutz--->>反复新建ioc容器的后果
xiaoxiao1992428
DAOmvcIOCnutz
问题:
public class DaoZ {
public static Dao dao() { // 每当需要使用dao的时候就取一次
Ioc ioc = new NutIoc(new JsonLoader("dao.js"));
return ioc.get(