- 银行家算法
后会无期77
算法算法
文章目录银行家算法概述银行贷款案例A再次申请50万,能批准吗?B再次申请40万,能批准吗?或者C申请20万,能批准吗?安全序列和不安全序列多维度资源分配操作系统资源分配银行家算法总结数据结构银行家算法的步骤安全性算法步骤死锁的避免银行家算法概述银行家算法(Banker’sAlgorithm)是一个避免死锁(Deadlock)的著名算法,是由艾兹格·迪杰斯特拉在1965年为T.H.E系统设计的一种避
- java面试题
墨京
java面试java开发语言
1.list和set的区别?list底层是数组,有序可重复,按对象进入顺序保存元素,可以有多个null元素,可以使用该iterator迭代器取出元素,也可以直接get(intindex)下标,取出元素。底层数据结构:动态数组(arraylist)或链表(Linkedlist)set底层是,无序不可重复,最多只能储存一个null元素,只能使用iterator接口取出所有元素,再逐一遍历各个元素。底层
- 深入理解Redis
深入理解Redis:高性能内存数据库的核心原理与应用实践1.引言在现代互联网应用中,高性能、低延迟的数据访问是至关重要的。传统的关系型数据库(如MySQL)虽然功能强大,但在高并发场景下往往成为性能瓶颈。Redis(RemoteDictionaryServer)应运而生,作为一个开源的内存键值数据库,它凭借极快的读写速度、丰富的数据结构和灵活的扩展能力,成为缓存、会话存储、消息队列等场景的首选解决
- 数据结构笔记3:双向链表
逑之
数据结构笔记链表c语言学习经验分享算法
目录双向链表的方法:双向链表的初始化方法我们可以对比双向链表和单链表方法在实现上的区别:双向链表的实现引进头结点的概念:双向链表的优势:1、尾插尾删2、指定位置的插入和删除双向链表:也叫做有头节点的双向循环链表双向链表的方法:typedefintLTDataType;typedefstructListNode{LTDataTypex;structListNode*next;structListNo
- 列表反转:reverse() 方法的深度剖析
测试者家园
测试开发和测试Python零基础学Python人工智能Python零基础学Python零基础职场和发展软件开发和测试智能化测试
数据结构的基本操作始终是打牢编程基础的关键。而在对列表(list)这一核心数据结构的操作中,反转(reversing)是一项既常用又容易被低估的重要操作。Python提供了原地反转的reverse()方法,与返回新序列的切片[::-1]或内置函数reversed()形成了鲜明对比。本文将全面剖析list.reverse()方法,从其语义、实现机制、适用场景,到其在测试、开发与自动化中的实际运用,力
- AcWing--数据结构1
谢耳朵(wer~wer~)
Acwing学习数据结构c++算法
用数组来模拟链表。这种实现链表的方式也叫静态链表。1.单链表写邻接表:存储图和树我们定义:e[N]用来表示某个点的值是多少;ne[N]用来表示某个点的next指针是多少e和ne是用下标关联起来的如:head->3->5->7->9->空(下标从0开始,3的下标是0,以此类推,空的下标为-1)那么e[0]=3,ne[0]=1;e[1]=5,ne[1]=2;...e[3]=9,ne[3]=-1//单
- 红黑树与2-3树:插入、删除操作的时间复杂度与实现机制比较
一键难忘
红黑树数据结构
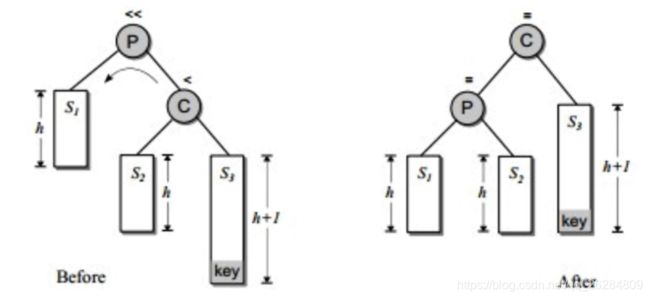
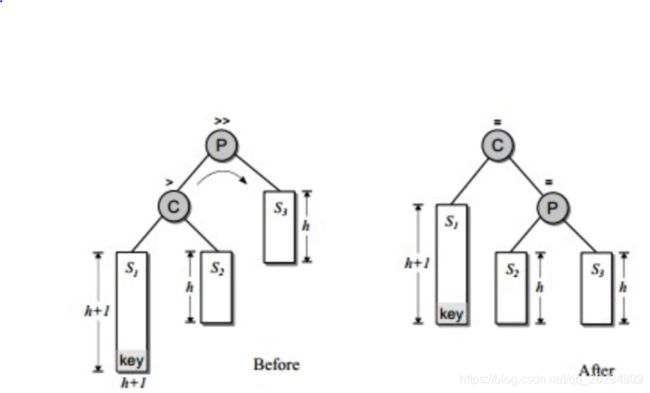
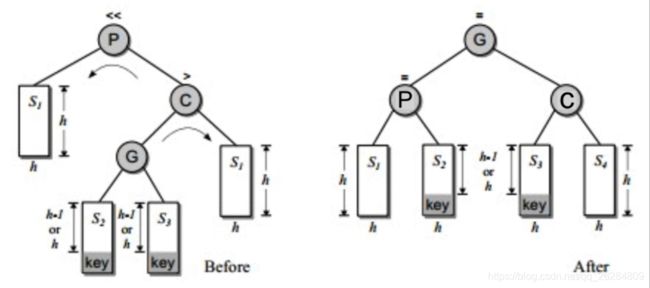
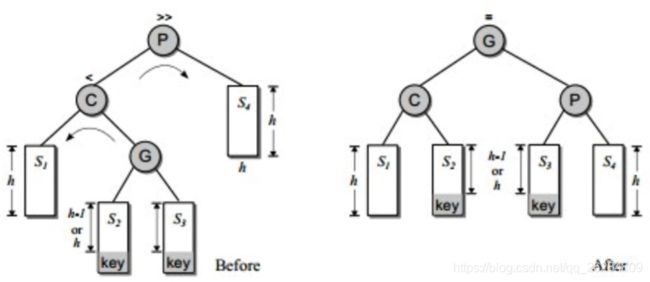
本文收录于专栏:算法之翼红黑树与2-3树:插入、删除操作的时间复杂度与实现机制比较红黑树(Red-BlackTree)和2-3树(2-3Tree)是两种广泛用于平衡二叉查找树的自平衡树结构。它们在插入、删除和查找操作中的性能都表现良好,并且可以确保树的高度是对数级别,从而保证了高效的操作时间。本文将对红黑树和2-3树进行深入的比较,并结合代码实例说明它们的实现和应用。1.数据结构简介1.1红黑树简
- linux驱动开发(20)-DMA(四)
yyc_audio
linux驱动开发驱动开发linux服务器
分散/聚集映射分散/聚集映射通过将虚拟地址上分散的DMA缓冲区通过一个类型为structscatterlist的数组或者链表组织起来,然后通过一次的DMA传输操作在主存RAM与设备之间传输数据,如图所示:图中显示了主存中三个分散的物理页面与设备之间进行的一次DMA传输时分散/聚集映射示意,其中单个物理页面与设备之间可以看做是一个单一的流式映射,每个这样的单一映射在内核中有数据结构structsca
- Go 中的 range 表达式详解:遍历数组、切片、字符串与 Map
Code季风
golang学习开发语言后端
在Go语言中,range是一个非常常用的结构,用于遍历集合类型的数据。它简洁、安全且易于使用,是Go开发者日常开发中最常使用的语法之一。本文将深入讲解Go的range表达式的使用方式、返回值含义以及常见错误,并通过多个示例帮助你更好地理解和应用range。一、什么是range?range是Go中用于迭代(遍历)集合类型的内置关键字,支持以下几种数据结构:数组(Array)切片(Slice)字符串(
- 数据结构与算法中单调栈的常见误区
数据结构与算法学习
服务器运维ai
数据结构与算法中单调栈的常见误区关键词:单调栈、数据结构、算法、误区、栈、时间复杂度、应用场景摘要:单调栈是一种特殊的数据结构,它在解决某些特定问题时非常高效。然而,许多初学者在使用单调栈时容易陷入一些常见的误区。本文将详细介绍单调栈的概念、原理和应用,重点分析使用单调栈时的常见误区,并通过实际代码示例展示如何正确使用单调栈解决问题。背景介绍目的和范围本文旨在帮助读者深入理解单调栈的概念和工作原理
- linux ARM64架构下进程切换核心代码分析
一、概述阶段核心代码/函数ARM64实现细节相关数据结构作用调度入口__schedule()调用context_switch()完成实际切换16structrq触发调度流程,选择下一个运行进程地址空间切换switch_mm_irqs_off()通过ttbr0_el1寄存器更新进程页表基址(PGD)3,处理ASID和TLB失效410structmm_struct(含pgd
- 【LangGraph】langgraph.store.base 模块:定义持久化键值存储的核心模块
彬彬侠
LangGraphLangGraphstorebase
有条理的详细介绍langgraph.store.base模块langgraph.store.base模块是LangGraph框架中用于定义持久化键值存储的核心模块,提供了标准化的接口和数据结构,以支持状态管理和长时记忆存储。它是LangGraph的重要组成部分,特别适合构建复杂、状态化的多代理应用。本文将从背景、功能、主要组件、使用方法、实际应用及注意事项等方面,详细介绍该模块,帮助开发者理解其设
- Python collections.abc模块介绍
qq_27390023
python开发语言
collections.abc是Python标准库中的一个模块,提供了一系列抽象基类(AbstractBaseClasses,ABCs),用于定义和检查容器类型(如序列、映射、集合等)的接口。这些抽象基类为常见的数据结构提供了统一的接口和行为规范,使得开发者可以更方便地实现和使用这些数据结构。1.collections.abc的作用collections.abc模块的主要作用是提供一组抽象基类,用
- 从Python到数据结构:为什么这是每个自学者必经的进阶之路
流水煮香茗
python数据结构mooc
当你熟练掌握Python语法后,下一步应该学什么?答案是数据结构。本文将深入分析为什么数据结构是编程进阶的关键,以及如何选择合适的学习资源。一、Python学会了,然后呢?如果你正在读这篇文章,很可能你已经:用Python写过小工具,能解决工作和生活中的一些小需求做过数据分析,会用pandas处理Excel表格但是,当你想要进一步提升时,却发现了一些困惑:困惑1:代码能跑,但总觉得"不够优雅"你的
- 【项目实战】Redis使用场景之基于Redis实现分布式限流
本本本添哥
002-进阶开发能力003-数据库redis分布式数据库
一、技术概览1.1定义分布式限流是指在分布式系统中限制请求的速率,以保护后端服务不被过多的请求压垮。它可以帮助我们控制系统的负载,保证服务的稳定性。Redis是一个高性能的键值存储系统,常用于缓存、消息队列和实时分析等场景。由于其支持丰富的数据结构和原子操作,非常适合用来实现分布式限流。专业术语:令牌桶算法(TokenBucket):一种流量整形算法,允许突发流量但不超过平均速度。漏桶算法(Lea
- 基于Anaconda环境开发IntelliJ IDEA实用JSON转Java实体插件
七夜zippoe
后端#Javajavajsonintellij-idea
在软件开发中,将JSON数据转换为Java实体类是常见需求。借助Anaconda环境强大的包管理能力与IntelliJIDEA的插件开发体系,我们可以打造一款高效实用的JSON转Java实体插件,显著提升开发效率。下面将从需求分析、技术选型、开发实现到优化部署,全方位阐述这款插件的开发过程。需求分析:明确痛点与功能方向在日常开发中,开发者经常需要根据JSON数据结构手动创建对应的Java实体类,这
- 整合性安全总结(ISS)早期规划
qq_34062333
临床试验NDA
1.ISS统一性建设工作启动1.1研究元数据标准化1.1.1不同类型研究元数据规范DBL研究锁定数据库后,需梳理元数据,确保信息完整准确,为后续分析奠定基础。OL研究进行中,实时更新元数据,反映研究进展,避免数据偏差影响结果。新启动研究,依据统一模板构建元数据,减少初期工作量,提高研究效率。1.1.2cADaM规范建立结合各类研究特点,制定跨研究核心分析数据集规范,提升数据整合性。规范涵盖数据结构
- 47、文件系统操作与管理
nnn11
C++编程精华:从基础到高级C++文件系统std::filesystem
文件系统操作与管理1.文件系统的概述文件系统是操作系统中用于组织、管理和存储文件的数据结构。在C++中,文件系统的操作主要依赖于标准库中的头文件,该库提供了丰富的API来处理文件和目录。通过std::filesystem命名空间,开发者可以轻松地进行文件路径解析、目录遍历、文件属性查询等操作,极大地提高了代码的可读性和可维护性。2.库简介C++17引入了库,使得文件系统操作更加简便和高效。std:
- 【学习】《算法图解》第七章学习笔记:树
程序员
前言在前面的章节中,我们学习了数组、链表、散列表等基本数据结构,以及一些基础算法。本章将介绍一种非常重要的数据结构——树(Tree),特别是二叉搜索树(BinarySearchTree)。树结构在计算机科学中应用广泛,从文件系统到数据库再到人工智能,都能看到树的身影。《算法图解》第七章深入浅出地介绍了树的基本概念、实现和应用,帮助读者理解这一关键数据结构。一、树的基本概念(一)什么是树树是一种分层
- 弹幕系统开发实战:QT框架与VS2015源码解析
Paula-柒月拾
本文还有配套的精品资源,点击获取简介:本源码项目融合了三个关键技术领域:弹幕系统设计、Qt框架开发和VisualStudio2015集成。它详细阐述了弹幕系统的核心功能实现,包括弹幕数据结构、渲染、碰撞检测和用户交互。同时,本项目介绍了如何利用Qt5的信号与槽机制、GUI组件和绘图系统来开发弹幕效果,并展示了如何在VisualStudio2015中进行项目管理、编辑、调试和构建。此项目提供了全面的
- Collection的子接口之【List】
丶小鱼丶
Java集合框架list数据结构
目录List自身提供了和index相关的方法List的特点List的常见实现类ArrayList底层数据结构是数组懒加载的体现最大容量为int类型的最大值扩容机制使用equals方法来判断是否包含某个元素随机增删元素效率较低,需要移动元素,时间复杂度为O(n)LinkedList底层数据结构是双向链表add(Ee)和remove()方法获取元素需要遍历节点,效率较低,时间复杂度为O(n)随机增删元
- python 内存空间管理、垃圾回收机制、对象的引用机制、引用计数法
贵哥的编程之路(热爱分享 为后来者)
开发语言python
一、对象与内存空间在Python中,一切皆对象。每当你创建一个变量、数据结构、函数、类实例等,Python都会在内存中为它分配空间。对象的内存空间由Python的内存管理器自动分配和回收,开发者无需手动管理。二、垃圾回收(GarbageCollection)垃圾回收指的是:当对象不再被使用时,Python会自动销毁该对象并释放其占用的内存空间。这样可以防止“内存泄漏”,让程序长期运行也不会因为无用
- 【学习】《算法图解》第七章学习笔记:树
自学也学好编程
程序人生
前言在前面的章节中,我们学习了数组、链表、散列表等基本数据结构,以及一些基础算法。本章将介绍一种非常重要的数据结构——树(Tree),特别是二叉搜索树(BinarySearchTree)。树结构在计算机科学中应用广泛,从文件系统到数据库再到人工智能,都能看到树的身影。《算法图解》第七章深入浅出地介绍了树的基本概念、实现和应用,帮助读者理解这一关键数据结构。一、树的基本概念(一)什么是树树是一种分层
- Python性能优化指南:让你的代码提速10倍的实用技巧
天天进步2015
pythonpython
Python以其简洁易用著称,但在性能方面常被诟病。其实,通过一些实用的优化技巧,你的Python代码性能完全可以提升数倍甚至十倍。本文将结合实际经验,系统介绍Python性能优化的常见思路与方法,并给出具体案例,助你写出高效的Python程序。1.算法与数据结构优化优先选择合适的数据结构:如查找用set/dict,顺序存储用list。避免不必要的嵌套循环,能用集合操作、字典映射解决的,绝不用暴力
- 虚幻引擎编程反射系统实现
污领巾
虚幻php游戏引擎
提示:文章写完后,目录可以自动生成,如何生成可参考右边的帮助文档文章目录前言1、反射的核心实现流程1.1宏定义标记1.2UnrealHeaderTool(UHT)处理1.3生成的代码结构1.4运行时反射数据注册2、反射系统的关键数据结构2.1UClass2.2UProperty及其派生类2.3UFunction3、反射的实际应用场景3.1蓝图与C++交互3.2序列化与反序列化3.3网络同步(Rep
- C# 索引器(Indexer)
C#索引器(Indexer)引言在C#编程语言中,索引器(Indexer)是一种特殊类型的属性,它允许我们通过索引来访问和设置对象的成员。索引器是动态数组和集合的基石,同时也可以用于创建自定义的数据结构,如字典等。本文将深入探讨C#索引器的概念、实现方式以及在实际开发中的应用。索引器的基本概念索引器是一种属性,它允许通过索引来访问和设置对象的成员。与普通的属性相比,索引器可以接受一个或多个参数,从
- AI表格数据分析
简单发一篇文章,最近看到AI数据分析是越来越火了哈,把简单的流程进行一次简要的分享。AI数据分析的本质,是“结构化数据→模式识别→可视化表达+洞察输出”。1、分析流程详解:(1)数据预处理什么是数据预处理呢?其实它可以理解成你给的是什么。步骤1:识别数据结构表头,字段的含义等。步骤2:清洗数据去除空值、格式错误、重复数据。步骤3:类型识别判断哪些是时间字段?哪些是数值型?哪些是分类字段?总结:类似
- 力扣 Hot 100 刷题记录 - LRU 缓存
a李兆洋
leetcode缓存算法
力扣Hot100刷题记录-LRU缓存题目描述LRU缓存是力扣Hot100中的一道经典题目,题目要求如下:请你设计并实现一个满足LRU(最近最少使用)缓存约束的数据结构。实现LRUCache类:LRUCache(intcapacity):以正整数作为容量capacity初始化LRU缓存。intget(intkey):如果关键字key存在于缓存中,则返回关键字的值,否则返回-1。voidput(int
- Java 中的LinkedList特点
liangblog
Java生产环境Java进阶java开发语言
在Java中,LinkedList是java.util包中的一个类,它实现了双向链表(DoublyLinkedList)数据结构。LinkedList不仅可以作为普通的列表使用,还支持高效的插入和删除操作,非常适合用于需要频繁增删元素的场景。一、JavaLinkedList的基本特点特性描述数据结构双向链表实现接口List,Deque索引访问支持,但效率较低(O(n))增删操作在头尾或中间插入/删
- Java LinkedList 详解
飞滕人生TYF
java算法数据结构javaLinkedList
在Java中,LinkedList是一个双向链表的实现,它是List接口的一个具体实现类,位于java.util包中。与其他常见的集合类(如ArrayList)不同,LinkedList基于链表数据结构,因此在元素的插入和删除操作上具有一定的优势。以下是对LinkedList的详细解析:1.LinkedList的定义和实现LinkedList是一个有序的集合,允许重复元素,并且实现了List接口以
- 多线程编程之理财
周凡杨
java多线程生产者消费者理财
现实生活中,我们一边工作,一边消费,正常情况下会把多余的钱存起来,比如存到余额宝,还可以多挣点钱,现在就有这个情况:我每月可以发工资20000万元 (暂定每月的1号),每月消费5000(租房+生活费)元(暂定每月的1号),其中租金是大头占90%,交房租的方式可以选择(一月一交,两月一交、三月一交),理财:1万元存余额宝一天可以赚1元钱,
- [Zookeeper学习笔记之三]Zookeeper会话超时机制
bit1129
zookeeper
首先,会话超时是由Zookeeper服务端通知客户端会话已经超时,客户端不能自行决定会话已经超时,不过客户端可以通过调用Zookeeper.close()主动的发起会话结束请求,如下的代码输出内容
Created /zoo-739160015
CONNECTEDCONNECTED
.............CONNECTEDCONNECTED
CONNECTEDCLOSEDCLOSED
- SecureCRT快捷键
daizj
secureCRT快捷键
ctrl + a : 移动光标到行首ctrl + e :移动光标到行尾crtl + b: 光标前移1个字符crtl + f: 光标后移1个字符crtl + h : 删除光标之前的一个字符ctrl + d :删除光标之后的一个字符crtl + k :删除光标到行尾所有字符crtl + u : 删除光标至行首所有字符crtl + w: 删除光标至行首
- Java 子类与父类这间的转换
周凡杨
java 父类与子类的转换
最近同事调的一个服务报错,查看后是日期之间转换出的问题。代码里是把 java.sql.Date 类型的对象 强制转换为 java.sql.Timestamp 类型的对象。报java.lang.ClassCastException。
代码:
- 可视化swing界面编辑
朱辉辉33
eclipseswing
今天发现了一个WindowBuilder插件,功能好强大,啊哈哈,从此告别手动编辑swing界面代码,直接像VB那样编辑界面,代码会自动生成。
首先在Eclipse中点击help,选择Install New Software,然后在Work with中输入WindowBui
- web报表工具FineReport常用函数的用法总结(文本函数)
老A不折腾
finereportweb报表工具报表软件java报表
文本函数
CHAR
CHAR(number):根据指定数字返回对应的字符。CHAR函数可将计算机其他类型的数字代码转换为字符。
Number:用于指定字符的数字,介于1Number:用于指定字符的数字,介于165535之间(包括1和65535)。
示例:
CHAR(88)等于“X”。
CHAR(45)等于“-”。
CODE
CODE(text):计算文本串中第一个字
- mysql安装出错
林鹤霄
mysql安装
[root@localhost ~]# rpm -ivh MySQL-server-5.5.24-1.linux2.6.x86_64.rpm Preparing... #####################
- linux下编译libuv
aigo
libuv
下载最新版本的libuv源码,解压后执行:
./autogen.sh
这时会提醒找不到automake命令,通过一下命令执行安装(redhat系用yum,Debian系用apt-get):
# yum -y install automake
# yum -y install libtool
如果提示错误:make: *** No targe
- 中国行政区数据及三级联动菜单
alxw4616
近期做项目需要三级联动菜单,上网查了半天竟然没有发现一个能直接用的!
呵呵,都要自己填数据....我了个去这东西麻烦就麻烦的数据上.
哎,自己没办法动手写吧.
现将这些数据共享出了,以方便大家.嗯,代码也可以直接使用
文件说明
lib\area.sql -- 县及县以上行政区划分代码(截止2013年8月31日)来源:国家统计局 发布时间:2014-01-17 15:0
- 哈夫曼加密文件
百合不是茶
哈夫曼压缩哈夫曼加密二叉树
在上一篇介绍过哈夫曼编码的基础知识,下面就直接介绍使用哈夫曼编码怎么来做文件加密或者压缩与解压的软件,对于新手来是有点难度的,主要还是要理清楚步骤;
加密步骤:
1,统计文件中字节出现的次数,作为权值
2,创建节点和哈夫曼树
3,得到每个子节点01串
4,使用哈夫曼编码表示每个字节
- JDK1.5 Cyclicbarrier实例
bijian1013
javathreadjava多线程Cyclicbarrier
CyclicBarrier类
一个同步辅助类,它允许一组线程互相等待,直到到达某个公共屏障点 (common barrier point)。在涉及一组固定大小的线程的程序中,这些线程必须不时地互相等待,此时 CyclicBarrier 很有用。因为该 barrier 在释放等待线程后可以重用,所以称它为循环的 barrier。
CyclicBarrier支持一个可选的 Runnable 命令,
- 九项重要的职业规划
bijian1013
工作学习
一. 学习的步伐不停止 古人说,活到老,学到老。终身学习应该是您的座右铭。 世界在不断变化,每个人都在寻找各自的事业途径。 您只有保证了足够的技能储
- 【Java范型四】范型方法
bit1129
java
范型参数不仅仅可以用于类型的声明上,例如
package com.tom.lang.generics;
import java.util.List;
public class Generics<T> {
private T value;
public Generics(T value) {
this.value =
- 【Hadoop十三】HDFS Java API基本操作
bit1129
hadoop
package com.examples.hadoop;
import org.apache.hadoop.conf.Configuration;
import org.apache.hadoop.fs.FSDataInputStream;
import org.apache.hadoop.fs.FileStatus;
import org.apache.hadoo
- ua实现split字符串分隔
ronin47
lua split
LUA并不象其它许多"大而全"的语言那样,包括很多功能,比如网络通讯、图形界面等。但是LUA可以很容易地被扩展:由宿主语言(通常是C或 C++)提供这些功能,LUA可以使用它们,就像是本来就内置的功能一样。LUA只包括一个精简的核心和最基本的库。这使得LUA体积小、启动速度快,从 而适合嵌入在别的程序里。因此在lua中并没有其他语言那样多的系统函数。习惯了其他语言的字符串分割函
- java-从先序遍历和中序遍历重建二叉树
bylijinnan
java
public class BuildTreePreOrderInOrder {
/**
* Build Binary Tree from PreOrder and InOrder
* _______7______
/ \
__10__ ___2
/ \ /
4
- openfire开发指南《连接和登陆》
开窍的石头
openfire开发指南smack
第一步
官网下载smack.jar包
下载地址:http://www.igniterealtime.org/downloads/index.jsp#smack
第二步
把smack里边的jar导入你新建的java项目中
开始编写smack连接openfire代码
p
- [移动通讯]手机后盖应该按需要能够随时开启
comsci
移动
看到新的手机,很多由金属材质做的外壳,内存和闪存容量越来越大,CPU速度越来越快,对于这些改进,我们非常高兴,也非常欢迎
但是,对于手机的新设计,有几点我们也要注意
第一:手机的后盖应该能够被用户自行取下来,手机的电池的可更换性应该是必须保留的设计,
- 20款国外知名的php开源cms系统
cuiyadll
cms
内容管理系统,简称CMS,是一种简易的发布和管理新闻的程序。用户可以在后端管理系统中发布,编辑和删除文章,即使您不需要懂得HTML和其他脚本语言,这就是CMS的优点。
在这里我决定介绍20款目前国外市面上最流行的开源的PHP内容管理系统,以便没有PHP知识的读者也可以通过国外内容管理系统建立自己的网站。
1. Wordpress
WordPress的是一个功能强大且易于使用的内容管
- Java生成全局唯一标识符
darrenzhu
javauuiduniqueidentifierid
How to generate a globally unique identifier in Java
http://stackoverflow.com/questions/21536572/generate-unique-id-in-java-to-label-groups-of-related-entries-in-a-log
http://stackoverflow
- php安装模块检测是否已安装过, 使用的SQL语句
dcj3sjt126com
sql
SHOW [FULL] TABLES [FROM db_name] [LIKE 'pattern']
SHOW TABLES列举了给定数据库中的非TEMPORARY表。您也可以使用mysqlshow db_name命令得到此清单。
本命令也列举数据库中的其它视图。支持FULL修改符,这样SHOW FULL TABLES就可以显示第二个输出列。对于一个表,第二列的值为BASE T
- 5天学会一种 web 开发框架
dcj3sjt126com
Web框架framework
web framework层出不穷,特别是ruby/python,各有10+个,php/java也是一大堆 根据我自己的经验写了一个to do list,按照这个清单,一条一条的学习,事半功倍,很快就能掌握 一共25条,即便很磨蹭,2小时也能搞定一条,25*2=50。只需要50小时就能掌握任意一种web框架
各类web框架大同小异:现代web开发框架的6大元素,把握主线,就不会迷路
建议把本文
- Gson使用三(Map集合的处理,一对多处理)
eksliang
jsongsonGson mapGson 集合处理
转载请出自出处:http://eksliang.iteye.com/blog/2175532 一、概述
Map保存的是键值对的形式,Json的格式也是键值对的,所以正常情况下,map跟json之间的转换应当是理所当然的事情。 二、Map参考实例
package com.ickes.json;
import java.lang.refl
- cordova实现“再点击一次退出”效果
gundumw100
android
基本的写法如下:
document.addEventListener("deviceready", onDeviceReady, false);
function onDeviceReady() {
//navigator.splashscreen.hide();
document.addEventListener("b
- openldap configuration leaning note
iwindyforest
configuration
hostname // to display the computer name
hostname <changed name> // to change
go to: /etc/sysconfig/network, add/modify HOSTNAME=NEWNAME to change permenately
dont forget to change /etc/hosts
- Nullability and Objective-C
啸笑天
Objective-C
https://developer.apple.com/swift/blog/?id=25
http://www.cocoachina.com/ios/20150601/11989.html
http://blog.csdn.net/zhangao0086/article/details/44409913
http://blog.sunnyxx
- jsp中实现参数隐藏的两种方法
macroli
JavaScriptjsp
在一个JSP页面有一个链接,//确定是一个链接?点击弹出一个页面,需要传给这个页面一些参数。//正常的方法是设置弹出页面的src="***.do?p1=aaa&p2=bbb&p3=ccc"//确定目标URL是Action来处理?但是这样会在页面上看到传过来的参数,可能会不安全。要求实现src="***.do",参数通过其他方法传!//////
- Bootstrap A标签关闭modal并打开新的链接解决方案
qiaolevip
每天进步一点点学习永无止境bootstrap纵观千象
Bootstrap里面的js modal控件使用起来很方便,关闭也很简单。只需添加标签 data-dismiss="modal" 即可。
可是偏偏有时候需要a标签既要关闭modal,有要打开新的链接,尝试多种方法未果。只好使用原始js来控制。
<a href="#/group-buy" class="btn bt
- 二维数组在Java和C中的区别
流淚的芥末
javac二维数组数组
Java代码:
public class test03 {
public static void main(String[] args) {
int[][] a = {{1},{2,3},{4,5,6}};
System.out.println(a[0][1]);
}
}
运行结果:
Exception in thread "mai
- systemctl命令用法
wmlJava
linuxsystemctl
对比表,以 apache / httpd 为例 任务 旧指令 新指令 使某服务自动启动 chkconfig --level 3 httpd on systemctl enable httpd.service 使某服务不自动启动 chkconfig --level 3 httpd off systemctl disable httpd.service 检查服务状态 service h