Leetcode_入门_回溯

Backtracking（回溯）

1、电话号码的字母组合（17、Medium）

1）题目要求

2）我的解法

class Solution {
    List<String> result;
    Map<Integer,String> map;
    char[] temp;
    public void backtrack(int i,String digits){
        if(i>=digits.length())return;
        String s=map.get(digits.charAt(i)-'0');
        for(int j=0;j<s.length();j++){
            temp[i]=s.charAt(j);
            if(i==digits.length()-1)result.add(new String(temp));
            backtrack(i+1,digits);
        }
    }
    public List<String> letterCombinations(String digits) {
        map=new HashMap<>();
        result=new ArrayList<>();
        temp=new char[digits.length()];
        map.put(2,"abc");map.put(3,"def");map.put(4,"ghi");
        map.put(5,"jkl");map.put(6,"mno");map.put(7,"pqrs");
        map.put(8,"tuv");map.put(9,"wxyz");
        backtrack(0,digits);
        return result;
    }
}

3）其他解法

1、回溯

class Solution {
	//一个映射表，第二个位置是"abc“,第三个位置是"def"。。。
	//这里也可以用map，用数组可以更节省点内存
	String[] letter_map = {" ","*","abc","def","ghi","jkl","mno","pqrs","tuv","wxyz"};
	public List<String> letterCombinations(String digits) {
		//注意边界条件
		if(digits==null || digits.length()==0) {
			return new ArrayList<>();
		}
		iterStr(digits, new StringBuilder(), 0);
		return res;
	}
	//最终输出结果的list
	List<String> res = new ArrayList<>();
	
	//递归函数
	void iterStr(String str, StringBuilder letter, int index) {
		//递归的终止条件，注意这里的终止条件看上去跟动态演示图有些不同，主要是做了点优化
		//动态图中是每次截取字符串的一部分，"234"，变成"23"，再变成"3"，最后变成""，这样性能不佳
		//而用index记录每次遍历到字符串的位置，这样性能更好
		if(index == str.length()) {
			res.add(letter.toString());
			return;
		}
		//获取index位置的字符，假设输入的字符是"234"
		//第一次递归时index为0所以c=2，第二次index为1所以c=3，第三次c=4
		//subString每次都会生成新的字符串，而index则是取当前的一个字符，所以效率更高一点
		char c = str.charAt(index);
		//map_string的下表是从0开始一直到9， c-'0'就可以取到相对的数组下标位置
		//比如c=2时候，2-'0'，获取下标为2,letter_map[2]就是"abc"
		int pos = c - '0';
		String map_string = letter_map[pos];
		//遍历字符串，比如第一次得到的是2，页就是遍历"abc"
		for(int i=0;i<map_string.length();i++) {
			//调用下一层递归，用文字很难描述，请配合动态图理解
            letter.append(map_string.charAt(i));
            //如果是String类型做拼接效率会比较低
			//iterStr(str, letter+map_string.charAt(i), index+1);
            iterStr(str, letter, index+1);
            letter.deleteCharAt(letter.length()-1);
		}
	}
}

2、

class Solution {
	public List<String> letterCombinations(String digits) {
		if(digits==null || digits.length()==0) {
			return new ArrayList<String>();
		}
		//一个映射表，第二个位置是"abc“,第三个位置是"def"。。。
		//这里也可以用map，用数组可以更节省点内存
		String[] letter_map = {
			" ","*","abc","def","ghi","jkl","mno","pqrs","tuv","wxyz"
		};
		List<String> res = new ArrayList<>();
		//先往队列中加入一个空字符
		res.add("");
		for(int i=0;i<digits.length();i++) {
			//由当前遍历到的字符，取字典表中查找对应的字符串
			String letters = letter_map[digits.charAt(i)-'0'];
			int size = res.size();
			//计算出队列长度后，将队列中的每个元素挨个拿出来
			for(int j=0;j<size;j++) {
				//每次都从队列中拿出第一个元素
				String tmp = res.remove(0);
				//然后跟"def"这样的字符串拼接，并再次放到队列中
				for(int k=0;k<letters.length();k++) {
					res.add(tmp+letters.charAt(k));
				}
			}
		}
		return res;
	}
}

作者：wang_ni_ma
链接： link
来源：力扣（LeetCode）

4）自己的优化代码

class Solution {
    List<String> result;
    Map<Integer,String> map;
    char[] temp;
    public void backtrack(int i,String digits){
        if(i>=digits.length())return;
        String s=map.get(digits.charAt(i)-'0');
        for(int j=0;j<s.length();j++){
            temp[i]=s.charAt(j);
            if(i==digits.length()-1)result.add(new String(temp));
            backtrack(i+1,digits);
        }
    }
    public List<String> letterCombinations(String digits) {
        map=new HashMap<>();
        result=new ArrayList<>();
        temp=new char[digits.length()];
        map.put(2,"abc");map.put(3,"def");map.put(4,"ghi");
        map.put(5,"jkl");map.put(6,"mno");map.put(7,"pqrs");
        map.put(8,"tuv");map.put(9,"wxyz");
        backtrack(0,digits);
        return result;
    }
}

5）学到的东西

当题目中出现 “所有组合” 等类似字眼时，我们第一感觉就要想到用回溯。

StringBuilder的API：append(), deleteCharAt()

2、 复原IP地址（93、Medium）

1）题目要求

给定一个只包含数字的字符串，复原它并返回所有可能的 IP 地址格式。

有效的 IP 地址 正好由四个整数（每个整数位于 0 到 255 之间组成，且不能含有前导 0），整数之间用 ‘.’ 分隔。

例如：“0.1.2.201” 和 “192.168.1.1” 是 有效的 IP 地址，但是 “0.011.255.245”、“192.168.1.312” 和 “[email protected]” 是 无效的 IP 地址。

示例 1：

输入：s = “25525511135”
输出：[“255.255.11.135”,“255.255.111.35”]
示例 2：

输入：s = “0000”
输出：[“0.0.0.0”]
示例 3：

输入：s = “1111”
输出：[“1.1.1.1”]
示例 4：

输入：s = “010010”
输出：[“0.10.0.10”,“0.100.1.0”]
示例 5：

输入：s = “101023”
输出：[“1.0.10.23”,“1.0.102.3”,“10.1.0.23”,“10.10.2.3”,“101.0.2.3”]

2）我的解法

class Solution {
    List<String> result;
    int cur=0;
    public void backtrack(int i,int num,StringBuilder letter,String s){//num代表当前是第几个数
        double remainLength=s.length()-i;//字符串剩余字符数
        double remainNum=4-num+1;//剩余数字个数
        if(remainLength/remainNum>3||remainLength/remainNum<1)return;//剩余的数符合要求
        for(int j=1;j<=Math.min(3,remainLength);j++){
            //1-3个数依次尝试
            cur=0;
            if(num==4){//最后一个数就不用试了
                for(int k=0;k<remainLength;k++){letter.append(s.charAt(i+k));cur=cur*10+(s.charAt(i+k)-'0');}
                if(!(cur>255||(remainLength==2&&cur<10)||(remainLength==3&&cur<100)))result.add(letter.toString());
                for(int k=0;k<remainLength;k++)letter.deleteCharAt(letter.length()-1);
                return ;
            }
                for(int k=0;k<j;k++){letter.append(s.charAt(i+k));cur=cur*10+(s.charAt(i+k)-'0');}
                if(cur>255||(j==2&&cur<10)||(j==3&&cur<100)){//处理数据不合规范的情况（前缀有0或大于255）
                    for(int k=0;k<j;k++)letter.deleteCharAt(letter.length()-1);
                    return ;
                }
                letter.append(".");
                backtrack(i+j,num+1,letter,s);
                for(int k=0;k<j+1;k++)letter.deleteCharAt(letter.length()-1);
        }
    }
    public List<String> restoreIpAddresses(String s) {
        result=new ArrayList<>();
        if(s.length()>12||s.length()<4)return result;
        backtrack(0,1,new StringBuilder(),s);
        return result;
    }
}

3）其他解法

    public List<String> restoreIpAddresses(String s) {
        List<String> ret = new ArrayList<>();

        StringBuilder ip = new StringBuilder();
        
        for(int a = 1 ; a < 4 ; ++ a)
            for(int b = 1 ; b < 4 ; ++ b)
                for(int c = 1 ; c < 4 ; ++ c)
                    for(int d = 1 ; d < 4 ; ++ d)
                    {
                        if(a + b + c + d == s.length() )
                        {
                            int n1 = Integer.parseInt(s.substring(0, a));
                            int n2 = Integer.parseInt(s.substring(a, a+b));
                            int n3 = Integer.parseInt(s.substring(a+b, a+b+c));
                            int n4 = Integer.parseInt(s.substring(a+b+c));
                            if(n1 <= 255 && n2 <= 255 && n3 <= 255 && n4 <= 255)
                            {
                                ip.append(n1).append('.').append(n2)
                                        .append('.').append(n3).append('.').append(n4);
                                if(ip.length() == s.length() + 3) ret.add(ip.toString());
                                ip.delete(0, ip.length());
                            }
                        }
                    }
        return ret;
    }

作者：reals
链接：link
来源：力扣（LeetCode）

import java.util.ArrayDeque;
import java.util.ArrayList;
import java.util.Deque;
import java.util.List;

public class Solution {

    public List<String> restoreIpAddresses(String s) {
        int len = s.length();
        List<String> res = new ArrayList<>();
        if (len > 12 || len < 4) {
            return res;
        }

        Deque<String> path = new ArrayDeque<>(4);
        dfs(s, len, 0, 4, path, res);
        return res;
    }

    // 需要一个变量记录剩余多少段还没被分割

    private void dfs(String s, int len, int begin, int residue, Deque<String> path, List<String> res) {
        if (begin == len) {
            if (residue == 0) {
                res.add(String.join(".", path));
            }
            return;
        }

        for (int i = begin; i < begin + 3; i++) {
            if (i >= len) {
                break;
            }

            if (residue * 3 < len - i) {
                continue;
            }

            if (judgeIpSegment(s, begin, i)) {
                String currentIpSegment = s.substring(begin, i + 1);
                path.addLast(currentIpSegment);

                dfs(s, len, i + 1, residue - 1, path, res);
                path.removeLast();
            }
        }
    }

    private boolean judgeIpSegment(String s, int left, int right) {
        int len = right - left + 1;
        if (len > 1 && s.charAt(left) == '0') {
            return false;
        }

        int res = 0;
        while (left <= right) {
            res = res * 10 + s.charAt(left) - '0';
            left++;
        }

        return res >= 0 && res <= 255;
    }
}

作者：liweiwei1419
链接：link
来源：力扣（LeetCode）

4）自己的优化代码

class Solution {
    List<String> result;
    int cur=0;
    public void backtrack(int i,int num,StringBuilder letter,String s){//num代表当前是第几个数
        double remainLength=s.length()-i;//字符串剩余字符数
        double remainNum=4-num+1;//剩余数字个数
        if(remainLength/remainNum>3||remainLength/remainNum<1)return;//剩余的数符不合要求
        for(int j=1;j<=Math.min(3,remainLength);j++){
            //1-3个数依次尝试
            cur=0;
            if(num==4){//最后一个数就不用试了
                for(int k=0;k<remainLength;k++){letter.append(s.charAt(i+k));cur=cur*10+(s.charAt(i+k)-'0');}
                if(!(cur>255||(remainLength==2&&cur<10)||(remainLength==3&&cur<100)))result.add(letter.toString());
                for(int k=0;k<remainLength;k++)letter.deleteCharAt(letter.length()-1);//细节：一定记得删
                return ;
            }
                for(int k=0;k<j;k++){letter.append(s.charAt(i+k));cur=cur*10+(s.charAt(i+k)-'0');}
                if(cur>255||(j==2&&cur<10)||(j==3&&cur<100)){//处理数据不合规范的情况（前缀有0或大于255）
                    for(int k=0;k<j;k++)letter.deleteCharAt(letter.length()-1);
                    return ;
                }
                letter.append(".");
                backtrack(i+j,num+1,letter,s);
                for(int k=0;k<j+1;k++)letter.deleteCharAt(letter.length()-1);
        }
    }
    public List<String> restoreIpAddresses(String s) {
        result=new ArrayList<>();
        if(s.length()>12||s.length()<4)return result;
        backtrack(0,1,new StringBuilder(),s);
        return result;
    }
}

5）学到的东西

回溯思想

先画出树，明确递归截至条件（一定一定要先确定好，比如本题中第四个数特殊处理）、什么时候截枝，再写

不管什么情况，在递归结束返回时（即回溯时）一定要记得在把当前结点再次标记为未访问（本题中即把它从letter中删掉）

3、单词搜索（79、Medium）

1）题目要求

给定一个二维网格和一个单词，找出该单词是否存在于网格中。

单词必须按照字母顺序，通过相邻的单元格内的字母构成，其中“相邻”单元格是那些水平相邻或垂直相邻的单元格。同一个单元格内的字母不允许被重复使用。

示例:

board =
[
[‘A’,‘B’,‘C’,‘E’],
[‘S’,‘F’,‘C’,‘S’],
[‘A’,‘D’,‘E’,‘E’]
]

给定 word = “ABCCED”, 返回 true
给定 word = “SEE”, 返回 true
给定 word = “ABCB”, 返回 false

提示：

board 和 word 中只包含大写和小写英文字母。
1 <= board.length <= 200
1 <= board[i].length <= 200
1 <= word.length <= 10^3

2）我的解法

class Solution {
    boolean[][] visit;
    public boolean dfs(int i,int j,int num,char[][] board, String word){
        if(i<0||i>=board.length||j<0||j>=board[0].length)return false;
        if(board[i][j]!=word.charAt(num))return false;
        if(visit[i][j])return false;
        if(num==word.length()-1)return true;
        visit[i][j]=true;
        boolean tag=dfs(i-1,j,num+1,board,word)||dfs(i+1,j,num+1,board,word)||dfs(i,j-1,num+1,board,word)||dfs(i,j+1,num+1,board,word);
        visit[i][j]=false;
        return tag;
        
    }
    public boolean exist(char[][] board, String word) {
        visit=new boolean[board.length][board[0].length];
        for(int i=0;i<board.length;i++){
            for(int j=0;j<board[0].length;j++){
                if(board[i][j]==word.charAt(0)){
                    if(dfs(i,j,0,board,word))return true;
                }
            }
        }
        return false;
    }
}

3）其他解法

class Solution {
    public boolean exist(char[][] board, String word) {
        int h = board.length, w = board[0].length;
        boolean[][] visited = new boolean[h][w];
        for (int i = 0; i < h; i++) {
            for (int j = 0; j < w; j++) {
                boolean flag = check(board, visited, i, j, word, 0);
                if (flag) {
                    return true;
                }
            }
        }
        return false;
    }

    public boolean check(char[][] board, boolean[][] visited, int i, int j, String s, int k) {
        if (board[i][j] != s.charAt(k)) {
            return false;
        } else if (k == s.length() - 1) {
            return true;
        }
        visited[i][j] = true;
        int[][] directions = {{0, 1}, {0, -1}, {1, 0}, {-1, 0}};
        boolean result = false;
        for (int[] dir : directions) {
            int newi = i + dir[0], newj = j + dir[1];
            if (newi >= 0 && newi < board.length && newj >= 0 && newj < board[0].length) {
                if (!visited[newi][newj]) {
                    boolean flag = check(board, visited, newi, newj, s, k + 1);
                    if (flag) {
                        result = true;
                        break;
                    }
                }
            }
        }
        visited[i][j] = false;
        return result;
    }
}

作者：LeetCode-Solution
链接：link
来源：力扣（LeetCode）

4）自己的优化代码

class Solution {
    public boolean dfs(int i,int j,int num,char[][] board, String word){
        if(i<0||i>=board.length||j<0||j>=board[0].length)return false;
        if(board[i][j]!=word.charAt(num))return false;
        if(num==word.length()-1)return true;
        board[i][j]='0';
        boolean tag=dfs(i-1,j,num+1,board,word)||dfs(i+1,j,num+1,board,word)||dfs(i,j-1,num+1,board,word)||dfs(i,j+1,num+1,board,word);
        board[i][j]=word.charAt(num);
        return tag;
        
    }
    public boolean exist(char[][] board, String word) {
        for(int i=0;i<board.length;i++){
            for(int j=0;j<board[0].length;j++){
                if(board[i][j]==word.charAt(0)){
                    if(dfs(i,j,0,board,word))return true;
                }
            }
        }
        return false;
    }
}

5）学到的东西

回溯思想
递归返回（回溯）时，一定记得把当前结点重新置为未访问

4、 二叉树的所有路径（257、Easy）

1）题目要求

给定一个二叉树，返回所有从根节点到叶子节点的路径。

说明: 叶子节点是指没有子节点的节点。

示例:

输入:

1
/
2 3

5

输出: [“1->2->5”, “1->3”]

解释: 所有根节点到叶子节点的路径为: 1->2->5, 1->3

2）我的解法

/**
 * Definition for a binary tree node.
 * public class TreeNode {
 *     int val;
 *     TreeNode left;
 *     TreeNode right;
 *     TreeNode(int x) { val = x; }
 * }
 */
class Solution {
    List<String> result;
    StringBuilder s=new StringBuilder();
    public void backtrack(TreeNode root){
        if(root==null)return ;
        if(root.left==null&&root.right==null){
            s.append(root.val);
            result.add(s.toString());
            for(int i=0;i<getWei(root.val);i++)s.deleteCharAt(s.length()-1);
            return;
        }
        s.append(root.val);
        s.append("->");
        backtrack(root.left);
        for(int i=0;i<getWei(root.val);i++)s.deleteCharAt(s.length()-1);
        s.deleteCharAt(s.length()-1);s.deleteCharAt(s.length()-1);

        s.append(root.val);
        s.append("->");
        backtrack(root.right);
        for(int i=0;i<getWei(root.val);i++)s.deleteCharAt(s.length()-1);
        s.deleteCharAt(s.length()-1);s.deleteCharAt(s.length()-1);
    }
    public int getWei(int num){
        int re=1;
        if(num<0)re++;
        if(num/10!=0)re++;
        if(num/100!=0)re++;
        return re;
    }
    public List<String> binaryTreePaths(TreeNode root) {
        result=new ArrayList<>();
        backtrack(root);
        return result;
    }
}

3）其他解法

class Solution {
    public List<String> binaryTreePaths(TreeNode root) {
        List<String> paths = new ArrayList<String>();
        constructPaths(root, "", paths);
        return paths;
    }

    public void constructPaths(TreeNode root, String path, List<String> paths) {
        if (root != null) {
            StringBuffer pathSB = new StringBuffer(path);
            pathSB.append(Integer.toString(root.val));
            if (root.left == null && root.right == null) {  // 当前节点是叶子节点
                paths.add(pathSB.toString());  // 把路径加入到答案中
            } else {
                pathSB.append("->");  // 当前节点不是叶子节点，继续递归遍历
                constructPaths(root.left, pathSB.toString(), paths);
                constructPaths(root.right, pathSB.toString(), paths);
            }
        }
    }
}

class Solution {
    public List<String> binaryTreePaths(TreeNode root) {
        List<String> paths = new ArrayList<String>();
        if (root == null) {
            return paths;
        }
        Queue<TreeNode> nodeQueue = new LinkedList<TreeNode>();
        Queue<String> pathQueue = new LinkedList<String>();

        nodeQueue.offer(root);
        pathQueue.offer(Integer.toString(root.val));

        while (!nodeQueue.isEmpty()) {
            TreeNode node = nodeQueue.poll(); 
            String path = pathQueue.poll();

            if (node.left == null && node.right == null) {
                paths.add(path);
            } else {
                if (node.left != null) {
                    nodeQueue.offer(node.left);
                    pathQueue.offer(new StringBuffer(path).append("->").append(node.left.val).toString());
                }

                if (node.right != null) {
                    nodeQueue.offer(node.right);
                    pathQueue.offer(new StringBuffer(path).append("->").append(node.right.val).toString());
                }
            }
        }
        return paths;
    }
}

作者：LeetCode-Solution
链接：link
来源：力扣（LeetCode）

4）自己的优化代码

/**
 * Definition for a binary tree node.
 * public class TreeNode {
 *     int val;
 *     TreeNode left;
 *     TreeNode right;
 *     TreeNode(int x) { val = x; }
 * }
 */
class Solution {
    List<String> result;
    public void backtrack(TreeNode root,String path){
        if(root==null)return ;
        StringBuilder s=new StringBuilder(path);
        if(root.left==null&&root.right==null){
            s.append(root.val);
            result.add(s.toString());
            return;
        }
        s.append(root.val);
        s.append("->");
        backtrack(root.left,s.toString());
        backtrack(root.right,s.toString());
    }
    public List<String> binaryTreePaths(TreeNode root) {
        result=new ArrayList<>();
        backtrack(root,"");
        return result;
    }
}

5）学到的东西

回溯思想

把备忘录作为backpack函数的形参，这样每次回溯时不必更新备忘录（不必把当前结点重新设为未访问）

5、 全排列（46、Medium）

1）题目要求

给定一个 没有重复 数字的序列，返回其所有可能的全排列。

示例:

输入: [1,2,3]
输出:
[
[1,2,3],
[1,3,2],
[2,1,3],
[2,3,1],
[3,1,2],
[3,2,1]
]

2）我的解法

class Solution {
    List<List<Integer>> result;
    List<Integer> temp=new ArrayList<>();
    public void backpack(List<Integer> list){

        if(list.size()==1){
            temp.add(list.get(0));
            List<Integer> l=new ArrayList<>();
            for(int i=0;i<temp.size();i++)l.add(temp.get(i));
            result.add(l);
            temp.remove(temp.size()-1);
            return;
        }
        for(int i=0;i<list.size();i++){
            int tem=list.get(0);
            temp.add(tem);
            list.remove(0);
            backpack(list);
            temp.remove(temp.size()-1);
            list.add(tem);
        }
    }
    public List<List<Integer>> permute(int[] nums) {
        result=new ArrayList<>();
        List<Integer> list=new ArrayList<>();
        for(int i=0;i<nums.length;i++)list.add(nums[i]);
        backpack(list);
        return result;
    }
}

3）其他解法

class Solution {
    public List<List<Integer>> permute(int[] nums) {
        List<List<Integer>> res = new ArrayList<List<Integer>>();

        List<Integer> output = new ArrayList<Integer>();
        for (int num : nums) {
            output.add(num);
        }

        int n = nums.length;
        backtrack(n, output, res, 0);
        return res;
    }

    public void backtrack(int n, List<Integer> output, List<List<Integer>> res, int first) {
        // 所有数都填完了
        if (first == n) {
            res.add(new ArrayList<Integer>(output));
        }
        for (int i = first; i < n; i++) {
            // 动态维护数组
            Collections.swap(output, first, i);
            // 继续递归填下一个数
            backtrack(n, output, res, first + 1);
            // 撤销操作
            Collections.swap(output, first, i);
        }
    }
}

作者：LeetCode-Solution
链接：link
来源：力扣（LeetCode）

4）自己的优化代码

class Solution {
    List<List<Integer>> result;
    List<Integer> temp=new ArrayList<>();
    public void backpack(List<Integer> list){

        if(list.size()==1){
            temp.add(list.get(0));
            List<Integer> l=new ArrayList<>(temp);
            result.add(l);
            temp.remove(temp.size()-1);
            return;
        }
        for(int i=0;i<list.size();i++){
            int tem=list.get(0);
            temp.add(tem);
            list.remove(0);
            backpack(list);
            temp.remove(temp.size()-1);
            list.add(tem);
        }
    }
    public List<List<Integer>> permute(int[] nums) {
        result=new ArrayList<>();
        List<Integer> list=new ArrayList<>();
        for(int i=0;i<nums.length;i++)list.add(nums[i]);
        backpack(list);
        return result;
    }
}

5）学到的东西

回溯思想

构造：new ArrayList<>(另一个arraylist)

Collections.swap():参考 java.util.Collections.swap()方法实例

6、全排列 II（47、Medium）

1）题目要求

给定一个可包含重复数字的序列，返回所有不重复的全排列。

示例:

输入: [1,1,2]
输出:
[
[1,1,2],
[1,2,1],
[2,1,1]
]

2）我的解法

class Solution {
    List<List<Integer>> result=new ArrayList<>();
    List<Integer> temp=new ArrayList<>();
    public void backpack(List<Integer> nums){
        if(nums.size()==0)return;
        if(nums.size()==1){
            temp.add(nums.get(0));
            result.add(new ArrayList<Integer>(temp));
            temp.remove(temp.size()-1);
            return;
        }
        for(int j=0;j<nums.size();j++){
            if(j>0&&nums.get(j)==nums.get(j-1))continue;
            temp.add(nums.get(j));
            nums.remove(j);
            backpack(nums);
            nums.add(j,temp.get(temp.size()-1));
            temp.remove(temp.size()-1);
        }
    }
    public List<List<Integer>> permuteUnique(int[] nums) {
        List<Integer> list=new ArrayList<>();
        Arrays.sort(nums);
        for(int i=0;i<nums.length;i++)list.add(nums[i]);
        backpack(list);
        return result;
    }
}

3）其他解法

import java.util.ArrayDeque;
import java.util.ArrayList;
import java.util.Arrays;
import java.util.Deque;
import java.util.List;

public class Solution {

    public List<List<Integer>> permuteUnique(int[] nums) {
        int len = nums.length;
        List<List<Integer>> res = new ArrayList<>();
        if (len == 0) {
            return res;
        }

        // 排序（升序或者降序都可以），排序是剪枝的前提
        Arrays.sort(nums);

        boolean[] used = new boolean[len];
        // 使用 Deque 是 Java 官方 Stack 类的建议
        Deque<Integer> path = new ArrayDeque<>(len);
        dfs(nums, len, 0, used, path, res);
        return res;
    }

    private void dfs(int[] nums, int len, int depth, boolean[] used, Deque<Integer> path, List<List<Integer>> res) {
        if (depth == len) {
            res.add(new ArrayList<>(path));
            return;
        }

        for (int i = 0; i < len; ++i) {
            if (used[i]) {
                continue;
            }

            // 剪枝条件：i > 0 是为了保证 nums[i - 1] 有意义
            // 写 !used[i - 1] 是因为 nums[i - 1] 在深度优先遍历的过程中刚刚被撤销选择
            if (i > 0 && nums[i] == nums[i - 1] && !used[i - 1]) {
                continue;
            }

            path.addLast(nums[i]);
            used[i] = true;

            dfs(nums, len, depth + 1, used, path, res);
            // 回溯部分的代码，和 dfs 之前的代码是对称的
            used[i] = false;
            path.removeLast();
        }
    }
}

作者：liweiwei1419
链接：link
来源：力扣（LeetCode）

4）自己的优化代码

class Solution {
    List<List<Integer>> result=new ArrayList<>();
    List<Integer> temp=new ArrayList<>();
    public void backpack(List<Integer> nums){
        if(nums.size()==0)return;
        if(nums.size()==1){
            temp.add(nums.get(0));
            result.add(new ArrayList<Integer>(temp));
            temp.remove(temp.size()-1);
            return;
        }
        for(int j=0;j<nums.size();j++){
            if(j>0&&nums.get(j)==nums.get(j-1))continue;
            temp.add(nums.get(j));
            nums.remove(j);
            backpack(nums);
            nums.add(j,temp.get(temp.size()-1));
            temp.remove(temp.size()-1);
        }
    }
    public List<List<Integer>> permuteUnique(int[] nums) {
        List<Integer> list=new ArrayList<>();
        Arrays.sort(nums);
        for(int i=0;i<nums.length;i++)list.add(nums[i]);
        backpack(list);
        return result;
    }
}

5）学到的东西

先画树再写

分析重复原因，进行剪枝

尽量把int[]换成list,方便增删

7、组合（77、Medium）

1）题目要求

给定两个整数 n 和 k，返回 1 … n 中所有可能的 k 个数的组合。

示例:

输入: n = 4, k = 2
输出:
[
[2,4],
[3,4],
[2,3],
[1,2],
[1,3],
[1,4],
]

2）我的解法

class Solution {
    List<Integer> temp=new ArrayList<>();
    int deep;
    int N;
    List<List<Integer>> result=new ArrayList<>();
    public void backpack(int start,int ceng){
        if(start>N)return;
        if(ceng==deep){
            for(int i=start;i<=N;i++){
                temp.add(i);
                result.add(new ArrayList<>(temp));
                temp.remove(temp.size()-1);
            }
            return;
        }
        for(int i=start;i<=N;i++){
            temp.add(i);
            backpack(i+1,ceng+1);
            temp.remove(temp.size()-1);
        }
    }
    public List<List<Integer>> combine(int n, int k) {
        deep=k;
        N=n;
        backpack(1,1);
        return result;
    }
}

3）其他解法

import java.util.ArrayDeque;
import java.util.ArrayList;
import java.util.Deque;
import java.util.List;

public class Solution {

    public List<List<Integer>> combine(int n, int k) {
        List<List<Integer>> res = new ArrayList<>();
        if (k <= 0 || n < k) {
            return res;
        }
        Deque<Integer> path = new ArrayDeque<>();
        dfs(n, k, 1, path, res);
        return res;
    }

    private void dfs(int n, int k, int index, Deque<Integer> path, List<List<Integer>> res) {
        if (path.size() == k) {
            res.add(new ArrayList<>(path));
            return;
        }

        // 只有这里 i <= n - (k - path.size()) + 1 与参考代码 1 不同
        for (int i = index; i <= n - (k - path.size()) + 1; i++) {
            path.addLast(i);
            dfs(n, k, i + 1, path, res);
            path.removeLast();
        }
    }
}

import java.util.ArrayDeque;
import java.util.ArrayList;
import java.util.Deque;
import java.util.List;

public class Solution {

    public List<List<Integer>> combine(int n, int k) {
        List<List<Integer>> res = new ArrayList<>();
        if (k <= 0 || n < k) {
            return res;
        }

        // 为了防止底层动态数组扩容，初始化的时候传入最大长度
        Deque<Integer> path = new ArrayDeque<>(k);
        dfs(1, n, k, path, res);
        return res;
    }

    private void dfs(int begin, int n, int k, Deque<Integer> path, List<List<Integer>> res) {
        if (k == 0) {
            res.add(new ArrayList<>(path));
            return;
        }

        // 基础版本的递归终止条件：if (begin == n + 1) {
        if (begin > n - k + 1) {
            return;
        }
        // 不选当前考虑的数 begin，直接递归到下一层
        dfs(begin + 1, n, k, path, res);

        // 不选当前考虑的数 begin，递归到下一层的时候 k - 1，这里 k 表示还需要选多少个数
        path.addLast(begin);
        dfs(begin + 1, n, k - 1, path, res);
        // 深度优先遍历有回头的过程，因此需要撤销选择
        path.removeLast();
    }
}

作者：liweiwei1419
链接： link
来源：力扣（LeetCode）

4）自己的优化代码

class Solution {
    List<Integer> temp=new ArrayList<>();
    int deep;
    int N;
    List<List<Integer>> result=new ArrayList<>();
    public void backpack(int start,int ceng){
        if(start>N)return;
        if(ceng==deep){
            for(int i=start;i<=N;i++){
                temp.add(i);
                result.add(new ArrayList<>(temp));
                temp.remove(temp.size()-1);
            }
            return;
        }
        for(int i=start;i<=N-deep+ceng;i++){
            temp.add(i);
            backpack(i+1,ceng+1);
            temp.remove(temp.size()-1);
        }
    }
    public List<List<Integer>> combine(int n, int k) {
        deep=k;
        N=n;
        backpack(1,1);
        return result;
    }
}

5）学到的东西

剪枝除了可以用来去重，还可以提高效率，比如本题去重后还可以继续剪枝

8、组合总和（39、Medium）

1）题目要求

给定一个无重复元素的数组 candidates 和一个目标数 target ，找出 candidates 中所有可以使数字和为 target 的组合。

candidates 中的数字可以无限制重复被选取。

说明：

所有数字（包括 target）都是正整数。
解集不能包含重复的组合。
示例 1：

输入：candidates = [2,3,6,7], target = 7,
所求解集为：
[
[7],
[2,2,3]
]
示例 2：

输入：candidates = [2,3,5], target = 8,
所求解集为：
[
[2,2,2,2],
[2,3,3],
[3,5]
]

提示：

1 <= candidates.length <= 30
1 <= candidates[i] <= 200
candidate 中的每个元素都是独一无二的。
1 <= target <= 500

2）我的解法

class Solution {
    List<List<Integer>> result;
    List<Integer> temp=new ArrayList<>();
    public void backpack(int[] candidates, int target){
        if(target==0){
            result.add(new ArrayList<Integer>(temp));
            return;
        }
        if(target<0)return;
        for(int i=0;i<candidates.length;i++){
            temp.add(candidates[i]);
            backpack(candidates,target-candidates[i]);
            temp.remove(temp.size()-1);
        }
    }
    public void quchong(){
        for(int i=0;i<result.size();i++){
            for(int j=i+1;j<result.size();j++){
                if(result.get(i).size()==result.get(j).size()){
                    result.get(i).sort((o1,o2)->o1-o2);
                    result.get(j).sort((o1,o2)->o1-o2);
                    if(result.get(i).equals(result.get(j))){
                        result.remove(j);
                        j--;
                    }
                }
            }
        }
    }
    public List<List<Integer>> combinationSum(int[] candidates, int target) {
        result=new ArrayList<>();
        backpack(candidates,target);
        quchong();
        return result;
    }
}

优化（排序后剪枝）

class Solution {
    List<List<Integer>> result;
    List<Integer> temp=new ArrayList<>();
    public void backpack(int[] candidates,int start, int target){
        if(target==0){
            result.add(new ArrayList<Integer>(temp));
            return;
        }
        if(target<0)return;
        for(int i=start;i<candidates.length;i++){
            temp.add(candidates[i]);
            backpack(candidates,i,target-candidates[i]);
            //之后不得访问当前结点之前的元素，但可以继续访问当前结点
            temp.remove(temp.size()-1);
        }
    }
    public List<List<Integer>> combinationSum(int[] candidates, int target) {
        result=new ArrayList<>();
        Arrays.sort(candidates);
        backpack(candidates,0,target);
        return result;
    }
}

3）其他解法

import java.util.ArrayDeque;
import java.util.ArrayList;
import java.util.Deque;
import java.util.List;

public class Solution {

    public List<List<Integer>> combinationSum(int[] candidates, int target) {
        int len = candidates.length;
        List<List<Integer>> res = new ArrayList<>();
        if (len == 0) {
            return res;
        }

        Deque<Integer> path = new ArrayDeque<>();
        dfs(candidates, 0, len, target, path, res);
        return res;
    }

    /**
     * @param candidates 候选数组
     * @param begin      搜索起点
     * @param len        冗余变量，是 candidates 里的属性，可以不传
     * @param target     每减去一个元素，目标值变小
     * @param path       从根结点到叶子结点的路径，是一个栈
     * @param res        结果集列表
     */
    private void dfs(int[] candidates, int begin, int len, int target, Deque<Integer> path, List<List<Integer>> res) {
        // target 为负数和 0 的时候不再产生新的孩子结点
        if (target < 0) {
            return;
        }
        if (target == 0) {
            res.add(new ArrayList<>(path));
            return;
        }

        // 重点理解这里从 begin 开始搜索的语意
        for (int i = begin; i < len; i++) {
            path.addLast(candidates[i]);

            // 注意：由于每一个元素可以重复使用，下一轮搜索的起点依然是 i，这里非常容易弄错
            dfs(candidates, i, len, target - candidates[i], path, res);

            // 状态重置
            path.removeLast();
        }
    }
}

import java.util.ArrayDeque;
import java.util.ArrayList;
import java.util.Arrays;
import java.util.Deque;
import java.util.List;

public class Solution {

    public List<List<Integer>> combinationSum(int[] candidates, int target) {
        int len = candidates.length;
        List<List<Integer>> res = new ArrayList<>();
        if (len == 0) {
            return res;
        }

        // 排序是剪枝的前提
        Arrays.sort(candidates);
        Deque<Integer> path = new ArrayDeque<>();
        dfs(candidates, 0, len, target, path, res);
        return res;
    }

    private void dfs(int[] candidates, int begin, int len, int target, Deque<Integer> path, List<List<Integer>> res) {
        // 由于进入更深层的时候，小于 0 的部分被剪枝，因此递归终止条件值只判断等于 0 的情况
        if (target == 0) {
            res.add(new ArrayList<>(path));
            return;
        }

        for (int i = begin; i < len; i++) {
            // 重点理解这里剪枝，前提是候选数组已经有序，
            if (target - candidates[i] < 0) {
                break;
            }
            
            path.addLast(candidates[i]);
            dfs(candidates, i, len, target - candidates[i], path, res);
            path.removeLast();
        }
    }
}

作者：liweiwei1419
链接：link
来源：力扣（LeetCode）

4）自己的优化代码

class Solution {
    List<List<Integer>> result;
    List<Integer> temp=new ArrayList<>();
    public void backpack(int[] candidates,int start, int target){
        if(target==0){
            result.add(new ArrayList<Integer>(temp));
            return;
        }
        if(target<0)return;
        for(int i=start;i<candidates.length;i++){//排序，并且之后不得访问当前结点之前的元素，但可以继续访问当前结点
            temp.add(candidates[i]);
            backpack(candidates,i,target-candidates[i]);
            temp.remove(temp.size()-1);
        }
    }
    public List<List<Integer>> combinationSum(int[] candidates, int target) {
        result=new ArrayList<>();
        Arrays.sort(candidates);
        backpack(candidates,0,target);
        return result;
    }
}

5）学到的东西

回溯思想

尽量不在dfs之后去重，而是在搜索过程中剪枝

思想：指定下一层从哪个结点开始

9、组合总和 II（40、Medium）

1）题目要求

给定一个数组 candidates 和一个目标数 target ，找出 candidates 中所有可以使数字和为 target 的组合。

candidates 中的每个数字在每个组合中只能使用一次。

说明：

所有数字（包括目标数）都是正整数。
解集不能包含重复的组合。
示例 1:

输入: candidates = [10,1,2,7,6,1,5], target = 8,
所求解集为:
[
[1, 7],
[1, 2, 5],
[2, 6],
[1, 1, 6]
]
示例 2:

输入: candidates = [2,5,2,1,2], target = 5,
所求解集为:
[
[1,2,2],
[5]
]

2）我的解法

class Solution {
    List<List<Integer>> result=new ArrayList<>();
    List<Integer> temp=new ArrayList<>();
    public void backpack(int[] candidates,int start, int target){
        if(target==0){
            result.add(new ArrayList<Integer>(temp));
            return;
        }
        if(target<0)return;
        for(int i=start;i<candidates.length;i++){
            if(i>start&&candidates[i]==candidates[i-1])continue;
            temp.add(candidates[i]);
            backpack(candidates,i+1,target-candidates[i]);
            temp.remove(temp.size()-1);
        }
    }
    public List<List<Integer>> combinationSum2(int[] candidates, int target) {
        Arrays.sort(candidates);
        backpack(candidates,0,target);
        return result;
    }
}

3）其他解法

import java.util.ArrayDeque;
import java.util.ArrayList;
import java.util.Arrays;
import java.util.Deque;
import java.util.List;

public class Solution {

    public List<List<Integer>> combinationSum2(int[] candidates, int target) {
        int len = candidates.length;
        List<List<Integer>> res = new ArrayList<>();
        if (len == 0) {
            return res;
        }

        // 关键步骤
        Arrays.sort(candidates);

        Deque<Integer> path = new ArrayDeque<>(len);
        dfs(candidates, len, 0, target, path, res);
        return res;
    }

    /**
     * @param candidates 候选数组
     * @param len        冗余变量
     * @param begin      从候选数组的 begin 位置开始搜索
     * @param target     表示剩余，这个值一开始等于 target，基于题目中说明的"所有数字（包括目标数）都是正整数"这个条件
     * @param path       从根结点到叶子结点的路径
     * @param res
     */
    private void dfs(int[] candidates, int len, int begin, int target, Deque<Integer> path, List<List<Integer>> res) {
        if (target == 0) {
            res.add(new ArrayList<>(path));
            return;
        }
        for (int i = begin; i < len; i++) {
            // 大剪枝：减去 candidates[i] 小于 0，减去后面的 candidates[i + 1]、candidates[i + 2] 肯定也小于 0，因此用 break
            if (target - candidates[i] < 0) {
                break;
            }

            // 小剪枝：同一层相同数值的结点，从第 2 个开始，候选数更少，结果一定发生重复，因此跳过，用 continue
            if (i > begin && candidates[i] == candidates[i - 1]) {
                continue;
            }

            path.addLast(candidates[i]);
            // 调试语句 ①
            // System.out.println("递归之前 => " + path + "，剩余 = " + (target - candidates[i]));

            // 因为元素不可以重复使用，这里递归传递下去的是 i + 1 而不是 i
            dfs(candidates, len, i + 1, target - candidates[i], path, res);

            path.removeLast();
            // 调试语句 ②
            // System.out.println("递归之后 => " + path + "，剩余 = " + (target - candidates[i]));
        }
    }

    public static void main(String[] args) {
        int[] candidates = new int[]{10, 1, 2, 7, 6, 1, 5};
        int target = 8;
        Solution solution = new Solution();
        List<List<Integer>> res = solution.combinationSum2(candidates, target);
        System.out.println("输出 => " + res);
    }
}

作者：liweiwei1419
链接： link
来源：力扣（LeetCode）

4）自己的优化代码

class Solution {
    List<List<Integer>> result=new ArrayList<>();
    List<Integer> temp=new ArrayList<>();
    public void backpack(int[] candidates,int start, int target){
        if(target==0){
            result.add(new ArrayList<Integer>(temp));
            return;
        }
        if(target<0)return;
        for(int i=start;i<candidates.length;i++){
            if(i>start&&candidates[i]==candidates[i-1])continue;
            temp.add(candidates[i]);
            backpack(candidates,i+1,target-candidates[i]);
            temp.remove(temp.size()-1);
        }
    }
    public List<List<Integer>> combinationSum2(int[] candidates, int target) {
        Arrays.sort(candidates);
        backpack(candidates,0,target);
        return result;
    }
}

5）学到的东西

回溯思想

在去重时，分析重复原因，并据此在搜索过程中进行剪枝

10、组合总和 III（216、Medium）

1）题目要求

找出所有相加之和为 n 的 k 个数的组合。组合中只允许含有 1 - 9 的正整数，并且每种组合中不存在重复的数字。

说明：

所有数字都是正整数。
解集不能包含重复的组合。
示例 1:

输入: k = 3, n = 7
输出: [[1,2,4]]
示例 2:

输入: k = 3, n = 9
输出: [[1,2,6], [1,3,5], [2,3,4]]

2）我的解法

class Solution {
    List<List<Integer>> result=new ArrayList<>();
    int N;//目标值
    int deep;//最大深度
    int max;//每层最大结点
    int remainCeng;//剩余层
    List<Integer> temp=new ArrayList<>();
    public void backpack(int start,int ceng,int target){
        //start为本层开始结点，ceng为当前层数，target为目标数
        if(ceng==deep){//最后一层不必遍历，直接找
            if(target>9||target<start)return;
            temp.add(target);
            result.add(new ArrayList<Integer>(temp));
            temp.remove(temp.size()-1);
            return;
        }
        remainCeng=deep-ceng+1;
        max=(target-((remainCeng-1)*(1+remainCeng-1)/2))/remainCeng;//每层不用把1-9都遍历一遍，找出最大结点，遍历到最大结点就停即可
        //3*x+3<=7  x<=1
        for(int i=start;i<=9&&i<=max;i++){
            temp.add(i);
            backpack(i+1,ceng+1,target-i);
            temp.remove(temp.size()-1);
        }
    }
    public List<List<Integer>> combinationSum3(int k, int n) {
        N=n;
        deep=k;
        backpack(1,1,n);
        return result;
    }
}

3）其他解法

import java.util.ArrayDeque;
import java.util.ArrayList;
import java.util.Deque;
import java.util.List;

public class Solution {

    public List<List<Integer>> combinationSum3(int k, int n) {
        List<List<Integer>> res = new ArrayList<>();

        // 一开始做一些特殊判断
        if (k <= 0 || n <= 0 || k >= n) {
            return res;
        }

        // 寻找 n 的上限：[9, 8, ... , (9 - k + 1)]，它们的和为 (19 - k) * k / 2
        // 比上限还大，就不用搜索了：
        if (n > (19 - k) * k / 2) {
            return res;
        }

        // 根据官方对 Stack 的使用建议，这里将 Deque 对象当做 stack 使用
        // 注意只使用关于栈的接口
        Deque<Integer> path = new ArrayDeque<>();
        dfs(k, n, 1, path, res);
        return res;
    }

    /**
     * @param k       剩下要找 k 个数
     * @param residue 剩余多少
     * @param start   下一轮搜索的起始元素是多少
     * @param path    深度优先遍历的路径参数（状态变量）
     * @param res     保存结果集的列表
     */
    private void dfs(int k, int residue, int start, Deque<Integer> path, List<List<Integer>> res) {
        // 剪枝：[start, 9] 这个区间里的数都不够 k 个，不用继续往下搜索
        if (10 - start < k) {
            return;
        }
        if (k == 0) {
            if (residue == 0) {
                res.add(new ArrayList<>(path));
                return;
            }
        }

        // 枚举起点值 [..., 7, 8, 9]
        // 找 3 个数，起点最多到 7
        // 找 2 个数，起点最多到 8
        // 规律是，起点上界 + k = 10，故起点上界 = 10 - k
        for (int i = start; i <= 10 - k; i++) {

//            if ((2 * i + k - 1) * k / 2 > residue) {
//                break;
//            }

            // 剪枝
            if (residue - i < 0) {
                break;
            }
            path.addLast(i);
            dfs(k - 1, residue - i, i + 1, path, res);
            path.removeLast();
        }
    }
}

作者：liweiwei1419
链接：link
来源：力扣（LeetCode）
著作权归作者所有。商业转载请联系作者获得授权，非商业转载请注明出处。

4）自己的优化代码

class Solution {
    List<List<Integer>> result=new ArrayList<>();
    int N;//目标值
    int deep;//最大深度
    int max;//每层最大结点
    int remainCeng;//剩余层
    List<Integer> temp=new ArrayList<>();
    public void backpack(int start,int ceng,int target){
        //start为本层开始结点，ceng为当前层数，target为目标数
        if(ceng==deep){//最后一层不必遍历，直接找
            if(target>9||target<start)return;
            temp.add(target);
            result.add(new ArrayList<Integer>(temp));
            temp.remove(temp.size()-1);
            return;
        }
        remainCeng=deep-ceng+1;
        max=(target-((remainCeng-1)*(1+remainCeng-1)/2))/remainCeng;//每层不用把1-9都遍历一遍，找出最大结点，遍历到最大结点就停即可
        //3*x+3<=7  x<=1
        for(int i=start;i<=9&&i<=max;i++){
            temp.add(i);
            backpack(i+1,ceng+1,target-i);
            temp.remove(temp.size()-1);
        }
    }
    public List<List<Integer>> combinationSum3(int k, int n) {
        N=n;
        deep=k;
        backpack(1,1,n);
        return result;
    }
}

5）学到的东西

画树，把能剪的枝条全剪掉

11、子集（78、Medium）

1）题目要求

给定一组不含重复元素的整数数组 nums，返回该数组所有可能的子集（幂集）。

说明：解集不能包含重复的子集。

示例:

输入: nums = [1,2,3]
输出:
[
[3],
[1],
[2],
[1,2,3],
[1,3],
[2,3],
[1,2],
[]
]

2）我的解法

class Solution {
    List<List<Integer>> result=new ArrayList<>();
    List<Integer> temp=new ArrayList<>();
    public void backpack(int start,int[] nums){
        for(int i=start;i<nums.length;i++){
            temp.add(nums[i]);
            result.add(new ArrayList<Integer>(temp));
            backpack(i+1,nums);
            temp.remove(temp.size()-1);
        }
    }
    public List<List<Integer>> subsets(int[] nums) {
        result.add(new ArrayList<Integer>());
        backpack(0,nums);
        return result;
    }
}

3）其他解法

class Solution {
    List<Integer> t = new ArrayList<Integer>();
    List<List<Integer>> ans = new ArrayList<List<Integer>>();

    public List<List<Integer>> subsets(int[] nums) {
        int n = nums.length;
        for (int mask = 0; mask < (1 << n); ++mask) {
            t.clear();
            for (int i = 0; i < n; ++i) {
                if ((mask & (1 << i)) != 0) {
                    t.add(nums[i]);
                }
            }
            ans.add(new ArrayList<Integer>(t));
        }
        return ans;
    }
}

2、回溯

class Solution {
    List<Integer> t = new ArrayList<Integer>();
    List<List<Integer>> ans = new ArrayList<List<Integer>>();

    public List<List<Integer>> subsets(int[] nums) {
        dfs(0, nums);
        return ans;
    }

    public void dfs(int cur, int[] nums) {
        if (cur == nums.length) {
            ans.add(new ArrayList<Integer>(t));
            return;
        }
        t.add(nums[cur]);
        dfs(cur + 1, nums);
        t.remove(t.size() - 1);
        dfs(cur + 1, nums);
    }
}

作者：LeetCode-Solution
链接：link
来源：力扣（LeetCode）

4）自己的优化代码

class Solution {
    List<List<Integer>> result=new ArrayList<>();
    List<Integer> temp=new ArrayList<>();
    public void backpack(int start,int[] nums){
        for(int i=start;i<nums.length;i++){
            temp.add(nums[i]);
            result.add(new ArrayList<Integer>(temp));
            backpack(i+1,nums);
            temp.remove(temp.size()-1);
        }
    }
    public List<List<Integer>> subsets(int[] nums) {
        result.add(new ArrayList<Integer>());
        backpack(0,nums);
        return result;
    }
}

5）学到的东西

不要钻牛角尖过于追求效率高

先画出最普通的回溯树，再在此基础上剪去不必要的枝条即可，不要想一些骚套路

以往都是回溯到最后一层记录，本题为在回溯过程中记录

12、子集 II（90、Medium）

1）题目要求

给定一个可能包含重复元素的整数数组 nums，返回该数组所有可能的子集（幂集）。

说明：解集不能包含重复的子集。

示例:

输入: [1,2,2]
输出:
[
[2],
[1],
[1,2,2],
[2,2],
[1,2],
[]
]

2）我的解法

class Solution {
    List<List<Integer>> result=new ArrayList<>();
    List<Integer> temp=new ArrayList<>();
    public void backpack(int start,int[] nums){
        for(int i=start;i<nums.length;i++){
            if(i>start&&nums[i]==nums[i-1])continue;
            temp.add(nums[i]);
            result.add(new ArrayList<Integer>(temp));
            backpack(i+1,nums);
            temp.remove(temp.size()-1);
        }
    }
    public List<List<Integer>> subsetsWithDup(int[] nums) {
        result.add(new ArrayList<Integer>());
        Arrays.sort(nums);
        backpack(0,nums);
        return result;
    }
}

3）其他解法

public List<List<Integer>> subsetsWithDup(int[] nums) {
    List<List<Integer>> ans = new ArrayList<>();
    Arrays.sort(nums); //排序
    getAns(nums, 0, new ArrayList<>(), ans);
    return ans;
}

private void getAns(int[] nums, int start, ArrayList<Integer> temp, List<List<Integer>> ans) {
    ans.add(new ArrayList<>(temp));
    for (int i = start; i < nums.length; i++) {
        //和上个数字相等就跳过
        if (i > start && nums[i] == nums[i - 1]) {
            continue;
        }
        temp.add(nums[i]);
        getAns(nums, i + 1, temp, ans);
        temp.remove(temp.size() - 1);
    }
}

作者：windliang
链接：link
来源：力扣（LeetCode）

4）自己的优化代码

class Solution {
    List<List<Integer>> result=new ArrayList<>();
    List<Integer> temp=new ArrayList<>();
    public void backpack(int start,int[] nums){
        for(int i=start;i<nums.length;i++){
            if(i>start&&nums[i]==nums[i-1])continue;
            temp.add(nums[i]);
            result.add(new ArrayList<Integer>(temp));
            backpack(i+1,nums);
            temp.remove(temp.size()-1);
        }
    }
    public List<List<Integer>> subsetsWithDup(int[] nums) {
        result.add(new ArrayList<Integer>());
        Arrays.sort(nums);
        backpack(0,nums);
        return result;
    }
}

5）学到的东西

回溯、剪枝

位操作

13、 分割回文串（131、Medium）

1）题目要求

给定一个字符串 s，将 s 分割成一些子串，使每个子串都是回文串。

返回 s 所有可能的分割方案。

示例:

输入: “aab”
输出:
[
[“aa”,“b”],
[“a”,“a”,“b”]
]

2）我的解法

按照截取位置，比如aab，其截取位置有两个，即a 1 a 2 b，然后此题就变成了找出[1，2]的所有子集，找出来之后再进行剪枝，剪去不是回文的即可

class Solution {
    List<List<String>> result=new ArrayList<>();
    List<String> temp=new ArrayList<>();
    public void backpack(String s){
        for(int i=0;i<s.length()-1;i++){
             if(!isHuiWen(s.substring(0,i+1)))continue;
            temp.add(s.substring(0,i+1));
            if(isHuiWen(s.substring(i+1))){
                temp.add(s.substring(i+1));
                result.add(new ArrayList<String>(temp));
                temp.remove(temp.size()-1);
            }
            backpack(s.substring(i+1));
            temp.remove(temp.size()-1);
        }
    }
    public boolean isHuiWen(String s){
         for(int i=0;2*i+1<=s.length();i++){
             if(s.charAt(i)!=s.charAt(s.length()-i-1))return false;
         }
         return true;
    }
    public List<List<String>> partition(String s) {
        backpack(s);
        if(isHuiWen(s)){temp.add(s);result.add(new ArrayList<>(temp));}//s本身单独判断
        return result;
    }
}

3）其他解法

import java.util.ArrayDeque;
import java.util.ArrayList;
import java.util.Deque;
import java.util.List;

public class Solution {

    public List<List<String>> partition(String s) {
        int len = s.length();
        List<List<String>> res = new ArrayList<>();
        if (len == 0) {
            return res;
        }

        // Stack 这个类 Java 的文档里推荐写成 Deque stack = new ArrayDeque();
        // 注意：只使用 stack 相关的接口
        Deque<String> stack = new ArrayDeque<>();
        backtracking(s, 0, len, stack, res);
        return res;
    }

    /**
     * @param s
     * @param start 起始字符的索引
     * @param len   字符串 s 的长度，可以设置为全局变量
     * @param path  记录从根结点到叶子结点的路径
     * @param res   记录所有的结果
     */
    private void backtracking(String s, int start, int len, Deque<String> path, List<List<String>> res) {
        if (start == len) {
            res.add(new ArrayList<>(path));
            return;
        }

        for (int i = start; i < len; i++) {

            // 因为截取字符串是消耗性能的，因此，采用传子串索引的方式判断一个子串是否是回文子串
            // 不是的话，剪枝
            if (!checkPalindrome(s, start, i)) {
                continue;
            }

            path.addLast(s.substring(start, i + 1));
            backtracking(s, i + 1, len, path, res);
            path.removeLast();
        }
    }

    /**
     * 这一步的时间复杂度是 O(N)，因此，可以采用动态规划先把回文子串的结果记录在一个表格里
     *
     * @param str
     * @param left  子串的左边界，可以取到
     * @param right 子串的右边界，可以取到
     * @return
     */
    private boolean checkPalindrome(String str, int left, int right) {
        // 严格小于即可
        while (left < right) {
            if (str.charAt(left) != str.charAt(right)) {
                return false;
            }
            left++;
            right--;
        }
        return true;
    }
}

import java.util.ArrayDeque;
import java.util.ArrayList;
import java.util.Deque;
import java.util.List;
import java.util.Stack;

public class Solution {

    public List<List<String>> partition(String s) {
        int len = s.length();
        List<List<String>> res = new ArrayList<>();
        if (len == 0) {
            return res;
        }

        // 预处理
        // 状态：dp[i][j] 表示 s[i][j] 是否是回文
        boolean[][] dp = new boolean[len][len];
        // 状态转移方程：在 s[i] == s[j] 的时候，dp[i][j] 参考 dp[i + 1][j - 1]
        for (int right = 0; right < len; right++) {
            // 注意：left <= right 取等号表示 1 个字符的时候也需要判断
            for (int left = 0; left <= right; left++) {
                if (s.charAt(left) == s.charAt(right) && (right - left <= 2 || dp[left + 1][right - 1])) {
                    dp[left][right] = true;
                }
            }
        }

        Deque<String> stack = new ArrayDeque<>();
        backtracking(s, 0, len, dp, stack, res);
        return res;
    }

    private void backtracking(String s,
                              int start,
                              int len,
                              boolean[][] dp,
                              Deque<String> path,
                              List<List<String>> res) {
        if (start == len) {
            res.add(new ArrayList<>(path));
            return;
        }

        for (int i = start; i < len; i++) {
            // 剪枝
            if (!dp[start][i]) {
                continue;
            }
            path.addLast(s.substring(start, i + 1));
            backtracking(s, i + 1, len, dp, path, res);
            path.removeLast();
        }
    }
}

作者：liweiwei1419
链接：link
来源：力扣（LeetCode）

4）自己的优化代码

class Solution {
    List<List<String>> result=new ArrayList<>();
    List<String> temp=new ArrayList<>();
    public void backpack(String s){
        for(int i=0;i<s.length()-1;i++){
             if(!isHuiWen(s.substring(0,i+1)))continue;
            temp.add(s.substring(0,i+1));
            if(isHuiWen(s.substring(i+1))){
                temp.add(s.substring(i+1));
                result.add(new ArrayList<String>(temp));
                temp.remove(temp.size()-1);
            }
            backpack(s.substring(i+1));
            temp.remove(temp.size()-1);
        }
    }
    public boolean isHuiWen(String s){
         for(int i=0;2*i+1<=s.length();i++){
             if(s.charAt(i)!=s.charAt(s.length()-i-1))return false;
         }
         return true;
    }
    public List<List<String>> partition(String s) {
        backpack(s);
        if(isHuiWen(s)){temp.add(s);result.add(new ArrayList<>(temp));}//s本身单独判断
        return result;
    }
}

5）学到的东西

画树、剪枝

利用动态规划判断回文

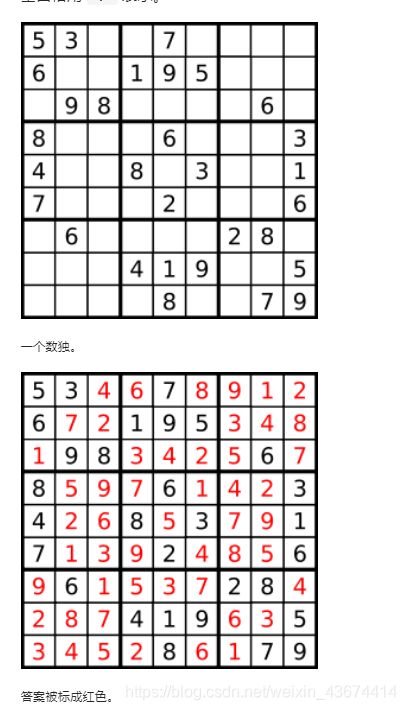
14、数独（37、Hard）

1）题目要求

编写一个程序，通过填充空格来解决数独问题。

一个数独的解法需遵循如下规则：

数字 1-9 在每一行只能出现一次。
数字 1-9 在每一列只能出现一次。
数字 1-9 在每一个以粗实线分隔的 3x3 宫内只能出现一次。
空白格用 ‘.’ 表示。

提示：

给定的数独序列只包含数字 1-9 和字符 ‘.’ 。
你可以假设给定的数独只有唯一解。
给定数独永远是 9x9 形式的。

2）我的解法

1、严重超时，但能得出正确结果

class Solution {
    int[][][] visited =new int[9][9][9];
    int nexti=0,nextj=0;
    public boolean backpack(int i,int j,char[][] board){
        if(i>=9)return true;
                if(board[i][j]!='.'){
                    if(visited[i][j][board[i][j]-'1']>0)return false;
                    makeVisited(i,j,board);
                    if(j<8){nexti=i;nextj=j+1;}
                    if(j==8){nexti=i+1;nextj=0;}
                    if(backpack(nexti,nextj,board))return true;
                    makeUnVisited(i,j,board);
                    return false;
                }
                else{
                    for(int k=0;k<9;k++){
                        if(visited[i][j][k]>0)continue;
                        board[i][j]=(char)(k+'1');
                        makeVisited(i,j,board);
                        System.out.println(i+" "+j+" "+board[i][j]);
                        if(j<8){nexti=i;nextj=j+1;}
                        if(j==8){nexti=i+1;nextj=0;}
                        if(backpack(nexti,nextj,board))return true;
                        makeUnVisited(i,j,board);
                        board[i][j]='.';
                    }
                    return false;
                }


    }
    public void makeVisited(int i,int j,char[][] board){
        for(int k=0;k<9;k++){
            visited[i][k][board[i][j]-'1']++;
            visited[k][j][board[i][j]-'1']++;
        }
        int x=i/3,y=j/3;
        x*=3;y*=3;
        for(int p=x;p<x+3;p++){
            for(int q=y;q<y+3;q++){
                visited[p][q][board[i][j]-'1']++;
            }
        }
    }
    public void makeUnVisited(int i,int j,char[][] board){
        for(int k=0;k<9;k++){
            visited[i][k][board[i][j]-'1']--;
            visited[k][j][board[i][j]-'1']--;
        }
        int x=i/3,y=j/3;
        x*=3;y*=3;
        for(int p=x;p<x+3;p++){
            for(int q=y;q<y+3;q++){
                visited[p][q][board[i][j]-'1']--;
            }
        }
    }
    public void solveSudoku(char[][] board) {
        backpack(0,0,board);
    }
}

2、改进备忘录，用空间换时间：还是超时。。.。

class Solution {
    boolean[][] Cvisited =new boolean[9][9];//行
    boolean[][] Rvisited =new boolean[9][9];//列
    boolean[][][] CRvisited =new boolean[3][3][9];//3*3
    int nexti=0,nextj=0;
    public boolean backpack(int i,int j,char[][] board){
        if(i>=9)return true;
                if(board[i][j]!='.'){
                    if(IsVisited(i,j,board[i][j]-'1'))return false;
                    makeVisited(i,j,board);
                    if(j<8){nexti=i;nextj=j+1;}
                    if(j==8){nexti=i+1;nextj=0;}
                    if(backpack(nexti,nextj,board))return true;
                    makeUnVisited(i,j,board);
                    return false;
                }
                else{
                    for(int k=0;k<9;k++){
                        if(IsVisited(i,j,k))continue;
                        board[i][j]=(char)(k+'1');
                        makeVisited(i,j,board);
                        if(j<8){nexti=i;nextj=j+1;}
                        if(j==8){nexti=i+1;nextj=0;}
                        if(backpack(nexti,nextj,board))return true;
                        makeUnVisited(i,j,board);
                        board[i][j]='.';
                    }
                    return false;
                }


    }
    public boolean IsVisited(int i,int j,int k){
        return Cvisited[i][k]||Rvisited[j][k]||CRvisited[i/3][j/3][k];
    }
    public void makeVisited(int i,int j,char[][] board){
        Cvisited[i][board[i][j]-'1']=true;
        Rvisited[j][board[i][j]-'1']=true;
        CRvisited[i/3][j/3][board[i][j]-'1']=true;

    }
    public void makeUnVisited(int i,int j,char[][] board){
        Cvisited[i][board[i][j]-'1']=false;
        Rvisited[j][board[i][j]-'1']=false;
        CRvisited[i/3][j/3][board[i][j]-'1']=false;

    }
    public void solveSudoku(char[][] board) {
        backpack(0,0,board);
    }
}

3、再次改进，先进行初始化：通过

class Solution {
    boolean[][] Cvisited =new boolean[9][9];//行
    boolean[][] Rvisited =new boolean[9][9];//列
    boolean[][][] CRvisited =new boolean[3][3][9];//3*3
    int nexti=0,nextj=0;
    public boolean backpack(int i,int j,char[][] board){
        if(i>=9)return true;
                if(board[i][j]!='.'){
                    if(j<8){nexti=i;nextj=j+1;}
                    if(j==8){nexti=i+1;nextj=0;}
                    if(backpack(nexti,nextj,board))return true;
                    return false;
                }
                else{
                    for(int k=0;k<9;k++){
                        if(IsVisited(i,j,k))continue;
                        board[i][j]=(char)(k+'1');
                        makeVisited(i,j,board);
                        if(j<8){nexti=i;nextj=j+1;}
                        if(j==8){nexti=i+1;nextj=0;}
                        if(backpack(nexti,nextj,board))return true;
                        makeUnVisited(i,j,board);
                        board[i][j]='.';
                    }
                    return false;
                }


    }
    public boolean IsVisited(int i,int j,int k){
        return Cvisited[i][k]||Rvisited[j][k]||CRvisited[i/3][j/3][k];
    }
    public void makeVisited(int i,int j,char[][] board){
        Cvisited[i][board[i][j]-'1']=true;
        Rvisited[j][board[i][j]-'1']=true;
        CRvisited[i/3][j/3][board[i][j]-'1']=true;

    }
    public void makeUnVisited(int i,int j,char[][] board){
        Cvisited[i][board[i][j]-'1']=false;
        Rvisited[j][board[i][j]-'1']=false;
        CRvisited[i/3][j/3][board[i][j]-'1']=false;

    }
    public void solveSudoku(char[][] board) {
        for(int i=0;i<9;i++){
            for(int j=0;j<9;j++){
                if(board[i][j]!='.'){
                    makeVisited(i,j,board);
                }
            }
        }
        backpack(0,0,board);
    }
}

3）其他解法

解数独思路：
类似人的思考方式去尝试，行，列，还有 3*3 的方格内数字是 1~9 不能重复。

我们尝试填充，如果发现重复了，那么擦除重新进行新一轮的尝试，直到把整个数组填充完成。

算法步骤:
数独首先行，列，还有 3*3 的方格内数字是 1~9 不能重复。

声明布尔数组，表明行列中某个数字是否被使用了， 被用过视为 true，没用过为 false。

初始化布尔数组，表明哪些数字已经被使用过了。

尝试去填充数组，只要行，列， 还有 3*3 的方格内 出现已经被使用过的数字，我们就不填充，否则尝试填充。

如果填充失败，那么我们需要回溯。将原来尝试填充的地方改回来。

class Solution {
    public void solveSudoku(char[][] board) {
        // 三个布尔数组 表明 行, 列, 还有 3*3 的方格的数字是否被使用过
        boolean[][] rowUsed = new boolean[9][10];
        boolean[][] colUsed = new boolean[9][10];
        boolean[][][] boxUsed = new boolean[3][3][10];
        // 初始化
        for(int row = 0; row < board.length; row++){
            for(int col = 0; col < board[0].length; col++) {
                int num = board[row][col] - '0';
                if(1 <= num && num <= 9){
                    rowUsed[row][num] = true;
                    colUsed[col][num] = true;
                    boxUsed[row/3][col/3][num] = true;
                }
            }
        }
        // 递归尝试填充数组 
        recusiveSolveSudoku(board, rowUsed, colUsed, boxUsed, 0, 0);
    }
    
    private boolean recusiveSolveSudoku(char[][]board, boolean[][]rowUsed, boolean[][]colUsed, boolean[][][]boxUsed, int row, int col){
        // 边界校验, 如果已经填充完成, 返回true, 表示一切结束
        if(col == board[0].length){
            col = 0;
            row++;
            if(row == board.length){
                return true;
            }
        }
        // 是空则尝试填充, 否则跳过继续尝试填充下一个位置
        if(board[row][col] == '.') {
            // 尝试填充1~9
            for(int num = 1; num <= 9; num++){
                boolean canUsed = !(rowUsed[row][num] || colUsed[col][num] || boxUsed[row/3][col/3][num]);
                if(canUsed){
                    rowUsed[row][num] = true;
                    colUsed[col][num] = true;
                    boxUsed[row/3][col/3][num] = true;
                    
                    board[row][col] = (char)('0' + num);
                    if(recusiveSolveSudoku(board, rowUsed, colUsed, boxUsed, row, col + 1)){
                        return true;
                    }
                    board[row][col] = '.';
                    
                    rowUsed[row][num] = false;
                    colUsed[col][num] = false;
                    boxUsed[row/3][col/3][num] = false;
                }
            }
        } else {
            return recusiveSolveSudoku(board, rowUsed, colUsed, boxUsed, row, col + 1);
        }
        return false;
    }
}

作者：I_use_java
链接：link
来源：力扣（LeetCode）

4）自己的优化代码

class Solution {
    boolean[][] Cvisited =new boolean[9][9];//行
    boolean[][] Rvisited =new boolean[9][9];//列
    boolean[][][] CRvisited =new boolean[3][3][9];//3*3
    int nexti=0,nextj=0;
    public boolean backpack(int i,int j,char[][] board){
        if(i>=9)return true;
                if(board[i][j]!='.'){
                    if(j<8){nexti=i;nextj=j+1;}
                    if(j==8){nexti=i+1;nextj=0;}
                    if(backpack(nexti,nextj,board))return true;
                    return false;
                }
                else{
                    for(int k=0;k<9;k++){
                        if(IsVisited(i,j,k))continue;
                        board[i][j]=(char)(k+'1');
                        makeVisited(i,j,board);
                        if(j<8){nexti=i;nextj=j+1;}
                        if(j==8){nexti=i+1;nextj=0;}
                        if(backpack(nexti,nextj,board))return true;
                        makeUnVisited(i,j,board);
                        board[i][j]='.';
                    }
                    return false;
                }


    }
    public boolean IsVisited(int i,int j,int k){
        return Cvisited[i][k]||Rvisited[j][k]||CRvisited[i/3][j/3][k];
    }
    public void makeVisited(int i,int j,char[][] board){
        Cvisited[i][board[i][j]-'1']=true;
        Rvisited[j][board[i][j]-'1']=true;
        CRvisited[i/3][j/3][board[i][j]-'1']=true;

    }
    public void makeUnVisited(int i,int j,char[][] board){
        Cvisited[i][board[i][j]-'1']=false;
        Rvisited[j][board[i][j]-'1']=false;
        CRvisited[i/3][j/3][board[i][j]-'1']=false;

    }
    public void solveSudoku(char[][] board) {
        for(int i=0;i<9;i++){
            for(int j=0;j<9;j++){
                if(board[i][j]!='.'){
                    makeVisited(i,j,board);
                }
            }
        }
        backpack(0,0,board);
    }
}

5）学到的东西

回溯思想 1-9一个个试

改进思路：
（1）用空间换时间，每行、每列、每个小方块做备忘录，大大提高效率

（2）先初始化，之后遇到不是’.'的直接跳过即可

15、N 皇后（51、Hard）

1）题目要求

示例：

输入：4
输出：[
[".Q…", // 解法 1
“…Q”,
“Q…”,
“…Q.”],

["…Q.", // 解法 2
“Q…”,
“…Q”,
“.Q…”]
]
解释: 4 皇后问题存在两个不同的解法。

提示：

皇后彼此不能相互攻击，也就是说：任何两个皇后都不能处于同一条横行、纵行或斜线上。

2）我的解法

class Solution {
    List<List<String>> result=new ArrayList<>();
    boolean[] Rvisited;//列
    boolean[] LXvisited;//左斜线
    boolean[] RXvisited;//右斜线
    int N=0;
    StringBuilder s=new StringBuilder();
    List<String> temp=new ArrayList<>();
    public void backpack(int i){
        if(i>=N){
            result.add(new ArrayList<String>(temp));
            return;
        }
        for(int j=0;j<N;j++){
            if(isVisited(i,j))continue;
            for(int k=0;k<N;k++){
                if(k==j)s.append("Q");
                else s.append(".");
            }
            temp.add(s.toString());
            for(int k=0;k<N;k++)s.deleteCharAt(s.length()-1);
            Rvisited[j]=true;
            RXvisited[j-i+N-1]=true;
            LXvisited[i+j]=true;
            backpack(i+1);
            Rvisited[j]=false;
            RXvisited[j-i+N-1]=false;
            LXvisited[i+j]=false;
            temp.remove(temp.size()-1);
        }
        return ;

    }
    public boolean isVisited(int i,int j){
        return Rvisited[j]||LXvisited[i+j]||RXvisited[j-i+N-1];
    }
    public List<List<String>> solveNQueens(int n) {
        N=n;
        Rvisited=new boolean[n];
        RXvisited=new boolean[2*n-1];
        LXvisited=new boolean[2*n-1];
        backpack(0);
        return result;
    }
}

3）其他解法

1、

import java.util.ArrayDeque;
import java.util.ArrayList;
import java.util.Deque;
import java.util.List;

public class Solution {

    private int n;
    // 记录某一列是否放置了皇后
    private boolean[] col;
    // 记录主对角线上的单元格是否放置了皇后
    private boolean[] sub;
    // 记录了副对角线上的单元格是否放置了皇后
    private boolean[] main;
    private List<List<String>> res;

    public List<List<String>> solveNQueens(int n) {
        res = new ArrayList<>();
        if (n == 0) {
            return res;
        }

        // 设置成员变量，减少参数传递，具体作为方法参数还是作为成员变量，请参考团队开发规范
        this.n = n;
        this.col = new boolean[n];
        this.sub = new boolean[2 * n - 1];
        this.main = new boolean[2 * n - 1];
        Deque<Integer> path = new ArrayDeque<>();
        dfs(0, path);
        return res;
    }

    private void dfs(int row, Deque<Integer> path) {
        if (row == n) {
            // 深度优先遍历到下标为 n，表示 [0.. n - 1] 已经填完，得到了一个结果
            List<String> board = convert2board(path);
            res.add(board);
            return;
        }

        // 针对下标为 row 的每一列，尝试是否可以放置
        for (int j = 0; j < n; j++) {
            if (!col[j] && !sub[row + j] && !main[row - j + n - 1]) {
                path.addLast(j);
                col[j] = true;
                sub[row + j] = true;
                main[row - j + n - 1] = true;

                dfs(row + 1, path);

                main[row - j + n - 1] = false;
                sub[row + j] = false;
                col[j] = false;
                path.removeLast();
            }
        }
    }

    private List<String> convert2board(Deque<Integer> path) {
        List<String> board = new ArrayList<>();
        for (Integer num : path) {
            StringBuilder row = new StringBuilder();
            row.append(".".repeat(Math.max(0, n)));
            row.replace(num, num + 1, "Q");
            board.add(row.toString());
        }
        return board;
    }
}

2、HashSet

import java.util.ArrayDeque;
import java.util.ArrayList;
import java.util.Deque;
import java.util.HashSet;
import java.util.List;
import java.util.Set;

public class Solution {

    private Set<Integer> col;
    private Set<Integer> sub;
    private Set<Integer> main;
    private int n;
    private List<List<String>> res;

    public List<List<String>> solveNQueens(int n) {
        this.n = n;
        res = new ArrayList<>();
        if (n == 0) {
            return res;
        }

        col = new HashSet<>();
        sub = new HashSet<>();
        main = new HashSet<>();

        Deque<Integer> path = new ArrayDeque<>();
        dfs(0, path);
        return res;
    }

    private void dfs(int row, Deque<Integer> path) {
        if (row == n) {
            List<String> board = convert2board(path);
            res.add(board);
            return;
        }

        // 针对每一列，尝试是否可以放置
        for (int i = 0; i < n; i++) {
            if (!col.contains(i) && !sub.contains(row + i) && !main.contains(row - i)) {
                path.addLast(i);
                col.add(i);
                sub.add(row + i);
                main.add(row - i);

                dfs(row + 1, path);

                main.remove(row - i);
                sub.remove(row + i);
                col.remove(i);
                path.removeLast();
            }
        }
    }

    private List<String> convert2board(Deque<Integer> path) {
        List<String> board = new ArrayList<>();
        for (Integer num : path) {
            StringBuilder row = new StringBuilder();
            row.append(".".repeat(Math.max(0, n)));
            row.replace(num, num + 1, "Q");
            board.add(row.toString());
        }
        return board;
    }
}

作者：liweiwei1419
链接：link
来源：力扣（LeetCode）

4）自己的优化代码

class Solution {
    List<List<String>> result=new ArrayList<>();
    boolean[] Rvisited;//列
    boolean[] LXvisited;//左斜线
    boolean[] RXvisited;//右斜线
    int N=0;
    StringBuilder s=new StringBuilder();
    List<String> temp=new ArrayList<>();
    public void backpack(int i){
        if(i>=N){
            result.add(new ArrayList<String>(temp));
            return;
        }
        for(int j=0;j<N;j++){
            if(isVisited(i,j))continue;
            for(int k=0;k<N;k++){
                if(k==j)s.append("Q");
                else s.append(".");
            }
            temp.add(s.toString());
            for(int k=0;k<N;k++)s.deleteCharAt(s.length()-1);
            Rvisited[j]=true;
            RXvisited[j-i+N-1]=true;
            LXvisited[i+j]=true;
            backpack(i+1);
            Rvisited[j]=false;
            RXvisited[j-i+N-1]=false;
            LXvisited[i+j]=false;
            temp.remove(temp.size()-1);
        }
        return ;

    }
    public boolean isVisited(int i,int j){
        return Rvisited[j]||LXvisited[i+j]||RXvisited[j-i+N-1];
    }
    public List<List<String>> solveNQueens(int n) {
        N=n;
        Rvisited=new boolean[n];
        RXvisited=new boolean[2*n-1];
        LXvisited=new boolean[2*n-1];
        backpack(0);
        return result;
    }
}

5）学到的东西

回溯思想

多弄点备忘录、找规律