2017第八届蓝桥杯省赛JAVA B组真题解析(带源码及解析)
蓝桥杯历年真题及解析.
目录:
- 蓝桥杯历年真题及解析.
-
- A: 购物单(难度:★★)
-
- 分析:
- 代码:
- B:纸牌三角形(难度:★★★)
-
- 分析:
- 代码:
- C:承压计算(难度:★★★)
-
- 分析:
- 代码:
- D:魔方状态(难度:★★★★★)
-
- 分析:
- 代码:
- E:取数位(难度:★)
-
- 分析:
- 代码:
- F:最大公共子串(难度:★★)
-
- 分析:
- 代码:
- G:日期问题(难度:★)
-
- 分析:
- 代码:
- H:包子凑数(难度:★★★★)
-
- 分析:
- 代码:
- I:分巧克力(难度:★★★★)
-
- 分析:
- 代码:
- J:K倍区间(难度:★★★★★)
- 写在最后
A: 购物单(难度:★★)
小明刚刚找到工作,老板人很好,只是老板夫人很爱购物。老板忙的时候经常让小明帮忙到商场代为购物。小明很厌烦,但又不好推辞。
这不,XX大促销又来了!老板夫人开出了长长的购物单,都是有打折优惠的。
小明也有个怪癖,不到万不得已,从不刷卡,直接现金搞定。
现在小明很心烦,请你帮他计算一下,需要从取款机上取多少现金,才能搞定这次购物。
取款机只能提供100元面额的纸币。小明想尽可能少取些现金,够用就行了。
你的任务是计算出,小明最少需要取多少现金。
以下是让人头疼的购物单,为了保护隐私,物品名称被隐藏了。
**** 180.90 88折
**** 10.25 65折
**** 56.14 9折
**** 104.65 9折
**** 100.30 88折
**** 297.15 半价
**** 26.75 65折
**** 130.62 半价
**** 240.28 58折
**** 270.62 8折
**** 115.87 88折
**** 247.34 95折
**** 73.21 9折
**** 101.00 半价
**** 79.54 半价
**** 278.44 7折
**** 199.26 半价
**** 12.97 9折
**** 166.30 78折
**** 125.50 58折
**** 84.98 9折
**** 113.35 68折
**** 166.57 半价
**** 42.56 9折
**** 81.90 95折
**** 131.78 8折
**** 255.89 78折
**** 109.17 9折
**** 146.69 68折
**** 139.33 65折
**** 141.16 78折
**** 154.74 8折
**** 59.42 8折
**** 85.44 68折
**** 293.70 88折
**** 261.79 65折
**** 11.30 88折
**** 268.27 58折
**** 128.29 88折
**** 251.03 8折
**** 208.39 75折
**** 128.88 75折
**** 62.06 9折
**** 225.87 75折
**** 12.89 75折
**** 34.28 75折
**** 62.16 58折
**** 129.12 半价
**** 218.37 半价
**** 289.69 8折
需要说明的是,88折指的是按标价的88%计算,而8折是按80%计算,余者类推。
特别地,半价是按50%计算。
请提交小明要从取款机上提取的金额,单位是元。
答案是一个整数,类似4300的样子,结尾必然是00,不要填写任何多余的内容。
特别提醒:不许携带计算器入场,也不能打开手机。
分析:
这是一个字符串处理问题,我们只需要获取对应的数据,然后打对应的折扣,
最终将他们添加到sum,对sum进行题目对应的取整操作。
代码:
满分
package JB2017;
import java.util.Scanner;
public class A购物单 {
public static double check(String three){
if(three.equals("半价")){
return 0.5;
}else{
three=three.replace("折", "");
if(three.length()==1){
return Integer.valueOf(three)/10.0;
}else{
return Integer.valueOf(three)/100.0;
}
}
}
public static void main(String[] args) {
Scanner sc=new Scanner(System.in);
String one;
double two;
String three;
double sum=0;
for(int i=0;i<50;i++){
one=sc.next();
two=sc.nextDouble();
three=sc.next();
sum+=(two*check(three));
}
System.out.println((((int)(sum+1)/100)+1)*100);
}
}
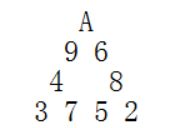
B:纸牌三角形(难度:★★★)
A,2,3,4,5,6,7,8,9 共9张纸牌排成一个正三角形(A按1计算)。要求每个边的和相等。
下图就是一种排法。
这样的排法可能会有很多。
如果考虑旋转、镜像后相同的算同一种,一共有多少种不同的排法呢?
请你计算并提交该数字。
注意:需要提交的是一个整数,不要提交任何多余内容。
分析:
这是一个全排列问题,我们只需要枚举出所有的排列情况,
然后对每一种情况进行 check,统计次数即可。
考虑翻转与镜像,我们需要对最终结果/6.
代码:
=满分
package JB2017;
public class B纸牌三角形 {
public static int arr[]={1,2,3,4,5,6,7,8,9};
public static int count=0;
public static void check(){
if(arr[0]+arr[1]+arr[2]+arr[3]==arr[3]+arr[4]+arr[5]+arr[6]
&&arr[0]+arr[1]+arr[2]+arr[3]==arr[6]+arr[7]+arr[8]+arr[0]){
count++;
}
}
public static void qpl(int k){
if(k==9)check();
for(int i=k;i<9;i++){
int t=arr[k];arr[k]=arr[i];arr[i]=t;
qpl(k+1);
t=arr[k];arr[k]=arr[i];arr[i]=t;
}
}
public static void main(String[] args) {
qpl(0);
System.out.println(count/6);
}
}
C:承压计算(难度:★★★)
X星球的高科技实验室中整齐地堆放着某批珍贵金属原料。
每块金属原料的外形、尺寸完全一致,但重量不同。
金属材料被严格地堆放成金字塔形。
7
5 8
7 8 8
9 2 7 2
8 1 4 9 1
8 1 8 8 4 1
7 9 6 1 4 5 4
5 6 5 5 6 9 5 6
5 5 4 7 9 3 5 5 1
7 5 7 9 7 4 7 3 3 1
4 6 4 5 5 8 8 3 2 4 3
1 1 3 3 1 6 6 5 5 4 4 2
9 9 9 2 1 9 1 9 2 9 5 7 9
4 3 3 7 7 9 3 6 1 3 8 8 3 7
3 6 8 1 5 3 9 5 8 3 8 1 8 3 3
8 3 2 3 3 5 5 8 5 4 2 8 6 7 6 9
8 1 8 1 8 4 6 2 2 1 7 9 4 2 3 3 4
2 8 4 2 2 9 9 2 8 3 4 9 6 3 9 4 6 9
7 9 7 4 9 7 6 6 2 8 9 4 1 8 1 7 2 1 6
9 2 8 6 4 2 7 9 5 4 1 2 5 1 7 3 9 8 3 3
5 2 1 6 7 9 3 2 8 9 5 5 6 6 6 2 1 8 7 9 9
6 7 1 8 8 7 5 3 6 5 4 7 3 4 6 7 8 1 3 2 7 4
2 2 6 3 5 3 4 9 2 4 5 7 6 6 3 2 7 2 4 8 5 5 4
7 4 4 5 8 3 3 8 1 8 6 3 2 1 6 2 6 4 6 3 8 2 9 6
1 2 4 1 3 3 5 3 4 9 6 3 8 6 5 9 1 5 3 2 6 8 8 5 3
2 2 7 9 3 3 2 8 6 9 8 4 4 9 5 8 2 6 3 4 8 4 9 3 8 8
7 7 7 9 7 5 2 7 9 2 5 1 9 2 6 5 3 9 3 5 7 3 5 4 2 8 9
7 7 6 6 8 7 5 5 8 2 4 7 7 4 7 2 6 9 2 1 8 2 9 8 5 7 3 6
5 9 4 5 5 7 5 5 6 3 5 3 9 5 8 9 5 4 1 2 6 1 4 3 5 3 2 4 1
X X X X X X X X X X X X X X X X X X X X X X X X X X X X X X
其中的数字代表金属块的重量(计量单位较大)。
最下一层的X代表30台极高精度的电子秤。
假设每块原料的重量都十分精确地平均落在下方的两个金属块上,
最后,所有的金属块的重量都严格精确地平分落在最底层的电子秤上。
电子秤的计量单位很小,所以显示的数字很大。
工作人员发现,其中读数最小的电子秤的示数为:2086458231
请你推算出:读数最大的电子秤的示数为多少?
注意:需要提交的是一个整数,不要填写任何多余的内容。
分析:
这就是一个大数的杨辉三角问题,定义long以免溢出
为了防止精度溢出问题,我们可以提前给每个位置进行乘2的29次方。
纯暴力问题。
代码:
满分
package JB2017;
import java.util.Arrays;
import java.util.Scanner;
public class C承压计算 {
public static void main(String[] args) {
Scanner sc=new Scanner(System.in);
long arr[][]=new long [30][30];
for(int i=0;i<29;i++){
for(int j=0;j<=i;j++){
arr[i][j]=sc.nextLong();
}
}
for(int i=0;i<30;i++){
for(int j=0;j<30;j++){
arr[i][j]*=Math.pow(2,29);
}
}
for(int i=1;i<=29;i++){
for(int j=0;j<=i;j++){
arr[i][j]+=(j-1<0?0:arr[i-1][j-1]/2)+arr[i-1][j]/2;
}
}
Arrays.sort(arr[29]);
long t=2086458231/arr[29][0];
System.out.println(arr[29][29]*t);
}
}
D:魔方状态(难度:★★★★★)
二阶魔方就是只有2层的魔方,只由8个小块组成。
如图p1.png所示。

小明很淘气,他只喜欢3种颜色,所有把家里的二阶魔方重新涂了颜色,如下:
前面:橙色
右面:绿色
上面:黄色
左面:绿色
下面:橙色
后面:黄色
请你计算一下,这样的魔方被打乱后,一共有多少种不同的状态。
如果两个状态经过魔方的整体旋转后,各个面的颜色都一致,则认为是同一状态。
请提交表示状态数的整数,不要填写任何多余内容或说明文字。
分析:
做出此题,直接国一
此题简直神仙难度,官方给的题解是枚举出所有情况。
首先用字符串表示当前状态,用BFS+队列进行操作。
我怕我电脑跑死,大家自己观摩下蓝桥官方给的代码吧。
代码:
满分
package JB2017;
/*
10 11
9 8
----------
20 21
23 22
----------
8 9 | 12 13 | 0 1 | 4 5 | 8 9
11 10 | 15 14 | 3 2 | 7 6 | 11 10
----------
16 17
19 18
----------
10 11
9 8
中心是前面
5
3 0 1 2
4
*/
import java.util.*;
class MFState
{
public int a;
public int b;
public int c;
public MFState(char[] x){
for(int i=0; i<8; i++){
a = a * 10 + (x[i]-'0');
}
for(int i=8; i<16; i++){
b = b * 10 + (x[i]-'0');
}
for(int i=16; i<24; i++){
c = c * 10 + (x[i]-'0');
}
}
public int hashCode(){
return a*600*600 + b*600 + c;
}
public boolean equals(Object other){
if(other instanceof MFState == false) return false;
MFState m = (MFState)other;
return a==m.a && b==m.b && c==m.c;
}
public char[] getState(){
String s = String.format("%8d%8d%8d", a, b, c);
return s.toCharArray();
}
public String toString(){
return new String(getState());
}
}
public class D魔方状态
{
// 某个面顺时针旋转
static int[][][] ACT = {{{0,1,2,3},{4,17,14,23},{7,16,13,22}}, //前
{{8,9,10,11},{5,20,15,18},{6,21,12,19}}, //后
{{4,5,6,7},{1,21,11,17},{2,22,8,18}}, //右
{{12,13,14,15},{0,16,10,20},{3,19,9,23}}, //左
{{20,21,22,23},{0,12,8,4},{1,13,9,5}}, //上
{{16,17,18,19},{2,6,10,14},{3,7,11,15}}}; //下
// 同态变换
static int[][] SAME = new int[24][];
// 基本变换
static int[][] BASE = new int[9][];
///
// 用ACT 第k个,对a状态进行变换
static char[] f(char[] a, int k)
{
char[] b = new char[a.length];
for(int i=0; i<b.length; i++) b[i] = a[i];
for(int i=0; i<ACT[k].length; i++){
for(int j=0; j<ACT[k][i].length; j++){
b[ACT[k][i][(j+1)%ACT[k][i].length]] = a[ACT[k][i][j]];
}
}
return b;
}
// 返回恒等变换
static int[] t_id(){
int[] r = new int[24];
for(int i=0; i<r.length; i++) r[i] = i;
return r;
}
//ACT第k条转为变换
static int[] a_to_t(int k){
int[] b = new int[24];
for(int i=0; i<b.length; i++) b[i] = i;
for(int i=0; i<ACT[k].length; i++){
for(int j=0; j<ACT[k][i].length; j++){
b[ACT[k][i][(j+1)%ACT[k][i].length]] = ACT[k][i][j];
}
}
return b;
}
// 变换x与y的复合
static int[] t_t(int[] x, int[] y){
int[] r = new int[x.length];
for(int i=0; i<y.length; i++){
r[i] = x[y[i]];
}
return r;
}
//变换t,作用于a,返回变换结果
static char[] g(char[] a, int[] t)
{
char[] r = new char[a.length];
for(int i=0; i<t.length; i++){
r[i] = a[t[i]];
}
return r;
}
// 对状态a 进行同态变换,返回结果
static char[][] tong(char[] a){
char[][] aa = new char[SAME.length][];
for(int i=0; i<SAME.length; i++){
aa[i] = g(a,SAME[i]);
}
return aa;
}
// 执行一系列ACT,生成等效变换,
static int[] act_to_t(int[] act){
int[] r = t_id();
for(int i=0; i<act.length; i++){
r = t_t(r,a_to_t(act[i]));
}
return r;
}
static {
// 24种同态
SAME[0] = act_to_t(new int[]{});
SAME[1] = act_to_t(new int[]{4,5,5,5});
SAME[2] = act_to_t(new int[]{4,4,5,5});
SAME[3] = act_to_t(new int[]{4,4,4,5});
SAME[4] = act_to_t(new int[]{2,3,3,3});
SAME[5] = act_to_t(new int[]{2,3,3,3,0,1,1,1});
SAME[6] = act_to_t(new int[]{2,3,3,3,0,0,1,1});
SAME[7] = act_to_t(new int[]{2,3,3,3,0,0,0,1});
SAME[8] = act_to_t(new int[]{2,2,3,3});
SAME[9] = act_to_t(new int[]{2,2,3,3,4,5,5,5});
SAME[10] = act_to_t(new int[]{2,2,3,3,4,4,5,5});
SAME[11] = act_to_t(new int[]{2,2,3,3,4,4,4,5});
SAME[12] = act_to_t(new int[]{2,2,2,3});
SAME[13] = act_to_t(new int[]{2,2,2,3,0,1,1,1});
SAME[14] = act_to_t(new int[]{2,2,2,3,0,0,1,1});
SAME[15] = act_to_t(new int[]{2,2,2,3,0,0,0,1});
SAME[16] = act_to_t(new int[]{0,1,1,1});
SAME[17] = act_to_t(new int[]{0,1,1,1,3,2,2,2});
SAME[18] = act_to_t(new int[]{0,1,1,1,3,3,2,2});
SAME[19] = act_to_t(new int[]{0,1,1,1,3,3,3,2});
SAME[20] = act_to_t(new int[]{0,0,0,1});
SAME[21] = act_to_t(new int[]{0,0,0,1,3,2,2,2});
SAME[22] = act_to_t(new int[]{0,0,0,1,3,3,2,2});
SAME[23] = act_to_t(new int[]{0,0,0,1,3,3,3,2});
//9种转法
BASE[0] = act_to_t(new int[]{2});
BASE[1] = act_to_t(new int[]{2,2});
BASE[2] = act_to_t(new int[]{2,2,2});
BASE[3] = act_to_t(new int[]{0});
BASE[4] = act_to_t(new int[]{0,0});
BASE[5] = act_to_t(new int[]{0,0,0});
BASE[6] = act_to_t(new int[]{4});
BASE[7] = act_to_t(new int[]{4,4});
BASE[8] = act_to_t(new int[]{4,4,4});
}
public static void main(String[] args)
{
//char[] a = "111111113333444411111111".toCharArray();
//char[] a = "111122223333222211113333".toCharArray();
char[] a = "111122223333444455556666".toCharArray();
Set set = new HashSet();
set.add(new MFState(a));
for(int w=0; w<12; w++){
Set set2 = new HashSet();
for(Object obj: set){
MFState it = (MFState)obj;
L1: for(int i=0; i<BASE.length; i++){
char[] ag = g(it.getState(), BASE[i]);
char[][] ag_tong = tong(ag);
for(int j=0; j<ag_tong.length; j++){
if(set.contains(new MFState(ag_tong[j]))) continue L1;
if(set2.contains(new MFState(ag_tong[j]))) continue L1;
}
set2.add(new MFState(ag_tong[0]));
// if(set.contains(ag)) continue;
// if(set2.contains(ag)) continue;
// set2.add(new MFState(ag));
}
}
if(set2.isEmpty()) break;
System.out.print("+ " + set2.size());
set.addAll(set2);
System.out.println("= " + set.size());
}
System.out.println("final: " + set.size());
}
}
E:取数位(难度:★)
求1个整数的第k位数字有很多种方法。
以下的方法就是一种。
public class Main
{
static int len(int x){
if(x<10) return 1;
return len(x/10)+1;
}
// 取x的第k位数字
static int f(int x, int k){
if(len(x)-k==0) return x%10;
return ______________________; //填空
}
public static void main(String[] args)
{
int x = 23513;
//System.out.println(len(x));
System.out.println(f(x,3));
}
}
对于题目中的测试数据,应该打印5。
请仔细分析源码,并补充划线部分所缺少的代码。
注意:只提交缺失的代码,不要填写任何已有内容或说明性的文字。
分析:
题目中需要填空的位置明显需要进行一次递归操作。
因为空格之前执行的语句让我们判断出len(x)是大于k的,
所以k不变,x删除个位。
空格内容为 f(x/10,k)
代码:
满分
package JB2017;
public class E取数位
{
static int len(int x){
if(x<10) return 1;
return len(x/10)+1;
}
// 取x的第k位数字
static int f(int x, int k){
if(len(x)-k==0) return x%10;
// return ______________________; //填空
return f(x/10,k);
}
public static void main(String[] args)
{
int x = 23513;
//System.out.println(len(x));
System.out.println(f(x,3));
}
}
F:最大公共子串(难度:★★)
最大公共子串长度问题就是:
求两个串的所有子串中能够匹配上的最大长度是多少。
比如:“abcdkkk” 和 “baabcdadabc”,
可以找到的最长的公共子串是"abcd",所以最大公共子串长度为4。
下面的程序是采用矩阵法进行求解的,这对串的规模不大的情况还是比较有效的解法。
请分析该解法的思路,并补全划线部分缺失的代码。
public class Main
{
static int f(String s1, String s2)
{
char[] c1 = s1.toCharArray();
char[] c2 = s2.toCharArray();
int[][] a = new int[c1.length+1][c2.length+1];
int max = 0;
for(int i=1; i<a.length; i++){
for(int j=1; j<a[i].length; j++){
if(c1[i-1]==c2[j-1]) {
a[i][j] = __________________; //填空
if(a[i][j] > max) max = a[i][j];
}
}
}
return max;
}
public static void main(String[] args){
int n = f("abcdkkk", "baabcdadabc");
System.out.println(n);
}
}
注意:只提交缺少的代码,不要提交已有的代码和符号。也不要提交说明性文字。
分析:
这是一个典型的动态规划问题,
填空处我们需要填写一个数据用来保证当前位置状况下,最大公共子串的长度。
由条件可以很明显的得出,c1[i-1]==c2[j-1],
即我们需要由上一个状态的最大公共子串长度+1获得。
代码:
满分
package JB2017;
public class F最大公共子串
{
static int f(String s1, String s2)
{
char[] c1 = s1.toCharArray();
char[] c2 = s2.toCharArray();
int[][] a = new int[c1.length+1][c2.length+1];
int max = 0;
for(int i=1; i<a.length; i++){
for(int j=1; j<a[i].length; j++){
if(c1[i-1]==c2[j-1]) {
// a[i][j] = __________________; //填空
a[i][j]=a[i-1][j-1]+1;
if(a[i][j] > max) max = a[i][j];
}
}
}
return max;
}
public static void main(String[] args){
int n = f("abcdkkk", "baabcdadabc");
System.out.println(n);
}
}
G:日期问题(难度:★)
小明正在整理一批历史文献。这些历史文献中出现了很多日期。小明知道这些日期都在1960年1月1日至2059年12月31日。令小明头疼的是,这些日期采用的格式非常不统一,有采用年/月/日的,有采用月/日/年的,还有采用日/月/年的。更加麻烦的是,年份也都省略了前两位,使得文献上的一个日期,存在很多可能的日期与其对应。
比如02/03/04,可能是2002年03月04日、2004年02月03日或2004年03月02日。
给出一个文献上的日期,你能帮助小明判断有哪些可能的日期对其对应吗?
输入
一个日期,格式是"AA/BB/CC"。 (0 <= A, B, C <= 9)
输入
输出若干个不相同的日期,每个日期一行,格式是"yyyy-MM-dd"。多个日期按从早到晚排列。
样例输入
c
样例输出
2002-03-04
2004-02-03
2004-03-02
资源约定:
峰值内存消耗(含虚拟机) < 256M
CPU消耗 < 1000ms
请严格按要求输出,不要画蛇添足地打印类似:“请您输入…” 的多余内容。
所有代码放在同一个源文件中,调试通过后,拷贝提交该源码。
不要使用package语句。不要使用jdk1.7及以上版本的特性。
主类的名字必须是:Main,否则按无效代码处理。
分析:
这个还是相对而言比较简单的。
首先录入数据,将三个数字按照题目所给的三种顺序进行排列,
对每种排列进行check,符合要求即输出。
代码:
满分
package JB2017;
import java.util.Scanner;
public class G日期问题 {
public static void one(int y,int m,int d){
int year=y+(y<60?2000:1900);
month[1]=((year%4==0&&year%100!=0)||year%400==0)?29:28;
if(m<13&&m>0&&d>0&&d<=month[m-1]){
System.out.println(year+"-"+(m<10?"0"+m:m)+"-"+(d<10?"0"+d:d));
}
}
public static int month[]={31,28,31,30,31,30,31,31,30,31,30,31};
public static void main(String[] args) {
Scanner sc=new Scanner(System.in);
String s=sc.next();
String ss[]=s.split("/");
int arr[]=new int[3];
for(int i=0;i<3;i++){
arr[i]=Integer.valueOf(ss[i]);
}
one(arr[0],arr[1],arr[2]);
one(arr[2],arr[0],arr[1]);
one(arr[2],arr[1],arr[0]);
}
}
H:包子凑数(难度:★★★★)
小明几乎每天早晨都会在一家包子铺吃早餐。他发现这家包子铺有N种蒸笼,其中第i种蒸笼恰好能放Ai个包子。每种蒸笼都有非常多笼,可以认为是无限笼。
每当有顾客想买X个包子,卖包子的大叔就会迅速选出若干笼包子来,使得这若干笼中恰好一共有X个包子。比如一共有3种蒸笼,分别能放3、4和5个包子。当顾客想买11个包子时,大叔就会选2笼3个的再加1笼5个的(也可能选出1笼3个的再加2笼4个的)。
当然有时包子大叔无论如何也凑不出顾客想买的数量。比如一共有3种蒸笼,分别能放4、5和6个包子。而顾客想买7个包子时,大叔就凑不出来了。
小明想知道一共有多少种数目是包子大叔凑不出来的。
输入
第一行包含一个整数N。(1 <= N <= 100)
以下N行每行包含一个整数Ai。(1 <= Ai <= 100)
输出
一个整数代表答案。如果凑不出的数目有无限多个,输出INF。
例如,
输入:
2
4
5
程序应该输出:
6
再例如,
输入:
2
4
6
程序应该输出:
INF
样例解释:
对于样例1,凑不出的数目包括:1, 2, 3, 6, 7, 11。
对于样例2,所有奇数都凑不出来,所以有无限多个。
资源约定:
峰值内存消耗(含虚拟机) < 256M
CPU消耗 < 1000ms
分析:
很多人说这是一个DP,但我偏说他是一个筛法,
思路很简单,根据已有的笼的包子树,不断叠加十万次。
如果答案不是INF,则在十万次前后一百次中必定全部可以凑到。
如果周围一百次不可以凑到,那么就说明答案是INF。
代码:
=满分
package JB2017;
import java.util.Scanner;
public class H包子凑数 {
public static void main(String[] args) {
Scanner sc=new Scanner(System.in);
int n=sc.nextInt();
int count=0;
boolean ans[]=new boolean[100001];
ans[0]=true;
for(int i=0;i<n;i++){
ans[sc.nextInt()]=true;
}
for(int i=1;i<100001;i++){
for(int j=1;j<101&&j<=i;j++){
if(ans[j]&&ans[i-j]){
ans[i]=true;
count++;
break;
}
}
}
boolean flag=false;
for(int i=99900;i<100001;i++){
if(!ans[i]){
flag=true;
}
}
if(flag)System.out.println("INF");
else{
System.out.println(100000-count);
}
}
}
I:分巧克力(难度:★★★★)
儿童节那天有K位小朋友到小明家做客。小明拿出了珍藏的巧克力招待小朋友们。
小明一共有N块巧克力,其中第i块是Hi x Wi的方格组成的长方形。
为了公平起见,小明需要从这 N 块巧克力中切出K块巧克力分给小朋友们。切出的巧克力需要满足:
- 形状是正方形,边长是整数
- 大小相同
例如一块6x5的巧克力可以切出6块2x2的巧克力或者2块3x3的巧克力。
当然小朋友们都希望得到的巧克力尽可能大,你能帮小Hi计算出最大的边长是多少么?
输入
第一行包含两个整数N和K。(1 <= N, K <= 100000)
以下N行每行包含两个整数Hi和Wi。(1 <= Hi, Wi <= 100000)
输入保证每位小朋友至少能获得一块1x1的巧克力。
输出
输出切出的正方形巧克力最大可能的边长。
样例输入:
2 10
6 5
5 6
样例输出:
2
资源约定:
峰值内存消耗(含虚拟机) < 256M
CPU消耗 < 1000ms
分析:
这是一道明显的二分题目,
我们只要二分期间不断地check,不断地缩小区间范围,并确定某一个值就可得解。
代码:
满分
package JB2017;
import java.util.Scanner;
public class I分巧克力 {
public static int arr[][],n;
public static long check(int k){
long ans=0;
for(int i=0;i<n;i++){
ans+=(arr[i][0]/k)*(arr[i][1]/k);
}
return ans;
}
public static void main(String[] args) {
Scanner sc=new Scanner(System.in);
n=sc.nextInt();
int k=sc.nextInt();
arr=new int [n][2];
for(int i=0;i<n;i++){
arr[i][0]=sc.nextInt();
arr[i][1]=sc.nextInt();
}
int bit[]=new int[20];
bit[0]=1;
for(int i=1;i<20;i++){
bit[i]=bit[i-1]*2;
}
int ans=bit[19];
int p=18;
long a=check(ans),b=check(ans+1);
while(!(a>=k&&b<k)){
if(a>=k&&b>=k){
ans+=bit[p];
}else if(a<k&&b<k){
ans-=bit[p];
}
p--;
a=check(ans);
b=check(ans+1);
}
System.out.println(ans);
}
}
J:K倍区间(难度:★★★★★)
这个题目后续更新或者放在交流群里。