刷题记录Day16-二叉树（二叉树的最大最小深度、完全二叉树的节点个数）

刷题记录Day16-二叉树（二叉树的最大最小深度、完全二叉树的节点个数）

---

---

前言

题目来源：leetcode
刷题顺序：代码随想录
刷题工具：VSCode+leetcode插件
补充：延毕时间充裕，会结合LeetCode 101: A LeetCode Grinding Guide (C++ Version)相似题目一起做。

---

一、二叉树的最大深度

1. 104二叉树的最大深度

这道题在前一篇文章中用层序遍历做过一次 这次使用后序递归遍历做

题目：
给定一个二叉树 root ，返回其最大深度。
二叉树的 最大深度 是指从根节点到最远叶子节点的最长路径上的节点数。

示例：

输入：root = [3,9,20,null,null,15,7]
输出：3

代码：

class Solution {
public:
    int maxDepth(TreeNode* root) {
    //采取后序遍历递归的方法
        if (root == NULL) return 0;
        int leftHeight = maxDepth(root->left); //左
        int rightHeight = maxDepth(root->right);  //右
        int height = 1 + max(leftHeight, rightHeight);  //中
        return height;
    }
};

2. 559n叉树的最大深度

题目：
给定一个 N 叉树，找到其最大深度。
最大深度是指从根节点到最远叶子节点的最长路径上的节点总数。
N 叉树输入按层序遍历序列化表示，每组子节点由空值分隔（请参见示例）。

示例：

输入：root = [1,null,3,2,4,null,5,6]
输出：3

代码：

class Solution {
public:
    int maxDepth(Node* root) {
        if (root == NULL) return 0;
        int height = 0;
        for (auto node : root->children){
            height = max(height,maxDepth(node));
        }
        return 1+height;
    }
};

二、二叉树最小深度

1. 111最小深度

这道题也在昨天练习中用层序遍历做过了 今天用后序递归迭代再做一次

题目：

给定一个二叉树，找出其最小深度。
最小深度是从根节点到最近叶子节点的最短路径上的节点数量。
说明：叶子节点是指没有子节点的节点。

示例：

输入：root = [3,9,20,null,null,15,7]
输出：2

代码：

class Solution {
public:
    int minDepth(TreeNode* root) {
        if (root == NULL) return 0;
        int leftHeight = minDepth(root->left);
        int rightHeight = minDepth(root->right);
        if (root->left == NULL && root->right != NULL){
            return 1+rightHeight;
        }
        if (root->right == NULL && root->left != NULL){
            return 1+leftHeight;
        }
        int height = 1 + min(leftHeight,rightHeight);
        return height;
    }
};

三、 完全二叉树的节点个数

1. 222完全二叉树的节点个数

题目：
给你一棵 完全二叉树 的根节点 root ，求出该树的节点个数。
完全二叉树 的定义如下：在完全二叉树中，除了最底层节点可能没填满外，其余每层节点数都达到最大值，并且最下面一层的节点都集中在该层最左边的若干位置。若最底层为第 h 层，则该层包含 1~ 2h 个节点。

示例：

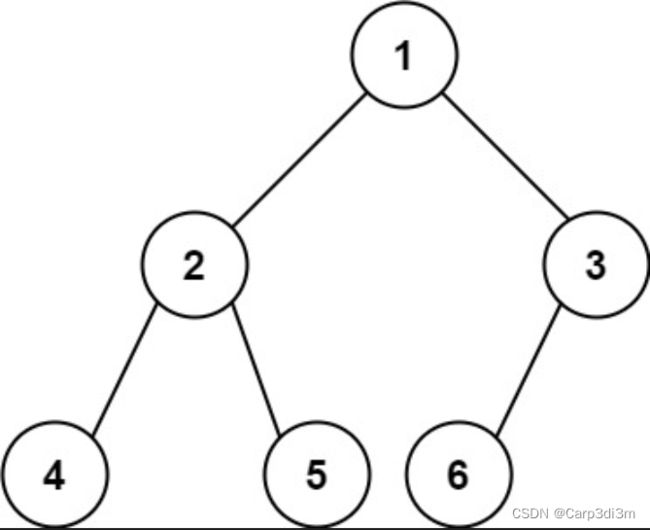
输入：root = [1,2,3,4,5,6]
输出：6

代码：

方法一，后序遍历递归

class Solution {
public:
    int countNodes(TreeNode* root) {
        if (root == NULL) return 0;
        int lcount = countNodes(root->left);
        int rcount = countNodes(root->right);
        int count = 1 + lcount + rcount;
        return count;
    }
};

方法二，层序遍历

class Solution {
public:
    int countNodes(TreeNode* root) {
        int res = 0;
        queue<TreeNode*> que;
        if (root != NULL) que.push(root);
        while (!que.empty()){
            int size = que.size();
            while (size--){
                TreeNode* node = que.front();
                que.pop();
                ++res;
                if (node->left) que.push(node->left);
                if (node->right) que.push(node->right);
            }
        }
        return res;
    }
};

方法三，基于完全二叉树的性质
如果左深度等于右深度，说明子树是一个满二叉树，那就可以直接返回2^深度-1。
如果不相等，则遍历下一层的左右子树。
相当于增加了迭代终止条件。

class Solution {
public:
    int countNodes(TreeNode* root) {
        if(root == NULL) return 0;
        TreeNode* leftNode = root->left;
        TreeNode* rightNode = root->right;
        int leftHeight =0, rightHeight = 0;
        while (leftNode){
            leftNode = leftNode->left;
            ++leftHeight;
        }
        while (rightNode){
            rightNode = rightNode->right;
            ++rightHeight;
        }
        if (leftHeight == rightHeight){
            return (2 << leftHeight)-1;
        }
        return countNodes(root->left)+countNodes(root->right)+1;
    }
};

---

总结

感觉二叉树最重要的地方在于掌握递归和迭代，尤其是递归很多地方比较难理解。