【东华大学oj】18 长方形?（面向对象）

18 长方形?

作者: Turbo时间限制: 1S章节: 类与对象

问题描述 :

实验目的：学习友元的使用。

实验内容：

定义一个Point类，包括两个私有成员：int x, int y，它们分别表示一个点的x和y座标。

再定义构造函数：

Point(int x, int y)，即传两个参数，构造一个点对象。

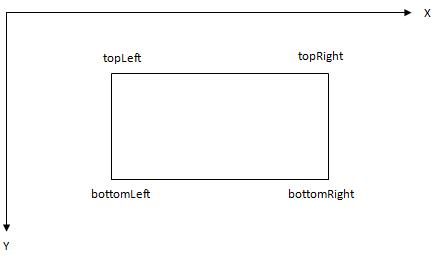
定义一个Rectangle类，包括4个私有成员：Point topLeft, topRight,bottomLeft, bottomRight，它们分别表示长方形4个顶点的座标。

注意：在计算机系统里，座标系如下定义：屏幕的左上角的座标是（0,0），x轴是横轴，屏幕的最右端x值最大，y轴是纵轴，屏幕的最下方y值最大。图如下：

再定义构造函数：

Rectangle(Point topLeft, Point topRight, Point bottomLeft, Point bottomRight)

以及实例方法：

bool isRectangle()  //判断4个顶点构成的图形是不是长方形，是则返回true，否则返回false。这个方法只在本类中调用，所以可声明为private的。

int getArea()    //如果是长方形则返回长方形的面积，否则返回0

bool isIn(Point p)   //如果是长方形则判断传入的点是否在该图形之内（不包括边界），如果在内部返回true，不在内部则返回false。如果不是长方形，则一律返回false。

说明：由于在Rectangle类中有大量的语句需要使用到Point类的私有成员x和y，因此，使用友元可直接访问x和y，从而可减少编程中的麻烦。

使用main函数测试以上getArea方法和isIn方法。main函数可参考如下代码：

int main()
{
   int topLeftX, topLeftY, topRightX, topRightY, bottomLeftX, bottomLeftY, bottomRightX, bottomRightY;
   int px, py;

   cin >> topLeftX >> topLeftY >> topRightX >> topRightY >> bottomLeftX >> bottomLeftY >> bottomRightX >> bottomRightY;
   cin >> px >> py;

   Point p(px, py);
   Rectangle r(Point(topLeftX, topLeftY), Point(topRightX, topRightY), Point(bottomLeftX, bottomLeftY), Point(bottomRightX, bottomRightY));
   cout << r.getArea() << endl;
   if (r.isIn(p))
        cout << "In" << endl;
   else
        cout << "Not in" << endl;

   return 0;
}

输入说明 :

第一行输入长方形r的信息，包括四个顶点x座标及y座标，顶点的输入顺序为左上、右上、左下、右下。

第二行输入一个点p的信息，包括其x座标和y座标。

所有输入都为非负整数，之间以一个空格分隔。无多余空格或空行。

输出说明 :

输出两行，第一行输出长方形面积，第二行输出点p是否位于长方形r之内，如果在内部，则输出“In”，否则输出“Not in”。

#include 
#include 
#include 

using namespace std;

class Point
{
private :
    int x,y;
public :
    Point(int x,int y):x(x),y(y) {}
    int getx()
    {
        return x;
    }
    int gety()
    {
        return y;
    }
    friend class Rectangle;
};

class Rectangle
{
private:
    Point topLeft,topRight,bottomLeft, bottomRight;
    bool isRectangle()
    {
        if(topLeft.getx()==bottomLeft.getx()&&
           topRight.getx()==bottomRight.getx()&&
                topLeft.gety()==topRight.gety()&&
           bottomLeft.gety()==bottomRight.gety()&&
                topLeft.getx()topLeft.getx()&&p.gety()>topLeft.gety()&&p.gety()> topLeftX >> topLeftY >> topRightX >> topRightY >> bottomLeftX >> bottomLeftY >> bottomRightX >> bottomRightY;
   cin >> px >> py;

   Point p(px, py);
   Rectangle r(Point(topLeftX, topLeftY), Point(topRightX, topRightY), Point(bottomLeftX, bottomLeftY), Point(bottomRightX, bottomRightY));
   cout << r.getArea() << endl;
   if (r.isIn(p))
        cout << "In" << endl;
   else
        cout << "Not in" << endl;

   return 0;
}