- 贪心算法----区间选点问题(POJ1201)
苦茶子12138
贪心算法java算法
题目:正在上传…重新上传取消题目的大致意思是,给定n个闭区间,并且这个闭区间上的点都是整数,现在要求你使用最少的点来覆盖这些区间并且每个区间的覆盖的点的数量满足输入的要求点覆盖区间的数量。输入:第一行输入n,代表n个区间。接下来的n行每行的第一个数代表区间起点,第二个数代表区间终点,第三个数代表这个区间必须要选取的点的数量。输出:输出最少的点的数量,这些最少的点要覆盖全部区间。这个题是区间选点问题
- Lombok 在 IntelliJ IDEA 中的使用步骤
阿乾之铭
intellij-ideajavaide
Lombok是一个非常流行的Java库,它通过注解简化Java类的开发,特别是在处理POJO(PlainOldJavaObjects)类时,如生成getter、setter、toString等常用方法。Lombok在减少样板代码(boilerplatecode)方面非常有用。在IntelliJIDEA中使用Lombok插件,可以极大简化开发过程。1.安装Lombok插件要在IntelliJIDEA
- 2025java面试常见八股文整理
Java八股文面试
面试职场和发展javaspringbootjvmspringspringcloud
1.多线程编程下,怎么解决线程的数据安全问题?如果线程存在竞争临界资源,多线程访问下添加同步代码块synchronized解决,或者分布式排他锁进行临界资源控制。在分布式多线程环境下,线程的数据安全尽量不要产生连接资源,使用线程本地化ThreadLocal实现线程资源隔离。2.SpringIOC依赖注入怎么理解,spring有几种方式属性注入,setter构建pojo实体类和有参构造方法工厂方法注
- java 快速生成javaBean类
angen2018
javajava
packagecom.angen.util;importjava.io.FileWriter;importjava.io.IOException;importjava.sql.*;importjava.util.ArrayList;importjava.util.List;/***@description:PojoUtil*@date:2022/7/20*/publicclassPojoUtil{
- 详解mybatis的一二级缓存以及缓存失效原因
仰望天花板
缓存数据库mybatisjavamysql
数据库的大部分场景下是从磁盘读取,如果数据从内存进行读取,速度较比磁盘要快得多。但因为内存的容量有限,所以一般只会把使用和查询较多的数据缓存起来,以便快速反应,其他使用率不太多的继续存放在磁盘。mybatis分为一级缓存和二级缓存1.一级缓存一级缓存存放在SqlSqeeion上,默认开启1.1pojo@DatapublicclassRole{privateLongid;privateStringr
- WORD批量转换器MultiDoc Converter
uolian
工作word
WORD批量转换器MultiDocConverterhttps://www.52pojie.cn/thread-1318745-1-1.html可批量将doc、docx等文件格式转成doc、docx、pdf、rtf、txt、html、epub等格式。安装包下载地址:https://wws.lanzouj.com/irvVbiz0pkd最终下载文件打包地址(未作成单文件,不确定是否可以直接使用):h
- MyBatis系统学习(一)——项目结构及其含义
OEC小胖胖
MyBatismybatis学习web后端
1.MyBatis简介MyBatis是一款优秀的持久层框架,它通过SQL映射的方式实现Java对数据库操作的映射,既保留了SQL语句的灵活性,也简化了代码的编写。在一个MyBatis项目中,核心部分主要有:配置文件(mybatis-config.xml)映射文件(Mapper.xml)实体类(Entity/POJO)接口类(Mapper接口)MyBatis会话工厂(SqlSessionFactor
- SpringBoot项目
俺叫啥好嘞
spring系列springspringboot
SpringBoot项目大概分为四层:(1)DAO层:包括XxxMapper.java(数据库访问接口类),XxxMapper.xml(数据库链接实现);(这个命名,有人喜欢用Dao命名,有人喜欢用Mapper,看个人习惯了吧)(2)Bean层:也叫model层,模型层,entity层,实体层,就是数据库表的映射实体类,存放POJO对象;(3)Service层:也叫服务层,业务层,包括XxxSer
- 数据结构OJ作业——队列
nnbs
数据结构数据结构poj队列
POJ3984:http://poj.org/problem?id=3984迷宫,输出最短路径,bfs#include#include#include#includeusingnamespacestd;intmaze[5][5];pairpath[5][5];queue>q;intdx[]={1,-1,0,0};intdy[]={0,0,1,-1};voidbfs(intx,inty){q.pus
- c语言练习:POJ 1005 我想我需要一艘船屋(I Think I Need a Houseboat)
七月初七淮水竹亭~
C语言入门c语言
题目相关信息描述弗雷德·马珀(FredMapper)正在考虑在路易斯安那州购买一些土地来建造他的房子。在调查这片土地的过程中,他了解到,由于密西西比河造成的侵蚀,路易斯安那州实际上每年都在缩小50平方英里。由于弗雷德希望一辈子都住在这所房子里,他需要知道他的土地是否会因侵蚀而消失。在做了更多的研究之后,弗雷德了解到正在失去的土地形成了一个半圆形。这个半圆是以(0,0)为中心的圆的一部分,将圆平分的
- java开发中pojo、model和entity的区别及DTO与VO
leighy
javaspringbootmvc
一、pojo(PlainOrdinaryJavaObject无规则简单Java对象)简单java对象简单的javabean的对象,对应数据库某一张表,表的字段与pojo类的属性都要一一对应?(查阅发现没有具体对pojo描述,有的说是作为业务协作类不需要一一对应)但在实际开发中较少以pojo来对包命名。二、entity(实体类)数据表对应到实体类的映射则类属性与数据库表字段一一对应在实际开发中较多以
- SpringData JPA之Respository接口的使用
OVA_Won
SpringDatamysqljavaspring
SpringDataJPA之Respository接口的使用Respository:最顶层的接口也是标志接口,目的是为了统一所有Repository的类型,且能让组件扫描的时候自动识别。准备工作导入JAR包:别忘了导入Junit测试包,否则后面没法单元测试编写Spring和数据库配置文件applicationContext.xml文件com.OVA.pojojdbc.propertiesjdbc.
- POJ 1062 : 昂贵的聘礼 - 最短路Dijkstra+枚举(难)
bookybooky
图论最短路Dijsktrapojzoj图论
dijkstra处理权值非负情形,最近才开始看最短路。题目大意:(中文题容易理解)大致就是说,最终要得到酋长的许诺,每件物品可能有其他物品(1件)能让此物品价格优惠,你可通过交易获得物品从而以最少金钱达到酋长许诺。交易受到“等级限制”。其中的等级限制处理需要一定的技巧,细节一定要处理好!输入:(单Case输入)第一行两个整数M,N(1>30)-1足够,邻接矩阵用int也足够,并不像DISCUSS中
- 【Mybatis】Web中的数据库操作
科马
java数据库mybatisservletsql
Mybatis工作机制1.加载配置文件:2.创建SqlSessionFactory:3.获取SqlSession:4.获取Mapper接口:5.执行SQL语句:6.SQL语句解析和执行:7.结果映射:8.事务管理:9.关闭SqlSession:10.返回结果:与JDBC对比三个显著特点代码示例1.配置MyBatis2.创建数据库表3.创建POJO类4.编写Mapper接口5.编写SQL映射文件6.
- spring揭秘-概念以BeanFactory介绍
liangxifeng833
本质:Spring框架为POJO提供的各种服务共同组成了Spring的生命之树Paste_Image.pngspring框架为基础,有很多家庭成员,比如(SpringWebFlow,SpringWebServices,SpringSecurity,SpringBatch等等),这些家族成员全部以ApacheLisenceVersion2.0协议发布,共同组成了SpringProjects组合,因为
- SpringBoot项目中使用SpringData-JPA持久化数据
火星刻苦的杏仁
springbootjavamybatis
目录一、什么是JPA二、SpringData-JPA与MybatisPuls的区别三、项目中使用JPAJPA常用注解JPA进阶使用技巧一、什么是JPAJPA是JavaPersistenceAPI的简称,中文名Java持久层API,其设计目标主要是为了简化现有的持久化开发工作和整合ORM技术。简单地说,JPA就是为POJO(PlainOrdinaryJavaObject)提供持久化的标准规范,即将J
- 算法入门篇(八) 之 查找算法
战族狼魂
算法哈希算法
目录一、哈希表哈希函数哈希函数的应用常见的哈希函数线性探测、二次探测、链地址1.线性探测(LinearProbing)2.二次探测(QuadraticProbing)3.链地址法(Chaining)4.总结POJ3349、POJ1840、POJ2002POJ3349-AncientCipherPOJ1840-MaximumNumberPOJ2002-TournamentScheduling二、字符
- Spring AI
java_heartLake
人工智能人工智能springjava
本文介绍Spring的一级项目SpringAI简介:SpringAI是一个用于AI工程的应用程序框架。其目标是将Spring生态系统的设计原则(如可移植性和模块化设计)应用于AI领域,并推动将POJO(PlainOldJavaObjects,简单老式Java对象)作为应用程序的构建块引入到AI领域。主要特性:支持跨AI提供商的便携式API,用于聊天、文本到图像和嵌入模型。同时支持同步和流API选项
- 【iOS安全】iOS安装Alook浏览器
Jouzzy
iOS安全iOS开发ios浏览器
下载Alook的ipa下载地址参考:https://www.52pojie.cn/thread-1725470-1-1.html其中出现的下载链接:https://wwes.lanzouf.com/iyGQ00icergd下载ipa后使用爱思助手安装
- MyBatis注解开发详解
hoypte
mybatis
MyBatis注解开发详解一、前言MyBatis是一个优秀的持久层框架,它支持定制化SQL、存储过程以及高级映射。MyBatis免除了几乎所有的JDBC代码和手动设置参数以及获取结果集。MyBatis可以使用简单的XML或注解来配置和映射原始类型、接口和JavaPOJO(PlainOldJavaObjects,普通的Java对象)为数据库中的记录。在MyBatis中,注解提供了一种更加简洁和直观的
- @Import注解作用
y我见青山多妩媚
java开发语言
@Import注解作用理解springboot自动装配时,发现@SpringBootApplication注解下的@EnableAutoConfiguration注解头上有一个@Import注解。关于这个注解的作用,上网查找后发现理解的不是很明白,于是写了下面的Demo去理解。两个pojo类:publicclassPerson{}publicclassStudent{}测试类@Configurat
- 【spring boot结合rabbit mq 到点执行,可精确到秒】
ljh_learn_from_base
javaspringbootspringbootjava后端java-rabbitmq
【springboot结合rabbitmq到点执行,可精确到秒】创建队列枚举创建自定义的队列消息pojo创建队列和延迟队列发送mq消息接收mq消息DateTimeUtil测试注意点创建队列枚举publicenumQueueEnum{/***各种异步消息频道*/TEST(1,"test","队列频道"),DELAY_TEST(2,"delay_test","延迟延迟频道"),;privateInte
- Eclipse中Run As On Server和Run As Java Application
CAE虚拟与现实
JavaIDE开发环境javaeclipseide
一、名词释义runjavaapplication(作为Java应用程序运行)是运行javamain方法。runonserver是启动一个web应用服务器。二、两者的区别Eclipse中可以创建javaproject也可以创建javawebpoject。javaproject是可以直接在命令行运行,或者直接在Eclipse中运行此时运行是jvm直接调用了main方法。Eclipse中创建的javaw
- 不同编程网站应当注意的点
见合8
比赛总结c++c语言
文章目录引入:洛谷:POJ:C语言:C++:CF:个人建议:补充:引入:小伙伴们有没有遇到过这种情况:到一个新的网站去编程,思路、算法完全正确,提交上去却是WrongAnswer,RuntimeError,ComplieError,TimeLimitExceed。这里,我总结了以下几个网站的注意点:洛谷:link提交时右上角有一个选项为O2优化。这并不可以随便选。可能有些暴力程序开了O2就过了,但
- 阿里java开发手册泰山版_总结
林坰
Javajava数据库mysql
编程规约常量定义不允许任何预先未定义的常量出现在代码中。不要使用一个常量类维护所有的常量,要按常量功能进行归类,分开维护。OOP规约所有的POJO类属性必须使用包装数据类型。RPC方法的返回值和参数必须使用包装数据类型。POJO类是最简单最普通的JAVA对象,内在含义是有一些private的参数作为对象的属性,然后针对每一个参数定义get和set方法访问的接口。【没有从任何类继承、也没有实现任何接
- 【转载】ACM入门 .
dongfan1861
人工智能phpc/c++
初期:一.基本算法:(1)枚举.(poj1753,poj2965)(2)贪心(poj1328,poj2109,poj2586)(3)递归和分治法.(4)递推.(5)构造法.(poj3295)(6)模拟法.(poj1068,poj2632,poj1573,poj2993,poj2996)二.图算法:(1)图的深度优先遍历和广度优先遍历.(2)最短路径算法(dijkstra,bellman-ford,
- 如何下载52pojie、CSDN、简书、Myitmx、博客园的文章?(最新教程)
星梦客
问题记录程序人生
使用的油猴插件,具体怎么安装问一下度娘。我用的火狐,点点点就行了,省事先安装油猴拓展,启用一下Tampermonkey–下载Firefox扩展(zh-CN)在安装插件SaveToPDF脚本安装后,浏览CSDN文章时,博主信息下方会出现“收藏”按钮,52pojie页面,"收藏"按钮在发贴回复旁边,简书页面,左侧悬浮按钮下方,Myitmx页面,顶部最右侧,博客园页面,顶部管理菜单旁边点击收藏会弹出打印
- SpringBoot之基本概念
Daylight629
SpringBootjavaspringspringbootmaventomcat
SpringBoot概念一、什么是SpringSpring是一个开源框架,2003年兴起的一个轻量级的Java开发框架,作者:RodJohnson。Spring是为了解决企业级应用开发的复杂性而创建的,简化开发。可以看我之前的文章关于spring的介绍与使用二、Spring是如何简化Java开发的为了降低Java开发的复杂性,Spring采用了以下4种关键策略:1、基于POJO的轻量级和最小侵入性
- 使用IDEA构建微服务Maven聚合工程保姆级教程
程序喵锅
经验分享intellij-idea微服务maven经验分享学习
本次喵子给大家分享一个通用的聚合工程搭建教程,细致入微、充满人文关怀的保姆级教程,基本上搭建一次后续即可作为通用模版,应用到其它项目里。一、简单了解微服务二、Maven构建聚合工程1.环境说明2.开始构建2.1.创建空的父工程2.2.创建common子工程2.3.创建pojo子工程2.4.创建mapper子工程2.5.创建service子工程2.6.创建apis子工程2.7.工程安装三、总结一、简
- java中实体pojo对于布尔类型属性命名尽量别以is开头,否则 fastjson可能会导致属性读取不到
阿文弟
java开发语言
假如我们有一个场景,就是需要将一个对象以字符串的形式,也就是jsonString存到一个地方,比如mysql,或者redis的String结构。现在有一个实体,我们自己创建的,叫做CusPojo.java有两个属性是布尔类型的,一个属性是有is开头,一个是没有is开头的,我们就可以做个对比。现在我导入fastjson依赖,创建一个CusPojo对象,然后序列化为jsonString,我们打印结果如
- LeetCode[位运算] - #137 Single Number II
Cwind
javaAlgorithmLeetCode题解位运算
原题链接:#137 Single Number II
要求:
给定一个整型数组,其中除了一个元素之外,每个元素都出现三次。找出这个元素
注意:算法的时间复杂度应为O(n),最好不使用额外的内存空间
难度:中等
分析:
与#136类似,都是考察位运算。不过出现两次的可以使用异或运算的特性 n XOR n = 0, n XOR 0 = n,即某一
- 《JavaScript语言精粹》笔记
aijuans
JavaScript
0、JavaScript的简单数据类型包括数字、字符创、布尔值(true/false)、null和undefined值,其它值都是对象。
1、JavaScript只有一个数字类型,它在内部被表示为64位的浮点数。没有分离出整数,所以1和1.0的值相同。
2、NaN是一个数值,表示一个不能产生正常结果的运算结果。NaN不等于任何值,包括它本身。可以用函数isNaN(number)检测NaN,但是
- 你应该更新的Java知识之常用程序库
Kai_Ge
java
在很多人眼中,Java 已经是一门垂垂老矣的语言,但并不妨碍 Java 世界依然在前进。如果你曾离开 Java,云游于其它世界,或是每日只在遗留代码中挣扎,或许是时候抬起头,看看老 Java 中的新东西。
Guava
Guava[gwɑ:və],一句话,只要你做Java项目,就应该用Guava(Github)。
guava 是 Google 出品的一套 Java 核心库,在我看来,它甚至应该
- HttpClient
120153216
httpclient
/**
* 可以传对象的请求转发,对象已流形式放入HTTP中
*/
public static Object doPost(Map<String,Object> parmMap,String url)
{
Object object = null;
HttpClient hc = new HttpClient();
String fullURL
- Django model字段类型清单
2002wmj
django
Django 通过 models 实现数据库的创建、修改、删除等操作,本文为模型中一般常用的类型的清单,便于查询和使用: AutoField:一个自动递增的整型字段,添加记录时它会自动增长。你通常不需要直接使用这个字段;如果你不指定主键的话,系统会自动添加一个主键字段到你的model。(参阅自动主键字段) BooleanField:布尔字段,管理工具里会自动将其描述为checkbox。 Cha
- 在SQLSERVER中查找消耗CPU最多的SQL
357029540
SQL Server
返回消耗CPU数目最多的10条语句
SELECT TOP 10
total_worker_time/execution_count AS avg_cpu_cost, plan_handle,
execution_count,
(SELECT SUBSTRING(text, statement_start_of
- Myeclipse项目无法部署,Undefined exploded archive location
7454103
eclipseMyEclipse
做个备忘!
错误信息为:
Undefined exploded archive location
原因:
在工程转移过程中,导致工程的配置文件出错;
解决方法:
- GMT时间格式转换
adminjun
GMT时间转换
普通的时间转换问题我这里就不再罗嗦了,我想大家应该都会那种低级的转换问题吧,现在我向大家总结一下如何转换GMT时间格式,这种格式的转换方法网上还不是很多,所以有必要总结一下,也算给有需要的朋友一个小小的帮助啦。
1、可以使用
SimpleDateFormat SimpleDateFormat
EEE-三位星期
d-天
MMM-月
yyyy-四位年
- Oracle数据库新装连接串问题
aijuans
oracle数据库
割接新装了数据库,客户端登陆无问题,apache/cgi-bin程序有问题,sqlnet.log日志如下:
Fatal NI connect error 12170.
VERSION INFORMATION: TNS for Linux: Version 10.2.0.4.0 - Product
- 回顾java数组复制
ayaoxinchao
java数组
在写这篇文章之前,也看了一些别人写的,基本上都是大同小异。文章是对java数组复制基础知识的回顾,算是作为学习笔记,供以后自己翻阅。首先,简单想一下这个问题:为什么要复制数组?我的个人理解:在我们在利用一个数组时,在每一次使用,我们都希望它的值是初始值。这时我们就要对数组进行复制,以达到原始数组值的安全性。java数组复制大致分为3种方式:①for循环方式 ②clone方式 ③arrayCopy方
- java web会话监听并使用spring注入
bewithme
Java Web
在java web应用中,当你想在建立会话或移除会话时,让系统做某些事情,比如说,统计在线用户,每当有用户登录时,或退出时,那么可以用下面这个监听器来监听。
import java.util.ArrayList;
import java.ut
- NoSQL数据库之Redis数据库管理(Redis的常用命令及高级应用)
bijian1013
redis数据库NoSQL
一 .Redis常用命令
Redis提供了丰富的命令对数据库和各种数据库类型进行操作,这些命令可以在Linux终端使用。
a.键值相关命令
b.服务器相关命令
1.键值相关命令
&
- java枚举序列化问题
bingyingao
java枚举序列化
对象在网络中传输离不开序列化和反序列化。而如果序列化的对象中有枚举值就要特别注意一些发布兼容问题:
1.加一个枚举值
新机器代码读分布式缓存中老对象,没有问题,不会抛异常。
老机器代码读分布式缓存中新对像,反序列化会中断,所以在所有机器发布完成之前要避免出现新对象,或者提前让老机器拥有新增枚举的jar。
2.删一个枚举值
新机器代码读分布式缓存中老对象,反序列
- 【Spark七十八】Spark Kyro序列化
bit1129
spark
当使用SparkContext的saveAsObjectFile方法将对象序列化到文件,以及通过objectFile方法将对象从文件反序列出来的时候,Spark默认使用Java的序列化以及反序列化机制,通常情况下,这种序列化机制是很低效的,Spark支持使用Kyro作为对象的序列化和反序列化机制,序列化的速度比java更快,但是使用Kyro时要注意,Kyro目前还是有些bug。
Spark
- Hybridizing OO and Functional Design
bookjovi
erlanghaskell
推荐博文:
Tell Above, and Ask Below - Hybridizing OO and Functional Design
文章中把OO和FP讲的深入透彻,里面把smalltalk和haskell作为典型的两种编程范式代表语言,此点本人极为同意,smalltalk可以说是最能体现OO设计的面向对象语言,smalltalk的作者Alan kay也是OO的最早先驱,
- Java-Collections Framework学习与总结-HashMap
BrokenDreams
Collections
开发中常常会用到这样一种数据结构,根据一个关键字,找到所需的信息。这个过程有点像查字典,拿到一个key,去字典表中查找对应的value。Java1.0版本提供了这样的类java.util.Dictionary(抽象类),基本上支持字典表的操作。后来引入了Map接口,更好的描述的这种数据结构。
&nb
- 读《研磨设计模式》-代码笔记-职责链模式-Chain Of Responsibility
bylijinnan
java设计模式
声明: 本文只为方便我个人查阅和理解,详细的分析以及源代码请移步 原作者的博客http://chjavach.iteye.com/
/**
* 业务逻辑:项目经理只能处理500以下的费用申请,部门经理是1000,总经理不设限。简单起见,只同意“Tom”的申请
* bylijinnan
*/
abstract class Handler {
/*
- Android中启动外部程序
cherishLC
android
1、启动外部程序
引用自:
http://blog.csdn.net/linxcool/article/details/7692374
//方法一
Intent intent=new Intent();
//包名 包名+类名(全路径)
intent.setClassName("com.linxcool", "com.linxcool.PlaneActi
- summary_keep_rate
coollyj
SUM
BEGIN
/*DECLARE minDate varchar(20) ;
DECLARE maxDate varchar(20) ;*/
DECLARE stkDate varchar(20) ;
DECLARE done int default -1;
/* 游标中 注册服务器地址 */
DE
- hadoop hdfs 添加数据目录出错
daizj
hadoophdfs扩容
由于原来配置的hadoop data目录快要用满了,故准备修改配置文件增加数据目录,以便扩容,但由于疏忽,把core-site.xml, hdfs-site.xml配置文件dfs.datanode.data.dir 配置项增加了配置目录,但未创建实际目录,重启datanode服务时,报如下错误:
2014-11-18 08:51:39,128 WARN org.apache.hadoop.h
- grep 目录级联查找
dongwei_6688
grep
在Mac或者Linux下使用grep进行文件内容查找时,如果给定的目标搜索路径是当前目录,那么它默认只搜索当前目录下的文件,而不会搜索其下面子目录中的文件内容,如果想级联搜索下级目录,需要使用一个“-r”参数:
grep -n -r "GET" .
上面的命令将会找出当前目录“.”及当前目录中所有下级目录
- yii 修改模块使用的布局文件
dcj3sjt126com
yiilayouts
方法一:yii模块默认使用系统当前的主题布局文件,如果在主配置文件中配置了主题比如: 'theme'=>'mythm', 那么yii的模块就使用 protected/themes/mythm/views/layouts 下的布局文件; 如果未配置主题,那么 yii的模块就使用 protected/views/layouts 下的布局文件, 总之默认不是使用自身目录 pr
- 设计模式之单例模式
come_for_dream
设计模式单例模式懒汉式饿汉式双重检验锁失败无序写入
今天该来的面试还没来,这个店估计不会来电话了,安静下来写写博客也不错,没事翻了翻小易哥的博客甚至与大牛们之间的差距,基础知识不扎实建起来的楼再高也只能是危楼罢了,陈下心回归基础把以前学过的东西总结一下。
*********************************
- 8、数组
豆豆咖啡
二维数组数组一维数组
一、概念
数组是同一种类型数据的集合。其实数组就是一个容器。
二、好处
可以自动给数组中的元素从0开始编号,方便操作这些元素
三、格式
//一维数组
1,元素类型[] 变量名 = new 元素类型[元素的个数]
int[] arr =
- Decode Ways
hcx2013
decode
A message containing letters from A-Z is being encoded to numbers using the following mapping:
'A' -> 1
'B' -> 2
...
'Z' -> 26
Given an encoded message containing digits, det
- Spring4.1新特性——异步调度和事件机制的异常处理
jinnianshilongnian
spring 4.1
目录
Spring4.1新特性——综述
Spring4.1新特性——Spring核心部分及其他
Spring4.1新特性——Spring缓存框架增强
Spring4.1新特性——异步调用和事件机制的异常处理
Spring4.1新特性——数据库集成测试脚本初始化
Spring4.1新特性——Spring MVC增强
Spring4.1新特性——页面自动化测试框架Spring MVC T
- squid3(高命中率)缓存服务器配置
liyonghui160com
系统:centos 5.x
需要的软件:squid-3.0.STABLE25.tar.gz
1.下载squid
wget http://www.squid-cache.org/Versions/v3/3.0/squid-3.0.STABLE25.tar.gz
tar zxf squid-3.0.STABLE25.tar.gz &&
- 避免Java应用中NullPointerException的技巧和最佳实践
pda158
java
1) 从已知的String对象中调用equals()和equalsIgnoreCase()方法,而非未知对象。 总是从已知的非空String对象中调用equals()方法。因为equals()方法是对称的,调用a.equals(b)和调用b.equals(a)是完全相同的,这也是为什么程序员对于对象a和b这么不上心。如果调用者是空指针,这种调用可能导致一个空指针异常
Object unk
- 如何在Swift语言中创建http请求
shoothao
httpswift
概述:本文通过实例从同步和异步两种方式上回答了”如何在Swift语言中创建http请求“的问题。
如果你对Objective-C比较了解的话,对于如何创建http请求你一定驾轻就熟了,而新语言Swift与其相比只有语法上的区别。但是,对才接触到这个崭新平台的初学者来说,他们仍然想知道“如何在Swift语言中创建http请求?”。
在这里,我将作出一些建议来回答上述问题。常见的
- Spring事务的传播方式
uule
spring事务
传播方式:
新建事务
required
required_new - 挂起当前
非事务方式运行
supports
&nbs
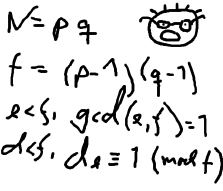 The young and very promising cryptographer Odd Even has implemented the security module of a large system with thousands of users, which is now in use in his company. The cryptographic keys are created from the product of two primes, and are believed to be secure because there is no known method for factoring such a product effectively.
The young and very promising cryptographer Odd Even has implemented the security module of a large system with thousands of users, which is now in use in his company. The cryptographic keys are created from the product of two primes, and are believed to be secure because there is no known method for factoring such a product effectively.

