- 奥林巴斯道Olympus DAO、奥拉丁模式、诺瓦银行、RWA模型合约解析开发
白马区块Crypto100
web3区块链区块链项目
关于OlympusDAO技术合约解析的文章草稿,整体结构偏向技术向,适合有一定DeFi或区块链背景的读者。你可以根据自己的需求微调。技术帮助“Crypto100”深入理解DeFi2.0的创新机制一、引言2021年,OlympusDAO凭借其颠覆性的机制和“协议拥有流动性”(Protocol-OwnedLiquidity,POL)概念引发了DeFi世界的巨大关注。它不是一个传统意义上的稳定币项目,而
- 国产信创AI IDE:开启智能编程新时代
InsCode AI IDE
国产信创AIIDE:开启智能编程新时代随着信息技术的迅猛发展,软件开发工具也在不断演进。近年来,人工智能(AI)技术的应用为编程工具带来了革命性的变化。其中,国产信创AIIDE——InsCodeAIIDE,作为一款由CSDN、GitCode和华为云CodeArtsIDE联合开发的新一代集成开发环境(IDE),以其智能化、高效化的特点,正在引领智能编程的新时代。最新接入DeepSeek-V3模型,点
- 如何用Python批量将CSV文件编码转换为UTF-8并转为Excel格式?
字节王德发
pythonpythonexcel开发语言
在处理数据时,CSV文件格式常常用作数据的交换格式。不过,很多情况下我们会遇到编码问题,特别是当文件不是UTF-8编码时。为了更好地处理这些文件,可能需要将它们转换为UTF-8编码,并且将其转换为Excel格式,这样可以方便后续的数据分析和使用。今天就来聊聊如何用Python实现这一过程。准备工作:安装必要的库我们需要确保安装了所需的Python库。主要用到的库有pandas和openpyxl。p
- 【产品小白】什么是AI产品经理
百事不可口y
产品经理的一步一步人工智能产品经理学习产品运营内容运营用户运营
一、AI产品经理的定义与角色定位AI产品经理是人工智能技术与商业应用之间的核心桥梁,负责将复杂的AI技术转化为满足市场需求的产品。需同时具备技术理解力、商业洞察力和用户思维,既要参与算法选型与数据建模,又要定义产品功能与市场策略,是贯穿产品全生命周期的关键角色。与传统互联网产品经理相比,AI产品经理的独特之处在于:技术深度参与:需理解机器学习、自然语言处理(NLP)、计算机视觉等技术原理,并参与数
- 【图像处理基石】什么是HDR图片?
AndrewHZ
AI算法工程师面试指北图像处理基石图像处理算法计算机视觉HDR高动态范围包围曝光opencv
1.什么是HDR图片?HDR(高动态范围图像,HighDynamicRange)是一种通过技术手段扩展照片明暗细节的成像方式。以下是关于HDR的详细说明:核心原理动态范围:指图像中最亮和最暗区域之间的亮度差。人眼能感知的动态范围远高于普通相机,HDR通过合成多张不同曝光的照片(如欠曝、正常、过曝),平衡高光和阴影细节。HDR的优势保留细节:避免强光下过曝(如天空发白)或阴影中欠曝(如暗部死黑)。增
- C语言中的函数
NaZiMeKiY
C/C++c语言linux开发语言
一.函数:1.函数的概念:函数就是程序中独立的功能2.函数的使用场景:我们需要反复书写的代码,但是又不确定什么时候会用到二.函数的定义和调用:①:函数的结构返回值类型函数名(形参1,形参2,...,形参n){函数体;return返回值;}②:举例:#includevoidstudy(){printf("准备开始学习\n");printf("打开b站\n");printf("打开自己想要学习的课程\
- 单片机中断系统
C_Xurn
单片机stm32
前言:中断系统是单片机非常重要的组成部分,是为了使单片机能够对外部或者内部随机发生的事件实时处理而设置的。一:单片机的概念一个高速主机和一个低俗外设连接时,效率极低,低俗外设工作时无端大量占用CPU时间。一个高速主机和多个低速外设连接时,高速主机无法进行多任务并行处理。此时需引入“中断”。CPU在处理某一事件A时,另一事件B发出请求(中断请求);CPU暂时中断当前的工作,转去处理事件B(中断响应和
- 普通鼠标的500连击的工具来了!!!
2501_90713682
计算机外设
今天介绍的这款软件叫:鼠标录制器,是一款大小只有54K的鼠标连点器,软件是绿色单文件版。抢票,拍牌,摇号都能用上。文末有分享链接在使用先我们先设置快捷键,这样我们在录制和停止录制的时候会更方便。软件使用很简单,首先是录制,点击【录制启停】或者按上面设置的快捷键,然后软件就开始录制。录制完成后在【名称】处,我们可以对此次的录制命个名,这样方便我们下次使用。我们还可以设置播放次数,然后点【播放】即可。
- 小蓝的图书馆
琴声码语
算法蓝桥杯c++哈希表unordered_map
问题描述小蓝是一个热爱阅读的年轻人,他有一个小型图书馆。为了能够管理他的书籍库存,他需要一个程序来记录图书的信息并执行两种操作:添加图书add和查找作者find。初始小蓝没有书,给出n个操作。add操作给出两个字符串bookname,author,表示添加的图书图书名和作者;find操作给出一个字符串author,你需要输出小蓝的图书馆里这个author有多少本图书。输入格式第一行一个整数n,表示
- 知识库中的知识如何进行分类和标签管理?
自不量力的A同学
人工智能
知识库中的知识如何进行分类和标签管理?基于主题分类明确主题层级:首先确定主要的知识主题领域,如在一个企业知识库中,可以分为“人力资源”“市场营销”“技术研发”等大主题。然后在每个大主题下细分小主题,例如在“人力资源”主题下可以有“招聘”“培训”“绩效考核”等子主题。这样的层级分类有助于用户快速定位到自己需要的知识领域。参考行业标准和最佳实践:按照行业内公认的分类标准来划分知识。以医学知识库为例,可
- AI编程工具领域:深度理解项目架构篇
xinxiyinhe
AI编程python人工智能AI编程人工智能
AI编程工具领域:深度理解项目架构篇在AI编程工具领域,能够读取项目目录并深度理解项目架构的工具主要通过代码索引、上下文感知和智能问答等功能实现。以下是基于最新信息的工具评估与分析:1.通义灵码(阿里云)核心能力:@workspace功能:基于RAG技术,支持本地代码库的索引和深度感知,可分析项目完整结构,生成文件解释、代码逻辑查询和整体修改建议。多语言支持:覆盖200+编程语言,兼容VSCode
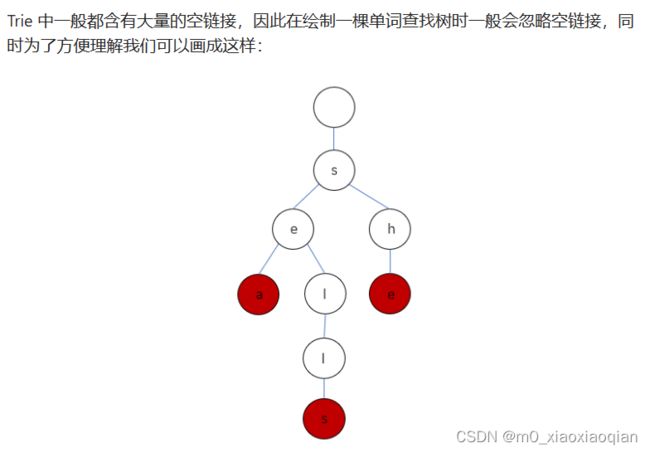
- 软件设计师之树与二叉树:非线性数据结构的深度探索
一杯年华@编程空间
软考中级数据结构
软件设计师之树与二叉树:非线性数据结构的深度探索在软件开发领域,数据结构是程序设计的核心基础,其中树和二叉树作为重要的非线性数据结构,在众多场景中都有着广泛应用。我写这篇博客,就是希望和大家一起学习进步,深入解析树和二叉树的相关知识,用通俗易懂的语言结合图表和Java代码示例进行讲解,帮助大家更好地掌握这些内容。一、树的定义与基本概念树的定义树是由n(n≥0)个结点组成的有限集合。当n=0时,为空
- 清晰易懂的Java8安装教程
Tee xm
windowsjava
小白也能看懂的Java8安装教程(JDK和JRE分目录安装)本教程将手把手教你如何在Windows系统上安装Java8(JDK1.8),并将JDK和JRE安装到不同的目录中,同时提供国内Java8下载源和方法。即使你是编程小白,也能轻松学会!一、准备工作操作系统:Windows10或更高版本。下载工具:一个浏览器(如Chrome、Edge)。存储空间:确保你的电脑有至少500MB的可用空间。二、下
- 数据湖:Apache Iceberg在腾讯的探索和实践
学而知之@
数据库腾讯大数据java编程语言
摘要:今天分享的是ApacheIceberg在腾讯内部的探索和实践。本文结合腾讯大数据技术分享内容和2020全球软件开发大会分享内容进行整理,主要内容包括:1、数据湖技术概述2、ApacheIceberg的简介3、腾讯为什么选择ApacheIceberg4、腾讯看点万亿数据下的业务痛点5、ApacheIceberg在看点实践6、ApacheIceberg读写和删除ApacheIceberg新一代数
- c51中断优先级c语言,51单片机的中断优先级及中断嵌套
金融四十人论坛
c51中断优先级c语言
说最基本的,老的51单片机(80C51系列)有5个中断源,2个优先级,可以实现二级中断服务嵌套。现在很多扩展的51单片机已经有4个优先级(或更多)和更多的中断源了。在说到中断之前,我先来定义一下优先级,明白了什么是优先级,后面的阐述就容易明白了。实际上很多人都是混淆了优先级的含义,所以才觉得糊里糊涂。中断的优先级有两个:查询优先级和执行优先级。什么是查询优级呢?我们从datasheet或书上看到的
- 深入了解 Ubuntu 中的 build-essential:开发者的必备工具
scoone
Linuxubuntulinux运维
摘要:本文将介绍Ubuntu系统中的build-essential包,包括其作用、包含的工具和库,以及如何在Ubuntu上安装和使用build-essential。正文:一、什么是build-essential?build-essential是Ubuntu和其他基于Debian的Linux发行版中的一个元包,它包含了编译软件所必需的工具和库。这个包主要面向开发人员,尤其是那些需要从源代码编译软件的
- 人工智能(AI)系统化学习路线
xiaoyu❅
python人工智能学习
一、为什么需要系统化学习AI?人工智能技术正在重塑各行各业,但许多初学者容易陷入误区:❌盲目跟风:直接学习TensorFlow/PyTorch,忽视数学与算法基础。❌纸上谈兵:只看理论不写代码,无法解决实际问题。❌方向模糊:对CV/NLP/RL等细分领域缺乏认知,难以针对性提升。正确的学习姿势:“金字塔式”分层学习(理论→算法→框架→应用→工程化),逐步构建完整的AI知识体系。二、人工智能学习路线
- AI 时代,学习 Java 应如何入手?
琢磨先生David
人工智能java
一、Java的现状:生态繁荣与AI融合的双重机遇在2025年的技术版图中,Java依然稳坐企业级开发的“头把交椅”。根据行业统计,Java在全球企业级应用中的市场份额仍超过65%,尤其在微服务架构、大数据平台和物联网(IoT)领域占据核心地位。随着云原生技术的普及,Java生态正经历新一轮进化:轻量化框架通过无服务器架构优化,启动速度提升300%,内存占用降低50%,使得Java在容器化部署中更具
- Java 处理 json 格式数据解析为 csv 格式
李昊哲小课
数据分析Java大数据javajson开发语言大数据数据分析
Java处理json格式数据解析为csv格式如果不使用JSON工具库,你可以手动解析JSON格式字符串并将其转换为CSV格式字符串。以下是一个简单示例,展示如何实现这一功能。示例代码下面的示例代码手动处理JSON字符串,将其转换为CSV格式字符串:/***接收JSON字符串,去掉开头和结尾的方括号,按对象划分。*通过extractKeys方法提取字段名,添加到CSV的第一行。*逐项解析JSON对象
- http框架核心之ngx_http.c源码分析
qiuhui00
nginx源码分析nginx源码分析http框架
ngx_http.c内主要实现了一个模块:ngx_http_module。ngx_http_module是nginx的http框架的一部分,它是所有http模块能够被加载的唯一入口,承担了http块配置解析,合并,以及http框架及其相关数据结构的初始化。它本身是NGX_CORE_MODULE类型,只有一个指令,就是http,如下所示:staticngx_command_tngx_http_com
- 代替Windows系统的最佳系统开发:开源、国产与跨平台的选择指南
夏末之花
windows开源
近年来,随着技术自主化和隐私安全需求的提升,越来越多的用户开始寻求Windows系统的替代方案。本文结合国内外热门操作系统及开发工具,分析其核心优势与适用场景,助你找到最适合的开发与日常使用平台。一、开源之王:Linux发行版1.Ubuntu与LinuxMint作为最受欢迎的Linux发行版,Ubuntu和LinuxMint以用户友好性著称,尤其适合从Windows迁移的用户。其内置的软件包管理器
- 3DMAX点云算法:实现毫米级BIM模型偏差检测(附完整代码)
夏末之花
人工智能
摘要本文基于激光雷达点云数据与BIM模型的高精度对齐技术,提出一种融合动态体素化与多模态特征匹配的偏差检测方法。通过点云预处理、语义分割、模型配准及差异分析,最终实现建筑构件毫米级偏差的可视化检测。文中提供关键代码实现,涵盖点云处理、特征提取与深度学习模型搭建。一、核心算法流程点云预处理与特征增强去噪与下采样:采用统计滤波与体素网格下采样,去除离群点并降低数据量。语义分割:基于PointNet++
- Python-有效字母异位词
m0_37763377
python哈希算法算法数据结构
一、什么是字母异位词字母异位词是指由相同字母组成但排列顺序不同的单词。例如,"eat"、"tea"和"ate"都是字母异位词,因为它们由相同的字母组成,只是排列顺序不同。二、思路(一)暴力解法这里可以用两层循环来判断2个字符串的元素是否一样,显然时间复杂度为O(n²),在这里大家可以自己写一下,文章就不再提供演示。(二)哈希表解法1.什么是哈希表?哈希表(HashTable),也称为散列表,是
- LeetCode135☞分糖果
fantasy_4
LeetCode刷题javapythonleetcode贪心算法算法
关联LeetCode题号135本题特点贪心两次遍历,一次正序遍历,只比较左边,左边比右边大的情况i-1i一次倒序遍历,只比较右边的,右边比左边大i+1i本题思路classSolution:defcandy(self,ratings:List[int])->int:candy=[1]*len(ratings)#右大于左foriinrange(1,len(ratings)):ifratings[i]>
- LeetCode134☞加油站
fantasy_4
LeetCode刷题pythonleetcodejava算法贪心算法
关联LeetCode题号134本题特点贪心局部最优解-部分差值如果小于0(消耗大于油站油量)就从下一个加油站开始,因为如果中间有小于0的情况当前站就不可能是始发站,整体最优解-整体差值如果小于0,那么就是不能有始发站本题思路classSolution:defcanCompleteCircuit(self,gas:List[int],cost:List[int])->int:curSum=0tota
- LeetCode56☞合并区间
fantasy_4
LeetCode刷题leetcodepythonjava算法贪心算法
关联LeetCode题号56本题特点贪心本题思路将二维数组排序按照左边界排序。排序后,右边界的大小成为找到局部最大值的关键。由题意合并区间可知,应该取数组的’并集‘,局部最优解推出全局最优解,每次找到局部最大的范围,整体就会合并成一个大区间Python写法defmerge(self,intervals):result=[]iflen(intervals)==0:returnresult#区间集合为
- Apache Doris整合Iceberg + Flink CDC构建实时湖仓体的联邦查询分析架构
MfvShell
apacheflink架构Flink
随着大数据技术的迅猛发展,构建实时湖仓体并进行联邦查询分析成为了许多企业的迫切需求。在这篇文章中,我们将探讨如何利用ApacheDoris整合Iceberg和FlinkCDC来构建这样一个架构,并提供相应的源代码示例。简介实时湖仓体是一种灵活、可扩展的数据架构,结合了数据湖和数据仓库的优势。ApacheDoris是一款开源的分布式SQL引擎,专注于实时分析和查询。Iceberg是一种开放式表格格式
- 【python】图形用户界面和游戏开发
usp1994
pythonuiide
图形用户界面和游戏开发文章目录图形用户界面和游戏开发基于tkinter模块的GUI使用Pygame进行游戏开发制作游戏窗口在窗口中绘图加载图像实现动画效果碰撞检测事件处理基于tkinter模块的GUIGUI是图形用户界面的缩写,图形化的用户界面对使用过计算机的人来说应该都不陌生,在此也无需进行赘述。Python默认的GUI开发模块是tkinter(在Python3以前的版本中名为Tkinter),
- 【Apache Storm】
茉菇
apachestorm大数据
一、Storm简介1、概述官网地址:https://storm.apache.org/index.htmlApacheStorm是一个开源的、分布式的实时计算系统,专为处理流式数据而设计。它能够处理大量数据流并在极低的延迟下提供实时的结果。相比于传统的批处理系统,Storm具有处理无限数据流的能力,支持非常高的可扩展性和容错机制。Storm可以适用于多种编程语言,具有高度的灵活性。2、核心功能分布
- Python 爬虫实战:如何爬取小红书数据并进行分析
Python爬虫项目
python爬虫开发语言selenium测试工具
一、引言随着社交电商的崛起,小红书(Xiaohongshu)作为一款结合了社交和电商的应用,吸引了大量年轻用户。用户在平台上分享购物心得、生活经验以及个性化的消费推荐内容,形成了庞大的用户数据与内容生态。因此,如何从小红书获取数据进行分析,成为了数据科学、市场营销和社交媒体研究中的一个重要课题。本文将介绍如何使用Python编写爬虫爬取小红书的数据,分析如何通过小红书的开放API获取用户信息、帖子
- springmvc 下 freemarker页面枚举的遍历输出
杨白白
enumfreemarker
spring mvc freemarker 中遍历枚举
1枚举类型有一个本地方法叫values(),这个方法可以直接返回枚举数组。所以可以利用这个遍历。
enum
public enum BooleanEnum {
TRUE(Boolean.TRUE, "是"), FALSE(Boolean.FALSE, "否");
- 实习简要总结
byalias
工作
来白虹不知不觉中已经一个多月了,因为项目还在需求分析及项目架构阶段,自己在这段
时间都是在学习相关技术知识,现在对这段时间的工作及学习情况做一个总结:
(1)工作技能方面
大体分为两个阶段,Java Web 基础阶段和Java EE阶段
1)Java Web阶段
在这个阶段,自己主要着重学习了 JSP, Servlet, JDBC, MySQL,这些知识的核心点都过
了一遍,也
- Quartz——DateIntervalTrigger触发器
eksliang
quartz
转载请出自出处:http://eksliang.iteye.com/blog/2208559 一.概述
simpleTrigger 内部实现机制是通过计算间隔时间来计算下次的执行时间,这就导致他有不适合调度的定时任务。例如我们想每天的 1:00AM 执行任务,如果使用 SimpleTrigger,间隔时间就是一天。注意这里就会有一个问题,即当有 misfired 的任务并且恢复执行时,该执行时间
- Unix快捷键
18289753290
unixUnix;快捷键;
复制,删除,粘贴:
dd:删除光标所在的行 &nbs
- 获取Android设备屏幕的相关参数
酷的飞上天空
android
包含屏幕的分辨率 以及 屏幕宽度的最大dp 高度最大dp
TextView text = (TextView)findViewById(R.id.text);
DisplayMetrics dm = new DisplayMetrics();
text.append("getResources().ge
- 要做物联网?先保护好你的数据
蓝儿唯美
数据
根据Beecham Research的说法,那些在行业中希望利用物联网的关键领域需要提供更好的安全性。
在Beecham的物联网安全威胁图谱上,展示了那些可能产生内外部攻击并且需要通过快速发展的物联网行业加以解决的关键领域。
Beecham Research的技术主管Jon Howes说:“之所以我们目前还没有看到与物联网相关的严重安全事件,是因为目前还没有在大型客户和企业应用中进行部署,也就
- Java取模(求余)运算
随便小屋
java
整数之间的取模求余运算很好求,但几乎没有遇到过对负数进行取模求余,直接看下面代码:
/**
*
* @author Logic
*
*/
public class Test {
public static void main(String[] args) {
// TODO A
- SQL注入介绍
aijuans
sql注入
二、SQL注入范例
这里我们根据用户登录页面
<form action="" > 用户名:<input type="text" name="username"><br/> 密 码:<input type="password" name="passwor
- 优雅代码风格
aoyouzi
代码
总结了几点关于优雅代码风格的描述:
代码简单:不隐藏设计者的意图,抽象干净利落,控制语句直截了当。
接口清晰:类型接口表现力直白,字面表达含义,API 相互呼应以增强可测试性。
依赖项少:依赖关系越少越好,依赖少证明内聚程度高,低耦合利于自动测试,便于重构。
没有重复:重复代码意味着某些概念或想法没有在代码中良好的体现,及时重构消除重复。
战术分层:代码分层清晰,隔离明确,
- 布尔数组
百合不是茶
java布尔数组
androi中提到了布尔数组;
布尔数组默认的是false, 并且只会打印false或者是true
布尔数组的例子; 根据字符数组创建布尔数组
char[] c = {'p','u','b','l','i','c'};
//根据字符数组的长度创建布尔数组的个数
boolean[] b = new bool
- web.xml之welcome-file-list、error-page
bijian1013
javaweb.xmlservleterror-page
welcome-file-list
1.定义:
<welcome-file-list>
<welcome-file>login.jsp</welcome>
</welcome-file-list>
2.作用:用来指定WEB应用首页名称。
error-page1.定义:
<error-page&g
- richfaces 4 fileUpload组件删除上传的文件
sunjing
clearRichfaces 4fileupload
页面代码
<h:form id="fileForm"> <rich:
- 技术文章备忘
bit1129
技术文章
Zookeeper
http://wenku.baidu.com/view/bab171ffaef8941ea76e05b8.html
http://wenku.baidu.com/link?url=8thAIwFTnPh2KL2b0p1V7XSgmF9ZEFgw4V_MkIpA9j8BX2rDQMPgK5l3wcs9oBTxeekOnm5P3BK8c6K2DWynq9nfUCkRlTt9uV
- org.hibernate.hql.ast.QuerySyntaxException: unexpected token: on near line 1解决方案
白糖_
Hibernate
文章摘自:http://blog.csdn.net/yangwawa19870921/article/details/7553181
在编写HQL时,可能会出现这种代码:
select a.name,b.age from TableA a left join TableB b on a.id=b.id
如果这是HQL,那么这段代码就是错误的,因为HQL不支持
- sqlserver按照字段内容进行排序
bozch
按照内容排序
在做项目的时候,遇到了这样的一个需求:
从数据库中取出的数据集,首先要将某个数据或者多个数据按照地段内容放到前面显示,例如:从学生表中取出姓李的放到数据集的前面;
select * fro
- 编程珠玑-第一章-位图排序
bylijinnan
java编程珠玑
import java.io.BufferedWriter;
import java.io.File;
import java.io.FileWriter;
import java.io.IOException;
import java.io.Writer;
import java.util.Random;
public class BitMapSearch {
- Java关于==和equals
chenbowen00
java
关于==和equals概念其实很简单,一个是比较内存地址是否相同,一个比较的是值内容是否相同。虽然理解上不难,但是有时存在一些理解误区,如下情况:
1、
String a = "aaa";
a=="aaa";
==> true
2、
new String("aaa")==new String("aaa
- [IT与资本]软件行业需对外界投资热情保持警惕
comsci
it
我还是那个看法,软件行业需要增强内生动力,尽量依靠自有资金和营业收入来进行经营,避免在资本市场上经受各种不同类型的风险,为企业自主研发核心技术和产品提供稳定,温和的外部环境...
如果我们在自己尚未掌握核心技术之前,企图依靠上市来筹集资金,然后使劲往某个领域砸钱,然
- oracle 数据块结构
daizj
oracle块数据块块结构行目录
oracle 数据块是数据库存储的最小单位,一般为操作系统块的N倍。其结构为:
块头--〉空行--〉数据,其实际为纵行结构。
块的标准大小由初始化参数DB_BLOCK_SIZE指定。具有标准大小的块称为标准块(Standard Block)。块的大小和标准块的大小不同的块叫非标准块(Nonstandard Block)。同一数据库中,Oracle9i及以上版本支持同一数据库中同时使用标
- github上一些觉得对自己工作有用的项目收集
dengkane
github
github上一些觉得对自己工作有用的项目收集
技能类
markdown语法中文说明
回到顶部
全文检索
elasticsearch
bigdesk elasticsearch管理插件
回到顶部
nosql
mapdb 支持亿级别map, list, 支持事务. 可考虑做为缓存使用
C
- 初二上学期难记单词二
dcj3sjt126com
englishword
dangerous 危险的
panda 熊猫
lion 狮子
elephant 象
monkey 猴子
tiger 老虎
deer 鹿
snake 蛇
rabbit 兔子
duck 鸭
horse 马
forest 森林
fall 跌倒;落下
climb 爬;攀登
finish 完成;结束
cinema 电影院;电影
seafood 海鲜;海产食品
bank 银行
- 8、mysql外键(FOREIGN KEY)的简单使用
dcj3sjt126com
mysql
一、基本概念
1、MySQL中“键”和“索引”的定义相同,所以外键和主键一样也是索引的一种。不同的是MySQL会自动为所有表的主键进行索引,但是外键字段必须由用户进行明确的索引。用于外键关系的字段必须在所有的参照表中进行明确地索引,InnoDB不能自动地创建索引。
2、外键可以是一对一的,一个表的记录只能与另一个表的一条记录连接,或者是一对多的,一个表的记录与另一个表的多条记录连接。
3、如
- java循环标签 Foreach
shuizhaosi888
标签java循环foreach
1. 简单的for循环
public static void main(String[] args) {
for (int i = 1, y = i + 10; i < 5 && y < 12; i++, y = i * 2) {
System.err.println("i=" + i + " y="
- Spring Security(05)——异常信息本地化
234390216
exceptionSpring Security异常信息本地化
异常信息本地化
Spring Security支持将展现给终端用户看的异常信息本地化,这些信息包括认证失败、访问被拒绝等。而对于展现给开发者看的异常信息和日志信息(如配置错误)则是不能够进行本地化的,它们是以英文硬编码在Spring Security的代码中的。在Spring-Security-core-x
- DUBBO架构服务端告警Failed to send message Response
javamingtingzhao
架构DUBBO
废话不多说,警告日志如下,不知道有哪位遇到过,此异常在服务端抛出(服务器启动第一次运行会有这个警告),后续运行没问题,找了好久真心不知道哪里错了。
WARN 2015-07-18 22:31:15,272 com.alibaba.dubbo.remoting.transport.dispatcher.ChannelEventRunnable.run(84)
- JS中Date对象中几个用法
leeqq
JavaScriptDate最后一天
近来工作中遇到这样的两个需求
1. 给个Date对象,找出该时间所在月的第一天和最后一天
2. 给个Date对象,找出该时间所在周的第一天和最后一天
需求1中的找月第一天很简单,我记得api中有setDate方法可以使用
使用setDate方法前,先看看getDate
var date = new Date();
console.log(date);
// Sat J
- MFC中使用ado技术操作数据库
你不认识的休道人
sqlmfc
1.在stdafx.h中导入ado动态链接库
#import"C:\Program Files\Common Files\System\ado\msado15.dll" no_namespace rename("EOF","end")2.在CTestApp文件的InitInstance()函数中domodal之前写::CoIniti
- Android Studio加速
rensanning
android studio
Android Studio慢、吃内存!启动时后会立即通过Gradle来sync & build工程。
(1)设置Android Studio
a) 禁用插件
File -> Settings... Plugins 去掉一些没有用的插件。
比如:Git Integration、GitHub、Google Cloud Testing、Google Cloud
- 各数据库的批量Update操作
tomcat_oracle
javaoraclesqlmysqlsqlite
MyBatis的update元素的用法与insert元素基本相同,因此本篇不打算重复了。本篇仅记录批量update操作的
sql语句,懂得SQL语句,那么MyBatis部分的操作就简单了。 注意:下列批量更新语句都是作为一个事务整体执行,要不全部成功,要不全部回滚。
MSSQL的SQL语句
WITH R AS(
SELECT 'John' as name, 18 as
- html禁止清除input文本输入缓存
xp9802
input
多数浏览器默认会缓存input的值,只有使用ctl+F5强制刷新的才可以清除缓存记录。如果不想让浏览器缓存input的值,有2种方法:
方法一: 在不想使用缓存的input中添加 autocomplete="off"; eg: <input type="text" autocomplete="off" name