C++ unordered_map和unordered_set的使用,哈希表的实现
文章目录
- unordered_map,unorder_set和map ,set的差异
- 哈希表的实现
-
- 概念
- 直接定址法
- 哈希冲突
-
- 哈希冲突举个例子
- 负载因子
- 将关键字转为整数
- 哈希函数
-
- 除法散列法/除留余数法
- 哈希冲突的解决方法
-
- 开放定址法
-
- 线性探测
- 二次探测
- 开放定址法代码实现
- 哈希表的代码
unordered_map,unorder_set和map ,set的差异
unordered_map,unordered_set在功能方面和map,set基本一致
unordered_map和unordered_set底层是哈希表,遍历无序,查找删除修改的效率为O(1)
map和set底层是红黑树,遍历有序,查找删除修改的效率为O(logN)
- 功能:迭代器之间也有差异,set是双向迭代器,unordered_set是单向迭代器,set底层是红黑树,走中序是有序的,所以迭代器的遍历是有序+去重,unordered_set底层是哈希表,迭代器遍历是无序+去重
- 效率:unordered_set和set性能方面也有差异,set是O(logN),unordered_set是O(1)
- 使用要求:对key的要求不同,set要求key支持小于的比较,unordered_set要求key转为整形且要支持等于的比较
- unordered_set,unordered_map和map,set要求基本一致
- unordered_multimap和unordered_multiset支持键值冗余(key冗余)
- 效率上无序的比有序的总体上要好很多,但是在排有序的数的时候,有序的删除,插入比无序的效率高,但是查找效率还是无序的效率高

void set_test1()
{
// 迭代器遍历
set<int> s = { 3,1,6,7,8,2};
set<int>::iterator it = s.begin();
while (it != s.end())
{
cout << *it << " ";
++it;
}
// 3 1 6 7 8 2
cout << endl;
}
int main()
{
set_test1();
return 0;
}
哈希表的实现
概念
- 哈希表也叫散列表,散列有散乱排列的意思。通过关键字Key跟存储位置建立一个映射关系
直接定址法
- 适合关键字的范围比较集中的,直接定址法是非常高效的方法。比如给’a’ - 'z’的小写字母为范围,通过直接映射或相对映射,关键字的值就是存储位置的下标,下面也有一道题是这样的。只适合整形,字符串,浮点数都不行
字符串中的第一个唯一字符
class Solution
{
public:
int firstUniqChar(string s)
{
int hash[26] = {0};
for(auto ch : s)
{
hash[ch - 'a']++;
}
for(int i = 0;i < s.size();i++)
{
if(hash[s[i] - 'a'] == 1)
return i;
}
return -1;
}
};
哈希冲突
- 直接定址法的缺点非常明显,当关键字比较分散时,会浪费空间甚至空间会不够用。当有N个值要映射到M个空间中(M >= N),就要借助哈希函数,关键字key要放到数组的h(key)的位置,h(key)计算的值要在[0,M)中。
- 哈希冲突也叫哈希碰撞,就是两个不同的key映射到同一个位置了,我们要设计一个哈希函数来减少哈希冲突,在实际问题中哈希冲突是不可避免的
哈希冲突举个例子
N == 100 M == 200,N个数要存到M个空间中
除法散列法:h(key) = key % M
哈希冲突:3 % 200 = 3,203 % 200 = 3
负载因子
哈希表中已经存了N个值,哈希表的大小为M,负载因子 = N/M,负载因子也叫装载因子/载荷因子,负载因子越大,哈希冲突越高,空间利用率越高,相反就越低。
将关键字转为整数
比如是浮点数转为整数或者有符号的整数(负数)要转为正数,字符串转为整数。
哈希函数
一个好的哈希函数应该让N个关键字被等概率的均匀的散列分布到哈希表的M个空间中,但是实际中却很难做到,但是我们要尽量往这个方向去考量设计。
除法散列法/除留余数法
- 除法散列表是最常用的,哈希表的大小为M,通过key除以M的余数作为映射的下标,h(key) = key % M
- 使用这个方法时要注意M不要为2的幂,10的幂等。如果是2^X,那么key%2 ^ X,相当于保留key的后X位,那么key的后X位相同的值就哈希冲突了。
- 比如63,31,如果M是16,2 ^ 4,计算出的哈希值都是15,00111111为63,00011111为31,后4位都是1111,所以会哈希冲突。10进制的,比如123和345123,如果M = 10 ^ 3,保留后三位,都是123,也哈希冲突。
- 当使用除留余数法时,建议M取不太接近2的整数次幂的一个质数
- %其实用到了除,除的效率比位运算的效率更低,所以Java中用了位运算
哈希冲突的解决方法
开放定址法
- 开放定址法,哈希表是空的,把所有元素放到哈希表中,当关键字key用哈希函数找到了冲突位置,就按照某种规则去找一个没有存储数据的位置进行储存。这里有三种规则:线性探测,二次探测,双重探测
线性探测
现在给一组数据:
{19,30,5,36,13,20,21,12} 等这一组值映射到M=11的表中
h(19) = 8,h(30) = 8,h(5) = 5,h(36) = 3,
h(13) = 2,h(20) = 9,h(21) =10,h(12) = 1
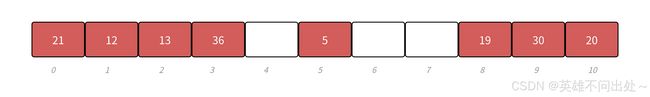
上面这组数据在8位置就冲突了
- 线性探测:从发生冲突的位置依次往后探测,直到找到一个没有存储数据的位置为止,如果走到哈希尾都没有找到,则回绕到哈希头继续往后找
- h(key) = hash0 = key % M , hash0位置冲突了,则线性探测公式为:hc(key, i) = hashi = (hash0 + i) % M, i = {1, 2, 3, …, M − 1},因为负载因子必须小于1(因为要留一个位置不放数据,不然会出问题),则最多探测M-1次,一定能找到一个存储key的位置。
- 连续冲突:hash0位置连续冲突,(你占我的位置,我占你的位置)这种现象叫做 群集/堆积,二次探测可以改善这样的问题
二次探测
二次探测和线性探测非常类似
如果往左走回绕到哈希表尾,用减,比如3-9,到5的位置停下,-6 + M,M == 11, -6 + M = 5

// 二次探测
int flag = 1;
while (_tables[hashi]._state == EXIST)
{
// 存在进行二次探测,删除和空就退出
hashi = (hash0 + i*i*flag) % _tables.size();
if (hashi < 0)
hashi += _tables.size();
if (flag == 1)
{
flag = -1;
}
else
{
flag = 1;
++i;
}
}
开放定址法代码实现
h(19) = 8,h(30) = 8,h(5) = 5,h(36) = 3,
h(13) = 2,h(20) = 9,h(21) =10,h(12) = 1
蓝色的四个位置连续冲突
查找的原则:遇到删除,存在才继续找,遇到空就结束查找
状态标识:存在,空和删除,空和删除可以放值,空就线性探测
要注意给每个存储值的位置加一个状态标识,否则删除一些值以后,会影响后面冲突的值的查找。
如下图,我们删除30,会导致查找20失败,当我们给每个位置加⼀个状态标识{EXIST,EMPTY,DELETE} ,删除30就可以不用删除值,而是把状态改为 DELETE ,那么查找20时是遇到 EMPTY 才能停止,就可以找到20。
哈希表的代码
#pragma once
#include> newtables(_tables.size()*2);
//for (auto& data : _tables)
//{
// // 把旧表的数据映射到新表
// // 不能直接拷贝,因为映射关系还是原来的(会乱)
// if (data._state == EMPTY)
// {
// size_t hash0 = data._kv.first % newtables.size();
// // ...
// }
//}
//_tables.swap(newtables);
// 上面的方法代码过于冗余
// 把新表和旧表交换
HashTable<K, V> newht;
// newht._tables.resize(_table.size() * 2);
newht._tables.resize(__stl_next_prime(_table.size()));
for (auto& data : _tables)
{
// 把旧表的数据映射到新表
if (data._state == EMPTY)
{
// 相当于递归
newht.Insert(data._kv);
}
}
// 函数结束,newht销毁,数据交换给旧表
_tables.swap(newht._tables);
}
// key / M , M哈希表的空间大小
size_t hash0 = kv.first % _tables.size();
size_t hashi = hash0;
size_t i = 1;
while (_tables[hashi]._state == EXIST)
{
// 存在进行线性探测,删除和空就退出
hashi = (hash0 + i) % _tables.size();
++i;
}
// 二次探测
//int flag = 1;
//while (_tables[hashi]._state == EXIST)
//{
// // 存在进行二次探测,删除和空就退出
// hashi = (hash0 + i*i*flag) % _tables.size();
// if (hashi < 0)
// hashi += _tables.size();
// if (flag == 1)
// {
// flag = -1;
// }
// else
// {
// flag = 1;
// ++i;
// }
//}
_tables[hashi]._kv = kv;
_tables[hashi]._state = EXIST;
++_n;
return true;
}
// 查找
HashData<K, V>* Find(const K& key)
{
size_t hash0 = key % _tables.size();
size_t hashi = hash0;
size_t i = 1;
// Find等于空就找不到了
while (_tables[hashi]._state != EMPTY)
{
if (_tables[hashi]._state == EXIST
&& _tables[hashi]._kv.first == key)
{
return &_tables[hashi];
}
// 存在进行线性探测,删除和空就退出
hashi = (hash0 + i) % _tables.size();
++i;
}
return nullptr;
}
// 删除
bool Erase(const K& key)
{
HashData<K, V>* ret = Find(key);
if (key)
{
ret->_state = DELETE;
return true;
}
else
{
return false;
}
}
private:
vector<HashData<K, V>> _tables;
size_t _n;// 记录哈希表中的数据个数
};
#include"HashTable.h"
int main()
{
int a[] = { 19,30,5,36,13,20,21,12 };
HashTable<int, int> ha;
for (auto& e : a)
{
ha.Insert({ e,e });
}
ha.Erase(20);
if (ha.Find(30))
{
cout << "找到了" << endl;
}
if (ha.Find(20))
{
cout << "找到了" << endl;
}
else
{
cout << "没有找到" << endl;
}
return 0;
}
