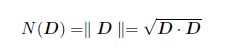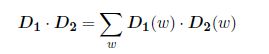MIT Introduction to Algorithms 学习笔记(二)

 

Lecture 2: Models of Computation(Ⅰ)

1. 什么是算法(Algorithm)?

   l  Mathematical abstraction of computer program

   l  Computational procedure to solve a problem

    

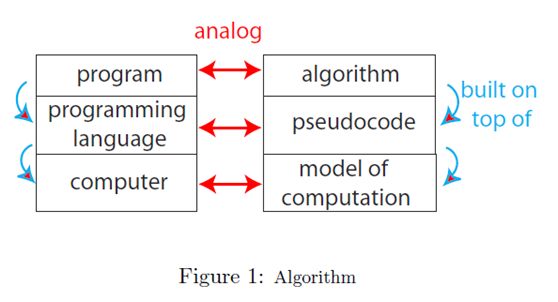
算法和程序的类比:

两种计算模型( Model of computation):

 

Random Access Machine (RAM)

 

• Random Access Memory (RAM) modeled by a big array

• Θ(1) registers (each 1 word)

• In Θ(1) time, can

• load word @ ri into register rj

• compute (+, −, ∗, /, &, |, ˆ) on registers

• store register rj into memory @ ri

• What’s a word? w ≥ lg (memory size) bits

• realistic and powerful → implement abstractions

 

 

Pointer Machine

 

 

• dynamically allocated objects (namedtuple)

•  object has O(1) fields

• field = word (e.g., int) or pointer to object/null (a.k.a. reference)

• weaker than (can be implemented on) RAM

 

2.   Document Distance Problem — compute d(D₁,D₂)

 

主要用于查找相似的文件，检测重复（维基百科和谷歌）和抄袭，网络搜索。

什么是Word 和Document?

 

• Word = sequence of alphanumeric characters

• Document = sequence of words (ignore space, punctuation, etc.)

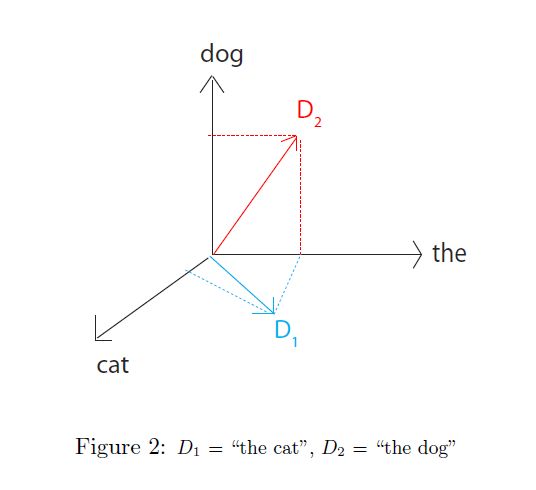
把文件D（document D）看作一个向量（vector）：D[w] = #  代表W在文件中出现的次数 .例如：

 

用向量D1和D2的夹角表示2个文件之间的重叠度。

 

其中，

 

 

Document Distance Algorithm

a)  split each document into words

b)  count word frequencies (document vectors)

c)  sort into order

d)  compute dot product (Compute θ)

 

代码（V1）：

 

# docdist1.py
# Author: Ronald L. Rivest
# Date Last Modified: February 14, 2007
# Changelog:
#   Version 1:
#     Initial version
#
# Usage:
#    docdist1.py filename1 filename2
#     
# This program computes the "distance" between two text files
# as the angle between their word frequency vectors (in radians).
#
# For each input file, a word-frequency vector is computed as follows:
#    (1) the specified file is read in
#    (2) it is converted into a list of alphanumeric "words"
#        Here a "word" is a sequence of consecutive alphanumeric
#        characters.  Non-alphanumeric characters are treated as blanks.
#        Case is not significant.
#    (3) for each word, its frequency of occurrence is determined
#    (4) the word/frequency lists are sorted into order alphabetically
#
# The "distance" between two vectors is the angle between them.
# If x = (x1, x2, ..., xn) is the first vector (xi = freq of word i)
# and y = (y1, y2, ..., yn) is the second vector,
# then the angle between them is defined as:
#    d(x,y) = arccos(inner_product(x,y) / (norm(x)*norm(y)))
# where:
#    inner_product(x,y) = x1*y1 + x2*y2 + ... xn*yn
#    norm(x) = sqrt(inner_product(x,x))
import math
    # math.acos(x) is the arccosine of x.
    # math.sqrt(x) is the square root of x.
import string
    # string.join(words,sep) takes a given list of words,
    #    and returns a single string resulting from concatenating them
    #    together, separated by the string sep .
    # string.lower(word) converts word to lower-case
import sys
##################################
# Operation 1: read a text file ##
##################################
def read_file(filename):
    """ 
    Read the text file with the given filename;
    return a list of the lines of text in the file.
    """
    try:
        fp = open(filename)
        L = fp.readlines()
    except IOError:
        print "Error opening or reading input file: ",filename
        sys.exit()
    return L
#################################################
# Operation 2: split the text lines into words ##
#################################################
def get_words_from_line_list(L):
    """
    Parse the given list L of text lines into words.
    Return list of all words found.
    """
    word_list = []
    for line in L:
        words_in_line = get_words_from_string(line)
        word_list = word_list + words_in_line
    return word_list
def get_words_from_string(line):
    """
    Return a list of the words in the given input string,
    converting each word to lower-case.
    Input:  line (a string)
    Output: a list of strings 
              (each string is a sequence of alphanumeric characters)
    """
    word_list = []          # accumulates words in line
    character_list = []     # accumulates characters in word
    for c in line:
        if c.isalnum():
            character_list.append(c)
        elif len(character_list)>0:
            word = string.join(character_list,"")
            word = string.lower(word)
            word_list.append(word)
            character_list = []
    if len(character_list)>0:
        word = string.join(character_list,"")
        word = string.lower(word)
        word_list.append(word)
    return word_list
##############################################
# Operation 3: count frequency of each word ##
##############################################
def count_frequency(word_list):
    """
    Return a list giving pairs of form: (word,frequency)
    """
    L = []
    for new_word in word_list:
        for entry in L:
            if new_word == entry[0]:
                entry[1] = entry[1] + 1
                break
        else:
            L.append([new_word,1])
    return L
###############################################################
# Operation 4: sort words into alphabetic order             ###
###############################################################
def insertion_sort(A):
    """
    Sort list A into order, in place.
    From Cormen/Leiserson/Rivest/Stein,
    Introduction to Algorithms (second edition), page 17,
    modified to adjust for fact that Python arrays use 
    0-indexing.
    """
    for j in range(len(A)):
        key = A[j]
        # insert A[j] into sorted sequence A[0..j-1]
        i = j-1
        while i>-1 and A[i]>key:
            A[i+1] = A[i]
            i = i-1
        A[i+1] = key
    return A
    
#############################################
## compute word frequencies for input file ##
#############################################
def word_frequencies_for_file(filename):
    """
    Return alphabetically sorted list of (word,frequency) pairs 
    for the given file.
    """
    line_list = read_file(filename)
    word_list = get_words_from_line_list(line_list)
    freq_mapping = count_frequency(word_list)
    insertion_sort(freq_mapping)
    print "File",filename,":",
    print len(line_list),"lines,",
    print len(word_list),"words,",
    print len(freq_mapping),"distinct words"
    return freq_mapping
def inner_product(L1,L2):
    """
    Inner product between two vectors, where vectors
    are represented as alphabetically sorted (word,freq) pairs.
    Example: inner_product([["and",3],["of",2],["the",5]],
                           [["and",4],["in",1],["of",1],["this",2]]) = 14.0 
    """
    sum = 0.0
    i = 0
    j = 0
    while i<len(L1) and j<len(L2):
        # L1[i:] and L2[j:] yet to be processed
        if L1[i][0] == L2[j][0]:
            # both vectors have this word
            sum += L1[i][1] * L2[j][1]
            i += 1
            j += 1
        elif L1[i][0] < L2[j][0]:
            # word L1[i][0] is in L1 but not L2
            i += 1
        else:
            # word L2[j][0] is in L2 but not L1
            j += 1
    return sum
def vector_angle(L1,L2):
    """
    The input is a list of (word,freq) pairs, sorted alphabetically.
    Return the angle between these two vectors.
    """
    numerator = inner_product(L1,L2)
    denominator = math.sqrt(inner_product(L1,L1)*inner_product(L2,L2))
    return math.acos(numerator/denominator)
def main():
    if len(sys.argv) != 3:
        print "Usage: docdist1.py filename_1 filename_2"
    else:
        filename_1 = sys.argv[1]
        filename_2 = sys.argv[2]
        sorted_word_list_1 = word_frequencies_for_file(filename_1)
        sorted_word_list_2 = word_frequencies_for_file(filename_2)
        distance = vector_angle(sorted_word_list_1,sorted_word_list_2)
        print "The distance between the documents is: %0.6f (radians)"%distance

if __name__ == "__main__":
    main()