考研复试机试题(2009)
转载请标明出处:牟尼的专栏 http://blog.csdn.net/u012027907
Problem A:请写一个程序,给出指定整数范围【a ,b】内所有的完数,一个数如果恰好等于除它本身外的所有因子之和,这个数就称为完数,例如6是完数,因为6=1+2+3。
输入说明:共一组数据,为两个正整数,分别表示a和b(1<a<b<10^5)。
输出说明:指定范围内的所有完数,每个数占一行。
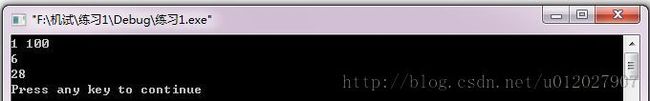
输入样本
1 100
输出样本
6
28
解答:
/*
* 描述: 机试题A解答
* 作者: 张亚超
* 博客: 牟尼的专栏 http://blog.csdn.net/u012027907
* 日期: 2014/7/23
*/
#include "stdio.h"
int main(){
int a,b;
int sum = 0;
scanf("%d%d",&a,&b);
for(int num = a; num <= b; num++){
sum = num;
for(int i = 1; i < num/2+1; i++){ //处理每个数
if(num % i == 0){ //能被i整除
sum -= i; //减去一个因子
}
}
if(sum == 0){
printf("%d\n",num); //输出完数
}
}
return 0;
}
Problem B:请写一个程序,对于一个m行m列的(1<m<10)的方阵,求其每一行,每一列及主对角线元素之和,最后按照从大到小的顺序依次输出。
输入说明:共一组数据,输入的第一行为一个正整数,表示m,接下来的m行,每行m个整数表示方阵元素。
输出说明:从大到小排列的一行整数,每个整数后跟一个空格,最后换行。
输入样本:
4
15 8 -26
31 24 18 71
-3-9 27 13
17 21 38 69
输出样本:
159 145 144 13581 60 44 32 2827
解答:
#include "stdio.h"
#define Max 25
void sort(int store[],int count){
//冒泡排序
for(int i = 0; i < count; i++)
for(int j = i+1; j < count; j++){
if(store[i] < store[j]){
int temp = store[i];
store[i] = store[j];
store[j] = temp;
}
}
}
void print(int store[],int count){
//打印输出
for(int i = 0; i < count; i++)
printf("%d ",store[i]);
printf("\n");
}
int main(){
int m;
int st[10][10],res[Max];
int count = 0;
scanf("%d",&m);
//统计每行的和
for(int i = 0; i < m; i++){
int sum = 0;
for(int j = 0; j < m; j++){
scanf("%d",&st[i][j]);
sum += st[i][j];
}
res[count++] = sum;
}
//统计每列的和
for(i = 0; i < m; i++){
int sum = 0;
for(int j = 0; j < m; j++){
sum += st[j][i];
}
res[count++] = sum;
}
int sum1,sum2;
sum1 = sum2 = 0;
for(i = 0; i < m; i++){ //统计主副对角线的和
sum1 += st[i][i];
sum2 += st[i][m-i-1];
}
res[count++] = sum1;
res[count++] = sum2;
sort(res,count);
print(res,count);
return 0;
}
Problem C:对于给定的字符序列,从左至右将所有的数字字符取出拼接成一个无符号整数(字符序列长度小于100,拼接出的整数小于2^31,),计算并输出该整数的最大因子(如果是素数,则其最大因子为自身)
输入说明:有多组数据,输入数据的第一行为一个正整数,表示字符序列的数目,每组数据为一行字符序列。
输出说明:对每个字符序列,取出所得整数的最大因子,若字符序列中没有数字或者找出的整数为0,则输出0,每个整数占一行输出。
输入样本:
3
sdf0ejg3.f?9f
?4afd0s&2d79*(g
abcde
输出样本:
13
857
0
#include "stdio.h"
#define Max 100
int maxPrimeFactor(int n)
{
int i,ans=0;
for(i=2;i*i<=n;i++)
{
while(n%i==0)
{
ans=i;
n/=i;
}
}
if(n>1)ans=n;
return ans;
}
bool isDigit(char ch){
//判断是否是数字
if(ch >= '0' && ch <= '9')
return true;
else
return false;
}
int main(){
char str[Max][Max],tmp;
int res[Max];
int n;
scanf("%d",&n);
for(int j = 0; j < n; j++){
scanf("%s",str[j]);
int sum = 0;
int i = 0;
res[j] = 0;
while(str[j][i] != '\0'){
tmp = str[j][i];
if(isDigit(tmp)){
sum = sum*10 + (tmp - '0');
}
i++;
}
res[j] = maxPrimeFactor(sum);
}
for(int i = 0; i < n; i++)
printf("%d\n",res[i]);
return 0;
}
Problem D: 已知某二叉树的先序序列和中序序列,编程计算并输出该二叉树的后序序列。
输入说明:仅一组数据,分为两行输入,第一行表示指定二叉树的先序序列,第二行表示该二叉树的中序序列,序列元素均为大写英文字符,表示二叉树的结点。
输出说明:在一行上输出该二叉树的后序序列。
输入样本:
ABDGCEFH
DGBAECHF
输出样本:
GDBEHFCA
#include <stdio.h>
#include <string.h>
struct Node{
Node *lchild;
Node *rchild;
char c;
}Tree[50];
int loc;
Node *creat(){
Tree[loc].lchild = Tree[loc].rchild = NULL;
return &Tree[loc++];
}
char str1[30],str2[30];
void PostOrder(Node *T){
if(T->lchild != NULL){
PostOrder(T->lchild);
}
if(T->rchild != NULL){
PostOrder(T->rchild);
}
printf("%c",T->c);
}
Node *build(int s1,int e1,int s2,int e2){
Node *ret = creat();
ret->c = str1[s1];
int rootIdx;
for(int i = s2; i <= e2; i++){
if(str2[i] == str1[s1]){
rootIdx = i;
break;
}
}
if(rootIdx != s2){
ret->lchild = build(s1+1,s1+(rootIdx-s2),s2,rootIdx-1);
}
if(rootIdx != e2){
ret->rchild = build(s1+(rootIdx-s2)+1,e1,rootIdx+1,e2);
}
return ret;
}
int main(){
while(scanf("%s",str1) != EOF){
scanf("%s",str2);
loc = 0;
int L1 = strlen(str1);
int L2 = strlen(str2);
Node *T = build(0,L1-1,0,L2-1);
PostOrder(T);
printf("\n");
}
return 0;
}

Problem E:请写一个程序,判断给定表达式中的括号是否匹配,表达式中的合法括号为”(“, “)”, “[", "]“, “{“, ”}”,这三个括号可以按照任意的次序嵌套使用。
输入说明:有多个表达式,输入数据的第一行是表达式的数目,每个表达式占一行。
输出说明:对每个表达式,若其中的括号是匹配的,则输出”yes”,否则输出”no”。
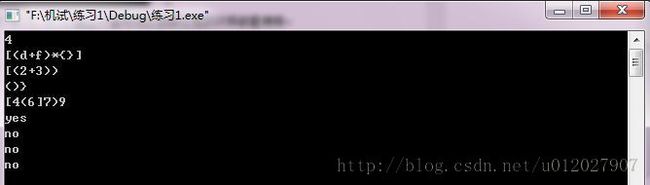
输入样本:
4
[(d+f)*{}]
[(2+3))
()}
[4(6]7)9
输出样本:
yes
no
no
no
#include <stdio.h>
#include <string.h>
#include <stack>
using namespace std;
#define N 100
int ans[N];
char buf[N][N];
int match(char exp[],int n){
stack<char> s;
int i = 0,tag = 1;
while(i < n && tag == 1){
if(exp[i] == '(' || exp[i] == '[' || exp[i] == '{'){
s.push(exp[i]);
}
else if(exp[i] == ')'){
if(s.empty()){
tag = 0;
break;
}
if(s.top() == '('){
s.pop();
}
else
tag = 0;
}
else if(exp[i] == ']'){
if(s.empty()){
tag = 0;
break;
}
if(s.top() == '['){
s.pop();
}
else
tag = 0;
}
else if(exp[i] == '}'){
if(s.empty()){
tag = 0;
break;
}
if(s.top() == '{'){
s.pop();
}
else
tag = 0;
}
i++;
}
if(s.empty() == false)
tag = 0;
return tag;
}
int main(){
int n;
while(scanf("%d",&n) != EOF){
for(int i = 0; i < n; i++)
scanf("%s",buf[i]);
int cnt = 0;
for(i = 0; i < n; i++){
int len = strlen(buf[i]);
if(match(buf[i],len))
ans[cnt++] = 1;
else
ans[cnt++] = 0;
}
for(i = 0; i < cnt; i++)
if(ans[i])
printf("yes\n");
else
printf("no\n");
}
return 0;
}
转载请标明出处:牟尼的专栏 http://blog.csdn.net/u012027907