【POJ 1191】 棋盘分割（DP）
【POJ 1191】 棋盘分割（DP）
| Time Limit: 1000MS | Memory Limit: 10000K | |
| Total Submissions: 13811 | Accepted: 4917 |
Description
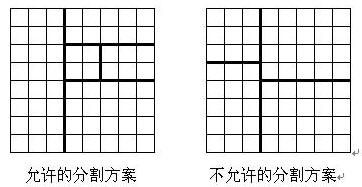
原棋盘上每一格有一个分值，一块矩形棋盘的总分为其所含各格分值之和。现在需要把棋盘按上述规则分割成n块矩形棋盘，并使各矩形棋盘总分的均方差最小。
均方差
| Time Limit: 1000MS | Memory Limit: 10000K | |
| Total Submissions: 13811 | Accepted: 4917 |
Description
原棋盘上每一格有一个分值，一块矩形棋盘的总分为其所含各格分值之和。现在需要把棋盘按上述规则分割成n块矩形棋盘，并使各矩形棋盘总分的均方差最小。
均方差 ，其中平均值
，x
i为第i块矩形棋盘的总分。
请编程对给出的棋盘及n，求出O'的最小值。
Input
第2行至第9行每行为8个小于100的非负整数，表示棋盘上相应格子的分值。每行相邻两数之间用一个空格分隔。
Output
Sample Input
3 1 1 1 1 1 1 1 3 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 0 1 1 1 1 1 1 0 3
Sample Output
1.633
Source
[Submit] [Go Back] [Status] [Discuss]
Home Page
Go Back 
To top
，其中平均值
，x
i为第i块矩形棋盘的总分。
请编程对给出的棋盘及n，求出O'的最小值。
Input
第2行至第9行每行为8个小于100的非负整数，表示棋盘上相应格子的分值。每行相邻两数之间用一个空格分隔。
Output
Sample Input
3 1 1 1 1 1 1 1 3 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 0 1 1 1 1 1 1 0 3
Sample Output
1.633
Source
刷了一圈，刷回到最原始的东西。其实也是学不完的东西。。。DP……花样太多了，烧脑特辑。。。
题目很简单粗暴
8*8的一个正方形，每个点有值(0~100)要求切割n-1次，每次只能从余下部分的边出发切割到另一边，题目中的图画的挺清楚的。然后问最小的方差。。。
方差公式是给了。但如果死盯着这公式。。想再久应该也是想不粗来的……
需要变一下形。就借用别的博客的推导了～～
S^2 = 1/n∑(Xi - X)^2
= 1/n(n*X^2 + ∑Xi^2 - 2*X∑Xi)
= 1/n∑Xi^2 - X^2;
（http://blog.csdn.net/xymscau/article/details/6854410）
这样其实应该就有点小想法了～你会发现X（均值）是固定的(sum/n)，只要保证∑Xi^2尽可能小，最终的结果就一定是最小值。并且可以发现S^2不会出现负值，也就是说∑Xi^2再小也比X^2要大，因此就可以放心地dp了。或者说，放心地(记忆化)搜索了，每次切割时，继续搜索的部分继续搜，另一部分需要快速给出一个合值，因此可以预处理一下所有的区间的平方，或者每次用到暴力跑也可以。
需要注意的是切出n个矩形要切n-1刀。
还有，dp数组要记得记录切割刀数。。一开始就是因为没记录，导致混乱，最终WA
另外，dp最先初始化的时候不要初始化为INF，因为可能某些部分最终结果就是INF，也就是无解，如果一开始初始化为INF，并且以这为记忆化返回值的判断，会导致超时。因为到此状态时仍会向下dp。正解应为代码中初始化未-1，当发现是第一次搜索该状态时，立马转变为INF，然后搜出该状态最小值(若不合法，恰巧就是上一步赋值的INF(无穷大))
代码如下：
#include <iostream>
#include <cmath>
#include <vector>
#include <cstdlib>
#include <cstdio>
#include <cstring>
#include <queue>
#include <stack>
#include <list>
#include <algorithm>
#include <map>
#include <set>
#define LL long long
#define Pr pair<int,int>
#define fread() freopen("in.in","r",stdin)
#define fwrite() freopen("out.out","w",stdout)
using namespace std;
const int INF = 0x3f3f3f3f;
const int msz = 10000;
const int mod = 1e9+7;
const double eps = 1e-8;
double arg;
int n;
int mp[8][8];
int dp[16][8][8][8][8];
int sum[8][8][8][8];
void init()
{
for(int x1 = 0; x1 < 8; ++x1)
for(int y1 = 0; y1 < 8; ++y1)
for(int x2 = x1; x2 < 8; ++x2)
for(int y2 = y1; y2 < 8; ++y2)
{
int tmp = 0;
for(int i = x1; i <= x2; ++i)
for(int j = y1; j <= y2; ++j)
tmp += mp[i][j];
sum[x1][y1][x2][y2] = tmp*tmp;
//printf("sum[%d][%d][%d][%d]:%d\n",x1,y1,x2,y2,sum[x1][y1][x2][y2]);
}
}
int dfs(int s,int x1,int y1,int x2,int y2)
{
if(s == n) return sum[x1][y1][x2][y2];
if(x1 == x2 && y1 == y2) return INF;
if(~dp[s][x1][y1][x2][y2]) return dp[s][x1][y1][x2][y2];
dp[s][x1][y1][x2][y2] = INF;
for(int i = x1; i < x2; ++i)
{
int tmp = min(dfs(s+1,x1,y1,i,y2)+sum[i+1][y1][x2][y2],sum[x1][y1][i][y2]+dfs(s+1,i+1,y1,x2,y2));
//printf("(%d,%d),(%d,%d)->(%d,%d),(%d,%d) %d\n",x1,y1,i,y2,i+1,y1,x2,y2,tmp);
dp[s][x1][y1][x2][y2] = min(dp[s][x1][y1][x2][y2],tmp);
}
for(int i = y1; i < y2; ++i)
{
int tmp = min(dfs(s+1,x1,y1,x2,i)+sum[x1][i+1][x2][y2],sum[x1][y1][x2][i]+dfs(s+1,x1,i+1,x2,y2));
//printf("(%d,%d),(%d,%d)->(%d,%d),(%d,%d) %d\n",x1,y1,x2,i,x1,i+1,x2,y2,tmp);
dp[s][x1][y1][x2][y2] = min(dp[s][x1][y1][x2][y2],tmp);
}
return dp[s][x1][y1][x2][y2];
}
int main()
{
//fread();
//fwrite();
while(~scanf("%d",&n))
{
arg = 0;
for(int i = 0; i < 8; ++i)
for(int j = 0; j < 8; ++j)
{
scanf("%d",&mp[i][j]);
arg += mp[i][j];
}
memset(dp,-1,sizeof(dp));
init();
dfs(1,0,0,7,7);
//printf("%d\n",dp[0][0][7][7]);
//printf("%f\n",arg);
printf("%.3f\n",sqrt(dp[1][0][0][7][7]*1.0/n-arg/n*(arg*1.0/n)));
}
return 0;
}