- MongoDB知识概括
GeorgeLin98
持久层mongodb
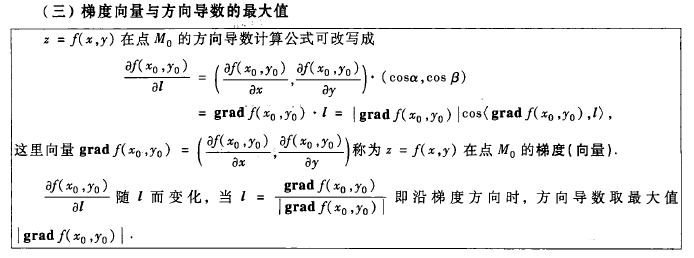
MongoDB知识概括MongoDB相关概念单机部署基本常用命令索引-IndexSpirngDataMongoDB集成副本集分片集群安全认证MongoDB相关概念业务应用场景:传统的关系型数据库(如MySQL),在数据操作的“三高”需求以及应对Web2.0的网站需求面前,显得力不从心。解释:“三高”需求:①Highperformance-对数据库高并发读写的需求。②HugeStorage-对海量数
- ERROR 1064 (42000): You have an error in your SQL syntax; check the manual that corresponds to your
†徐先森®
Oracle数据库Web相关错误集
createtablestudents(idintunsignedprimarykeyauto_increment,namevarchar(50)notnull,ageintunsigned,highdecimal(3,2),genderenum('男','女','中性','保密','妖')default'保密',cls_idintunsigned);在对数据库插入如上带有中文带有默认值的字段的时
- Vicky的ScalersTalk第六轮新概念朗读持续力训练Day73 20210411
Vicky_b9de
练习材料:ModerncavemenPart-3ˈmɒdənˈkeɪvmənpɑːt-3Theyplungedintothelake,andafterloadingtheirgearonaninflatablerubberdinghy,letthecurrentcarrythemtotheotherside.Toprotectthemselvesfromtheicywater,theyhadtow
- leetcode刷题day19|二叉树Part07(235. 二叉搜索树的最近公共祖先、701.二叉搜索树中的插入操作、450.删除二叉搜索树中的节点)
小冉在学习
leetcode算法数据结构
235.二叉搜索树的最近公共祖先思路:二叉搜索树首先考虑中序遍历。根据二叉搜索树的特性,如果p,q分别在中间节点的左右两边,该中间节点一定是最近公共祖先,如果在同一侧,则递归这一侧即可。递归三部曲:1、传入参数:根节点,p,q,返回节点。2、终止条件:因为p,q一定存在,所以不会遍历到树的最底层,因此可以不写终止条件3、递归逻辑:如果p,q均小于root的值,递归调用左子树;如果p,q均大于roo
- golang 实现文件上传下载
wangwei830
go
Gin框架上传下载上传(支持批量上传)httpRouter.POST("/upload",func(ctx*gin.Context){forms,err:=ctx.MultipartForm()iferr!=nil{fmt.Println("error",err)}files:=forms.File["fileName"]for_,v:=rangefiles{iferr:=ctx.SaveUplo
- 自定义分区
我的K8409
Hadoophdfshadoop大数据
通过简单例子了解partition分区类的重写方法分区是在MR的过程中进行的,属于Shuffle阶段但是在Job端不要忘记进行调用:job.setPartitionerClass(xxx.class)按照年龄分区:classAgePartitionerextendsPartitioner{@OverridepublicintgetPartition(MyComparablekey,NullWrit
- leetcode刷题day13|二叉树Part01(递归遍历、迭代遍历、统一迭代、层序遍历)
小冉在学习
leetcode算法职场和发展
递归遍历思路:使用递归的方式比较简单。1、递归函数的传参:因为最后输出一个数组,所以需要传入根节点和一个容器,本来想写数组,但发现长度不能确定,所以选择list。2、终止条件:当访问的节点为空时,return3、递归函数的逻辑:先访问一个节点,递归访问其他节点144.二叉树的前序遍历代码如下:classSolution{publicListpreorderTraversal(TreeNoderoo
- 101个浪漫的点子..哈哈有需要可以试试...中英对照~
Hecks
学习心得IDEAUPGo音乐网页游戏
Thisisafreebonusversionof101RomanticIdeas.Feelfreetoforwardtoormakecopiesforyourfriends.下面是101个浪漫的点子。可随意转发给你的朋友们IDEA#1点子1Ifyourpartnerisgoingawayforafewdays,tellherthatyouareworriedabouthersoyouhaveor
- py获取系统缩放比例
xu-jssy
Python自动化脚本python前端
fromwin32.libimportwin32conimportwin32api,win32gui,win32printscaling=1.0defget_real_resolution():"""获取真实的分辨率"""hDC=win32gui.GetDC(0)wide=win32print.GetDeviceCaps(hDC,win32con.DESKTOPHORZRES)high=win32
- CTF常见编码及加解密(超全)第二篇
不会代码的小徐
编码密码网络安全密码学预编码
HTML实体编码简述:字符实体是用一个编号写入HTML代码中来代替一个字符,在使用浏览器访问网页时会将这个编号解析还原为字符以供阅读。举例:highlighter-HTML明文:hello,world.十进制:hello,world.十六进制:hel
- FPGA器件在线配置方法概述
fpga和matlab
FPGA其他fpga开发FPGA在线配置
目录1.配置电路结构和原理2.ICR控制电路软件3.几种常见的FPGA在线配置方法3.1动态部分重配置(PartialReconfiguration,PR)3.2在系统编程(In-SystemProgramming,ISP)3.3多比特流配置(Multi-BitstreamConfiguration)3.4远程更新与配置3.5使用OpenCL或HLS工具FPGA(Field-Programmabl
- LVGL 控件之列表(lv_list)
Projectsauron
#LVGlLVGL嵌入式
目录一、概述二、列表1、添加列表按钮2、设置列表文本3、API函数一、概述List(列表)基本上是一个垂直布局的矩形,按钮指向该矩形并且可以添加文本。列表部件由两个部分组成:LV_PART_MAIN使用所有典型背景属性的列表的主要部分LV_PART_SCROLLBAR滚动条。二、列表1、添加列表按钮在默认的情况下,列表部件被创建出来后,只有一个矩形背景框,并没有任何的文本和按钮,用户需要自行往列表
- MySQL MHA
pokemon..
mysql数据库
一、MySQLMHA高可用环境概述1.什么是MHAMHA(MasterHighAvailability)是一款用于MySQL数据库集群的高可用解决方案,主要解决MySQL单点故障问题。在MySQL集群的主服务器发生故障时,MHA可以自动执行故障切换,将一个从服务器提升为新的主服务器,最大限度地保证数据的一致性。MHA的切换时间通常在0-30秒之间,极大提高了MySQL集群的可用性。2.MHA的组成
- 商务英语 Level 2 Unit 1 Part 2
流非沫
Listening【DailySchedule】Maryisanemployeeatarealestatecompany.Thisisherdailyworkschedule.ShegoestoworkfromMondaytoFriday.Sheusuallyworksfrom9a.m.to5p.m.Shegetsupat7:30inthemorning.Sheleavesforworkat8:1
- 技术周总结 09.09~09.15周日(C# WinForm WPF)
打破砂锅问到底007
wpfc#WinForm
文章目录一、09.09周一1.1)问题01:Windows桌面开发中,WPF和WinForm的区别和联系?联系:区别:二、09.12周四2.1)问题01:visualstudio的相关快捷键有哪些?通用快捷键编辑导航调试窗口管理2.2)问题02:publicpartialclassChoosePLReason:CommonBaseForm2.3)问题03:介绍WindowsForms中的Syste
- Python 报错 ImportError: cannot import name xxx from partially initialized module xxx
SmallerFL
其他问题fixPython相关python深度学习pytorch人工智能
文章目录1.报错2.原因3.参考1.报错ImportError:cannotimportname'SummaryWriter'frompartiallyinitializedmodule'torch.utils.tensorboard'(mostlikelyduetoacircularimport)(/Library/Frameworks/Python.framework/Versions/3.1
- HighCharts图表自动化简介
知识的宝藏
Selenium高级篇Selenium图表自动化测试highcharts图表自动化Selenium图表自动化图表自动化测试highchartsSelenium
什么是分析数据?在任何应用程序中捕获并以图形或图表形式显示的分析数据是任何产品或系统的关键部分,因为它提供了对实时数据的洞察。验证此类分析数据非常重要,因为不准确的数据可能会在报告中产生问题,并可能影响应用程序/系统的其他相关领域。什么是HighChart?Highcharts是一个用纯JavaScript编写的j基于SVG成图技术的图表库,提供了一种简单的方法来向您的网站或Web应用程序添加交互
- SIP RFC 关系图汇总--超全---part3
jenny_yu1025
RFCSIPsessionextensionprotocolsnetworktransitionauthorization
SIPRFC关系图汇总--超全---part3Topp.13261-32xx33xx34xx35xx36xx37xx38xx39xxPrevNext40xx41xx42xx43xx44xx45xx46xx47xx48xx49xx50xx51xx52xx53xx54xx55xx56xx57xx58xx59xx60xx61xx62xxBefore326140xx#RFC4028SessionTimer
- Java中将 File对象转MultipartFile对象 File >>> MultipartFile
奔腾的隔壁老王
javaspring开发语言
Java中将File类型的对象转换为MultipartFile类型的对象所需要的包:importjava.io.FileInputStream;importorg.springframework.web.multipart.MultipartFile;实现代码://传入一个File对象,获得一个转换后的MultipartFile对象publicMultipartFilegetMultipartFi
- Java File与MultipartFile互转
我在Java摸滚打趴
Java开发工具类java
pom中添加依赖commons-fileuploadcommons-fileupload1.4File转MultipartFile/***File转MultipartFile**@paramfile*@return*/publicstaticMultipartFilecreateFileItem(Filefile){FileItemFactoryfactory=newDiskFileItemFac
- Java url转MultipartFile inputStream转File file转multipartFile
Abel_JiaWei
java
Javaurl转MultipartFileinputStream转Filefile转multipartFile/***url转MultipartFile*@paramurl*@return*@throwsException*/publicstaticMultipartFileurlToMultipartFile(Stringurl)throwsException{Filefile=null;Mul
- 【Java】如何将二进制转换成MultipartFile
Mxin5
Javajava开发语言
业务场景:前端发送请求到后端进行文件上传,后端接收文件并调用第三方接口进行文件处理,响应格式为二进制,然后我们需要将二进制进行转换为MultipartFile进行文件上传。如果你想要将File转换成MultipartFile,可以参考:【Java】如何将File转换成MultipartFile_javafile转multipartfile_MXin5的博客-CSDN博客1.传递二进制respons
- 看《绝望主妇》学英语
高雅_1f79
第四季第九集剧集连接https://www.imeiju.cc/Play/3543-1-8.html单词1.tendverbUS/tend/1)tendverb(BELIKELY)趋向;倾向于[I]tobelikelytohappenortohaveaparticularcharacteristicoreffect:Wetendtoeatathome.Childrentendtobelikethe
- 【Java将File完美转为MultipartFile】:亲测有效
阿火~
java
由于multipartFile有很多有用的api,但是有时候我们接收到前台的参数是文件名和文件内容,而不是文件,此时不能使用multipartFile接收,导致处理起来很不方便,比如我用minio上传文件,minio需要multipartFile类型的文件,而我拿到前端传来的文件名和文件内容只能自己通过File类创建文件,所以如果想用multipartFile类型就需要自己转换,然而天不遂人愿,并
- 美国专利申请常用加快审查程序
察尔斯格伦
美国专利商标局(USPTO)针对专利申请人的不同需求开展了多种方式的加快审查。今天我们来聊一聊更为常用的专利申请高速公路(PatentProsecutionHighway,PPH)和优先审查(PrioritizedExamination,TrackOne)程序。一、专利申请高速公路(PPH)专利申请高速公路是适用于不同国家的知识产权局之间的加速审查通道,其根据是美国参与的双边和多边协议。根据专利申
- 2019-05-28 女性财富力作业
唐平子
Part1:理解和感悟三个核心习惯。1.我经手的任何事情都是我的个人品牌,我百分之百的为之负责。(1)对于这一点我感触最深的是对自己的个人职业发展负责。在过去,我总是依赖于领导给我分配任务,即便是每年年中个人发展回顾的时候,也没有认真思考和做功课。后面我意识到了这个问题,并做了一些调整。前段时间,我得知我们即将来一个项目,其中一个重要的角色是空缺的,通过跟有经验的同事交流,我发现这是一条新的业务线
- 软件架构,一切尽在权衡
元闰子
架构
荐语本文要介绍的是2021年O’Reilly出版的书籍SoftwareArchitecture:TheHardParts(后文简称SAHP),某种程度上,它是FundamentalsofSoftwareArchitecture(后文简称FSA)的延续,两本书的主要作者相同。FSA是基础篇,重点介绍了常见的8种架构模式;SAHP是进阶篇,重点介绍了如何从单体架构演进到分布式架构。软件架构,特别是分布
- MySQL数据库——多表查询
L_earning_
MySQL数据库mysqlsql
本文采用SQL99语法。所适用的相关例子及数据来源于尚硅谷。写在前面为什么需要建立多个数据表?减少数据冗余提高运行速度减轻数据库维护工作量提高工作效率,多个数据表可以同时被调用等一、多表查询1.1多表查询的分类1.1.1等值连接vsvsvs非等值连接等值连接#查询员工的employee_id,last_name,department_name,citySELECTemp.employee_id,e
- 【笔记】扩散模型(七):Latent Diffusion Models(Stable Diffusion)论文解读与代码实现
LittleNyima
DiffusionModels笔记stablediffusionAIGC人工智能
论文链接:High-ResolutionImageSynthesiswithLatentDiffusionModels官方实现:CompVis/latent-diffusion、CompVis/stable-diffusion这一篇文章的内容是LatentDiffusionModels(LDM),也就是大名鼎鼎的StableDiffusion。先前的扩散模型一直面临的比较大的问题是采样空间太大,学
- 泽平 的ScalersTalk第六轮新概念朗读持续力训练Day 75 20210606
郑泽平
练习材料:Lesson74OutofthelimelightAnancientbusstoppedbyadryriverbedandapartyoffamousactorsandactressesgotoff.Dressedindarkglassesandoldclothes,theyhadtakenspecialprecautionssothatnooneshouldrecognizethem.
- Java常用排序算法/程序员必须掌握的8大排序算法
cugfy
java
分类:
1)插入排序(直接插入排序、希尔排序)
2)交换排序(冒泡排序、快速排序)
3)选择排序(直接选择排序、堆排序)
4)归并排序
5)分配排序(基数排序)
所需辅助空间最多:归并排序
所需辅助空间最少:堆排序
平均速度最快:快速排序
不稳定:快速排序,希尔排序,堆排序。
先来看看8种排序之间的关系:
1.直接插入排序
(1
- 【Spark102】Spark存储模块BlockManager剖析
bit1129
manager
Spark围绕着BlockManager构建了存储模块,包括RDD,Shuffle,Broadcast的存储都使用了BlockManager。而BlockManager在实现上是一个针对每个应用的Master/Executor结构,即Driver上BlockManager充当了Master角色,而各个Slave上(具体到应用范围,就是Executor)的BlockManager充当了Slave角色
- linux 查看端口被占用情况详解
daizj
linux端口占用netstatlsof
经常在启动一个程序会碰到端口被占用,这里讲一下怎么查看端口是否被占用,及哪个程序占用,怎么Kill掉已占用端口的程序
1、lsof -i:port
port为端口号
[root@slave /data/spark-1.4.0-bin-cdh4]# lsof -i:8080
COMMAND PID USER FD TY
- Hosts文件使用
周凡杨
hostslocahost
一切都要从localhost说起,经常在tomcat容器起动后,访问页面时输入http://localhost:8088/index.jsp,大家都知道localhost代表本机地址,如果本机IP是10.10.134.21,那就相当于http://10.10.134.21:8088/index.jsp,有时候也会看到http: 127.0.0.1:
- java excel工具
g21121
Java excel
直接上代码,一看就懂,利用的是jxl:
import java.io.File;
import java.io.IOException;
import jxl.Cell;
import jxl.Sheet;
import jxl.Workbook;
import jxl.read.biff.BiffException;
import jxl.write.Label;
import
- web报表工具finereport常用函数的用法总结(数组函数)
老A不折腾
finereportweb报表函数总结
ADD2ARRAY
ADDARRAY(array,insertArray, start):在数组第start个位置插入insertArray中的所有元素,再返回该数组。
示例:
ADDARRAY([3,4, 1, 5, 7], [23, 43, 22], 3)返回[3, 4, 23, 43, 22, 1, 5, 7].
ADDARRAY([3,4, 1, 5, 7], "测试&q
- 游戏服务器网络带宽负载计算
墙头上一根草
服务器
家庭所安装的4M,8M宽带。其中M是指,Mbits/S
其中要提前说明的是:
8bits = 1Byte
即8位等于1字节。我们硬盘大小50G。意思是50*1024M字节,约为 50000多字节。但是网宽是以“位”为单位的,所以,8Mbits就是1M字节。是容积体积的单位。
8Mbits/s后面的S是秒。8Mbits/s意思是 每秒8M位,即每秒1M字节。
我是在计算我们网络流量时想到的
- 我的spring学习笔记2-IoC(反向控制 依赖注入)
aijuans
Spring 3 系列
IoC(反向控制 依赖注入)这是Spring提出来了,这也是Spring一大特色。这里我不用多说,我们看Spring教程就可以了解。当然我们不用Spring也可以用IoC,下面我将介绍不用Spring的IoC。
IoC不是框架,她是java的技术,如今大多数轻量级的容器都会用到IoC技术。这里我就用一个例子来说明:
如:程序中有 Mysql.calss 、Oracle.class 、SqlSe
- 高性能mysql 之 选择存储引擎(一)
annan211
mysqlInnoDBMySQL引擎存储引擎
1 没有特殊情况,应尽可能使用InnoDB存储引擎。 原因:InnoDB 和 MYIsAM 是mysql 最常用、使用最普遍的存储引擎。其中InnoDB是最重要、最广泛的存储引擎。她 被设计用来处理大量的短期事务。短期事务大部分情况下是正常提交的,很少有回滚的情况。InnoDB的性能和自动崩溃 恢复特性使得她在非事务型存储的需求中也非常流行,除非有非常
- UDP网络编程
百合不是茶
UDP编程局域网组播
UDP是基于无连接的,不可靠的传输 与TCP/IP相反
UDP实现私聊,发送方式客户端,接受方式服务器
package netUDP_sc;
import java.net.DatagramPacket;
import java.net.DatagramSocket;
import java.net.Ine
- JQuery对象的val()方法执行结果分析
bijian1013
JavaScriptjsjquery
JavaScript中,如果id对应的标签不存在(同理JAVA中,如果对象不存在),则调用它的方法会报错或抛异常。在实际开发中,发现JQuery在id对应的标签不存在时,调其val()方法不会报错,结果是undefined。
- http请求测试实例(采用json-lib解析)
bijian1013
jsonhttp
由于fastjson只支持JDK1.5版本,因些对于JDK1.4的项目,可以采用json-lib来解析JSON数据。如下是http请求的另外一种写法,仅供参考。
package com;
import java.util.HashMap;
import java.util.Map;
import
- 【RPC框架Hessian四】Hessian与Spring集成
bit1129
hessian
在【RPC框架Hessian二】Hessian 对象序列化和反序列化一文中介绍了基于Hessian的RPC服务的实现步骤,在那里使用Hessian提供的API完成基于Hessian的RPC服务开发和客户端调用,本文使用Spring对Hessian的集成来实现Hessian的RPC调用。
定义模型、接口和服务器端代码
|---Model
&nb
- 【Mahout三】基于Mahout CBayes算法的20newsgroup流程分析
bit1129
Mahout
1.Mahout环境搭建
1.下载Mahout
http://mirror.bit.edu.cn/apache/mahout/0.10.0/mahout-distribution-0.10.0.tar.gz
2.解压Mahout
3. 配置环境变量
vim /etc/profile
export HADOOP_HOME=/home
- nginx负载tomcat遇非80时的转发问题
ronin47
nginx负载后端容器是tomcat(其它容器如WAS,JBOSS暂没发现这个问题)非80端口,遇到跳转异常问题。解决的思路是:$host:port
详细如下:
该问题是最先发现的,由于之前对nginx不是特别的熟悉所以该问题是个入门级别的:
? 1 2 3 4 5
- java-17-在一个字符串中找到第一个只出现一次的字符
bylijinnan
java
public class FirstShowOnlyOnceElement {
/**Q17.在一个字符串中找到第一个只出现一次的字符。如输入abaccdeff,则输出b
* 1.int[] count:count[i]表示i对应字符出现的次数
* 2.将26个英文字母映射:a-z <--> 0-25
* 3.假设全部字母都是小写
*/
pu
- mongoDB 复制集
开窍的石头
mongodb
mongo的复制集就像mysql的主从数据库,当你往其中的主复制集(primary)写数据的时候,副复制集(secondary)会自动同步主复制集(Primary)的数据,当主复制集挂掉以后其中的一个副复制集会自动成为主复制集。提供服务器的可用性。和防止当机问题
mo
- [宇宙与天文]宇宙时代的经济学
comsci
经济
宇宙尺度的交通工具一般都体型巨大,造价高昂。。。。。
在宇宙中进行航行,近程采用反作用力类型的发动机,需要消耗少量矿石燃料,中远程航行要采用量子或者聚变反应堆发动机,进行超空间跳跃,要消耗大量高纯度水晶体能源
以目前地球上国家的经济发展水平来讲,
- Git忽略文件
Cwind
git
有很多文件不必使用git管理。例如Eclipse或其他IDE生成的项目文件,编译生成的各种目标或临时文件等。使用git status时,会在Untracked files里面看到这些文件列表,在一次需要添加的文件比较多时(使用git add . / git add -u),会把这些所有的未跟踪文件添加进索引。
==== ==== ==== 一些牢骚
- MySQL连接数据库的必须配置
dashuaifu
mysql连接数据库配置
MySQL连接数据库的必须配置
1.driverClass:com.mysql.jdbc.Driver
2.jdbcUrl:jdbc:mysql://localhost:3306/dbname
3.user:username
4.password:password
其中1是驱动名;2是url,这里的‘dbna
- 一生要养成的60个习惯
dcj3sjt126com
习惯
一生要养成的60个习惯
第1篇 让你更受大家欢迎的习惯
1 守时,不准时赴约,让别人等,会失去很多机会。
如何做到:
①该起床时就起床,
②养成任何事情都提前15分钟的习惯。
③带本可以随时阅读的书,如果早了就拿出来读读。
④有条理,生活没条理最容易耽误时间。
⑤提前计划:将重要和不重要的事情岔开。
⑥今天就准备好明天要穿的衣服。
⑦按时睡觉,这会让按时起床更容易。
2 注重
- [介绍]Yii 是什么
dcj3sjt126com
PHPyii2
Yii 是一个高性能,基于组件的 PHP 框架,用于快速开发现代 Web 应用程序。名字 Yii (读作 易)在中文里有“极致简单与不断演变”两重含义,也可看作 Yes It Is! 的缩写。
Yii 最适合做什么?
Yii 是一个通用的 Web 编程框架,即可以用于开发各种用 PHP 构建的 Web 应用。因为基于组件的框架结构和设计精巧的缓存支持,它特别适合开发大型应
- Linux SSH常用总结
eksliang
linux sshSSHD
转载请出自出处:http://eksliang.iteye.com/blog/2186931 一、连接到远程主机
格式:
ssh name@remoteserver
例如:
ssh
[email protected]
二、连接到远程主机指定的端口
格式:
ssh name@remoteserver -p 22
例如:
ssh i
- 快速上传头像到服务端工具类FaceUtil
gundumw100
android
快速迭代用
import java.io.DataOutputStream;
import java.io.File;
import java.io.FileInputStream;
import java.io.FileNotFoundException;
import java.io.FileOutputStream;
import java.io.IOExceptio
- jQuery入门之怎么使用
ini
JavaScripthtmljqueryWebcss
jQuery的强大我何问起(个人主页:hovertree.com)就不用多说了,那么怎么使用jQuery呢?
首先,下载jquery。下载地址:http://hovertree.com/hvtart/bjae/b8627323101a4994.htm,一个是压缩版本,一个是未压缩版本,如果在开发测试阶段,可以使用未压缩版本,实际应用一般使用压缩版本(min)。然后就在页面上引用。
- 带filter的hbase查询优化
kane_xie
查询优化hbaseRandomRowFilter
问题描述
hbase scan数据缓慢,server端出现LeaseException。hbase写入缓慢。
问题原因
直接原因是: hbase client端每次和regionserver交互的时候,都会在服务器端生成一个Lease,Lease的有效期由参数hbase.regionserver.lease.period确定。如果hbase scan需
- java设计模式-单例模式
men4661273
java单例枚举反射IOC
单例模式1,饿汉模式
//饿汉式单例类.在类初始化时,已经自行实例化
public class Singleton1 {
//私有的默认构造函数
private Singleton1() {}
//已经自行实例化
private static final Singleton1 singl
- mongodb 查询某一天所有信息的3种方法,根据日期查询
qiaolevip
每天进步一点点学习永无止境mongodb纵观千象
// mongodb的查询真让人难以琢磨,就查询单天信息,都需要花费一番功夫才行。
// 第一种方式:
coll.aggregate([
{$project:{sendDate: {$substr: ['$sendTime', 0, 10]}, sendTime: 1, content:1}},
{$match:{sendDate: '2015-
- 二维数组转换成JSON
tangqi609567707
java二维数组json
原文出处:http://blog.csdn.net/springsen/article/details/7833596
public class Demo {
public static void main(String[] args) { String[][] blogL
- erlang supervisor
wudixiaotie
erlang
定义supervisor时,如果是监控celuesimple_one_for_one则删除children的时候就用supervisor:terminate_child (SupModuleName, ChildPid),如果shutdown策略选择的是brutal_kill,那么supervisor会调用exit(ChildPid, kill),这样的话如果Child的behavior是gen_