poj 2506 递推+Java大数
Description
In how many ways can you tile a 2xn rectangle by 2x1 or 2x2 tiles?
Here is a sample tiling of a 2x17 rectangle.
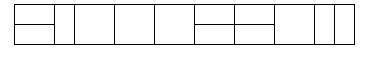
Here is a sample tiling of a 2x17 rectangle.

Input
Input is a sequence of lines, each line containing an integer number 0 <= n <= 250.
Output
For each line of input, output one integer number in a separate line giving the number of possible tilings of a 2xn rectangle.
Sample Input
2 8 12 100 200
Sample Output
3 171 2731 845100400152152934331135470251 1071292029505993517027974728227441735014801995855195223534251
分析:
题目大意就是有2×1和2×2两种规格的地板,现要拼2×n的形状,共有多少种情况,首先要做这道题目要先对递推有一定的了解。
假设我们已经铺好了2×(n-1)的情形,则要铺到2×n则只能用2×1的地板
假设我们已经铺好了2×(n-2)的情形,则要铺到2×n则可以选择1个2×2或两个2×1,故可能有下列三种铺法
其中要注意到第三个会与铺好2×(n-1)的情况重复,故不可取,故可以得到递推式
a[i]=2*a[i-2]+a[i-1];
有了递推公式,直接用java大数做就好:
import java.io.*;
import java.math.*;
import java.util.*;
public class Main{
static public void main(String[] args) {
Scanner cin=new Scanner(new BufferedInputStream(System.in));
BigInteger [] a=new BigInteger[255];
a[0]=a[1]=BigInteger.ONE;
a[2]=a[1].add(a[0].add(a[0]));
for(int i=3;i<252;i++){
a[i]=a[i-1].add(a[i-2].add(a[i-2]));
}
int n;
while(cin.hasNext()){
n=cin.nextInt();
System.out.println(a[n]);
}
}
}