poj 1324 Holedox Moving
Holedox Moving
| Time Limit: 5000MS | Memory Limit: 65536K | |
| Total Submissions: 12041 | Accepted: 2884 |
Description
During winter, the most hungry and severe time, Holedox sleeps in its lair. When spring comes, Holedox wakes up, moves to the exit of its lair, comes out, and begins its new life.
Holedox is a special snake, but its body is not very long. Its lair is like a maze and can be imagined as a rectangle with n*m squares. Each square is either a stone or a vacant place, and only vacant places allow Holedox to move in. Using ordered pair of row and column number of the lair, the square of exit located at (1,1).
Holedox's body, whose length is L, can be represented block by block. And let B1(r1,c1) B2(r2,c2) .. BL(rL,cL) denote its L length body, where Bi is adjacent to Bi+1 in the lair for 1 <= i <=L-1, and B1 is its head, BL is its tail.
To move in the lair, Holedox chooses an adjacent vacant square of its head, which is neither a stone nor occupied by its body. Then it moves the head into the vacant square, and at the same time, each other block of its body is moved into the square occupied by the corresponding previous block.
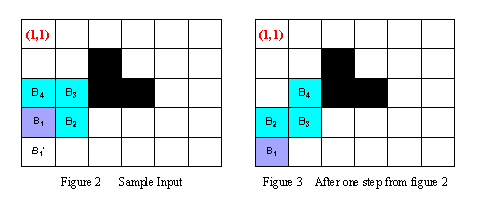
For example, in the Figure 2, at the beginning the body of Holedox can be represented as B1(4,1) B2(4,2) B3(3,2)B4(3,1). During the next step, observing that B1'(5,1) is the only square that the head can be moved into, Holedox moves its head into B1'(5,1), then moves B2 into B1, B3 into B2, and B4 into B3. Thus after one step, the body of Holedox locates in B1(5,1)B2(4,1)B3(4,2) B4(3,2) (see the Figure 3).
Given the map of the lair and the original location of each block of Holedox's body, your task is to write a program to tell the minimal number of steps that Holedox has to take to move its head to reach the square of exit (1,1).
Holedox is a special snake, but its body is not very long. Its lair is like a maze and can be imagined as a rectangle with n*m squares. Each square is either a stone or a vacant place, and only vacant places allow Holedox to move in. Using ordered pair of row and column number of the lair, the square of exit located at (1,1).
Holedox's body, whose length is L, can be represented block by block. And let B1(r1,c1) B2(r2,c2) .. BL(rL,cL) denote its L length body, where Bi is adjacent to Bi+1 in the lair for 1 <= i <=L-1, and B1 is its head, BL is its tail.
To move in the lair, Holedox chooses an adjacent vacant square of its head, which is neither a stone nor occupied by its body. Then it moves the head into the vacant square, and at the same time, each other block of its body is moved into the square occupied by the corresponding previous block.
For example, in the Figure 2, at the beginning the body of Holedox can be represented as B1(4,1) B2(4,2) B3(3,2)B4(3,1). During the next step, observing that B1'(5,1) is the only square that the head can be moved into, Holedox moves its head into B1'(5,1), then moves B2 into B1, B3 into B2, and B4 into B3. Thus after one step, the body of Holedox locates in B1(5,1)B2(4,1)B3(4,2) B4(3,2) (see the Figure 3).
Given the map of the lair and the original location of each block of Holedox's body, your task is to write a program to tell the minimal number of steps that Holedox has to take to move its head to reach the square of exit (1,1).

Input
The input consists of several test cases. The first line of each case contains three integers n, m (1<=n, m<=20) and L (2<=L<=8), representing the number of rows in the lair, the number of columns in the lair and the body length of Holedox, respectively. The next L lines contain a pair of row and column number each, indicating the original position of each block of Holedox's body, from B1(r1,c1) to BL(rL,cL) orderly, where 1<=ri<=n, and 1<=ci<=m,1<=i<=L. The next line contains an integer K, representing the number of squares of stones in the lair. The following K lines contain a pair of row and column number each, indicating the location of each square of stone. Then a blank line follows to separate the cases.
The input is terminated by a line with three zeros.
Note: Bi is always adjacent to Bi+1 (1<=i<=L-1) and exit square (1,1) will never be a stone.
The input is terminated by a line with three zeros.
Note: Bi is always adjacent to Bi+1 (1<=i<=L-1) and exit square (1,1) will never be a stone.
Output
For each test case output one line containing the test case number followed by the minimal number of steps Holedox has to take. "-1" means no solution for that case.
Sample Input
5 6 4 4 1 4 2 3 2 3 1 3 2 3 3 3 3 4 4 4 4 2 3 1 3 1 4 2 4 4 2 1 2 2 3 4 4 2 0 0 0
Sample Output
Case 1: 9 Case 2: -1
Hint
In the above sample case, the head of Holedox can follows (4,1)->(5,1)->(5,2)->(5,3)->(4,3)->(4,2)->(4,1)->(3,1)->(2,1)->(1,1) to reach the square of exit with minimal number of step, which is nine
/*这一题特坑,用g++交错了8次,然后用C++提交过了,把我气死了,在这一题中,主要是要把
蛇的不同状态记录下来,也就是用visit[25][25][17000],第三个参数是蛇的状态*/
#include <iostream>
#include<stdio.h>
#include<cstring>
using namespace std;
int lair[10][2][60000],map[25][25],n,m,l,sx,sy,dir[4][2]={{-1,0},{0,1},{1,0},{0,-1}},visit[25][25][17000];
struct tere{int x, y;int step;}queue[600000];
int state (int w)//根据蛇的坐标,得出蛇的状态
{
int i,x,y,primex,primey,sum;
primex=lair[0][0][w];
primey=lair[0][1][w];
sum=0;
for(i=1;i<l;i++)
{
x=lair[i][0][w];
y=lair[i][1][w];
if(primex==x)
{
if(primey==y+1)
{
sum=sum<<2;
}
else if(primey==y-1)
{
sum=sum<<2|1;
}
}
else if(primey==y)
{
if(primex==x+1)
{
sum=(sum<<1|1)<<1;
}
else if(primex==x-1)
{
sum=(((sum<<1)|1)<<1)|1;
}
}
primex=x;
primey=y;
}
return sum;
}
bool can(int diri,int primx,int primy,int queuet)//判断能否走到下一步
{
int i,x,y;
x=primx+dir[diri][0];
y=primy+dir[diri][1];
if(x>=1&&x<=n&&y>=1&&y<=m&&(map[x][y]==0))
{
for(i=1;i<=l-1;i++)
{
if((x==lair[i][0][queuet])&&(y==lair[i][1][queuet]))//不碰到自已
{
return false;
}
}
return true;
}
return false;
}
int bfs()//常归搜索!
{
if((sx==1)&&(sy==1))
return 0;
int t,w,y,x,i,j,xx,yy;
t=w=1;
x=sx;
y=sy;
queue[1].x=sx;
queue[1].y=sy;
queue[1].step=0;
visit[sx][sy][state(1)]=1;
while(t<=w)
{
x=queue[t].x;
y=queue[t].y;
// printf("%dt\n",t);
if((x==1)&&(y==1))//注意在这里判断是否已经到了
return queue[t].step;
for(i=0;i<4;i++)
{
if(can(i,x,y,t))
{
xx=x+dir[i][0];
yy=y+dir[i][1];
queue[++w].x=xx;
queue[w].y=yy;
queue[w].step=queue[t].step+1;
//printf("mzn%d %d %d %d %d\n",i,x,y,t,w);
lair[0][0][w]=xx;
lair[0][1][w]=yy;
for(j=1;j<l;j++)//蛇的坐标变换
{
lair[j][0][w]=lair[j-1][0][t];
lair[j][1][w]=lair[j-1][1][t];
}
if(visit[xx][yy][state(w)])//用VISIT访问
w--;
else
visit[xx][yy][state(w)]=1;
}
}
t++;
}
return -1;
}
int main()
{
int tt=0,i,x,y,k,j;
while(scanf("%d%d%d",&n,&m,&l)!=EOF)
{
if(n==0&&m==0&&l==0)
break;
tt++;
for(i=0;i<=n;i++)
for(j=0;j<=m;j++)
{
map[i][j]=0;
for(k=0;k<=17000;k++)
{
visit[i][j][k]=0;
}
}
for(i=0;i<l;i++)
{
scanf("%d%d",&x,&y);
if(i==0)
{
sx=x;
sy=y;
}
lair[i][0][1]=x;
lair[i][1][1]=y;
}
scanf("%d",&k);
for(i=0;i<k;i++)
{
scanf("%d%d",&x,&y);
map[x][y]=1;
}
int re=bfs();
printf("Case %d: ",tt);
printf("%d\n",re);
}
return 0;
}