CodeForces - 977E，E. Cyclic Components
E. Cyclic Components
time limit per test
2 seconds
memory limit per test
256 megabytes
input
standard input
output
standard output
You are given an undirected graph consisting of nn vertices and mm edges. Your task is to find the number of connected components which are cycles.
Here are some definitions of graph theory.
An undirected graph consists of two sets: set of nodes (called vertices) and set of edges. Each edge connects a pair of vertices. All edges are bidirectional (i.e. if a vertex aa is connected with a vertex bb, a vertex bb is also connected with a vertex aa). An edge can't connect vertex with itself, there is at most one edge between a pair of vertices.
Two vertices uu and vv belong to the same connected component if and only if there is at least one path along edges connecting uu and vv.
A connected component is a cycle if and only if its vertices can be reordered in such a way that:
- the first vertex is connected with the second vertex by an edge,
- the second vertex is connected with the third vertex by an edge,
- ...
- the last vertex is connected with the first vertex by an edge,
- all the described edges of a cycle are distinct.
A cycle doesn't contain any other edges except described above. By definition any cycle contains three or more vertices.
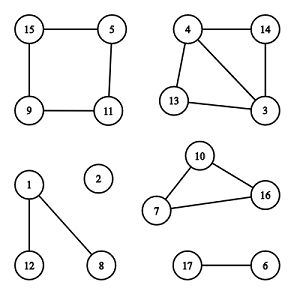There are 66 connected components, 22 of them are cycles: [7,10,16][7,10,16] and [5,11,9,15][5,11,9,15].
Input
The first line contains two integer numbers nn and mm (1≤n≤2⋅1051≤n≤2⋅105, 0≤m≤2⋅1050≤m≤2⋅105) — number of vertices and edges.
The following mm lines contains edges: edge ii is given as a pair of vertices vivi, uiui (1≤vi,ui≤n1≤vi,ui≤n, ui≠viui≠vi). There is no multiple edges in the given graph, i.e. for each pair (vi,uivi,ui) there no other pairs (vi,uivi,ui) and (ui,viui,vi) in the list of edges.
Output
Print one integer — the number of connected components which are also cycles.
Examples
input
Copy
5 4
1 2
3 4
5 4
3 5
output
Copy
1
input
Copy
17 15
1 8
1 12
5 11
11 9
9 15
15 5
4 13
3 13
4 3
10 16
7 10
16 7
14 3
14 4
17 6
output
Copy
2
Note
In the first example only component [3,4,5][3,4,5] is also a cycle.
The illustration above corresponds to the second example.
由于这种题做得少，虽然知道大体思路，但是具体到实施就不会了，于是看别人的代码 o#（这里、这里）#o 找想法
具体是，用dfs找环（vis标记），对于不成环或者环不标准的标记一下（cls），以后不会再找，能够成环的就加上去。
嗯，让我这么一写，运行时间是人家的代码的4倍，内存也大了1/4,，不过还好是一遍敲出来了。
#include
#include
#include
#include
#include
#include
using namespace std;
typedef long long ll;
vector Ed[200005];
vector vis,cls;
int flag;
void dfs(int cur)
{
if(flag)
return;
vis[cur]=1;
int t=Ed[cur].size();
if(t!=2)
{
flag=-1;
return;
}
for(int i=0;i>n>>m;
vis.resize(n+1);
cls.resize(n+1);
for(int i=0;i>x>>y;
Ed[x].push_back(y);
Ed[y].push_back(x);
}
ll ans=0;
for(int i=1;i<=n;i++)
{
if(!cls[i])
{
flag=0;
dfs(i);
ans+=max(0,flag);
clear(i);
}
}
cout<