Leetcode 股票合集 【121、122、714、309】

问题描述：【Math、DP】121. Best Time to Buy and Sell Stock

解题思路：

方法1（数学理解）：对于单次买入单次卖出股票问题，实际上是求后面一个数减去前面一个数的最大差值。因此，在遍历一遍的过程中，只需要维护一个最小值，和当前利润的最大值即可。时间复杂度 O(n)，空间复杂度 O(1)。

该问题的一般形式为： 求 A[j] - A[i] 的最大值，对于 j > i。

该问题的解决方式为：让 min_ 指向最小值 A[i]；在遍历的过程中更新 A[j] - min_ 的最大值。

Python3 实现：

class Solution:
    def maxProfit(self, prices):
        """
        :type prices: List[int]
        :rtype: int
        """
        if len(prices) == 0:
            return 0
        min_ = prices[0]
        ans = 0
        for i in range(1, len(prices)):
            ans = max(ans, prices[i] - min_)
            min_ = min(min_, prices[i])
        return ans

方法2（DP）：此题目和最大子段和 Leetcode 【53】给一个数组，求最大子段和 类似。

• 创建一个 dp[len(prices)]，其中 dp[i] 表示前 i 天的利润；
• 类似于最大字段和问题，容易找到转移方程 dp[i] = max(0, dp[i-1] + prices[i] - prices[i-1])，其中 0 表示利润出现负值时，这一天不买股票；
• max(dp) 就是最后的答案。

时间复杂度 O(n)，空间复杂度 O(n)（实际上空间复杂度也可以降为 O(1)，因为只用到前一天的结果）。

Python3 实现：

class Solution:
    def maxProfit(self, prices):
        """
        :type prices: List[int]
        :rtype: int
        """
        if len(prices) == 0:
            return 0
        dp = [0] * len(prices)
        for i in range(1, len(prices)):
            dp[i] = max(0, prices[i] - prices[i-1] + dp[i-1])
        return max(dp)

---

问题描述：【Greedy、DP、Math】122. Best Time to Buy and Sell Stock II

解题思路：

方法1（Greedy）：对于多次买入多次卖出股票问题，实际上是只要后面的数比前面的数大，就累加利润即可。这是一种贪心的思想。时间复杂度 O(n)，空间复杂度 O(1)。

Python3 实现：

class Solution:
    def maxProfit(self, prices):
        """
        :type prices: List[int]
        :rtype: int
        """
        ans = 0  # 最大利润
        for i in range(len(prices) - 1):
            if prices[i+1] > prices[i]:
                ans += prices[i+1] - prices[i]  # 累加利润
        return ans

方法2（DP）：当然，我们也可以使用动态规划求解。

• 创建一个 dp[len(prices)]，其中 dp[i] 表示前 i 天的最大利润；
• 容易找到转移方程 dp[i] = (dp[i-1] + prices[i] - prices[i-1]) if (prices[i] > prices[i-1]) else dp[i-1]；
• dp[-1] 就是最后的答案。

时间复杂度 O(n)，空间复杂度 O(n)（实际上空间复杂度也可以降为 O(1)，因为只用到前一天的结果）。

Python3 实现：

class Solution:
    def maxProfit(self, prices):
        """
        :type prices: List[int]
        :rtype: int
        """
        if len(prices) == 0:
            return 0
        dp = [0] * len(prices)
        for i in range(1, len(prices)):
            dp[i] = (dp[i-1] + prices[i] - prices[i-1]) if (prices[i] > prices[i-1]) else dp[i-1]
        return dp[-1]

方法3（数学理解）：

该问题的一般形式为： 将 A 划分为不重叠的 N 段，求这N 段 ∑ (A[j] - A[i]) 的最大值；每一段都有 j > i。

该问题的解决方式为：让 min_ 指向最小值 A[i]；在遍历的过程中，如果 A[j] > min_，则累加 A[j] - min_，同时更新 min_ 为 A[j]；否则，更新 min_ 为 min_ 和 A[j] 中的较小者。

Python3 实现：

class Solution:
    def maxProfit(self, prices):
        """
        :type prices: List[int]
        :rtype: int
        """
        if len(prices) == 0:
            return 0
        ans = 0
        min_ = prices[0]  # 第一个数为最小值
        for i in range(1, len(prices)):
            if prices[i] > min_:  # 可以卖股票
                ans += prices[i] - min_
                min_ = prices[i]
            else:  # 不能卖股票
                min_ = min(min_, prices[i])
        return ans

---

问题描述：【多状态DP、Math】714. Best Time to Buy and Sell Stock with Transaction Fee

解题思路：

方法1（双DP）：比起 Leetcode 122，这里多了一个买卖股票需要扣除费用。对于第 i 天来说，手中要么没有股票，要么手持一只股票。因此，可以采取两个 DP 数组，记录第 i 天持有股票或者第 i 天不持有股票的最大收益。

• 用 nohold 表示第 i 天不持有股票的收益，hold 表示第 i 天持有一只股票的收益；
• 未持有股票的情况：要么是前一天就没有股票，要么是今天卖掉了，所以这种情况下，未持有股票的最大收益就是这两者的最大值，即 nohold[i] = max(nohold[i-1], hold[i-1] + price[i] - fee)；
• 持有一只股票的情况：要么是前一天就持有股票，要么是今天刚好买了一只股票，取其中最大值，即 hold[i] = max(hold[i-1], nohold[i-1] - price[i])；
• 初始化 nohold[0] = 0，hold[0] = -prices[0]。最后，nohold[-1] 就是答案（因为最后手里肯定没有股票，hold 实际上是一个辅助数组，帮助计算 nohold 的）。

Python3 实现：

class Solution:
    def maxProfit(self, prices: List[int], fee: int) -> int:
        hold = [0] * len(prices)
        nohold = [0] * len(prices)
        hold[0] = -prices[0]
        for i in range(1, len(prices)):
            nohold[i] = max(nohold[i-1], hold[i-1] + prices[i] - fee)
            hold[i] = max(hold[i-1], nohold[i-1] - prices[i])
        return nohold[-1]

方法2（数学理解）：比起 Leetcode 122 的方法 3，这里对目标多了一项 -fee，方法类似。

该问题的一般形式为： 将 A 划分为不重叠的 N 段，求这N 段 ∑ (A[j] - A[i] - fee) 的最大值；每一段都有 j > i。

该问题的解决方式为：先将目标变形，得到求 N 段 ∑ ((A[j] - fee) - A[i]) 的最大值，即把 A[j] - fee 看成一个整体。这样，就能使用 Leetcode 122 的方法 3 的类似解法。让 min_ 指向最小值 A[i]；在遍历的过程中，如果 (A[j] - fee) > min_，则累加 (A[j] - fee) - min_，同时更新 min_ 为 (A[j] - fee)；否则，更新 min_ 为 min_ 和 A[j] 中的较小者（这里不需要将 A[j] 替换成 A[j] - fee 的原因是不能卖股票，所以不用减去 fee）。

Python3 实现：

class Solution:
    def maxProfit(self, prices: List[int], fee: int) -> int:
        ans = 0
        min_ = prices[0]  # 第一个数为最小值
        for i in range(1, len(prices)):
            if prices[i] - fee > min_:  # 可以卖股票
                ans += prices[i] - fee - min_
                min_ = prices[i] - fee
            else:  # 不能卖股票，不用将 prices[j] 减去 fee
                min_ = min(min_, prices[i])
        return ans

---

问题描述：【多状态DP】309. Best Time to Buy and Sell Stock with Cooldown

解题思路：

方法1（双状态DP）：这道题和 Leetcode 714 思想相同，只不过由于增加了一个冻结期（cooldown），导致状态转移方程有所区别。

• 仍然用 nohold 表示第 i 天不持有股票的收益，hold 表示第 i 天持有一只股票的收益；
• 未持有股票的情况：要么是前一天就没有股票，要么是今天卖掉了，即nohold[i] = max(nohold[i-1], hold[i-1] + price[i])；
• 持有一只股票的情况：要么是前一天就持有股票，要么是今天刚好买了一只股票，但是注意：如果是今天刚好买了股票，则昨天必须休息（因为有一天的cooldown），那么买股票应该依赖于前天（而不是昨天）的未持有股票的状态，即 hold[i] = max(hold[i-1], nohold[i-2] - price[i])；
• 初始化 nohold[0] = 0，hold[0] = -prices[0]，hold[1] = max(hold[0], -prices[1])，。最后，nohold[-1] 就是答案（因为最后手里肯定没有股票，hold 实际上是一个辅助数组，帮助计算 nohold 的）。

Python3 实现：

class Solution:
    def maxProfit(self, prices: List[int]) -> int:
        if len(prices) == 0:
            return 0
        nohold = [0] * len(prices)
        hold = [0] * len(prices)
        hold[0] = -prices[0]
        for i in range(1, len(prices)):
            nohold[i] = max(nohold[i-1], hold[i-1] + prices[i])
            hold[i] = max(hold[i-1], nohold[i-2] - prices[i]) if i >= 2 else max(hold[i-1], -prices[i])
        return nohold[-1]

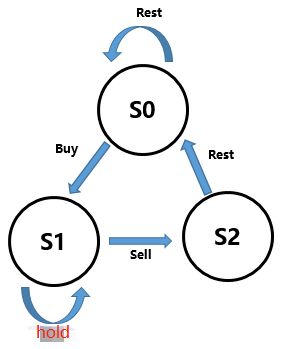
方法2（三状态DP）：方法1 划分成有股票和无股票两个状态，由于 cooldown 的存在，还可以将无股票状态再细分成两个不相交的状态（第 i 天休息和第 i 天卖出），因此我们得到以下三个状态：

• s0[i]：第 i 天休息（未持有股票），包括 cooldown；
• s1[i]：第 i 天持有股票；
• s2[i]：第 i 天卖出股票。

因此，我们可以划出状态转移图并写出状态转移方程：

• s0[i] = max(s0[i-1], s2[i-1])，前一天也休息或前一天刚卖出股票；
• s1[i] = max(s1[i-1], s0[i-1] - prices[i])，前一天也持有或前一天休息；
• s2[i] = s1[i-1] + prices，前一天持有。

注意：

• 初始化 s0[0] = 0，s1[0] = -prices[0]，s2[0] = 0；
• 最大利润应该是未持有股票的情况，因此结果应该是 max(s0[-1], s2[-1])。

Python3 实现：

class Solution:
    def maxProfit(self, prices: List[int]) -> int:
        if len(prices) == 0:
            return 0
        s0 = [0] * len(prices)
        s1 = [0] * len(prices)
        s2 = [0] * len(prices)
        s1[0] = -prices[0]
        for i in range(1, len(prices)):
            s0[i] = max(s0[i-1], s2[i-1])
            s1[i] = max(s1[i-1], s0[i-1] - prices[i])
            s2[i] = s1[i-1] + prices[i]
        return max(s0[-1], s2[-1])