2015 Multi-University Training Contest 2
1001 Angry Trees
1002 Buildings
偷看数据发现坑多。不容易考虑全。
假设m>=n,否则转置一下。
下面所描述的left,right,up,down全部和官方题解相同。
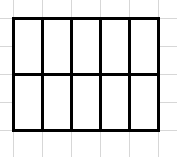
那么只要坏点左边(包含坏点)横向铺条。右边纵向铺条。一定能铺满。
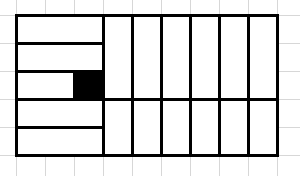
如图所示为n=5,m=9,x=3,y=3的情况。
ii)当min(left,right)>ans时。我们需要进一步讨论 。
①如果坏点在x方向上处于中间位置(n为奇数时的x=n/2+1,n为偶数时的x=n/2或x=n/2+1)那么用长度为ans纵向铺条仍然可以。
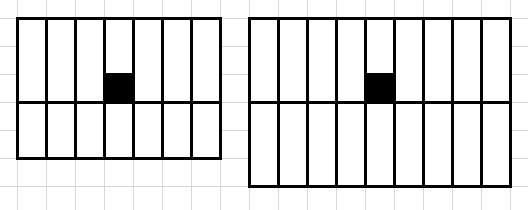
如图分别为n=5,m=7,x=3,y=4与n=6,m=9,x=3,y=5的情况。
②如果x不在中间位置。 那么长度为ans的条已经无法覆盖全图了。(无论横铺还是纵铺。)

如图分别为n=5,m=7,x=1,y=4与n=6,m=9,x=1,y=5的情况。
红色表示无法覆盖的区域。
此时我们有两种方案(不妨设ans<left<=right)
1)在坏点左边(包含坏点)用 left长度横铺,右边(不包含坏点)纵铺。(因为left>ans。这是必然可行的。)
2)用max(up,down) 长度全部纵铺。
那么在1)与2)中选择更优的即为答案。
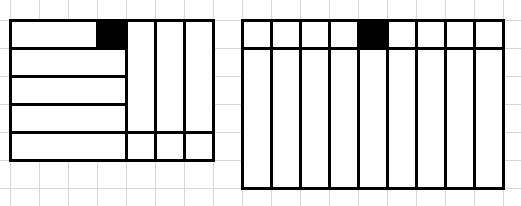
在n=5,m=7,x=1,y=4与n=6,m=9,x=1,y=5的情况下
方案1)与2)是相同的,两者均可。
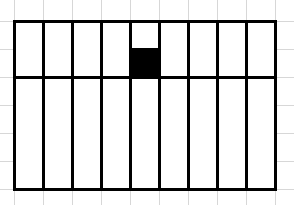
如果考虑n=6,m=9,x=2,y=5的情况。那么显然方案2)更优。
最后一个坑。边长为奇数的方形且坏点在中间。答案为ans-1。
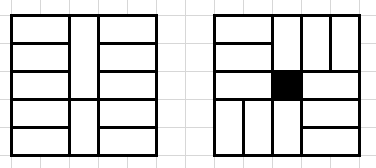
如图为n=5,m=5,x=3,y=3的情况。

1 # include <iostream> 2 # include <cstdio> 3 # include <algorithm> 4 using namespace std; 5 6 int main(void) 7 { 8 int n,m,x,y; 9 while((scanf("%d%d%d%d",&n,&m,&x,&y))!=EOF) 10 { 11 if(n>m) {swap(n,m); swap(x,y);} 12 int ans=(n+1)/2; 13 int left=y,right=m-y+1,up=x-1,down=n-x; 14 if(min(left,right)>ans&&max(up,down)-min(up,down)>1) 15 ans=min(min(left,right),max(up,down)); 16 if(n%2&&m==n&&left==right&&up==down) ans--; 17 printf("%d\n",ans); 18 } 19 return 0; 20 }
1003 Connect the Graph
1004 Delicious Apples
苹果数最多为1e5。所以把每个苹果看成一个点。
按中线将苹果分成左右两边。用dist表示每个苹果的距离。
cnt计两边苹果数。全部读好sort一遍。
先考虑贪心的取两边的苹果。
sum[i]表示在某一边取完1-i的苹果回去后走的最小路程。
递推sum数组直到sum[cnt]。
转移方程是i<=k时sum[i]=2*dist[i],i>k时sum[i]=sum[i-k]+2*dist[i]。
这样的话得到一个ans=suml[cntl]+sumr[cntr]。
然而会发现。如果贪心到最后在一边取的苹果不到k个。那么会存在走一圈更优的情况。
但是走一圈最多只有一次。因为如果多于一次就能拆成两个小于半圈的。必然小于一圈。
那么我们可以枚举走一圈的时候。分别在左半右半取了多少个。
例如左半取i个右半取k-i个。那么这i个一定要取左半距离最大的。右半同理。
这里要注意枚举时,枚举的个数不能超过左边和右边的总个数。
最后注意LL。

1 # include <iostream> 2 # include <cstdio> 3 # include <algorithm> 4 using namespace std; 5 typedef long long LL; 6 # define maxn 100010 7 int distl[maxn],distr[maxn]; 8 LL suml[maxn]={0},sumr[maxn]={0}; 9 10 int main(void) 11 { 12 int T; cin>>T; 13 while(T--) 14 { 15 LL L; int n,k; 16 scanf("%I64d%d%d",&L,&n,&k); 17 int cntl=0,cntr=0; 18 for(int i=1;i<=n;i++) 19 { 20 int x,a; scanf("%d%d",&x,&a); 21 for(int j=0;j<a;j++) 22 { 23 if(2*x<L) distl[++cntl]=x; 24 else distr[++cntr]=L-x; 25 } 26 } 27 sort(distl+1,distl+1+cntl); 28 sort(distr+1,distr+1+cntr); 29 for(int i=1;i<=cntl;i++) 30 { 31 if(i<=k) suml[i]=2*distl[i]; 32 else suml[i]=suml[i-k]+2*distl[i]; 33 } 34 for(int i=1;i<=cntr;i++) 35 { 36 if(i<=k) sumr[i]=2*distr[i]; 37 else sumr[i]=sumr[i-k]+2*distr[i]; 38 } 39 LL ans=suml[cntl]+sumr[cntr]; 40 for(int i=0;i<=k;i++) 41 { 42 int posl=max(0,cntl-(k-i)); 43 int posr=max(0,cntr-i); 44 ans=min(ans,L+suml[posl]+sumr[posr]); 45 } 46 printf("%I64d\n",ans); 47 } 48 return 0; 49 }
1005 Eastest Magical Day Seep Group's Summer
1006 Friends
连个暴搜都写不出来。不开心。
官方题解说搜点。加上两点优化:1.只考虑偶数边 2.最后一条边由前面的边确定。
在网上找到了简单易懂的做法。跑的不是很快是超级快。
先读边。每个点记下度数。如果有奇数的就直接输0过了。
所有点的度都是偶数的情况下。每个节点的on边与off边是相等的,即为度数一半。
直接搜边。枚举每条边分别是on边与off边的情况。
如果过程中有点的on边或者off边举完了。边还没搜完。
那么该情况就是不可行的。直接return。

1 # include <iostream> 2 # include <cstdio> 3 # include <cstring> 4 using namespace std; 5 # define CLR(x) memset(x,0,sizeof(x)) 6 int ans,m,n,deg[10],on[10],off[10]; 7 8 struct E 9 { 10 int from,to; 11 } edge[30]; 12 13 void dfs(int x) 14 { 15 if(x>m) {ans++; return;} 16 int u=edge[x].from,v=edge[x].to; 17 if(on[u]&&on[v]) 18 { 19 on[u]--; on[v]--; 20 dfs(x+1); 21 on[u]++; on[v]++; 22 } 23 if(off[u]&&off[v]) 24 { 25 off[u]--; off[v]--; 26 dfs(x+1); 27 off[u]++; off[v]++; 28 } 29 return; 30 } 31 32 int main(void) 33 { 34 int T; cin>>T; 35 while(T--) 36 { 37 CLR(edge); CLR(deg); CLR(on); CLR(off); 38 scanf("%d%d",&n,&m); 39 for(int i=1;i<=m;i++) 40 { 41 int u,v; scanf("%d%d",&u,&v); 42 edge[i].from=u; edge[i].to=v; 43 deg[u]++; deg[v]++; 44 } 45 int ok=1; 46 for(int i=1;i<=n;i++) if(deg[i]%2) {ok=0; break;} 47 if(!ok) {printf("0\n"); continue;} 48 for(int i=1;i<=n;i++) on[i]=off[i]=deg[i]/2; 49 ans=0; dfs(1); 50 printf("%d\n",ans); 51 } 52 return 0; 53 }
1007 Gorgeous Sequence
1008 He is Flying
1009 I Wanna Become A 24-Point Master
没什么技术含量。看官方题解。
只要能推出所有的情况就好。
然而把式子全部变成代码也挺不容易的。
一处WA整个都要重新检查一次。过的时候真的感动哭。
【第一次遇见说代码太长的情况。也有可能是HDU或者我网炸。】

1 # include <iostream> 2 # include <cstdio> 3 using namespace std; 4 5 int main(void) 6 { 7 int n; 8 while((scanf("%d",&n))!=EOF) 9 { 10 if(n<4) printf("-1\n"); 11 if(n==4) printf("1 * 2\n5 + 3\n6 + 4\n"); 12 if(n==5) printf("1 / 2\n6 / 3\n4 - 7\n5 * 8\n"); 13 if(n==6) printf("1 + 2\n3 + 7\n4 + 8\n5 + 9\n10 - 6\n"); 14 if(n==7) printf("1 * 2\n3 / 4\n5 + 6\n8 - 9\n11 / 10\n12 * 7\n"); 15 if(n==8) 16 { 17 printf("1 + 2\n9 + 3\n4 - 5\n11 * 6\n"); 18 printf("12 * 7\n13 * 8\n14 + 10\n"); 19 } 20 if(n==9) 21 { 22 printf("1 + 2\n10 + 3\n4 / 5\n6 / 7\n"); 23 printf("8 / 9\n11 - 12\n15 - 13\n16 - 14\n"); 24 } 25 if(n==10) 26 { 27 printf("1 / 2\n3 / 4\n5 / 6\n7 / 8\n9 + 10\n"); 28 printf("11 + 12\n16 + 13\n17 + 14\n18 + 15\n"); 29 } 30 if(n==11) 31 { 32 printf("1 + 2\n3 / 4\n5 / 6\n12 + 13\n15 + 14\n"); 33 printf("7 - 8\n17 * 9\n18 * 10\n19 * 11\n20 + 16\n"); 34 } 35 if(n==12) 36 { 37 printf("1 - 2\n"); 38 for(int i=0;i<8;i++) printf("%d * %d\n",i+3,i+13); 39 printf("11 + 12\n21 + 22\n"); 40 } 41 if(n==13) 42 { 43 printf("1 / 2\n3 - 14\n4 + 5\n16 / 6\n15 * 17\n7 - 8\n"); 44 for(int i=0;i<5;i++) printf("%d * %d\n",i+9,i+19); 45 printf("24 + 18\n"); 46 } 47 if(n==14) 48 { 49 printf("1 / 2\n3 / 4\n5 - 15\n17 - 16\n6 + 7\n19 / 8\n20 * 18\n9 - 10\n"); 50 for(int i=0;i<4;i++) printf("%d * %d\n",i+11,i+22); 51 printf("21 + 26\n"); 52 } 53 if(n>14) 54 { 55 printf("1 + 2\n3 + 4\n5 + 6\n7 + 8\n%d + 9\n",n+1); 56 for(int i=0;i<4;i++) printf("%d / %d\n",n+2+i,i+10); 57 printf("14 - 15\n"); 58 for(int i=0;i<n-15;i++) printf("%d * %d\n",i+16,n+10+i); 59 printf("%d + %d\n%d * %d\n%d * %d\n%d * %d\n",2*n-5,n+6,2*n-4,n+7,2*n-3,n+8,2*n-2,n+9); 60 } 61 } 62 return 0; 63 }
1010 JRY is Fighting
