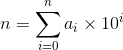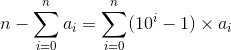- 机器学习与深度学习间关系与区别
ℒℴѵℯ心·动ꦿ໊ོ꫞
人工智能学习深度学习python
一、机器学习概述定义机器学习(MachineLearning,ML)是一种通过数据驱动的方法,利用统计学和计算算法来训练模型,使计算机能够从数据中学习并自动进行预测或决策。机器学习通过分析大量数据样本,识别其中的模式和规律,从而对新的数据进行判断。其核心在于通过训练过程,让模型不断优化和提升其预测准确性。主要类型1.监督学习(SupervisedLearning)监督学习是指在训练数据集中包含输入
- c++ 的iostream 和 c++的stdio的区别和联系
黄卷青灯77
c++算法开发语言iostreamstdio
在C++中,iostream和C语言的stdio.h都是用于处理输入输出的库,但它们在设计、用法和功能上有许多不同。以下是两者的区别和联系:区别1.编程风格iostream(C++风格):C++标准库中的输入输出流类库,支持面向对象的输入输出操作。典型用法是cin(输入)和cout(输出),使用>操作符来处理数据。更加类型安全,支持用户自定义类型的输入输出。#includeintmain(){in
- 【一起学Rust | 设计模式】习惯语法——使用借用类型作为参数、格式化拼接字符串、构造函数
广龙宇
一起学Rust#Rust设计模式rust设计模式开发语言
提示:文章写完后,目录可以自动生成,如何生成可参考右边的帮助文档文章目录前言一、使用借用类型作为参数二、格式化拼接字符串三、使用构造函数总结前言Rust不是传统的面向对象编程语言,它的所有特性,使其独一无二。因此,学习特定于Rust的设计模式是必要的。本系列文章为作者学习《Rust设计模式》的学习笔记以及自己的见解。因此,本系列文章的结构也与此书的结构相同(后续可能会调成结构),基本上分为三个部分
- 每日一题——第八十九题
互联网打工人no1
C语言程序设计每日一练c语言
题目:在字符串中找到提取数字,并统计一共找到多少整数,a123xxyu23&8889,那么找到的整数为123,23,8889//思想:#include#include#includeintmain(){charstr[]="a123xxyu23&8889";intcount=0;intnum=0;//用于临时存放当前正在构建的整数。boolinNum=false;//用于标记当前是否正在读取一个整
- 每日一题——第九十题
互联网打工人no1
C语言程序设计每日一练c语言
题目:判断子串是否与主串匹配#include#include#include//////判断子串是否在主串中匹配//////主串///子串///boolisSubstring(constchar*str,constchar*substr){intlenstr=strlen(str);//计算主串的长度intlenSub=strlen(substr);//计算子串的长度//遍历主字符串,对每个可能得
- 每日一题——第八十二题
互联网打工人no1
C语言程序设计每日一练c语言
题目:将一个控制台输入的字符串中的所有元音字母复制到另一字符串中#include#include#include#include#defineMAX_INPUT1024boolisVowel(charp);intmain(){charinput[MAX_INPUT];charoutput[MAX_INPUT];printf("请输入一串字符串:\n");fgets(input,sizeof(inp
- C#中使用split分割字符串
互联网打工人no1
c#
1、用字符串分隔:usingSystem.Text.RegularExpressions;stringstr="aaajsbbbjsccc";string[]sArray=Regex.Split(str,"js",RegexOptions.IgnoreCase);foreach(stringiinsArray)Response.Write(i.ToString()+"");输出结果:aaabbbc
- Goolge earth studio 进阶4——路径修改与平滑
陟彼高冈yu
Googleearthstudio进阶教程旅游
如果我们希望在大约中途时获得更多的城市鸟瞰视角。可以将相机拖动到这里并创建一个新的关键帧。camera_target_clip_7EarthStudio会自动平滑我们的路径,所以当我们通过这个关键帧时,不是一个生硬的角度,而是一个平滑的曲线。camera_target_clip_8路径上有贝塞尔控制手柄,允许我们调整路径的形状。右键单击,我们可以选择“平滑路径”,这是默认的自动平滑算法,或者我们可
- 基于社交网络算法优化的二维最大熵图像分割
智能算法研学社(Jack旭)
智能优化算法应用图像分割算法php开发语言
智能优化算法应用:基于社交网络优化的二维最大熵图像阈值分割-附代码文章目录智能优化算法应用:基于社交网络优化的二维最大熵图像阈值分割-附代码1.前言2.二维最大熵阈值分割原理3.基于社交网络优化的多阈值分割4.算法结果:5.参考文献:6.Matlab代码摘要:本文介绍基于最大熵的图像分割,并且应用社交网络算法进行阈值寻优。1.前言阅读此文章前,请阅读《图像分割:直方图区域划分及信息统计介绍》htt
- python是什么意思中文-在python中%是什么意思
编程大乐趣
Python中%有两种:1、数值运算:%代表取模,返回除法的余数。如:>>>7%212、%操作符(字符串格式化,stringformatting),说明如下:%[(name)][flags][width].[precision]typecode(name)为命名flags可以有+,-,''或0。+表示右对齐。-表示左对齐。''为一个空格,表示在正数的左侧填充一个空格,从而与负数对齐。0表示使用0填
- 121. 买卖股票的最佳时机
薄荷糖的味道_fb40
给定一个数组,它的第i个元素是一支给定股票第i天的价格。如果你最多只允许完成一笔交易(即买入和卖出一支股票),设计一个算法来计算你所能获取的最大利润。注意你不能在买入股票前卖出股票。示例1:输入:[7,1,5,3,6,4]输出:5解释:在第2天(股票价格=1)的时候买入,在第5天(股票价格=6)的时候卖出,最大利润=6-1=5。注意利润不能是7-1=6,因为卖出价格需要大于买入价格。示例2:输入:
- 【JS】执行时长(100分) |思路参考+代码解析(C++)
l939035548
JS算法数据结构c++
题目为了充分发挥GPU算力,需要尽可能多的将任务交给GPU执行,现在有一个任务数组,数组元素表示在这1秒内新增的任务个数且每秒都有新增任务。假设GPU最多一次执行n个任务,一次执行耗时1秒,在保证GPU不空闲情况下,最少需要多长时间执行完成。题目输入第一个参数为GPU一次最多执行的任务个数,取值范围[1,10000]第二个参数为任务数组长度,取值范围[1,10000]第三个参数为任务数组,数字范围
- 每日算法&面试题,大厂特训二十八天——第二十天(树)
肥学
⚡算法题⚡面试题每日精进java算法数据结构
目录标题导读算法特训二十八天面试题点击直接资料领取导读肥友们为了更好的去帮助新同学适应算法和面试题,最近我们开始进行专项突击一步一步来。上一期我们完成了动态规划二十一天现在我们进行下一项对各类算法进行二十八天的一个小总结。还在等什么快来一起肥学进行二十八天挑战吧!!特别介绍小白练手专栏,适合刚入手的新人欢迎订阅编程小白进阶python有趣练手项目里面包括了像《机器人尬聊》《恶搞程序》这样的有趣文章
- 回溯算法-重新安排行程
chirou_
算法数据结构图论c++图搜索
leetcode332.重新安排行程这题我还没自己ac过,只能现在凭着刚学完的热乎劲把我对题解的理解记下来。本题我认为对数据结构的考察比较多,用什么数据结构去存数据,去读取数据,都是很重要的。classSolution{private:unordered_map>targets;boolbacktracking(intticketNum,vector&result){//1.确定参数和返回值//2
- Redis系列:Geo 类型赋能亿级地图位置计算
Ly768768
redisbootstrap数据库
1前言我们在篇深刻理解高性能Redis的本质的时候就介绍过Redis的几种基本数据结构,它是基于不同业务场景而设计的:动态字符串(REDIS_STRING):整数(REDIS_ENCODING_INT)、字符串(REDIS_ENCODING_RAW)双端列表(REDIS_ENCODING_LINKEDLIST)压缩列表(REDIS_ENCODING_ZIPLIST)跳跃表(REDIS_ENCODI
- 基于CODESYS的多轴运动控制程序框架:逻辑与运动控制分离,快速开发灵活操作
GPJnCrbBdl
python开发语言
基于codesys开发的多轴运动控制程序框架,将逻辑与运动控制分离,将单轴控制封装成功能块,对该功能块的操作包含了所有的单轴控制(归零、点动、相对定位、绝对定位、设置当前位置、伺服模式切换等等)。程序框架由主程序按照状态调用分归零模式、手动模式、自动模式、故障模式,程序状态的跳转都已完成,只需要根据不同的工艺要求完成所需的动作即可。变量的声明、地址的规划都严格按照C++的标准定义,能帮助开发者快速
- C++ | Leetcode C++题解之第409题最长回文串
Ddddddd_158
经验分享C++Leetcode题解
题目:题解:classSolution{public:intlongestPalindrome(strings){unordered_mapcount;intans=0;for(charc:s)++count[c];for(autop:count){intv=p.second;ans+=v/2*2;if(v%2==1andans%2==0)++ans;}returnans;}};
- C++菜鸟教程 - 从入门到精通 第二节
DreamByte
c++
一.上节课的补充(数据类型)1.前言继上节课,我们主要讲解了输入,输出和运算符,我们现在来补充一下数据类型的知识上节课遗漏了这个知识点,非常的抱歉顺便说一下,博主要上高中了,更新会慢,2-4周更新一次对了,正好赶上中秋节,小编跟大家说一句:中秋节快乐!2.int类型上节课,我们其实只用了int类型int类型,是整数类型,它们存贮的是整数,不能存小数(浮点数)定义变量的方式很简单inta;//定义一
- Faiss:高效相似性搜索与聚类的利器
网络·魚
大数据faiss
Faiss是一个针对大规模向量集合的相似性搜索库,由FacebookAIResearch开发。它提供了一系列高效的算法和数据结构,用于加速向量之间的相似性搜索,特别是在大规模数据集上。本文将介绍Faiss的原理、核心功能以及如何在实际项目中使用它。Faiss原理:近似最近邻搜索:Faiss的核心功能之一是近似最近邻搜索,它能够高效地在大规模数据集中找到与给定查询向量最相似的向量。这种搜索是近似的,
- insert into select 主键自增_mybatis拦截器实现主键自动生成
weixin_39521651
insertintoselect主键自增mybatisdelete返回值mybatisinsert返回主键mybatisinsert返回对象mybatisplusinsert返回主键mybatisplus插入生成id
前言前阵子和朋友聊天,他说他们项目有个需求,要实现主键自动生成,不想每次新增的时候,都手动设置主键。于是我就问他,那你们数据库表设置主键自动递增不就得了。他的回答是他们项目目前的id都是采用雪花算法来生成,因此为了项目稳定性,不会切换id的生成方式。朋友问我有没有什么实现思路,他们公司的orm框架是mybatis,我就建议他说,不然让你老大把mybatis切换成mybatis-plus。mybat
- k均值聚类算法考试例题_k均值算法(k均值聚类算法计算题)
寻找你83497
k均值聚类算法考试例题
?算法:第一步:选K个初始聚类中心,z1(1),z2(1),…,zK(1),其中括号内的序号为寻找聚类中心的迭代运算的次序号。聚类中心的向量值可任意设定,例如可选开始的K个.k均值聚类:---------一种硬聚类算法,隶属度只有两个取值0或1,提出的基本根据是“类内误差平方和最小化”准则;模糊的c均值聚类算法:--------一种模糊聚类算法,是.K均值聚类算法是先随机选取K个对象作为初始的聚类
- Python实现简单的机器学习算法
master_chenchengg
pythonpython办公效率python开发IT
Python实现简单的机器学习算法开篇:初探机器学习的奇妙之旅搭建环境:一切从安装开始必备工具箱第一步:安装Anaconda和JupyterNotebook小贴士:如何配置Python环境变量算法初体验:从零开始的Python机器学习线性回归:让数据说话数据准备:从哪里找数据编码实战:Python实现线性回归模型评估:如何判断模型好坏逻辑回归:从分类开始理论入门:什么是逻辑回归代码实现:使用skl
- C语言 判断回文数
Y雨何时停T
c语言学习
一,回文数概念“回文”是指正读反读都能读通的句子,它是古今中外都有的一种修辞方式和文字游戏,如“我为人人,人人为我”等。在数学中也有这样一类数字有这样的特征,成为回文数。设n是一任意自然数。若将n的各位数字反向排列所得自然数n1与n相等,则称n为一回文数。例如,若n=1234321,则称n为一回文数;但若n=1234567,则n不是回文数。二,判断回文数实现思路一:数组与字符串将数字每一位按顺序放
- Linux sh命令
fengyehongWorld
Linuxlinux
目录一.基本语法二.选项2.1-c字符串中读取内容,并执行2.1.1基本用法2.1.2获取当前目录下失效的超链接2.2-x每个命令执行之前,将其打印出来2.3结合Here文档使用一.基本语法⏹Linux和Unix系统中用于执行shell脚本或运行命令的命令。sh[选项][脚本文件][参数...]⏹选项-c:从字符串中读取内容,并执行。-x:在每个命令执行之前,将其打印出来。-s:从标准流中读取内容
- 推荐算法_隐语义-梯度下降
_feivirus_
算法机器学习和数学推荐算法机器学习隐语义
importnumpyasnp1.模型实现"""inputrate_matrix:M行N列的评分矩阵,值为P*Q.P:初始化用户特征矩阵M*K.Q:初始化物品特征矩阵K*N.latent_feature_cnt:隐特征的向量个数max_iteration:最大迭代次数alpha:步长lamda:正则化系数output分解之后的P和Q"""defLFM_grad_desc(rate_matrix,l
- K近邻算法_分类鸢尾花数据集
_feivirus_
算法机器学习和数学分类机器学习K近邻
importnumpyasnpimportpandasaspdfromsklearn.datasetsimportload_irisfromsklearn.model_selectionimporttrain_test_splitfromsklearn.metricsimportaccuracy_score1.数据预处理iris=load_iris()df=pd.DataFrame(data=ir
- Java面试题精选:消息队列(二)
芒果不是芒
Java面试题精选javakafka
一、Kafka的特性1.消息持久化:消息存储在磁盘,所以消息不会丢失2.高吞吐量:可以轻松实现单机百万级别的并发3.扩展性:扩展性强,还是动态扩展4.多客户端支持:支持多种语言(Java、C、C++、GO、)5.KafkaStreams(一个天生的流处理):在双十一或者销售大屏就会用到这种流处理。使用KafkaStreams可以快速的把销售额统计出来6.安全机制:Kafka进行生产或者消费的时候会
- 数据结构 | 栈和队列
TT-Kun
数据结构与算法数据结构栈队列C语言
文章目录栈和队列1.栈:后进先出(LIFO)的数据结构1.1概念与结构1.2栈的实现2.队列:先进先出(FIFO)的数据结构2.1概念与结构2.2队列的实现3.栈和队列算法题3.1有效的括号3.2用队列实现栈3.3用栈实现队列3.4设计循环队列结论栈和队列在计算机科学中,栈和队列是两种基本且重要的数据结构,它们在处理数据存储和访问顺序方面有着独特的规则和应用。本文将详细介绍栈和队列的概念、结构、实
- 2024.9.6 Python,华为笔试题总结,字符串格式化,字符串操作,广度优先搜索解决公司组织绩效互评问题,无向图
RaidenQ
python华为leetcode算法力扣广度优先无向图
1.字符串格式化name="Alice"age=30formatted_string="Name:{},Age:{}".format(name,age)print(formatted_string)或者name="Alice"age=30formatted_string=f"Name:{name},Age:{age}"print(formatted_string)2.网络健康检查第一行有两个整数m
- 2024.9.14 Python,差分法解决区间加法,消除游戏,压缩字符串
RaidenQ
python游戏开发语言算法力扣
1.区间加法假设你有一个长度为n的数组,初始情况下所有的数字均为0,你将会被给出k个更新的操作。其中,每个操作会被表示为一个三元组:[startIndex,endIndex,inc],你需要将子数组A[startIndex…endIndex](包括startIndex和endIndex)增加inc。请你返回k次操作后的数组。示例:输入:length=5,updates=[[1,3,2],[2,4,
- Nginx负载均衡
510888780
nginx应用服务器
Nginx负载均衡一些基础知识:
nginx 的 upstream目前支持 4 种方式的分配
1)、轮询(默认)
每个请求按时间顺序逐一分配到不同的后端服务器,如果后端服务器down掉,能自动剔除。
2)、weight
指定轮询几率,weight和访问比率成正比
- RedHat 6.4 安装 rabbitmq
bylijinnan
erlangrabbitmqredhat
在 linux 下安装软件就是折腾,首先是测试机不能上外网要找运维开通,开通后发现测试机的 yum 不能使用于是又要配置 yum 源,最后安装 rabbitmq 时也尝试了两种方法最后才安装成功
机器版本:
[root@redhat1 rabbitmq]# lsb_release
LSB Version: :base-4.0-amd64:base-4.0-noarch:core
- FilenameUtils工具类
eksliang
FilenameUtilscommon-io
转载请出自出处:http://eksliang.iteye.com/blog/2217081 一、概述
这是一个Java操作文件的常用库,是Apache对java的IO包的封装,这里面有两个非常核心的类FilenameUtils跟FileUtils,其中FilenameUtils是对文件名操作的封装;FileUtils是文件封装,开发中对文件的操作,几乎都可以在这个框架里面找到。 非常的好用。
- xml文件解析SAX
不懂事的小屁孩
xml
xml文件解析:xml文件解析有四种方式,
1.DOM生成和解析XML文档(SAX是基于事件流的解析)
2.SAX生成和解析XML文档(基于XML文档树结构的解析)
3.DOM4J生成和解析XML文档
4.JDOM生成和解析XML
本文章用第一种方法进行解析,使用android常用的DefaultHandler
import org.xml.sax.Attributes;
- 通过定时任务执行mysql的定期删除和新建分区,此处是按日分区
酷的飞上天空
mysql
使用python脚本作为命令脚本,linux的定时任务来每天定时执行
#!/usr/bin/python
# -*- coding: utf8 -*-
import pymysql
import datetime
import calendar
#要分区的表
table_name = 'my_table'
#连接数据库的信息
host,user,passwd,db =
- 如何搭建数据湖架构?听听专家的意见
蓝儿唯美
架构
Edo Interactive在几年前遇到一个大问题:公司使用交易数据来帮助零售商和餐馆进行个性化促销,但其数据仓库没有足够时间去处理所有的信用卡和借记卡交易数据
“我们要花费27小时来处理每日的数据量,”Edo主管基础设施和信息系统的高级副总裁Tim Garnto说道:“所以在2013年,我们放弃了现有的基于PostgreSQL的关系型数据库系统,使用了Hadoop集群作为公司的数
- spring学习——控制反转与依赖注入
a-john
spring
控制反转(Inversion of Control,英文缩写为IoC)是一个重要的面向对象编程的法则来削减计算机程序的耦合问题,也是轻量级的Spring框架的核心。 控制反转一般分为两种类型,依赖注入(Dependency Injection,简称DI)和依赖查找(Dependency Lookup)。依赖注入应用比较广泛。
- 用spool+unixshell生成文本文件的方法
aijuans
xshell
例如我们把scott.dept表生成文本文件的语句写成dept.sql,内容如下:
set pages 50000;
set lines 200;
set trims on;
set heading off;
spool /oracle_backup/log/test/dept.lst;
select deptno||','||dname||','||loc
- 1、基础--名词解析(OOA/OOD/OOP)
asia007
学习基础知识
OOA:Object-Oriented Analysis(面向对象分析方法)
是在一个系统的开发过程中进行了系统业务调查以后,按照面向对象的思想来分析问题。OOA与结构化分析有较大的区别。OOA所强调的是在系统调查资料的基础上,针对OO方法所需要的素材进行的归类分析和整理,而不是对管理业务现状和方法的分析。
OOA(面向对象的分析)模型由5个层次(主题层、对象类层、结构层、属性层和服务层)
- 浅谈java转成json编码格式技术
百合不是茶
json编码java转成json编码
json编码;是一个轻量级的数据存储和传输的语言
在java中需要引入json相关的包,引包方式在工程的lib下就可以了
JSON与JAVA数据的转换(JSON 即 JavaScript Object Natation,它是一种轻量级的数据交换格式,非
常适合于服务器与 JavaScript 之间的数据的交
- web.xml之Spring配置(基于Spring+Struts+Ibatis)
bijian1013
javaweb.xmlSSIspring配置
指定Spring配置文件位置
<context-param>
<param-name>contextConfigLocation</param-name>
<param-value>
/WEB-INF/spring-dao-bean.xml,/WEB-INF/spring-resources.xml,
/WEB-INF/
- Installing SonarQube(Fail to download libraries from server)
sunjing
InstallSonar
1. Download and unzip the SonarQube distribution
2. Starting the Web Server
The default port is "9000" and the context path is "/". These values can be changed in &l
- 【MongoDB学习笔记十一】Mongo副本集基本的增删查
bit1129
mongodb
一、创建复本集
假设mongod,mongo已经配置在系统路径变量上,启动三个命令行窗口,分别执行如下命令:
mongod --port 27017 --dbpath data1 --replSet rs0
mongod --port 27018 --dbpath data2 --replSet rs0
mongod --port 27019 -
- Anychart图表系列二之执行Flash和HTML5渲染
白糖_
Flash
今天介绍Anychart的Flash和HTML5渲染功能
HTML5
Anychart从6.0第一个版本起,已经逐渐开始支持各种图的HTML5渲染效果了,也就是说即使你没有安装Flash插件,只要浏览器支持HTML5,也能看到Anychart的图形(不过这些是需要做一些配置的)。
这里要提醒下大家,Anychart6.0版本对HTML5的支持还不算很成熟,目前还处于
- Laravel版本更新异常4.2.8-> 4.2.9 Declaration of ... CompilerEngine ... should be compa
bozch
laravel
昨天在为了把laravel升级到最新的版本,突然之间就出现了如下错误:
ErrorException thrown with message "Declaration of Illuminate\View\Engines\CompilerEngine::handleViewException() should be compatible with Illuminate\View\Eng
- 编程之美-NIM游戏分析-石头总数为奇数时如何保证先动手者必胜
bylijinnan
编程之美
import java.util.Arrays;
import java.util.Random;
public class Nim {
/**编程之美 NIM游戏分析
问题:
有N块石头和两个玩家A和B,玩家A先将石头随机分成若干堆,然后按照BABA...的顺序不断轮流取石头,
能将剩下的石头一次取光的玩家获胜,每次取石头时,每个玩家只能从若干堆石头中任选一堆,
- lunce创建索引及简单查询
chengxuyuancsdn
查询创建索引lunce
import java.io.File;
import java.io.IOException;
import org.apache.lucene.analysis.Analyzer;
import org.apache.lucene.analysis.standard.StandardAnalyzer;
import org.apache.lucene.document.Docume
- [IT与投资]坚持独立自主的研究核心技术
comsci
it
和别人合作开发某项产品....如果互相之间的技术水平不同,那么这种合作很难进行,一般都会成为强者控制弱者的方法和手段.....
所以弱者,在遇到技术难题的时候,最好不要一开始就去寻求强者的帮助,因为在我们这颗星球上,生物都有一种控制其
- flashback transaction闪回事务查询
daizj
oraclesql闪回事务
闪回事务查询有别于闪回查询的特点有以下3个:
(1)其正常工作不但需要利用撤销数据,还需要事先启用最小补充日志。
(2)返回的结果不是以前的“旧”数据,而是能够将当前数据修改为以前的样子的撤销SQL(Undo SQL)语句。
(3)集中地在名为flashback_transaction_query表上查询,而不是在各个表上通过“as of”或“vers
- Java I/O之FilenameFilter类列举出指定路径下某个扩展名的文件
游其是你
FilenameFilter
这是一个FilenameFilter类用法的例子,实现的列举出“c:\\folder“路径下所有以“.jpg”扩展名的文件。 1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22 23 24 25 26 27 28
- C语言学习五函数,函数的前置声明以及如何在软件开发中合理的设计函数来解决实际问题
dcj3sjt126com
c
# include <stdio.h>
int f(void) //括号中的void表示该函数不能接受数据,int表示返回的类型为int类型
{
return 10; //向主调函数返回10
}
void g(void) //函数名前面的void表示该函数没有返回值
{
//return 10; //error 与第8行行首的void相矛盾
}
in
- 今天在测试环境使用yum安装,遇到一个问题: Error: Cannot retrieve metalink for repository: epel. Pl
dcj3sjt126com
centos
今天在测试环境使用yum安装,遇到一个问题:
Error: Cannot retrieve metalink for repository: epel. Please verify its path and try again
处理很简单,修改文件“/etc/yum.repos.d/epel.repo”, 将baseurl的注释取消, mirrorlist注释掉。即可。
&n
- 单例模式
shuizhaosi888
单例模式
单例模式 懒汉式
public class RunMain {
/**
* 私有构造
*/
private RunMain() {
}
/**
* 内部类,用于占位,只有
*/
private static class SingletonRunMain {
priv
- Spring Security(09)——Filter
234390216
Spring Security
Filter
目录
1.1 Filter顺序
1.2 添加Filter到FilterChain
1.3 DelegatingFilterProxy
1.4 FilterChainProxy
1.5
- 公司项目NODEJS实践0.1
逐行分析JS源代码
mongodbnginxubuntunodejs
一、前言
前端如何独立用nodeJs实现一个简单的注册、登录功能,是不是只用nodejs+sql就可以了?其实是可以实现,但离实际应用还有距离,那要怎么做才是实际可用的。
网上有很多nod
- java.lang.Math
liuhaibo_ljf
javaMathlang
System.out.println(Math.PI);
System.out.println(Math.abs(1.2));
System.out.println(Math.abs(1.2));
System.out.println(Math.abs(1));
System.out.println(Math.abs(111111111));
System.out.println(Mat
- linux下时间同步
nonobaba
ntp
今天在linux下做hbase集群的时候,发现hmaster启动成功了,但是用hbase命令进入shell的时候报了一个错误 PleaseHoldException: Master is initializing,查看了日志,大致意思是说master和slave时间不同步,没办法,只好找一种手动同步一下,后来发现一共部署了10来台机器,手动同步偏差又比较大,所以还是从网上找现成的解决方
- ZooKeeper3.4.6的集群部署
roadrunners
zookeeper集群部署
ZooKeeper是Apache的一个开源项目,在分布式服务中应用比较广泛。它主要用来解决分布式应用中经常遇到的一些数据管理问题,如:统一命名服务、状态同步、集群管理、配置文件管理、同步锁、队列等。这里主要讲集群中ZooKeeper的部署。
1、准备工作
我们准备3台机器做ZooKeeper集群,分别在3台机器上创建ZooKeeper需要的目录。
数据存储目录
- Java高效读取大文件
tomcat_oracle
java
读取文件行的标准方式是在内存中读取,Guava 和Apache Commons IO都提供了如下所示快速读取文件行的方法: Files.readLines(new File(path), Charsets.UTF_8); FileUtils.readLines(new File(path)); 这种方法带来的问题是文件的所有行都被存放在内存中,当文件足够大时很快就会导致
- 微信支付api返回的xml转换为Map的方法
xu3508620
xmlmap微信api
举例如下:
<xml>
<return_code><![CDATA[SUCCESS]]></return_code>
<return_msg><![CDATA[OK]]></return_msg>
<appid><