傅里叶变换及其应用笔记(part 1)
斯坦福EE261
目录
- 预备内容:
- 周期性:三角函数表示复杂函数
- 将一般周期函数表为简单周期函数和
- 应用(热方程)
- 傅里叶变换(周期现象到非周期现象)
- 傅里叶变换符号
- 高斯函数
- 对偶性(傅里叶变换的对偶性质)
- 三个重要问题:时延(delays),时域尺度变换,卷积
- 卷积运算
- 怎样利用卷积求热方程??
- 卷积核中心极限定理(conbolution and the Central Limit Theorem,CLT)
- 讨论积分收敛问题
- 傅里叶变换扩展(重新定义傅里叶变换,广义傅里叶变换)
- 分布的导数和卷积
- 傅里叶变换与衍射(diffraction)
- 晶体成像
- 采样于差值 sampling and interpolation
- 音乐上的采样,离散变换
- 离散傅里叶变换及其性质
- FFT(Fast Fourier Transform)的主要思想
预备内容:
由Fourier级数过渡到Fourier分析(源于周期性,空间周期性和时间周期性)
傅里叶变换作为Fourier级数的极限情况,用来分析非周期现象,有些概念两者通用,有些不同
分析:分解一个函数(信号)为一些简单的部分
合成:吧基本部分重组成信号本身
分析和合成是由线性运算完成的,就是积分和序列
Fourier分析是线性系统的一部分
和群论有关的研究对称性
周期性:三角函数表示复杂函数
并非所有现象都有周期性,即使为周期现象,最终也会消失,然而cos,sin是无限的,但在一段时间内,即使没有周期性也可以用周期延拓使其成为周期现象
用来表示复杂信号(函数),使用cos和sin(通过改变相位和频率相加)周期为1
∑ k = 1 N A k sin ( s π k t + φ k ) \sum_{k=1}^N A_k \sin(s\pi k t + \varphi_k) k=1∑NAksin(sπkt+φk)
可以看成音频的叠加(音乐音符的组合)可以产生声音
上式的另一个形式为
∑ k = 1 N a k sin 2 π k t + b k cos 2 π k t \sum_{k=1}^N a_k \sin 2\pi kt +b_k \cos 2\pi kt k=1∑Naksin2πkt+bkcos2πkt
再加一个常数
a 0 2 + ∑ k = 1 N a k sin 2 π k t + b k cos 2 π k t \frac{a_0}{2}+\sum_{k=1}^N a_k \sin 2\pi kt +b_k \cos 2\pi kt 2a0+k=1∑Naksin2πkt+bkcos2πkt
由 e 2 π i k t = cos 2 π k t + i sin 2 π k t e^{2\pi i kt} = \cos 2\pi kt + i \sin 2\pi kt e2πikt=cos2πkt+isin2πkt得
cos 2 π k t = e 2 π i k t + e − 2 π i k t 2 sin 2 π k t = e 2 π i k t − e − 2 π i k t 2 i \cos 2\pi kt = \frac{e^{2\pi i kt} + e^{-2\pi i kt}}{2}\\ \sin 2\pi kt = \frac{e^{2\pi i kt} - e^{-2\pi i kt}}{2i} cos2πkt=2e2πikt+e−2πiktsin2πkt=2ie2πikt−e−2πikt
得,和式可表示为( c k c_k ck为复数,满足对称性 c − k = c k ‾ c_{-k} = \overline{c_k} c−k=ck,因为结果为实数)
∑ k = − N N c k e 2 π i k t \sum_{k = -N}^Nc_k e^{2\pi i kt} k=−N∑Ncke2πikt
对于一个周期性为1的函数 f ( t ) f(t) f(t),它能表示成
f ( t ) = ∑ k = − n n c k e 2 π i k t f(t) = \sum_{k = -n}^nc_k e^{2\pi i kt} f(t)=k=−n∑ncke2πikt
先不管为什么,我们假设等式成立,那么怎么求 c k c_k ck,分离系数
c m e 2 π i m t = f ( t ) − ∑ k ≠ m e 2 π i k t c_m e^{2\pi i m t} = f(t) - \sum_{k \,\ne m} e^{2\pi i kt} cme2πimt=f(t)−k̸=m∑e2πikt
两边乘 e − 2 π i m t e^{-2\pi i m t} e−2πimt,的
c m = f ( t ) e − 2 π i m t − ∑ k ≠ m e 2 π i k t e − 2 π i m t c_m = f(t)e^{-2\pi i m t} - \sum_{k \,\ne m} e^{2\pi i kt} e^{-2\pi i m t} cm=f(t)e−2πimt−k̸=m∑e2πikte−2πimt
做积分(其实就是内积)
c m = ∫ 0 1 c m d t = ∫ 0 1 f ( t ) e − 2 π i m t d t + 0 c_m = \int_0^1 c_m dt =\int_0^1 f(t)e^{-2\pi i m t} dt + 0 cm=∫01cmdt=∫01f(t)e−2πimtdt+0
将一般周期函数表为简单周期函数和
考虑前面剩下的问题:为什么能这么表示,什么时候能表示
记 f ^ ( k ) = ∫ 0 1 f ( t ) e − 2 π i k t d t \hat{f}(k)=\int_0^1 f(t)e^{-2\pi i k t} dt f^(k)=∫01f(t)e−2πiktdt表示 f ( t ) f(t) f(t)的第 k k k个系数
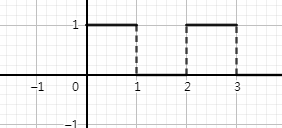
上面阶梯函数是不能表示成有限和的,对于
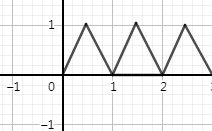
就算上面这样的连续函数也是不行的,因为无限可微的函数的有限组合也是无限可微的,所以表示成有限和的必要条件是无限可微,对于不是很光滑的地方,我们就需要更高频的成分去弥补它
所以为了表示更一般的周期现象,必须考虑无限和
∑ − ∞ ∞ f ( t ) e 2 π i k t \sum_{-\infty}^{\infty}f(t) e^{2\pi i kt} −∞∑∞f(t)e2πikt
还要确定它是否收敛,需要一些消去技巧(见傅里叶分析导论)
连续的情况它收敛于 f ( t ) f(t) f(t),为逐点收敛
如果 f ( t ) f(t) f(t)可微,那么级数一致收敛到 f ( t ) f(t) f(t)
对于不连续的情况,如果 t 0 t_0 t0为跳跃不连续点,那么它收敛于 1 2 ( f ( t 0 + ) + f ( t 0 − ) ) \frac{1}{2}(f(t_0^+)+f(t_0^-)) 21(f(t0+)+f(t0−))
当满足一些情况的时候,级数均方收敛
只要有一点不光滑,就会产生无穷的傅里叶级数
补充,内积定义:见分析学,可以定义内积为如下
< f , g > = ∫ 0 1 f ( t ) g ( t ) ‾ d t \left<f,g\right>=\int_0^1 f(t) \overline{g(t)}dt ⟨f,g⟩=∫01f(t)g(t)dt
范数为 < f , f > = ∥ f ∥ 2 = ∫ 0 1 f ( t ) f ( t ) ‾ d t = ∫ 0 1 ∣ f ( t ) ∣ 2 d t \left<f,f \right>=\|f\|^2 =\int_0^1 f(t) \overline{f(t)}dt =\int_0^1 |f(t)|^2dt ⟨f,f⟩=∥f∥2=∫01f(t)f(t)dt=∫01∣f(t)∣2dt
可以证明 e 2 π i n t , n ∈ Z e^{2\pi i nt},n \in \mathbb{Z} e2πint,n∈Z是 L 2 ( 0 , 1 ) L^2(0,1) L2(0,1)的正交基,并且是完备的
傅里叶级数可以看成分到正交基上分量的和
瑞利等式(Rayleigh equality):
∫ 0 1 ∣ f ∣ 2 d t = ∑ − ∞ ∞ ∣ f ^ ( k ) ∣ 2 \int_0^1 |f|^2 dt = \sum_{-\infty}^\infty |\hat{f}(k)|^2 ∫01∣f∣2dt=−∞∑∞∣f^(k)∣2
可以看成能量部分和为总和
应用(热方程)
heat flow(热流)物理导致傅里叶分析的快速发展
有一个空间区域,有初始温度分布 f ( x ) f(x) f(x),温度如何随时间和位置变化
关注一个热环(周长为1), u ( x , t ) u(x,t) u(x,t)为我们所要找的
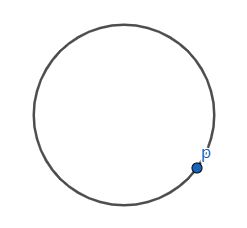
温度为空间的周期函数, u ( x + 1 , t ) = u ( x , t ) u(x+1,t) = u(x,t) u(x+1,t)=u(x,t),对 x x x进行展开
u ( x , t ) = ∑ − ∞ ∞ c k e 2 π i k x = ∑ − ∞ ∞ c k ( t ) e 2 π i k x u(x,t) = \sum_{-\infty}^\infty c_k e^{2\pi i kx} = \sum_{-\infty}^\infty c_k(t) e^{2\pi i kx} u(x,t)=−∞∑∞cke2πikx=−∞∑∞ck(t)e2πikx
Heat equation (热方程,下标表示偏导,这是偏微分方程):
温度随时间变化跟周围温度差大小成正比,原理见另一篇博客
多变量微积分中散度定理关于扩散方程的推导
一维: u t = a u x x u_t = au_{xx} ut=auxx( a a a和物理特性有关),为了方便这里令 a = 1 2 a=\frac{1}{2} a=21
u t = 1 2 u x x u_t = \frac{1}{2}u_{xx} ut=21uxx
u t = ∑ − ∞ ∞ c k ′ ( t ) e 2 π i k x u_t=\sum_{-\infty}^\infty c_k'(t) e^{2\pi i kx} ut=−∞∑∞ck′(t)e2πikx
u x x = ∑ − ∞ ∞ c k ( t ) ( 2 π i k ) 2 e 2 π i k x u_{xx}=\sum_{-\infty}^\infty c_k(t) (2\pi i k)^2e^{2\pi i kx} uxx=−∞∑∞ck(t)(2πik)2e2πikx
对应项相等,得到 c k ′ ( t ) = − 1 2 c k ( t ) ( 4 π 2 k 2 ) c_k'(t)=-\frac{1}{2}c_k(t)(4\pi^2k^2) ck′(t)=−21ck(t)(4π2k2)
即
c k ′ ( t ) = − 2 π 2 k 2 c k ( t ) c_k'(t) = -2\pi^2 k^2 c_k(t) ck′(t)=−2π2k2ck(t)
这是一个ODE解为
c k ( t ) = c k ( 0 ) e − 2 π 2 k 2 t c_k(t) = c_k(0)e^{-2\pi^2k^2t} ck(t)=ck(0)e−2π2k2t
由 u ( x , t ) = ∑ − ∞ ∞ c k ( t ) e 2 π i k x u(x,t) = \sum_{-\infty}^\infty c_k(t) e^{2\pi i kx} u(x,t)=−∞∑∞ck(t)e2πikx
令 t = 0 t=0 t=0,
u ( x , 0 ) = ∑ − ∞ ∞ c k ( 0 ) e 2 π i k x u(x,0) = \sum_{-\infty}^\infty c_k(0) e^{2\pi i kx} u(x,0)=−∞∑∞ck(0)e2πikx
也就是
f ( x ) = u ( x , 0 ) = ∑ k = − ∞ ∞ c k ( 0 ) e 2 π i k x f(x) = u(x,0) = \sum_{k=-\infty}^\infty c_k(0) e^{2\pi i kx} f(x)=u(x,0)=k=−∞∑∞ck(0)e2πikx
故 c k ( 0 ) = ∫ f ( x ) e − 2 π i k x d x = f ^ ( k ) c_k(0)=\int f(x) e^{-2\pi i k x}dx = \hat{f}(k) ck(0)=∫f(x)e−2πikxdx=f^(k)
代入上面的,得到
u ( x , t ) = ∑ k = − ∞ ∞ f ^ ( k ) e − 2 π 2 k 2 t e 2 π i k x u(x,t) = \sum_{k=-\infty}^\infty \hat{f}(k) e^{-2\pi^2 k^2 t }e^{2\pi ikx} u(x,t)=k=−∞∑∞f^(k)e−2π2k2te2πikx
以另一种方式写
f ^ ( k ) = ∫ 0 1 e − 2 π i k y f ( y ) d y \hat{f}(k) = \int_0^1 e^{-2\pi i ky}f(y)dy f^(k)=∫01e−2πikyf(y)dy
u ( x , t ) = ∑ k = − ∞ ∞ c k ( t ) e 2 π i k x = ∑ k = − ∞ ∞ c k ( 0 ) e − 2 π 2 k 2 t e 2 π i k x = ∑ k = − ∞ ∞ ∫ 0 1 f ( y ) e − 2 π i k y d y e − 2 π 2 k 2 t e 2 π i k x = ∫ 0 1 ( ∑ k = − ∞ ∞ e − 2 π i k y e − 2 π 2 k 2 t e 2 π i k x ) f ( y ) d y = ∫ 0 1 ( e 2 π i k ( x − y ) e − 2 π 2 k 2 t ) f ( y ) d y \begin{aligned}u(x,t) &= \sum_{k=-\infty}^\infty c_k(t)e^{2\pi ikx}\\ &= \sum_{k=-\infty}^\infty c_k(0) e^{-2\pi^2 k^2t} e^{2\pi ikx} \\ &= \sum_{k=-\infty}^\infty \int_0^1 f(y) e^{-2\pi i k y }dy \,e^{-2\pi^2 k^2t} e^{2\pi ikx}\\ &=\int_0^1\left(\sum_{k=-\infty}^\infty e^{-2\pi i k y }e^{-2\pi^2 k^2t} e^{2\pi ikx} \right) f(y) dy\\ &=\int_0^1\left(e^{2\pi i k(x - y)}e^{-2\pi^2 k ^2 t} \right) f(y) dy\end{aligned} u(x,t)=k=−∞∑∞ck(t)e2πikx=k=−∞∑∞ck(0)e−2π2k2te2πikx=k=−∞∑∞∫01f(y)e−2πikydye−2π2k2te2πikx=∫01(k=−∞∑∞e−2πikye−2π2k2te2πikx)f(y)dy=∫01(e2πik(x−y)e−2π2k2t)f(y)dy
记
g ( x , t ) = e 2 π i k x e − 2 π 2 k 2 t g(x,t)=e^{2\pi i kx}e^{-2\pi^2 k ^2 t} g(x,t)=e2πikxe−2π2k2t
则
u ( x , t ) = ∫ 0 1 g ( x − y , t ) f ( y ) d y u(x,t) = \int_0^1 g(x - y,t) f(y) dy u(x,t)=∫01g(x−y,t)f(y)dy
这表达了 u ( x m t ) u(xmt) u(xmt)是 f ( x ) f(x) f(x)与热核函数 g ( x , t ) g(x,t) g(x,t)的卷积
g的不同叫法:热核函数也称为格林函数
(泊松核和狄利克雷问题也会类似)
傅里叶变换(周期现象到非周期现象)
将非周期函数看做周期无限函数。傅里叶 变换是一种一般(也就是另一种极限的情况,即周期无限)情况,即傅里叶级数的极限形式
对于周期T的函数(再让 T → ∞ T\rightarrow \infty T→∞)
傅里叶基为 e 2 π i k ( t / T ) e^{2\pi i k (t/T)} e2πik(t/T),傅里叶级数为
f ( t ) = ∑ c k e 2 π i ( k / T ) t c k = 1 T ∫ 0 1 e − 2 π i ( k / T ) t f ( t ) d t f(t) = \sum c_k e^{2\pi i (k / T)t}\\ c_k = \frac{1}{T} \int_0^1 e^{-2\pi i (k/T)t}f(t) dt f(t)=∑cke2πi(k/T)tck=T1∫01e−2πi(k/T)tf(t)dt
(缩放即可,然而为什么基完备这是另一个问题)
可写成
c k = 1 T ∫ − T 2 T 2 e − 2 π i ( k / T ) t f ( t ) d t c_k = \frac{1}{T} \int_{-\frac{T}{2}}^{\frac{T}{2}} e^{-2\pi i (k/T)t}f(t) dt ck=T1∫−2T2Te−2πi(k/T)tf(t)dt
频谱Picture of frequency( spectrum)
它是对称的因为 c c c对称,周期为 T T T,则间隔为 1 T \frac{1}{T} T1
当 T → ∞ T\rightarrow \infty T→∞的时候,频谱变得连续。
不能直接让 T → ∞ T\rightarrow \infty T→∞得到傅里叶变换,因为当 T → ∞ T\rightarrow \infty T→∞时,由
c k = 1 T ∫ − T 2 T 2 e − 2 π i ( k / T ) t f ( t ) d t c_k = \frac{1}{T} \int_{-\frac{T}{2}}^{\frac{T}{2}} e^{-2\pi i (k/T)t}f(t) dt ck=T1∫−2T2Te−2πi(k/T)tf(t)dt
计算的 c k c_k ck趋于0
比如 f ( t ) f(t) f(t)是一个只在[a,b]上不为0的函数,将其周期延 − T 2 -\frac{T}{2} −2T到 T 2 \frac{T}{2} 2T包含[a,b]即可)
我们计算 c k = 1 T ∫ − T 2 T 2 e − 2 π i ( k / T ) t f ( t ) d t = 1 T ∫ a b e − 2 π i ( k / T ) t f ( t ) d t \begin{aligned}c_k &= \frac{1}{T} \int_{-\frac{T}{2}}^{\frac{T}{2}} e^{-2\pi i (k/T)t}f(t) dt\\ &=\frac{1}{T} \int_{a}^{b} e^{-2\pi i (k/T)t}f(t) dt\\\end{aligned} ck=T1∫−2T2Te−2πi(k/T)tf(t)dt=T1∫abe−2πi(k/T)tf(t)dt
∣ c k ∣ = 1 T ∣ ∫ a b e − 2 π i ( k / T ) t f ( t ) d t ∣ ≤ 1 T ∫ a b ∣ e − 2 π i ( k / T ) t ∣ ∣ f ( t ) ∣ d t ≤ 1 T ∫ a b ∣ f ( t ) ∣ d t ≤ M T → 0 \begin{aligned}|c_k|&= \frac{1}{T}\left| \int_{a}^{b} e^{-2\pi i (k/T)t}f(t) dt\right|\\ &\le \frac{1}{T}\int_{a}^{b} \left|e^{-2\pi i (k/T)t}\right|\left|f(t)\right| dt\\ &\le \frac{1}{T}\int_{a}^{b} \left|f(t)\right| dt\\ &\le \frac{M}{T}\rightarrow 0 \end{aligned} ∣ck∣=T1∣∣∣∣∣∫abe−2πi(k/T)tf(t)dt∣∣∣∣∣≤T1∫ab∣∣∣e−2πi(k/T)t∣∣∣∣f(t)∣dt≤T1∫ab∣f(t)∣dt≤TM→0
这不能得到有用的结果,必须寻找其他途径
记线性算子 F f ( k T ) = ∫ − T 2 T 2 e − 2 π i ( k / T ) t f ( t ) d t \mathcal{F}f(\frac{k}{T})=\int_{-\frac{T}{2}}^{\frac{T}{2}}e^{-2\pi i (k/T)t}f(t) dt Ff(Tk)=∫−2T2Te−2πi(k/T)tf(t)dt
则 f ( t ) = ∑ k = − ∞ ∞ F f ( k T ) e 2 π i ( k / T ) t 1 T f(t) = \sum_{k = -\infty}^\infty\mathcal{F}f(\frac{k}{T}) e^{2\pi i (k/T)t}\frac{1}{T} f(t)=k=−∞∑∞Ff(Tk)e2πi(k/T)tT1
当 T → ∞ T\rightarrow \infty T→∞时, k T \frac{k}{T} Tk趋于连续,记为 s ∈ ( − ∞ , ∞ ) s\in (-\infty,\infty) s∈(−∞,∞),则求和变成积分
F f ( s ) = ∫ − ∞ ∞ e − 2 π i s t f ( t ) d t \mathcal{F}f(s)=\int_{-\infty}^{\infty}e^{-2\pi i st}f(t) dt Ff(s)=∫−∞∞e−2πistf(t)dt
f ( t ) = ∫ − ∞ ∞ F f ( s ) e 2 π i s t d s f(t) = \int_{-\infty}^\infty \mathcal{F} f(s) e^{2\pi i st} ds f(t)=∫−∞∞Ff(s)e2πistds
我们需要知道积分的收敛问题。
傅里叶变换将 f ( t ) f(t) f(t)分解为组成成分 e 2 π i s t e^{2\pi i st} e2πist,逆变换则从组成成分合成原函数
f ( t ) f(t) f(t)定义在时域, F f ( s ) \mathcal{F}f(s) Ff(s)定义在频域
信号都有一个频谱,频谱确定了信号
正逆变换
F f ( s ) = ∫ − ∞ ∞ e − 2 π i s t f ( t ) d t F − 1 g ( t ) = ∫ − ∞ ∞ e 2 π i s t g ( s ) d s \mathcal{F}f(s) = \int_{-\infty}^\infty e^{-2\pi i st}f(t) dt\\ \mathcal{F}^{-1}g(t) = \int_{-\infty}^\infty e^{2\pi i st}g(s) ds Ff(s)=∫−∞∞e−2πistf(t)dtF−1g(t)=∫−∞∞e2πistg(s)ds
傅里叶 变换表明,对函数的傅里叶变换进行反变换得到原函数
F − 1 F f = f F F − 1 g = g \mathcal{F}^{-1}\mathcal{F}f=f\\ \mathcal{F}\mathcal{F}^{-1}g=g F−1Ff=fFF−1g=g
在0点处,傅里叶变换为函数的平均值
F f ( 0 ) = ∫ − ∞ ∞ e 2 π i 0 t f ( t ) d t = ∫ − ∞ ∞ f ( t ) d t \mathcal{F} f(0) = \int_{-\infty}^\infty e^{2\pi i 0t} f(t) dt = \int_{-\infty}^\infty f(t)dt Ff(0)=∫−∞∞e2πi0tf(t)dt=∫−∞∞f(t)dt
F − 1 g ( 0 ) = ∫ − ∞ ∞ g ( s ) d s \mathcal{F} ^{-1} g(0) = \int_{-\infty}^\infty g(s) ds F−1g(0)=∫−∞∞g(s)ds
Examples:
矩形函数,或者叫 − 1 2 到 1 2 的 特 征 函 数 -\frac{1}{2}到\frac{1}{2}的特征函数 −21到21的特征函数
Π ( t ) = { 1 ∣ t ∣ < 1 2 0 ∣ t ∣ ≥ 1 2 \Pi(t) = \begin{cases} \begin{aligned} 1 \quad &|t| < \frac{1}{2}\\ 0 \quad &|t|\ge \frac{1}{2} \end{aligned} \end{cases} Π(t)=⎩⎪⎨⎪⎧10∣t∣<21∣t∣≥21
F Π ( s ) = ∫ − ∞ ∞ e − 2 π i s t Π ( t ) d t = ∫ − 1 2 1 2 e − 2 π i s t d t = [ 1 − 2 π i s e − 2 π i s t ] ∣ − 1 2 1 2 = − 1 2 π i s e − π i s + 1 2 π i s e π i s = 1 π s ( e π i s − e − π i s 2 i ) = sin π s π s \begin{aligned}\mathcal{F} \Pi(s) &= \int_{-\infty}^\infty e^{-2\pi i st} \Pi(t) dt\\ &= \int_{-\frac{1}{2}}^{\frac{1}{2}}e^{-2\pi i st} dt\\ &=\left[\frac{1}{-2\pi i s} e^{-2\pi i st} \right] \left|_{-\frac{1}{2}}^{\frac{1}{2}} \right. = \frac{-1}{2\pi i s} e^{-\pi i s} + \frac{1}{2\pi i s} e^{\pi i s}\\ &=\frac{1}{\pi s} \left(\frac{e^{\pi i s} - e^{-\pi i s}}{2i} \right) = \frac{\sin \pi s}{\pi s} \end{aligned} FΠ(s)=∫−∞∞e−2πistΠ(t)dt=∫−2121e−2πistdt=[−2πis1e−2πist]∣∣∣−2121=2πis−1e−πis+2πis1eπis=πs1(2ieπis−e−πis)=πssinπs
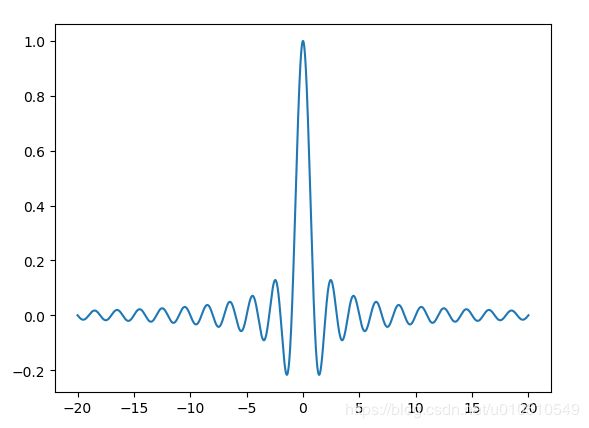
最后这个函数就是sinc函数 s i n c ( s ) = sin π s π s sinc(s) = \frac{\sin \pi s}{\pi s} sinc(s)=πssinπs
函数图像:
三角形函数
Λ ( t ) = { 1 − ∣ t ∣ ∣ t ∣ ≤ 1 0 ∣ t ∣ ≥ 1 \Lambda(t) = \begin{cases}1-|t| \quad &|t| \le 1\\ 0 \quad & |t| \ge 1 \end{cases} Λ(t)={1−∣t∣0∣t∣≤1∣t∣≥1
F Λ ( s ) = ∫ − ∞ ∞ e − 2 π i s t Λ ( t ) d t = ∫ − 1 0 e − 2 π i s t ( 1 + t ) d t + ∫ 0 1 e − 2 π i s t ( 1 − t ) d t = sin 2 π s π 2 s 2 = s i n c 2 ( s ) \begin{aligned}\mathcal{F} \Lambda(s) &= \int_{-\infty}^\infty e^{-2\pi i st}\Lambda (t) dt\\ &=\int_{-1}^0 e^{-2\pi i st} (1+t) dt + \int_0^1 e^{-2\pi i st}(1-t)dt\\ &=\frac{\sin^2 \pi s}{\pi^2 s^2} =sinc^2 (s)\end{aligned} FΛ(s)=∫−∞∞e−2πistΛ(t)dt=∫−10e−2πist(1+t)dt+∫01e−2πist(1−t)dt=π2s2sin2πs=sinc2(s)
傅里叶变换符号
在不同的上下文和材料中,傅里叶的定义和符号是变化的(不是唯一的)
比如
f ^ ( s ) = F f ( s ) \hat{f}(s) = \mathcal{F}f(s) f^(s)=Ff(s)
f ˇ ( t ) = F − 1 f ( t ) \check{f}(t) = \mathcal{F}^{-1}f(t) fˇ(t)=F−1f(t)
或者
f ( t ) , F ( s ) f(t),F(s) f(t),F(s)
有时候将正变换表示为(正负变换相调换)
F f ( s ) = ∫ − ∞ ∞ e − 2 π i s t f ( t ) d t \mathcal{F}f(s) = \int_{-\infty}^\infty e^{-2\pi i st}f(t) dt Ff(s)=∫−∞∞e−2πistf(t)dt
有时候表示为
F f ( s ) = ∫ − ∞ ∞ e 2 π i s t f ( t ) d t \mathcal{F}f(s) = \int_{-\infty}^\infty e^{2\pi i st}f(t) dt Ff(s)=∫−∞∞e2πistf(t)dt
高斯函数
f ( t ) = e − π t 2 f(t) = e^{-\pi t^2} f(t)=e−πt2指数加 π \pi π使得面积为1,则
F f ( s 0 = e − π s 2 \mathcal{F} f(s0 = e^{-\pi s^2} Ff(s0=e−πs2
为本身。我们得到结论,高斯函数的傅里叶变换等于本身
证明:
F ( s ) = ∫ − ∞ ∞ e − 2 π i s t e − π t 2 d t F(s) = \int_{-\infty}^\infty e^{-2\pi i st}e^{-\pi t^2}dt F(s)=∫−∞∞e−2πiste−πt2dt
求微分
F ′ ( s ) = ∫ − ∞ ∞ d d s ( e − 2 π i s t e − π t 2 ) d t = ∫ − ∞ ∞ − 2 π i t e − 2 π i s t e − π t 2 d t = i ∫ − ∞ ∞ e − 2 π i s t ( − 2 π t e − π t 2 ) d t \begin{aligned} F'(s)&=\int_{-\infty}^\infty \frac{d}{ds} \left(e^{-2\pi i st}e^{-\pi t^2} \right)dt \\ &= \int_{-\infty}^\infty -2\pi i t e^{-2\pi i st}e^{-\pi t^2}dt \\ &=i \int_{-\infty}^\infty e^{-2\pi i st} (-2\pi te^{-\pi t^2})dt \end{aligned} F′(s)=∫−∞∞dsd(e−2πiste−πt2)dt=∫−∞∞−2πite−2πiste−πt2dt=i∫−∞∞e−2πist(−2πte−πt2)dt
分部积分
= i ∫ − ∞ ∞ e − 2 π i s t d e − π t 2 = i e − 2 π i s t e − π t 2 ∣ − ∞ ∞ − i ∫ − ∞ ∞ e − π t 2 d e − 2 π i s t = − ∫ − ∞ ∞ 2 π s e − π t 2 e − 2 π i s t d t = − 2 π s ∫ − ∞ ∞ e − 2 π i s t e − π t 2 d t = − 2 π s F ( s ) \begin{aligned} &=i\int_{-\infty}^\infty e^{-2\pi i st} de^{-\pi t^2} \\ &=ie^{-2\pi i st} e^{-\pi t^2} \bigg| ^\infty_{-\infty} - i \int_{-\infty}^\infty e^{-\pi t^2} d e^{-2\pi i st}\\ &=- \int_{-\infty}^\infty 2\pi s \,e^{-\pi t^2}e^{-2\pi i st} dt\\ &=-2\pi s \int_{-\infty}^\infty e^{-2\pi i st} e^{-\pi t^2}dt\\ &=-2\pi s F(s) \end{aligned} =i∫−∞∞e−2πistde−πt2=ie−2πiste−πt2∣∣∣∣−∞∞−i∫−∞∞e−πt2de−2πist=−∫−∞∞2πse−πt2e−2πistdt=−2πs∫−∞∞e−2πiste−πt2dt=−2πsF(s)
于是我们得到等式
F ′ ( s ) = − 2 π s F ( s ) F'(s) = -2\pi sF(s) F′(s)=−2πsF(s)
(这个ODE表明) F ( 是 ) = F ( 0 ) e − π s 2 F(是) = F(0)e^{-\pi s^2} F(是)=F(0)e−πs2
而 F ( 0 ) = ∫ − ∞ ∞ f ( t ) d t = 1 F(0) = \int_{-\infty}^\infty f(t) dt= 1 F(0)=∫−∞∞f(t)dt=1
故 F ( s ) = e − π s 2 F(s)=e^{-\pi s^2} F(s)=e−πs2
证明完毕
对偶性(傅里叶变换的对偶性质)
根据定义有
F f ( − s ) = F − 1 f ( s ) \mathcal{F} f(-s) = \mathcal{F}^{-1}f(s) Ff(−s)=F−1f(s)
F f ( s ) = F − 1 f ( − s ) \mathcal{F} f(s) = \mathcal{F}^{-1}f(-s) Ff(s)=F−1f(−s)
定义 f − ( t ) = f ( − t ) f^-(t) = f(-t) f−(t)=f(−t)(反转信号),按照记号记 ( F f ) − ( s ) = F f ( − s ) (\mathcal{F}f)^-(s) = \mathcal{F}f(-s) (Ff)−(s)=Ff(−s)(这是一个记号),则按前面有
( F f ) − = F − 1 f (\mathcal{F}f)^- = \mathcal{F}^{-1}f (Ff)−=F−1f
还有
F ( f − ) = F − 1 f \mathcal{F} (f^-) = \mathcal{F}^{-1}f F(f−)=F−1f
所以
( F f ) − = F ( f − ) = F − 1 f (\mathcal{F}f)^- =\mathcal{F} (f^-) = \mathcal{F}^{-1}f (Ff)−=F(f−)=F−1f
根据前面有
F F f = f − \mathcal{F}\mathcal{F}f = f^- FFf=f−
因为 F F f = F F − 1 f − = f − \mathcal{F}\mathcal{F}f = \mathcal{F}\mathcal{F}^{-1} f^- = f^- FFf=FF−1f−=f−
例子: s i n c = sin π t π t sinc = \frac{\sin \pi t}{\pi t} sinc=πtsinπt
根据对偶性质
F s i n c = F F π = π − = π \mathcal{F} sinc = \mathcal{F}\mathcal{F}\pi = \pi^- = \pi Fsinc=FFπ=π−=π
如果直接按照定义求解积分,积分存在严重的收敛问题,难以计算
同样可以得到 F s i n c 2 = Λ \mathcal{F}sinc^2 = \Lambda Fsinc2=Λ
三个重要问题:时延(delays),时域尺度变换,卷积
时延:如果信号移动b,傅里叶变换会怎样
f ( t ) ↔ F ( s ) f(t) \leftrightarrow F(s) f(t)↔F(s)
f ( t − b ) ↔ ? f(t-b) \leftrightarrow ? f(t−b)↔?
F ( s ) = ∫ − ∞ ∞ − e − 2 π i s t f ( t ) d t F(s) = \int_{-\infty}^\infty -e^{-2\pi i st}f(t) dt F(s)=∫−∞∞−e−2πistf(t)dt
F f ( t − b ) = ∫ − ∞ ∞ e − 2 π i s t f ( t − b ) d t = ∫ − ∞ ∞ e − 2 π i s ( u + b ) f ( u ) d u = e − 2 π i s b ∫ − ∞ ∞ e − 2 π i s u f ( u ) d u = e − 2 π i s b F ( s ) \begin{aligned}\mathcal{F} f(t - b) &= \int_{-\infty}^\infty e^{-2\pi i st} f( t - b) dt\\ &=\int_{-\infty}^\infty e^{-2\pi i s(u+b)}f(u) du \\ &= e^{-2\pi i sb} \int_{-\infty}^\infty e^{-2\pi i su}f(u) du\\ &= e^{-2\pi i sb}F(s) \end{aligned} Ff(t−b)=∫−∞∞e−2πistf(t−b)dt=∫−∞∞e−2πis(u+b)f(u)du=e−2πisb∫−∞∞e−2πisuf(u)du=e−2πisbF(s)
即(时延定理),时域的位移导致频域的位移
F ( f ( t ± b ) ) ( s ) = e ± 2 π i s b F ( s ) \mathcal{F}\left(f(t\pm b) \right)(s) =e^{\pm 2\pi i sb} F(s) F(f(t±b))(s)=e±2πisbF(s)
记(复数表示成幅度乘以角度)
F ( s ) = ∣ F ( s ) ∣ e 2 π i θ ( s ) F(s) = |F(s)| e^{2\pi i \theta(s)} F(s)=∣F(s)∣e2πiθ(s)
故
e − 2 π i s b F ( s ) = ∣ F ( s ) ∣ e 2 π i ( θ ( s ) − s b ) e^{-2\pi i sb}F(s) = |F(s)| e^{2\pi i (\theta (s)- sb)} e−2πisbF(s)=∣F(s)∣e2πi(θ(s)−sb)
时域的位移导致频域角度的变换,幅度不变
接下来是尺度变换
f ( t ) ↔ F ( s ) f(t) \leftrightarrow F(s) f(t)↔F(s)
f ( a t ) ↔ ? f(at) \leftrightarrow ? f(at)↔?
F ( f ( a t ) ) ( s ) = ∫ − ∞ ∞ e − 2 π i s t f ( a t ) d t \mathcal{F}\left(f(at) \right)(s) = \int_{-\infty}^\infty e^{-2\pi i st} f(at) dt F(f(at))(s)=∫−∞∞e−2πistf(at)dt
i f a > 0 if\, a >0 ifa>0,令 u = a t u = at u=at
F ( f ( a t ) ) ( s ) = 1 a ∫ − ∞ ∞ e − 2 π i ( s / a ) u f ( u ) d u = 1 a ∫ − ∞ ∞ e − 2 π i ( s / a ) u f ( u ) d u = 1 a F ( s a ) \begin{aligned}\mathcal{F}\left(f(at) \right)(s) &= \frac{1}{a}\int_{-\infty}^\infty e^{-2\pi i (s/a)u} f(u) du \\ &=\frac{1}{a}\int_{-\infty}^\infty e^{-2\pi i (s/a)u} f(u) du=\frac{1}{a} F(\frac{s}{a}) \end{aligned} F(f(at))(s)=a1∫−∞∞e−2πi(s/a)uf(u)du=a1∫−∞∞e−2πi(s/a)uf(u)du=a1F(as)
i f a < 0 if\, a <0 ifa<0,令 u = a t u = at u=at
F ( f ( a t ) ) ( s ) = − 1 a ∫ − ∞ ∞ e − 2 π i ( s / a ) u f ( u ) d u = − 1 a F ( s a ) \mathcal{F}\left(f(at) \right)(s) = -\frac{1}{a}\int_{-\infty}^\infty e^{-2\pi i (s/a)u} f(u) du=-\frac{1}{a} F(\frac{s}{a}) F(f(at))(s)=−a1∫−∞∞e−2πi(s/a)uf(u)du=−a1F(as)
综上
F ( f ( a t ) ) ( s ) = 1 ∣ a ∣ F ( s a ) \mathcal{F}\left(f(at) \right)(s) = \frac{1}{|a|} F(\frac{s}{a}) F(f(at))(s)=∣a∣1F(as)
f ( a t ) ↔ 1 ∣ a ∣ F ( s a ) f(at) \leftrightarrow \frac{1}{|a|} F(\frac{s}{a}) f(at)↔∣a∣1F(as)
这个结果说明,当 ∣ a ∣ > 1 |a|>1 ∣a∣>1时,频谱被拉长了,而且变矮, ∣ a ∣ < 1 |a|<1 ∣a∣<1则相反
即时域压缩(伸长),则频域伸长(压缩)
Heisenberg测不准定理实际上也是依赖傅里叶变换证明,不能同事间压缩时域和频域,即不能同时知道位置和速度
卷积运算
关于怎样使用一个函数对另一个函数或信号进行调制,大部分情况下着手于改变信号的频谱
eg.线性和叠加性
F ( f + g ) = F ( f ) + F ( g ) \mathcal{F}(f+g) = \mathcal{F}(f) + \mathcal{F}(g) F(f+g)=F(f)+F(g)
通过改变频谱调制信号
如果是相乘呢
( F g ) ( F f ) = F ( ? ? f 和 g 的 某 种 组 合 ? ) (\mathcal{F}g)(\mathcal{F}f) = \mathcal{F}(??f和g的某种组合?) (Fg)(Ff)=F(??f和g的某种组合?)
G g ( s ) F f ( s ) = ∫ − ∞ ∞ e − 2 π i s t g ( t ) d t ∫ − ∞ ∞ e − 2 π i s x f ( x ) d x = ∫ − ∞ ∞ ∫ − ∞ ∞ e − 2 π i s ( t + x ) g ( t ) f ( x ) d t d x = ∫ − ∞ ∞ ( ∫ − ∞ ∞ e − 2 π i s ( t + x ) g ( t ) d t ) f ( x ) d x \begin{aligned}\mathcal{G} g(s) \mathcal{F} f(s) &= \int_{-\infty}^\infty e^{-2\pi i st } g(t) dt \int_{-\infty}^\infty e^{-2\pi i sx } f(x) dx\\ &= \int_{-\infty}^\infty \int_{-\infty}^\infty e^{-2\pi i s(t+ x) } g(t) f(x) dt dx\\ &= \int_{-\infty}^\infty \left( \int_{-\infty}^\infty e^{-2\pi i s(t+ x) } g(t)dt \right) f(x) dx\end{aligned} Gg(s)Ff(s)=∫−∞∞e−2πistg(t)dt∫−∞∞e−2πisxf(x)dx=∫−∞∞∫−∞∞e−2πis(t+x)g(t)f(x)dtdx=∫−∞∞(∫−∞∞e−2πis(t+x)g(t)dt)f(x)dx
令 u = t + x , d u = d t , t = u − x u=t+x,du=dt,t=u-x u=t+x,du=dt,t=u−x,的上式为
∫ − ∞ ∞ ( ∫ − ∞ ∞ e − 2 π i s u g ( u − x ) d u ) f ( x ) d x \int_{-\infty}^\infty \left( \int_{-\infty}^\infty e^{-2\pi i su } g(u-x)du \right) f(x) dx ∫−∞∞(∫−∞∞e−2πisug(u−x)du)f(x)dx
改变积分顺序
∫ − ∞ ∞ ( ∫ − ∞ ∞ g ( u − x ) f ( x ) d x ) e − 2 π i s u d u \int_{-\infty}^\infty \left( \int_{-\infty}^\infty g(u-x)f(x) dx \right) e^{-2\pi i su} du ∫−∞∞(∫−∞∞g(u−x)f(x)dx)e−2πisudu
定义 h ( u ) = ∫ − ∞ ∞ g ( u − x ) f ( x ) d x h(u)=\int_{-\infty}^\infty g(u-x)f(x) dx h(u)=∫−∞∞g(u−x)f(x)dx,上面就是
∫ − ∞ ∞ e − 2 π i s u h ( u ) d u = F h ( s ) \int_{-\infty}^\infty e^{-2\pi i su} h(u) du = \mathcal{F}h(s) ∫−∞∞e−2πisuh(u)du=Fh(s)
即 ( F g ) ( F f ) = F h ( s ) (\mathcal{F}g)(\mathcal{F}f) =\mathcal{F}h(s) (Fg)(Ff)=Fh(s)
h ( u ) h(u) h(u)即称为 g g g和 f f f的卷积函数,定义 g g g和 f f f的卷积:
( g ∗ f ) ( x ) = ∫ − ∞ ∞ g ( x − y ) f ( y ) d y (g*f)(x)=\int_{-\infty}^\infty g(x-y)f(y) dy (g∗f)(x)=∫−∞∞g(x−y)f(y)dy
依照上面的卷积定理我们有
F ( g ∗ f ) = F ( g ) F ( f ) \mathcal{F}(g*f) =\mathcal{F}(g)\mathcal{F}(f) F(g∗f)=F(g)F(f)
Briggs, William L.Henson写的离散傅里叶手册中有很好的问题例子
eg.:浑浊度研究:与测量睡的清澈度有关,假如得到一张测量数据 T T T。这个数据有很多的毛刺,我们需要去除掉这些毛刺,使之平滑。在频域进行处理,去掉高频的成分,方法是呈上一个矩形函数(也叫低通滤波) Π 2 V c ( 在 − V c 和 V c 之 间 为 1 , 其 他 为 0 ) \Pi_{2V_c}(在-V_c和V_c之间为1,其他为0) Π2Vc(在−Vc和Vc之间为1,其他为0)得到
Π 2 V c F T \Pi_{2V_c}\mathcal{F}T Π2VcFT
转换到时域得
2 V c s i n c ( 2 V c t ) ∗ T ( t ) 2V_c sinc(2V_c t) * T(t) 2Vcsinc(2Vct)∗T(t)
滤波通常(不总是)等同于卷积
在频域中(频域滤波)
G ( s ) = F ( s ) H ( s ) G(s) = F(s) H(s) G(s)=F(s)H(s)( H ( s ) H(s) H(s)为传递函数),设计滤波器就是设计一个 H H H。
比较常见的事低通滤波器,高通滤波器和带通滤波器
在频域更容易理解滤波器而在时域中转换为卷积就没那么好理解
有时卷积并不容易计算,甚至不能显式表示,如
∫ − ∞ ∞ s i n c ( x − y ) f ( y ) d y \int_{-\infty}^\infty sinc(x - y) f(y) dy ∫−∞∞sinc(x−y)f(y)dy
有什么关于卷积更好的解释? 有很多的解释
在很多情况下,卷积核滤波或平均相关
通常 f f f和 g g g的卷积比单独考虑 f , g f,g f,g更好(更平滑),比如
Π ∗ Π = Λ \Pi * \Pi = \Lambda Π∗Π=Λ
左边不连续,右边连续
如果 f f f可微 g g g不可微,则 f ∗ g f*g f∗g可微: ( f ∗ g ) ′ = f ′ ∗ g (f*g)' = f' * g (f∗g)′=f′∗g
怎样利用卷积求热方程??
首先
F ( f ′ ) ( s ) = 2 π i s F f ( s ) \mathcal{F}(f')(s) = 2\pi i s \mathcal{F}f(s) F(f′)(s)=2πisFf(s)
相似的
F ( f ( n ) ) ( s ) = ( 2 π i s ) n F f ( s ) \mathcal{F}(f^{(n)})(s) = (2\pi i s)^n \mathcal{F}f(s) F(f(n))(s)=(2πis)nFf(s)
证明:
假设当 t → ± ∞ , f ( t ) → 0 t\rightarrow \pm \infty ,f(t) \rightarrow 0 t→±∞,f(t)→0(一种特殊情形,一般要把函数限制在一个族里面,分析学专门讨论)
F ( f ′ ) ( s ) = ∫ − ∞ ∞ e − 2 π i s t f ′ ( t ) d t = e − 2 π i s t f ( t ) ∣ − ∞ ∞ + 2 π i s ∫ − ∞ ∞ e − 2 π i s t f ( t ) d t = 0 + 2 π i s F f ( s ) \begin{aligned} \mathcal{F}(f')(s) &= \int_{-\infty}^\infty e^{-2\pi i st}f'(t) dt\\ &=e^{-2\pi i st}f(t) \bigg|_{-\infty}^\infty + 2\pi i s \int_{-\infty}^\infty e^{-2\pi i st} f(t) dt \\ &=0 + 2\pi i s \mathcal{F}f(s)\end{aligned} F(f′)(s)=∫−∞∞e−2πistf′(t)dt=e−2πistf(t)∣∣∣∣−∞∞+2πis∫−∞∞e−2πistf(t)dt=0+2πisFf(s)
研究无限长实线热方程, u ( x , t ) , u ( x , 0 ) = f ( x ) u(x,t),u(x,0)=f(x) u(x,t),u(x,0)=f(x)无限周期 u t = 1 2 u x x u_t = \frac{1}{2} u_{xx} ut=21uxx
记 u ( x , t ) u(x,t) u(x,t)关于 x x x的傅里叶变换为 u ( s , t ) u(s,t) u(s,t),则
F u t = ∫ − ∞ ∞ e − 2 π i s x ∂ u ( x , t ) ∂ t d x = ∂ ∂ t ( ∫ − ∞ ∞ e − 2 π i s x u ( x , t ) d x ) = ∂ ∂ t u ( s , t ) \begin{aligned} \mathcal{F} u_t &= \int_{-\infty}^\infty e^{-2\pi i sx} \frac{\partial u(x,t)}{\partial t}dx\\ &=\frac{\partial}{\partial t}\left( \int_{-\infty}^\infty e^{-2\pi i sx} u (x,t) dx \right) \\ &=\frac{\partial}{\partial t} u(s,t)\end{aligned} Fut=∫−∞∞e−2πisx∂t∂u(x,t)dx=∂t∂(∫−∞∞e−2πisxu(x,t)dx)=∂t∂u(s,t)
F u x x = ( 2 π i s ) 2 u ( s , t ) \mathcal{F}u_{xx} = (2\pi i s)^2 u(s,t) Fuxx=(2πis)2u(s,t)
联合上面,得到热方程为
∂ ∂ t u ( s , t ) = − 2 ( π s ) 2 u ( s , t ) \frac{\partial}{\partial t} u(s,t)=- 2(\pi s)^2 u(s,t) ∂t∂u(s,t)=−2(πs)2u(s,t)
这是一个普通的微分方程,解得
u ( s , t ) = u ( s , 0 ) e − 2 π 2 s 2 t u(s,t) = u(s,0) e^{-2\pi^2 s^2 t} u(s,t)=u(s,0)e−2π2s2t
u ( s , 0 ) = ∫ − ∞ ∞ e − 2 π i s x u ( x , 0 ) d x = ∫ − ∞ ∞ e − 2 π i s x f ( x ) = F ( s ) u(s,0) = \int_{-\infty}^\infty e^{-2\pi i sx} u(x,0) dx = \int_{-\infty}^\infty e^{-2\pi i sx} f(x) = F(s) u(s,0)=∫−∞∞e−2πisxu(x,0)dx=∫−∞∞e−2πisxf(x)=F(s)
所以
u ( s , t ) = F ( s ) e − 2 π 2 s 2 t u(s,t) = F(s) e^{-2\pi^2 s^2 t} u(s,t)=F(s)e−2π2s2t
由于
F ( 1 2 π t e − x 2 / 2 t ) = e − 2 π 2 s 2 t \mathcal{F}\left(\frac{1}{\sqrt{2\pi t}}e^{-x^2 /2t} \right) = e^{-2\pi^2 s^2 t} F(2πt1e−x2/2t)=e−2π2s2t
故
u ( x , t ) = f ( x ) ∗ ( 1 2 π t e − x 2 / 2 t ) u(x,t) = f(x) * \left(\frac{1}{\sqrt{2\pi t}}e^{-x^2 /2t} \right) u(x,t)=f(x)∗(2πt1e−x2/2t)
卷积核中心极限定理(conbolution and the Central Limit Theorem,CLT)
CLT某种程度上阐述了一般钟形曲线的一般形式,也就是概率论中的高斯分布
大部分概率事件,可以看做可以按照钟形曲线进行计算和近似,或者说有高斯函数决定
p ( x ) = 1 2 π e − x 2 / 2 p(x) = \frac{1}{\sqrt{2\pi}}e^{-x^2/2} p(x)=2π1e−x2/2
p ( a ≤ m e a s u r e m e n t ) ≤ b = ∫ a b 1 2 π e − x 2 / 2 d x p(a\le measurement ) \le b = \int_a^b \frac{1}{\sqrt{2\pi}}e^{-x^2/2} dx p(a≤measurement)≤b=∫ab2π1e−x2/2dx
对矩形函数自身做一次卷积,得到一个三角形函数,做三次卷积则得到一个钟形(更平滑),做四个更平滑。令人惊叹(spooky)的是,不仅对矩形函数,对任意函数,自身卷积做若干次以后,将类似于一个钟形函数
建立:
X X X为随机变量, x x x为时机测量值,测量 x x x如何分布 P X ( x ) P_X(x) PX(x), P a , b ( a ≤ x ≤ b ) = ∫ a b p ( x ) d x P_{a,b}(a\le x \le b) = \int_a^b p(x) dx Pa,b(a≤x≤b)=∫abp(x)dx
∫ − ∞ ∞ p ( x ) d x = 1 , p ( x ) ≥ 0 \int_{-\infty}^{\infty} p(x) dx = 1,p(x) \ge 0 ∫−∞∞p(x)dx=1,p(x)≥0
怎么和卷积联系起来
假设 x 1 , x 2 x_1,x_2 x1,x2是独立随机变量 p 1 ( x 1 ) , p 2 ( x 2 ) , x 1 + x 2 p_1(x_1),p_2(x_2),x_1 + x_2 p1(x1),p2(x2),x1+x2的分布为 p 1 ∗ p 2 p_1 * p_2 p1∗p2(利用积分变量替换即可得到)
同样可以得到
p ( x 1 + ⋯ + x n ) = p 1 ∗ p 2 ∗ ⋯ ∗ p n p(x_1+\cdots+x_n) = p_1 * p_2 * \cdots * p_n p(x1+⋯+xn)=p1∗p2∗⋯∗pn
CLT建立过程
设 x 1 , ⋯ , x n x_1,\cdots,x_n x1,⋯,xn是独立同分布随机变量(iid),记为p(均值为0,方差为1)
即
∫ − ∞ ∞ x p ( x ) d x = 0 \int_{-\infty}^{\infty} xp(x) dx = 0 ∫−∞∞xp(x)dx=0
∫ − ∞ ∞ x 2 p ( x ) d x = 1 \int_{-\infty}^{\infty} x^2p(x) dx = 1 ∫−∞∞x2p(x)dx=1
∫ − ∞ ∞ p ( x ) d x = 1 \int_{-\infty}^{\infty} p(x) dx = 1 ∫−∞∞p(x)dx=1
S n = x 1 + ⋯ + x n S_n = x_1 + \cdots + x_n Sn=x1+⋯+xn,则 , E ( S n ) = 0 , E ( S n 2 ) = n \mathbb{E}(S_n) = 0, \sqrt{\mathbb{E}(S_n^2)} = \sqrt{n} E(Sn)=0,E(Sn2)=n(标准差)
故, S n n \frac{S_n}{\sqrt{n}} nSn方差为1
CLT:当 n → ∞ n\rightarrow \infty n→∞时,
lim n → ∞ p r o b ( a ≤ S n n ≤ b ) = 1 2 π ∫ a b e − x 2 / 2 d x \lim_{n\rightarrow \infty} prob(a \le \frac{S_n}{\sqrt{n}} \le b ) = \frac{1}{\sqrt{2\pi}} \int_a^b e^{-x^2/2} dx n→∞limprob(a≤nSn≤b)=2π1∫abe−x2/2dx
非积分形式:如果 P n ( x ) P_n(x) Pn(x)为 S n n \frac{S_n}{\sqrt{n}} nSn的分布,则当 n → ∞ n\rightarrow \infty n→∞时,
P n ( x ) → 1 2 π e − x 2 / 2 P_n(x) \rightarrow \frac{1}{\sqrt{2\pi}} e^{-x^2/2} Pn(x)→2π1e−x2/2
因为 x 1 + ⋯ + x n x_1+\cdots +x_n x1+⋯+xn的分布为 p ∗ n ( x ) = ( p ∗ ⋯ ∗ p ⎵ n 个 ) p^{*n}(x) =( \underbrace{p*\cdots * p}_{n个}) p∗n(x)=(n个 p∗⋯∗p),则
x 1 + ⋯ + x n n \frac{x_1+\cdots+x_n}{\sqrt{n}} nx1+⋯+xn的分布为 n p ∗ n ( n x ) \sqrt{n} p^{*n}(\sqrt{n}x) np∗n(nx). ( x 分 布 为 p ( x ) , 则 a x 的 分 布 为 1 a p ( x a ) (x分布为p(x),则ax的分布为\frac{1}{a} p(\frac{x}{a}) (x分布为p(x),则ax的分布为a1p(ax)
P r ( a X ≤ x ) = P r ( X ≤ x a ) = ∫ − ∞ p ( t ) d t = ∫ − ∞ x p ( t a ) d t a = 1 a ∫ − ∞ x p ( t a ) d t \begin{aligned}Pr(aX \le x) &= Pr(X \le \frac{x}{a}) = \int_{-\infty}^{ } p(t) dt \\ &= \int_{-\infty}^{ x} p\left(\frac{t}{a}\right) d\frac{t}{a} = \frac{1}{a} \int_{-\infty}^x p\left(\frac{t}{a}\right) dt \end{aligned} Pr(aX≤x)=Pr(X≤ax)=∫−∞p(t)dt=∫−∞xp(at)dat=a1∫−∞xp(at)dt
做傅里叶变换 F ( p n ( x ) ) = n F ( p ∗ n ( n x ) ) = n ∗ 1 n F ( p ∗ n ) ( s n ) = ( F p ) n ( s n ) = ( F p ( s n ) ) n \begin{aligned}\mathcal{F}(p_n(x)) &= \sqrt{n} \mathcal{F} \left(p^{*n}(\sqrt{n} x )\right)\\ &= \sqrt{n} * \frac{1}{\sqrt{n} } \mathcal{F} \left(p^{*n}\right) \left(\frac{s}{ \sqrt{n}} \right) \\ &= (\mathcal{F}p)^n \left(\frac{s}{ \sqrt{n}} \right)\\ &= \left(\mathcal{F}p\left(\frac{s}{ \sqrt{n}} \right) \right)^n \end{aligned} F(pn(x))=nF(p∗n(nx))=n∗n1F(p∗n)(ns)=(Fp)n(ns)=(Fp(ns))n
使用泰勒级数(展开) ( e x = 1 + x + x 2 2 + ⋯ ) (e^x = 1 +x + \frac{x^2}{2}+\cdots) (ex=1+x+2x2+⋯)
F p ( s n ) = ∫ − ∞ ∞ e − 2 π i ( s / n ) x p ( x ) d x = ∫ − ∞ ∞ ( 1 − 2 π i s x n − 1 2 ( 2 π i s x n ) 2 + ⋯ ) p ( x ) d x = ∫ − ∞ ∞ p ( x ) d x ⎵ = 1 − 2 π i s n ∫ − ∞ ∞ x p ( x ) d x ⎵ = 0 − 2 π 2 s 2 n ∫ − ∞ ∞ x 2 p ( x ) d x ⎵ = 1 + ⋯ = 1 − 2 π 2 s 2 n + ε ≈ 1 − 2 π 2 s 2 n \begin{aligned}\mathcal{F}p\left(\frac{s}{ \sqrt{n}} \right) &= \int_{-\infty}^\infty e^{-2\pi i (s/\sqrt{n})x} p(x) dx \\ &= \int_{-\infty}^\infty \left(1-\frac{2\pi i sx}{\sqrt{n}} - \frac{1}{2} \left(\frac{2\pi i sx}{\sqrt{n}}\right)^2 + \cdots \right) p(x) dx\\ &= \underbrace{\int_{-\infty}^\infty p(x) dx}_{=1} - \frac{2\pi i s}{\sqrt{n}}\underbrace{\int_{-\infty}^\infty xp(x) dx}_{=0} - \frac{2\pi^2 s^2}{n} \underbrace{ \int_{-\infty}^\infty x^2 p(x) dx}_{=1} + \cdots \\ &=1 -\frac{2\pi^2 s^2}{n}+ \varepsilon \approx 1 -\frac{2\pi^2 s^2}{n} \end{aligned} Fp(ns)=∫−∞∞e−2πi(s/n)xp(x)dx=∫−∞∞(1−n2πisx−21(n2πisx)2+⋯)p(x)dx==1 ∫−∞∞p(x)dx−n2πis=0 ∫−∞∞xp(x)dx−n2π2s2=1 ∫−∞∞x2p(x)dx+⋯=1−n2π2s2+ε≈1−n2π2s2
所以
( F p ( s n ) ) n ≈ ( 1 − 2 π 2 s 2 n ) n \left(\mathcal{F}p\left(\frac{s}{ \sqrt{n}} \right) \right)^n \approx \left(1 -\frac{2\pi^2 s^2}{n} \right)^n (Fp(ns))n≈(1−n2π2s2)n
当 n → + ∞ n\rightarrow +\infty n→+∞, ( 1 − 2 π 2 s 2 n ) n ⟶ e − 2 π 2 s 2 \left(1 -\frac{2\pi^2 s^2}{n} \right)^n \longrightarrow e^{-2\pi^2s^2} (1−n2π2s2)n⟶e−2π2s2
利用傅里叶反变换,饿到原分布密度为
p n ( x ) ≈ 1 2 π e − x 2 / 2 p_n(x) \approx \frac{1}{\sqrt{2\pi}} e^{-x^2/2} pn(x)≈2π1e−x2/2
讨论积分收敛问题
需要傅里叶变换的更严谨的定义才能处理一般信号。如果积分不收敛,怎么利用傅里叶变换
两种方式处理这种情况
1.一种是使用特定方法(ad hot)
2.重新研究基础,给出一个另外的定义
第一个例子: f ( t ) = Π ( t ) f(t) = \Pi(t) f(t)=Π(t)积分收敛可以进行傅里叶变换,然而问题出现在反变换上 F − 1 s i n c = Π \mathcal{F}^{-1} sinc = \Pi F−1sinc=Π
F − 1 s i n c = ∫ − ∞ ∞ e 2 π i s t sin π s π s d s = { 1 , ∣ s ∣ < 1 2 0 , ∣ s ∣ > 1 2 \mathcal{F}^{-1} sinc = \int_{-\infty}^\infty e^{2\pi i st } \frac{\sin \pi s}{\pi s} ds = \begin{cases}1,\quad &|s| < \frac{1}{2} \\ 0,\quad &|s| > \frac{1}{2} \end{cases} F−1sinc=∫−∞∞e2πistπssinπsds={1,0,∣s∣<21∣s∣>21
(无法用简单积分算出,需要用一些技巧)
在边界上即不连续点上 s = ± 1 2 s=\pm \frac{1}{2} s=±21有些问题,收敛于 1 2 \frac{1}{2} 21而不是0或者1
第二个例子中, f ( t ) = 1 f(t) = 1 f(t)=1,傅里叶积分是无意义的
∫ − ∞ ∞ e − 2 π i s t d t = 1 − 2 π i s e − 2 π i s t ∣ − ∞ ∞ = 0 \int_{-\infty}^\infty e^{-2\pi i st } dt = \frac{1}{-2\pi i s} e^{-2\pi i st } \bigg|_{-\infty}^\infty = 0 ∫−∞∞e−2πistdt=−2πis1e−2πist∣∣∣∣−∞∞=0
例子3: f ( t ) = sin ( 2 π t ) , g ( t ) = cos ( 2 π t ) f(t) = \sin(2\pi t),g(t) =\cos(2\pi t) f(t)=sin(2πt),g(t)=cos(2πt)
∫ − ∞ ∞ e − 2 π i s t sin ( 2 π t ) d t \int_{-\infty}^\infty e^{-2\pi i st } \sin(2\pi t) dt ∫−∞∞e−2πistsin(2πt)dt
是无法收敛的。对于等等这些情况,我们必须重新定义收敛的问题
怎样选取基本现象来啊研究其他一切现象
1940年代解决了这个问题。退一步,最好的情形是什么(定义为 s s s,Schwarz)
我们需要两个性质:
1.如果 f ∈ s f \in s f∈s,那么 F ∈ s \mathcal{F} \in s F∈s
2.傅里叶由方程定义
F F − 1 f = f \mathcal{F}\mathcal{F}^{-1}f = f FF−1f=f
更进一步的性质(Parseval等式Rayleigh 等式)
∫ − ∞ ∞ ∣ g ( s ) ∣ 2 d s = ∫ − ∞ ∞ ∣ f ( t ) ∣ 2 d t \int_{-\infty}^\infty |g(s) |^2 ds = \int_{-\infty}^\infty |f(t)|^2 dt ∫−∞∞∣g(s)∣2ds=∫−∞∞∣f(t)∣2dt
怎样定义 s s s(Laurent Schwartz找到了这个类):s指的就是这类速降函数
{ f ( x ) 为 光 滑 函 数 对 任 意 m , n ≥ 0 , 当 x → ± ∞ , ∣ x ∣ n ∣ d m d x m f ( x ) ∣ → 0 \begin{cases}f(x) 为光滑函数\\ 对任意m,n\ge 0,当x \rightarrow \pm \infty,|x|^n |\frac{d^m}{dx^m}f(x) | \rightarrow 0 \end{cases} {f(x)为光滑函数对任意m,n≥0,当x→±∞,∣x∣n∣dxmdmf(x)∣→0
即递降速度非常快,比 x n x^n xn快. 存在这样的函数吗
{ f ( x ) = e − π x 2 就 是 这 样 的 函 数 在 一 个 区 间 外 全 为 0 的 光 滑 函 数 ( 紧 支 集 光 滑 函 数 ) \begin{cases}f(x) = e^{-\pi x^2}就是这样的函数\\在一个区间外全为0的光滑函数(紧支集光滑函数) \end{cases} {f(x)=e−πx2就是这样的函数在一个区间外全为0的光滑函数(紧支集光滑函数)
这是从导数定理中来的
F ( f ( m ) ) ( s ) = ( 2 π i s ) m F f ( s ) \mathcal{F}(f^{(m)})(s) = (2\pi i s)^m \mathcal{F}f(s) F(f(m))(s)=(2πis)mFf(s)
这表明可微性和递降速率的关系
Parseval等式适用于s函数,适用于不涉及收敛问题的函数
∫ − ∞ ∞ ∣ F ( f ) ( s ) ∣ 2 d s = ∫ − ∞ ∞ ∣ f ( t ) ∣ 2 d t \int_{-\infty}^\infty |\mathcal{F}(f)(s) |^2 ds = \int_{-\infty}^\infty |f(t)|^2 dt ∫−∞∞∣F(f)(s)∣2ds=∫−∞∞∣f(t)∣2dt
下面证明一个更一般的等式
∫ − ∞ ∞ F f ( s ) F g ( s ) ‾ d s = ∫ − ∞ ∞ f ( t ) g ( t ) ‾ d t \int_{-\infty}^\infty \mathcal{F}f(s) \overline{\mathcal{F}g(s)} ds = \int_{-\infty}^\infty f(t) \overline{g(t)} dt ∫−∞∞Ff(s)Fg(s)ds=∫−∞∞f(t)g(t)dt
令 g ( t ) = ∫ − ∞ ∞ e 2 π i s t F g ( s ) d s g(t) = \int_{-\infty}^\infty e^{2\pi i st} \mathcal{F}g(s) ds g(t)=∫−∞∞e2πistFg(s)ds,即 g ( t ) = F − 1 F g g(t)=\mathcal{F}^{-1}\mathcal{F}g g(t)=F−1Fg
两边取共轭,将共轭算子放进积分中
g ( t ) ‾ = ∫ − ∞ ∞ e − 2 π i s t F g ( s ) ‾ d s \overline{g(t)} = \int_{-\infty}^\infty e^{-2\pi i st} \overline{\mathcal{F}g(s)} ds g(t)=∫−∞∞e−2πistFg(s)ds
故
∫ − ∞ ∞ f ( x ) g ( t ) ‾ d t = ∫ − ∞ ∞ f ( t ) ( ∫ − ∞ ∞ e − 2 π i s t F g ( s ) ‾ d s ) d t = ∫ − ∞ ∞ F g ( s ) ‾ ( ∫ − ∞ ∞ f ( t ) e − 2 π i s t d t ) d s = ∫ − ∞ ∞ F f ( s ) F g ( s ) ‾ d s \begin{aligned}\int_{-\infty}^\infty f(x)\overline{g(t)}dt &= \int_{-\infty}^\infty f(t) \left( \int_{-\infty}^\infty e^{-2\pi i st} \overline{\mathcal{F}g(s)} ds\right)dt \\ &=\int_{-\infty}^\infty \overline{\mathcal{F}g(s)} \left( \int_{-\infty}^\infty f(t) e^{-2\pi i st} dt\right)ds\\ &= \int_{-\infty}^\infty \mathcal{F}f(s) \overline{\mathcal{F}g(s)} ds \end{aligned} ∫−∞∞f(x)g(t)dt=∫−∞∞f(t)(∫−∞∞e−2πistFg(s)ds)dt=∫−∞∞Fg(s)(∫−∞∞f(t)e−2πistdt)ds=∫−∞∞Ff(s)Fg(s)ds
傅里叶变换扩展(重新定义傅里叶变换,广义傅里叶变换)
傅里叶变换的最佳集合,速降函数集合s
为什么这个对于傅里叶变幻时最佳的
Π ∉ s , Λ , sin , cos , s i n c ∉ s , 常 数 c ∉ s \Pi \notin s,\Lambda,\sin,\cos,sinc \notin s,常数c \notin s Π∈/s,Λ,sin,cos,sinc∈/s,常数c∈/s,许多常见的函数都不属于 s s s,怎么办,必须采取一条特别的路,那就是广义函数(也称为分布)
脉冲函数 δ \delta δ(单独考虑是没有意义的)
通常定义 δ \delta δ为
δ ( 0 ) = 0 , x ≠ 0 δ ( 0 ) = ∞ \delta(0) = 0,x \ne 0\\ \delta(0) = \infty \quad\quad\,\,\, δ(0)=0,x̸=0δ(0)=∞
∫ − ∞ ∞ δ ( x ) d x = 1 \int_{-\infty}^\infty \delta(x) dx = 1 ∫−∞∞δ(x)dx=1
∫ − ∞ ∞ δ ( 0 ) φ ( x ) d x = φ ( 0 ) \int_{-\infty}^\infty \delta(0) \varphi(x) dx = \varphi(0) ∫−∞∞δ(0)φ(x)dx=φ(0)
上面的定义完全是垃圾。
δ \delta