- 数据分析常用指标名词解释及计算公式
走过冬季
学习笔记数据分析大数据
数据分析中有大量常用指标,它们帮助我们量化业务表现、用户行为、产品健康度等。下面是一些核心指标的名词解释及计算方式,按常见类别分类:一、流量与用户规模指标页面浏览量名词解释:用户访问网站或应用时,每次加载或刷新一个页面就算一次PV。它衡量的是页面被打开的总次数。计算方式:PV=∑(所有页面被加载的次数)(通常由埋点或日志直接统计)独立访客数名词解释:在特定时间范围内(如一天、一周、一月),访问网站
- 第一次在CSDN 使用Markdown编辑页,就看到了完美的语法,在此处,我记录一下
撰卢
编辑器笔记
这里写自定义目录标题欢迎使用Markdown编辑器新的改变功能快捷键合理的创建标题,有助于目录的生成如何改变文本的样式插入链接与图片如何插入一段漂亮的代码片生成一个适合你的列表创建一个表格设定内容居中、居左、居右SmartyPants创建一个自定义列表如何创建一个注脚注释也是必不可少的KaTeX数学公式新的甘特图功能,丰富你的文章UML图表FLowchart流程图导出与导入导出导入欢迎使用Mark
- 搜广推校招面经九十三
Y1nhl
搜广推面经机器学习人工智能python算法推荐算法pytorch搜索算法
字节懂车帝一面一、NDCG(NormalizedDiscountedCumulativeGain)的计算NDCG是信息检索和排序任务中常用的评价指标,用于衡量模型预测的排序质量与真实相关性排序的一致程度。1.1.DCG@k(DiscountedCumulativeGain)DCG@k=∑i=1krelilog2(i+1)\text{DCG@k}=\sum_{i=1}^{k}\frac{rel_i
- 试题公式ocr识别数据集
试题公式ocr识别数据集insurance_formula_latexhttps://github.com/LeeXYZABC/insurance_formula_latex.gitreference---
- jmeter的时间戳函数使用
flower_1111
代码jmeterjmeter
原文:https://blog.csdn.net/jocleyn/article/details/834144331、__time:获取时间戳、格式化时间(1)、${__time(yyyy-MM-ddHH:mm:ss:SSS,time)}:格式化生成时间格式2018-10-2611:08:23:635(2)、${__time(,)}:默认该公式精确到毫秒级别,13位数1527822855323(3
- Python 实战人工智能数学基础:推荐系统应用
AI天才研究院
AI大模型企业级应用开发实战大数据人工智能语言模型JavaPython架构设计
作者:禅与计算机程序设计艺术文章目录1.背景介绍2.核心概念与联系2.1用户画像2.2相似性计算2.2.1基于物品的相似度2.2.2基于用户的相似度2.3协同过滤算法2.3.1基于用户的协同过滤算法2.3.2基于物品的协同过滤算法2.3.3基于上下文的协同过滤算法3.核心算法原理和具体操作步骤以及数学模型公式详细讲解3.1基于用户的协同过滤算法3.2基于物品的协同过滤算法3.3混合协同过滤算法3.
- word中viso/math type公式比文字大
主要是mathtype中字号和word中字号是分别设置的,需要单独点开复制之后的公式,进入编辑状态,调整大小,让mathtype所对应的pt磅值和word中所对应的字号一样。字号对应关系如何调mathtype大小viso同理
- 强化学习之 DQN、Double DQN、PPO
JNU freshman
强化学习强化学习
文章目录通俗理解DQNDoubleDQNPPO结合公式理解通俗理解DQN一个简单的比喻和分步解释来理解DQN(DeepQ-Network,深度Q网络),就像教小朋友学打游戏一样:先理解基础概念:Q学习(Q-Learning)想象你在教一只小狗玩电子游戏(比如打砖块)。小狗每做一个动作(比如“向左移动”或“发射球”),游戏会给出一个奖励(比如得分增加)或惩罚(比如球掉了)。小狗的目标是通过不断尝试,
- 李群与李代数2:李代数求导和李群扰动模型
龙焰智能
SLAM数学基础自动驾驶高等数学李群李代数BCH公式微分模型扰动模型相似变换群
李群与李代数2:李代数求导和李群扰动模型1.整体误差最小化引出求导问题2.BCH公式与近似形式2.1BCH公式2.2BCH线性近似2.3BCH近似的意义3.微分模型——李代数求导4.扰动模型求导(左乘)4.1SO(3)上的扰动模型求导4.2SE(3)上的扰动模型求导4.3伴随性质5.相似变换群相关5.1相似变换群Sim(3)Sim(3)Sim(3)5.2李代数sim(3)\mathfrak{sim
- 动态时间规整(Dynamic Time Warping,DTW)补充案例
EmorZhong
python人工智能机器学习算法动态规划
DTW的边界条件是确保累积距离矩阵计算“有起点、有规则”的基础,它规定了矩阵中第一行和第一列的累积距离如何计算(因为这两行/列是路径的“起点边缘”,没有“上一步”的全部选择)。下面结合具体场景和例子展开说明:为什么需要边界条件?累积距离矩阵(D[i][j])的核心递归公式是:[D[i][j]=\text{dist}[i][j]+\min\left(D[i-1][j],\D[i][j-1],\D[i
- 搜广推校招面经九十一
美团机器学习/数据挖掘算法工程师_二面一、介绍一下ESMM模型,是否有进行过函数推导传统的转化率建模方式:只用发生点击(click=1)的样本来训练CVR模型。CVR定义如下:CVR=P(y=1∣x,z=1)CVR=P(y=1|x,z=1)CVR=P(y=1∣x,z=1)y=1表示用户发生了转化(如购买)z=1表示用户点击了广告这样做的问题:样本选择偏差(SampleSelectionBias,S
- 技术岗面试中的一些常见问题
技术面试最重要的就是和岗位相关的技术能力,下面提到的这些问题并不是特别重要,面试面的多了就知道怎么说了,但为了避免大家踩坑,这里就先把这些常见的问题总结一些,因为这些问题比专业问题容易掌握的多。本片推文主要分为技术面喜欢问的问题、HR面喜欢问的问题、技术面反问面试官的问题及HR面反问面试官的问题。技术面喜欢问的问题:问:项目中遇到的困难?(高频问题)答:如果项目比较简单,还真没什么可讲的,这个只能
- 突破传统:Dell R730服务器RAID 5配置与智能监控全解析
芯作者
D2:ubuntu服务器linuxubuntu
在现代数据中心运维中,合理的存储配置是保障业务连续性的基石。今天,我们将深入探索DellPowerEdgeR730服务器的RAID5配置技巧,并结合热备盘策略、自动化监控脚本以及性能调优方案,为您呈现一份别开生面的技术指南。一、为什么RAID5+热备盘是企业级存储的黄金组合?RAID5通过分布式奇偶校验实现数据冗余,允许单块硬盘故障时不丢失数据。其存储效率公式为:Efficiency=\frac{
- 安防监控漏报频发?陌讯实时检测算法实测召回率98%
2501_92487721
目标跟踪计算机视觉人工智能算法
一、开篇痛点:安防监控的检测难题在夜间低光、遮挡、小目标等复杂场景下,传统YOLO系列算法常出现漏检(FN)和误检(FP)。某安防厂商测试数据显示:当目标像素<50×50时,开源模型召回率骤降至65%以下。二、技术解析:陌讯算法的三重创新陌讯视觉算法通过多尺度特征融合+自适应光照补偿提升鲁棒性:动态感受野机制在Backbone中引入可变形卷积(DeformableConv),公式表示为:y(p)=
- 无人机飞控系统中气压计软件驱动与应用入门指南
bother3000
无人机硬件嵌入式开发无人机无人机#传感器stm32无人机嵌入式硬件PX4航空仪表气压计可信计算技术
一、气压计在飞控系统中的核心作用1.1气压计的基本原理气压计(Barometer)基于压阻效应或电容效应工作,通过测量空气压力计算飞行高度。其基本公式为:其中:1.2在飞控系统中的关键应用应用场景具体作用高度测量提供绝对高度数据,精度通常为±0.5米(标准大气压下)定高飞行作为PID控制器的高度输入,实现稳定悬停气压趋势分析通过气压变化率判断上升/下降趋势,辅助姿态控制高度融合滤波与GPS高度、超
- 语音信号基础篇1-预加重(Pre-emphasis)
沐黎~
信号与系统语音识别人工智能
预加重就是对语音信号的高频进行补偿,语音信号90%能量集中在有效带宽低频分量上,高频分量频谱(一般我们用其幅度谱,通俗将就是频谱的模长或者绝对值长度)较小,我们让它变大一定,占比多,增强其高频分量。预加重原理也非常简单,其时域表达式非常简单,如下式子:公式中:一般取0.97时域看着就简单后一个减去前一个,看不出有什么规律,我们对其进行z变换,可得:合并同类项,可得:自变量为z,我们画出z变化后的频
- 2025社交电商新风口:推客小程序的商业逻辑与技术实现
wx_ywyy6798
信息可视化推客系统推客系统开发推客小程序推客小程序开发推客分销系统小程序
一、推客小程序市场前景与商业价值在当今社交电商蓬勃发展的时代,推客小程序已成为连接商家与消费者的重要桥梁。推客模式结合了社交传播与电商变现的双重优势,通过用户自发分享带来裂变式增长,为商家创造了全新的营销渠道。推客小程序的核心优势:低成本获客:利用用户社交关系链实现病毒式传播,大幅降低传统广告投放成本高转化率:基于熟人信任关系的推荐,转化率远超传统电商平台用户粘性强:通过佣金激励体系持续激活用户参
- 数据结构——1.数据结构和算法
爱看烟花的码农
数据结构数据结构
第一部分:笔试核心概念(理论知识)一、数据结构绪论什么是数据结构?数据结构不仅仅是数据,而是研究如何组织数据(结构化信息)的方法,目的是为了能够高效地处理这些数据。一个经典的公式是:算法+数据结构=程序。这表明,好的程序离不开高效的数据组织方式和处理算法。基本概念与术语数据(Data):是计算机可以识别、存储和处理的符号总称,是程序处理的“原料”。例如,一张图片、一段文字、股票行情、心电图数据等。
- 12 | 走向元宇宙:数字化工作与生活
_Rye_
元宇宙
专栏快接近尾声了。在之前的课程里,我们一直在用一个框架来概括元宇宙,那就是:元宇宙=立体互联网+价值互联网。这个公式可以帮助我们从宏观角度更好地理解元宇宙。当我们回归工作和生活,用更加个人化的角度来观察元宇宙时,我们可以换用另外一个等式:元宇宙=实体空间+数字空间。通过这个等式,我们可以看到,元宇宙将带给我们线上线下全面融合的数字生活。这一讲的讨论分成两个部分。首先,我们来看看自己周围的数字化发展
- 华为OD技术面试高频考点(算法篇、AI方向)
一、Transformer核心机制:自注意力(Self-Attention)公式:Attention=softmax(QK^T/√d_k)v运作原理:1.Q/K/V矩阵:输入向量通过线性变换生成Query(查询)、Key(键)、Value(值)2.注意力权重:Softmax(QKT/√d_k)→计算词与词之间的关联度3.输出:权重与Value加权求和→捕获长距离依赖-优势:并行计算、全局上下文感知
- 如何用python处理excel的数据(极值标准化)?
bug菌¹
全栈Bug调优(实战版)python极值标准化数据excel
本文收录于《全栈Bug调优(实战版)》专栏,主要记录项目实战过程中所遇到的Bug或因后果及提供真实有效的解决方案,希望能够助你一臂之力,帮你早日登顶实现财富自由;同时,欢迎大家关注&&收藏&&订阅!持续更新中,up!up!up!!全文目录:问题描述解决方案极值标准化公式:步骤:代码示例:代码说明:示例文件:额外功能:安装依赖:文末福利,等你来拿!✨️WhoamI?问题描述如何用python处理ex
- 掌握 Spring Data Redis,提升后端开发效率
掌握SpringDataRedis,提升后端开发效率关键词:SpringDataRedis、后端开发、缓存、数据持久化、效率提升摘要:本文旨在深入探讨SpringDataRedis这一强大的工具,帮助后端开发者更好地掌握它以提升开发效率。首先介绍SpringDataRedis的背景知识,包括其目的、适用读者等。接着详细阐述核心概念与联系,分析核心算法原理并给出具体操作步骤,通过数学模型和公式加深理
- 【论文阅读】Decoupled Knowledge Distillation
Bosenya12
论文阅读
摘要:最先进的蒸馏方法主要基于从中间层蒸馏出深层特征,而logit蒸馏的重要性则被大大忽视了。为了提供研究logit蒸馏的新观点,我们将经典的KD损失重新表述为两部分,即目标类知识蒸馏(TCKD)和非目标类知识蒸馏(NCKD)。我们实证调查并证明了两部分的效果:TCKD传递了有关训练样本“困难”的知识,而NCKD是logit蒸馏起作用的突出原因。更重要的是,我们揭示了经典的KD损失是一个耦合公式,
- Rust后端框架:助力快速开发
大厂资深架构师
SpringBoot开发实战rust网络开发语言ai
Rust后端框架:助力快速开发关键词:Rust、后端框架、快速开发、高性能、内存安全摘要:本文深入探讨了Rust后端框架在快速开发中的重要作用。首先介绍了Rust语言的背景及其在后端开发领域的崛起原因,接着详细阐述了常见Rust后端框架的核心概念、架构和工作原理,通过Python示例类比讲解了核心算法原理和具体操作步骤,还给出了相关的数学模型和公式。在项目实战部分,展示了如何搭建开发环境、实现源代
- 后端领域的自然语言处理技术应用
大厂资深架构师
SpringBoot开发实战自然语言处理easyui人工智能ai
后端领域的自然语言处理技术应用关键词:后端领域、自然语言处理、技术应用、算法原理、实际案例摘要:本文聚焦于后端领域中自然语言处理技术的应用。首先介绍了相关背景,包括目的范围、预期读者等。接着阐述核心概念与联系,通过文本示意图和Mermaid流程图展示其原理和架构。详细讲解了核心算法原理并给出Python源代码示例,同时介绍了数学模型和公式。通过项目实战,展示代码实际案例并进行详细解释。分析了自然语
- 题解:P13017 [GESP202506 七级] 线图
YLCHUP
刷题之路算法图论深度优先数学建模c++数据结构笔记
首先明白定义:线图L(G)L(G)L(G)的顶点对应原图GGG的边,当且仅当原图中的两条边有公共顶点时,对应的线图顶点之间有一条边。不难想到,对于原图中的每个顶点vvv,其度数d(v)d(v)d(v)对应的边集可以形成(d(v)2)\binom{d(v)}{2}(2d(v))对相邻边。每对相邻边在线图中会产生一条边。用公式表示就是这样的(设G=(V,E)G=(V,E)G=(V,E)):∣EL(G)
- 四、Actor-Critic Methods
沈夢昂志
DRL深度强化学习python深度学习
由于在看DRL论文中,很多公式都很难理解。因此最近在学习DRL的基本内容。再此说明,非常推荐B站“王树森老师的DRL强化学习”本文的图表及内容,都是基于王老师课程的后自行理解整理出的内容。目录A.书接上回1、Reinforce算法B.State-ValueFunctionC.PolicyNetWork(Actor)D.ActionValueNetwork(Critic)E.TraintheNeur
- 语言模型 RLHF 实践指南(一):策略网络、价值网络与 PPO 损失函数
在使用ProximalPolicyOptimization(PPO)对语言模型进行强化学习微调(如RLHF)时,大家经常会问:策略网络的动作概率是怎么来的?价值网络的得分是如何计算的?奖励从哪里来?损失函数怎么构建?微调后的旧轨迹还能用吗?这篇文章将以语言模型强化学习微调为例,结合实际实现和数学公式,深入解析PPO的关键计算流程。1️⃣策略网络:如何计算动作概率?策略网络πθ(a∣s)\pi_\t
- 万向节死锁公式推导
微小冷
机器人欧拉角旋转矩阵万向节万向节死锁旋转轴旋转
文章目录欧拉角的万向节死锁旋转轴欧拉角的万向节死锁如果把刚体的旋转沿着三个旋转轴进行拆分,那么可以变成三个旋转角的叠加,这三个旋转角就是欧拉角,分别对应旋转矩阵,为了书写方便,记Sθ=sinθ,Cθ=cosθS_\theta=\sin\theta,C_\theta=\cos\thetaSθ=sinθ,Cθ=cosθ,则三个旋转矩阵为Rx(θ)R_x(\theta)Rx(θ)Ry(θ)R_y(\
- BabelDOC入门手册一点就通
AhriProGramming
算法ocrpython
BabelDOC入门手册一点就通【#BubbleDOC震撼发布!科研党的终极翻译神器,PDF翻译从此告别排版灾难!】你是否经历过翻译PDF时公式错位、图表乱飞、版式崩坏的绝望?传统翻译工具的时代结束了!#BubbleDOC横空出世,凭借三大革命性突破,成为全球科研、金融、法律从业者的新宠✨核心亮点1️⃣无损解析:精准提取PDF内嵌公式、图表、脚注,误差<0.5mm,翻译后与原版像素级对齐;2️⃣智
- github中多个平台共存
jackyrong
github
在个人电脑上,如何分别链接比如oschina,github等库呢,一般教程之列的,默认
ssh链接一个托管的而已,下面讲解如何放两个文件
1) 设置用户名和邮件地址
$ git config --global user.name "xx"
$ git config --global user.email "
[email protected]"
- ip地址与整数的相互转换(javascript)
alxw4616
JavaScript
//IP转成整型
function ip2int(ip){
var num = 0;
ip = ip.split(".");
num = Number(ip[0]) * 256 * 256 * 256 + Number(ip[1]) * 256 * 256 + Number(ip[2]) * 256 + Number(ip[3]);
n
- 读书笔记-jquey+数据库+css
chengxuyuancsdn
htmljqueryoracle
1、grouping ,group by rollup, GROUP BY GROUPING SETS区别
2、$("#totalTable tbody>tr td:nth-child(" + i + ")").css({"width":tdWidth, "margin":"0px", &q
- javaSE javaEE javaME == API下载
Array_06
java
oracle下载各种API文档:
http://www.oracle.com/technetwork/java/embedded/javame/embed-me/documentation/javame-embedded-apis-2181154.html
JavaSE文档:
http://docs.oracle.com/javase/8/docs/api/
JavaEE文档:
ht
- shiro入门学习
cugfy
javaWeb框架
声明本文只适合初学者,本人也是刚接触而已,经过一段时间的研究小有收获,特来分享下希望和大家互相交流学习。
首先配置我们的web.xml代码如下,固定格式,记死就成
<filter>
<filter-name>shiroFilter</filter-name>
&nbs
- Array添加删除方法
357029540
js
刚才做项目前台删除数组的固定下标值时,删除得不是很完整,所以在网上查了下,发现一个不错的方法,也提供给需要的同学。
//给数组添加删除
Array.prototype.del = function(n){
- navigation bar 更改颜色
张亚雄
IO
今天郁闷了一下午,就因为objective-c默认语言是英文,我写的中文全是一些乱七八糟的样子,到不是乱码,但是,前两个自字是粗体,后两个字正常体,这可郁闷死我了,问了问大牛,人家告诉我说更改一下字体就好啦,比如改成黑体,哇塞,茅塞顿开。
翻书看,发现,书上有介绍怎么更改表格中文字字体的,代码如下
- unicode转换成中文
adminjun
unicode编码转换
在Java程序中总会出现\u6b22\u8fce\u63d0\u4ea4\u5fae\u535a\u641c\u7d22\u4f7f\u7528\u53cd\u9988\uff0c\u8bf7\u76f4\u63a5这个的字符,这是unicode编码,使用时有时候不会自动转换成中文就需要自己转换了使用下面的方法转换一下即可。
/**
* unicode 转换成 中文
- 一站式 Java Web 框架 firefly
aijuans
Java Web
Firefly是一个高性能一站式Web框架。 涵盖了web开发的主要技术栈。 包含Template engine、IOC、MVC framework、HTTP Server、Common tools、Log、Json parser等模块。
firefly-2.0_07修复了模版压缩对javascript单行注释的影响,并新增了自定义错误页面功能。
更新日志:
增加自定义系统错误页面功能
- 设计模式——单例模式
ayaoxinchao
设计模式
定义
Java中单例模式定义:“一个类有且仅有一个实例,并且自行实例化向整个系统提供。”
分析
从定义中可以看出单例的要点有三个:一是某个类只能有一个实例;二是必须自行创建这个实例;三是必须自行向系统提供这个实例。
&nb
- Javascript 多浏览器兼容性问题及解决方案
BigBird2012
JavaScript
不论是网站应用还是学习js,大家很注重ie与firefox等浏览器的兼容性问题,毕竟这两中浏览器是占了绝大多数。
一、document.formName.item(”itemName”) 问题
问题说明:IE下,可以使用 document.formName.item(”itemName”) 或 document.formName.elements ["elementName&quo
- JUnit-4.11使用报java.lang.NoClassDefFoundError: org/hamcrest/SelfDescribing错误
bijian1013
junit4.11单元测试
下载了最新的JUnit版本,是4.11,结果尝试使用发现总是报java.lang.NoClassDefFoundError: org/hamcrest/SelfDescribing这样的错误,上网查了一下,一般的解决方案是,换一个低一点的版本就好了。还有人说,是缺少hamcrest的包。去官网看了一下,如下发现:
- [Zookeeper学习笔记之二]Zookeeper部署脚本
bit1129
zookeeper
Zookeeper伪分布式安装脚本(此脚本在一台机器上创建Zookeeper三个进程,即创建具有三个节点的Zookeeper集群。这个脚本和zookeeper的tar包放在同一个目录下,脚本中指定的名字是zookeeper的3.4.6版本,需要根据实际情况修改):
#!/bin/bash
#!!!Change the name!!!
#The zookeepe
- 【Spark八十】Spark RDD API二
bit1129
spark
coGroup
package spark.examples.rddapi
import org.apache.spark.{SparkConf, SparkContext}
import org.apache.spark.SparkContext._
object CoGroupTest_05 {
def main(args: Array[String]) {
v
- Linux中编译apache服务器modules文件夹缺少模块(.so)的问题
ronin47
modules
在modules目录中只有httpd.exp,那些so文件呢?
我尝试在fedora core 3中安装apache 2. 当我解压了apache 2.0.54后使用configure工具并且加入了 --enable-so 或者 --enable-modules=so (两个我都试过了)
去make并且make install了。我希望在/apache2/modules/目录里有各种模块,
- Java基础-克隆
BrokenDreams
java基础
Java中怎么拷贝一个对象呢?可以通过调用这个对象类型的构造器构造一个新对象,然后将要拷贝对象的属性设置到新对象里面。Java中也有另一种不通过构造器来拷贝对象的方式,这种方式称为
克隆。
Java提供了java.lang.
- 读《研磨设计模式》-代码笔记-适配器模式-Adapter
bylijinnan
java设计模式
声明: 本文只为方便我个人查阅和理解,详细的分析以及源代码请移步 原作者的博客http://chjavach.iteye.com/
package design.pattern;
/*
* 适配器模式解决的主要问题是,现有的方法接口与客户要求的方法接口不一致
* 可以这样想,我们要写这样一个类(Adapter):
* 1.这个类要符合客户的要求 ---> 那显然要
- HDR图像PS教程集锦&心得
cherishLC
PS
HDR是指高动态范围的图像,主要原理为提高图像的局部对比度。
软件有photomatix和nik hdr efex。
一、教程
叶明在知乎上的回答:
http://www.zhihu.com/question/27418267/answer/37317792
大意是修完后直方图最好是等值直方图,方法是HDR软件调一遍,再结合不透明度和蒙版细调。
二、心得
1、去除阴影部分的
- maven-3.3.3 mvn archetype 列表
crabdave
ArcheType
maven-3.3.3 mvn archetype 列表
可以参考最新的:http://repo1.maven.org/maven2/archetype-catalog.xml
[INFO] Scanning for projects...
[INFO]
- linux shell 中文件编码查看及转换方法
daizj
shell中文乱码vim文件编码
一、查看文件编码。
在打开文件的时候输入:set fileencoding
即可显示文件编码格式。
二、文件编码转换
1、在Vim中直接进行转换文件编码,比如将一个文件转换成utf-8格式
&
- MySQL--binlog日志恢复数据
dcj3sjt126com
binlog
恢复数据的重要命令如下 mysql> flush logs; 默认的日志是mysql-bin.000001,现在刷新了重新开启一个就多了一个mysql-bin.000002
- 数据库中数据表数据迁移方法
dcj3sjt126com
sql
刚开始想想好像挺麻烦的,后来找到一种方法了,就SQL中的 INSERT 语句,不过内容是现从另外的表中查出来的,其实就是 MySQL中INSERT INTO SELECT的使用
下面看看如何使用
语法:MySQL中INSERT INTO SELECT的使用
1. 语法介绍
有三张表a、b、c,现在需要从表b
- Java反转字符串
dyy_gusi
java反转字符串
前几天看见一篇文章,说使用Java能用几种方式反转一个字符串。首先要明白什么叫反转字符串,就是将一个字符串到过来啦,比如"倒过来念的是小狗"反转过来就是”狗小是的念来过倒“。接下来就把自己能想到的所有方式记录下来了。
1、第一个念头就是直接使用String类的反转方法,对不起,这样是不行的,因为Stri
- UI设计中我们为什么需要设计动效
gcq511120594
UIlinux
随着国际大品牌苹果和谷歌的引领,最近越来越多的国内公司开始关注动效设计了,越来越多的团队已经意识到动效在产品用户体验中的重要性了,更多的UI设计师们也开始投身动效设计领域。
但是说到底,我们到底为什么需要动效设计?或者说我们到底需要什么样的动效?做动效设计也有段时间了,于是尝试用一些案例,从产品本身出发来说说我所思考的动效设计。
一、加强体验舒适度
嗯,就是让用户更加爽更加爽的用
- JBOSS服务部署端口冲突问题
HogwartsRow
java应用服务器jbossserverEJB3
服务端口冲突问题的解决方法,一般修改如下三个文件中的部分端口就可以了。
1、jboss5/server/default/conf/bindingservice.beans/META-INF/bindings-jboss-beans.xml
2、./server/default/deploy/jbossweb.sar/server.xml
3、.
- 第三章 Redis/SSDB+Twemproxy安装与使用
jinnianshilongnian
ssdbreidstwemproxy
目前对于互联网公司不使用Redis的很少,Redis不仅仅可以作为key-value缓存,而且提供了丰富的数据结果如set、list、map等,可以实现很多复杂的功能;但是Redis本身主要用作内存缓存,不适合做持久化存储,因此目前有如SSDB、ARDB等,还有如京东的JIMDB,它们都支持Redis协议,可以支持Redis客户端直接访问;而这些持久化存储大多数使用了如LevelDB、RocksD
- ZooKeeper原理及使用
liyonghui160com
ZooKeeper是Hadoop Ecosystem中非常重要的组件,它的主要功能是为分布式系统提供一致性协调(Coordination)服务,与之对应的Google的类似服务叫Chubby。今天这篇文章分为三个部分来介绍ZooKeeper,第一部分介绍ZooKeeper的基本原理,第二部分介绍ZooKeeper
- 程序员解决问题的60个策略
pda158
框架工作单元测试
根本的指导方针
1. 首先写代码的时候最好不要有缺陷。最好的修复方法就是让 bug 胎死腹中。
良好的单元测试
强制数据库约束
使用输入验证框架
避免未实现的“else”条件
在应用到主程序之前知道如何在孤立的情况下使用
日志
2. print 语句。往往额外输出个一两行将有助于隔离问题。
3. 切换至详细的日志记录。详细的日
- Create the Google Play Account
sillycat
Google
Create the Google Play Account
Having a Google account, pay 25$, then you get your google developer account.
References:
http://developer.android.com/distribute/googleplay/start.html
https://p
- JSP三大指令
vikingwei
jsp
JSP三大指令
一个jsp页面中,可以有0~N个指令的定义!
1. page --> 最复杂:<%@page language="java" info="xxx"...%>
* pageEncoding和contentType:
> pageEncoding:它
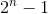
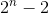
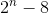

![f[n]=\prod_{i=0}^{n-1}\frac{2^n-2^i}{2^n}=\frac{\prod_{i=0}^{n-1}(2^n-2^i)}{2^{n^2}}](http://img.e-com-net.com/image/info8/46deee803edb4b9ba5b9c92ef2ec4ac1.gif)