- 动态规划DP问题(闫氏dp分析法+典例背包问题yxc讲解)
好喜欢吃红柚子
蓝桥杯动态规划c++蓝桥杯算法
1.DP问题总体分析我们需要找到的所有解是一个集合,由于需要考虑的数值涉及到物品数量i和背包重量j,所以使用一个二维数组f[i][j]来记录f[i][j]的含义是是从当前i个物品中选取物品加入背包,且物品总体积不超过j的物品最大价值最后的f[n][m]就是将n件物品装入背包时重量不超过m时的物品价值的最大值2.状态计算时的集合划分
- (nice!!!)(LeetCode 动态规划(基础版))309. 买卖股票的最佳时机含冷冻期(动态规划dp)
岁忧
C++JAVAGo版本LeetCodeleetcode动态规划算法c++gojava
题目:309.买卖股票的最佳时机含冷冻期思路:动态规划dp,时间复杂度为0(n)。细节看注释C++版本:classSolution{public:intmaxProfit(vector&prices){intn=prices.size();vector>f(n+2,vector(2,0));//f[i][0]:指在第i-2天时,没有持有股票的情况下的最大值//f[i][1]:指在第i-2天时,持有
- 2023年第十四届蓝桥杯省赛C++ 大学生A组
qq_56607982
蓝桥杯c++职场和发展
基本没有算法基础,第一次参加蓝桥杯,简单复盘一下。目录试题A幸运数分析枚举O(K)试题B有奖问答分析DFS试题C平方差分析枚举O(n^3)因数分解O(n*sqrt(n))奇偶判断O(n)试题D更小的数分析动态规划DPO(n^2)试题E颜色平衡树分析试题F买瓜分析试题I网络稳定性分析并查集+dijkstra算法试题A幸运数分析:1~10e8,不用考虑奇数位跳过的问题,直接枚举。枚举O(K)#incl
- Codeforces Round 946 (Div. 3)Codeforces Round 946 (Div. 3) 动态规划dp 01背包
DBWG
CF动态规划算法
Problem-E-Codeforces从第二天开始每天加x块钱。一共m天,每天都有一个商品,价格c[i],价值h[i]。问最后最多买多少h。本题就是在01背包上限制了个我们有的钱数。那这怎么办呢?其实我们可以问题分离,先把所有最优状态求出来,然后检查是否可行即可。dp[i]表示购买价值到i时,消耗的最少钱数。那么i-h[j]就是买这个商品j前的总价值,dp[i-h[j]]就是已经花的钱,而总钱数
- (nice!!!)(LeetCode 热题 100)32. 最长有效括号(动态规划dp)
岁忧
LeetCodejava版刷题LeetCode热题100leetcode动态规划算法javac++
题目:32.最长有效括号思路:动态规划,时间复杂度0(n)。C++版本:classSolution{public:intlongestValidParentheses(strings){intn=s.size();vectorf(n);//最长有效字符串intmx=0;//遍历每一个字符串的右端点for(inti=1;i=0&&s[i-f[i-1]-1]=='('){//是的话,可以组成更长的有效
- 【动态规划1】
m0_46150269
动态规划算法
力扣509.斐波那契数链接:link思路这是一道经典的动态规划DP题,做动态有5步:1.确定dp[i]含义,表示第i个数的斐波那契数值是dp[i]2.dp数组初始化3.确定递推公式4.确定遍历顺序,从递推公式可以知道dp[i]是依赖dp[i-1]和dp[i-2],那么遍历的顺序一定是从前到后遍历的5.举例推导,草稿完成classSolution{publicintfib(intn){if(n<=1
- 蓝桥杯算法基础(36)动态规划dp经典问题详解
湖前一人对影成双
算法蓝桥杯动态规划
动态规划-动态规划方法方法代表了这一类问题(最优子结构or子问题最优性)的有一半解法,是设计方法或者策略,不是具体算法-本质是递推,核心是找到状态转移的方式,写出dp方程-形式:记忆性递归递推01背包问题有n个重量和价值分别为wi,vi的物品,从这些物品中挑选出总重量不超过n的物品,求所有挑选方案中的值总和的最大值1=w[i]){intv1=v[i]+dfs(i+1,ww-w[i]);//选择当前
- 【动态规划】 解决背包问题 Python
Alexlllly
Python实现算法python算法动态规划leetcode
【动态规划】解决背包问题Python背包问题背包问题现在有3个物品篮球1kg1000元吉他3kg2000元单反4kg2500元有1个背包重4kg问怎么拿物品价值最大运用动态规划DP来解决此问题方法代码【源码】——思路来自麻省理工背包问题defbackpack(memory,item_weight,values,last_weight,index):'''memory:如果是已经计算过得分支则直接返
- (LeetCode 每日一题) 132. 分割回文串 II (动态规划dp)
岁忧
java版刷题LeetCodeleetcode动态规划算法javac++
题目:132.分割回文串II思路:最大分割次数为n-1,也就是分割为单个字符的次数。通过动态规划dp,可以得出字符串之间的哪段是回文串。再通过动态规划dp,算出使区间[0,i]为回文分割串所需要的最小分割次数f[i]。最后答案为f[n-1],时间复杂度为0(n^2)。classSolution{public:intn;vector>v;//动态规划一:判断回文串voiddp1(strings){v
- 数据结构与算法:动态规划dp:子序列相关力扣题(上):300. 最长递增子序列、674.最长连续递增序列
shanshandeisu
数据结构与算法LeetCode动态规划leetcode算法子序列力扣dp数据结构
300.最长递增子序列classSolution:deflengthOfLIS(self,nums:List[int])->int:length=len(nums)iflength==1:return1#dp[i]指的是以nums[i]为结尾的最长递增子序列的长度。dp=[1]*lengthmmax=1foriinrange(1,length):forjinrange(i):ifnums[i]>n
- 【Day38 LeetCode】动态规划DP 子序列问题Ⅱ
银河梦想家
leetcode动态规划算法
一、动态规划DP子序列问题Ⅱ1、最长公共子序列1143确定dp数组含义,dp[i][j]表示长度为[0,i-1]的字符串text1与长度为[0,j-1]的字符串text2的最长公共子序列的长度。dp转移关系,对于当前值dp[i][j],分为text1[i-1]与text2[j-1]相同与不相同两种情况。text1[i-1]与text2[j-1]相同时,这两个字符可以作为最长公共子序列的一部分,dp
- 奇怪的比赛(Python,递归,状态压缩动态规划dp)
不染_是非
python算法python动态规划算法蓝桥杯
目录前言:题目:思路:递归:代码及详细注释:状态压缩dp:代码及详细注释:总结:前言:这道题原本是蓝桥上的题,现在搜不到了,网上关于此题的讲解更是寥寥无几,仅有的讲解也只是递归思想,python讲解和状态压缩dp的解决方法都没有,这里就带大家用状态压缩dp方法来解决此题。题目:大奖赛计分规则:每位选手需要回答10个问题(其编号为1到10),越后面越有难度。答对的,当前分数翻倍;答错了,则扣掉与题号
- 数据结构与算法:动态规划dp:理论基础和相关力扣题(509.斐波那契数列、70.爬楼梯、62. 不同路径、63.不同路径Ⅱ、343.整数拆分)
shanshandeisu
数据结构与算法LeetCode动态规划leetcode算法dp力扣数据结构
1.0.理论基础动态规划主要解决的问题种类有:背包问题打家劫舍股票问题子序列问题解决步骤:dp数组及其下标的意义递推公式dp数组初始化遍历顺序打印dp数组2.0.相关力扣题509.斐波那契数列classSolution:deffib(self,n:int)->int:ifn==0:return0ifn==1:return1dp=[0]*35dp[1]=1foriinrange(2,31):dp[i
- 力扣_字符串2—最长有效括号
qweasdwxc
leetcode算法职场和发展
题目给你一个只包含‘(’和‘)’的字符串sss,找出最长有效(格式正确且连续)括号子串的长度。方法动态规划dp[i]dp[i]dp[i]表示以s[i]s[i]s[i]结尾的最长有效括号的长度如果s[i]s[i]s[i]为左括号,则dp[i]=0dp[i]=0dp[i]=0如果s[i]s[i]s[i]为右括号,若s[i−1]s[i-1]s[i−1]为左括号,则dp[i]=dp[i−2]+2dp[i]
- python蓝桥杯真题刷题打卡 | day2
代码魔法师!
python算法python蓝桥杯动态规划
目录数字三角形卡片排序成绩分析等差素数列数字三角形2020省赛动态规划dp输入输出样例输入:5738810274445265输出:27代码:importosimportsys#请在此输入您的代码h=int(input())#输入数据W=[list(map(int,input().split()))foriinrange(h)]#循环遍历计算到每一行的和的最大值foriinrange(1,h):fo
- USACO黄金级 USACO白金级
dllglvzhenfeng
USACO科普信息技术算法计算机考研信奥CSP-JC++
USACO黄金级参赛资格:通过银级比赛的选手难度等级:需要有一定的算法基础,理解一些抽象的方法(例:最短路径,动态规划),并且学生要对数据结构有比较深的了解。USACO信息学奥赛例题和算法金牌部分动态规划DP3(CSES1637RemovingDigitsUSACO信息学奥赛例题和算法金牌部分动态规划DP3(CSES1637RemovingDigits一维最小值递推)_哔哩哔哩_bilibiliU
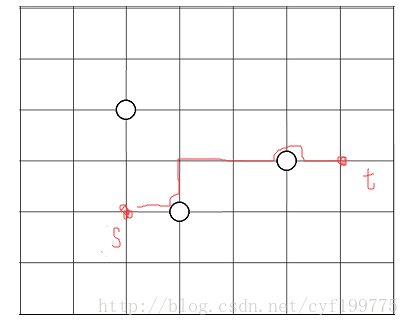
- ACWing动态规划DP采花生
Soraku7
刷题集刷题学习动态规划算法图论
HelloKitty想摘点花生送给她喜欢的米老鼠。她来到一片有网格状道路的矩形花生地(如下图),从西北角进去,东南角出来。地里每个道路的交叉点上都有种着一株花生苗,上面有若干颗花生,经过一株花生苗就能摘走该它上面所有的花生。HelloKitty只能向东或向南走,不能向西或向北走。问HelloKitty最多能够摘到多少颗花生。输入格式第一行是一个整数T,代表一共有多少组数据。接下来是T组数据。每组数
- 手把手教你dp:摘花生问题(递归改动态规划DP)
Lhw_666
动态规划算法c++递归法
dp系列手把手教你dp:01背包问题(递归改动态规划DP)手把手教你dp:摘花生问题(递归改动态规划DP)手把手教你dp:蓝桥杯-地宫寻宝(递归改动态规划DP)1摘花生HelloKitty想摘点花生送给她喜欢的米老鼠。她来到一片有网格状道路的矩形花生地(如下图),从西北角进去,东南角出来。地里每个道路的交叉点上都有种着一株花生苗,上面有若干颗花生,经过一株花生苗就能摘走该它上面所有的花生。Hell
- leetcode刷题笔记_二叉树相关
小白要努力sgy
leetcode笔记算法
二叉树相关合并二叉树:二叉搜索树相关第98题验证二叉搜索树第99题恢复二叉搜索树第1382题将二叉搜索树变平衡中序遍历+有序数组构建AVL树:*通过递归,自下而上不断地旋转,直到二叉搜索树满足平衡树AVL的条件:第938题二叉搜索树的范围和第110题高度平衡的二叉树自顶向下递归:自底向上递归:不同的二叉搜索树:第96题不同的二叉搜索树动态规划DP:*第95题不同的二叉搜索树II递归回溯:*第222
- Leetcode1143. 最长公共子序列
魑魅魍魉114
动态规划
解题思路求两个数组或者字符串的最长公共子序列问题,肯定是要用动态规划的。下面的题解并不难,你肯定能看懂。首先,区分两个概念:子序列可以是不连续的;子数组(子字符串)需要是连续的;另外,动态规划也是有套路的:单个数组或者字符串要用动态规划时,可以把动态规划dp[i]定义为nums[0:i]中想要求的结果;当两个数组或者字符串要用动态规划时,可以把动态规划定义成两维的dp[i][j],其含义是在A[0
- 【备战蓝桥杯】吃奶酪问题 / 超硬核,文附template拓展知识!
松叶子吖
蓝桥杯备赛新手帖蓝桥杯职场和发展
蓝桥杯备赛|洛谷做题打卡day9文章目录蓝桥杯备赛|洛谷做题打卡day9再来了解一下状压dp**简介(Introduction)****描述(Description)**-吃奶酪题目描述输入格式输出格式样例#1样例输入#1样例输出#1提示数据规模与约定提示*template拓展知识我的一些话【引入】今天的题目要用到动态规划DP的知识,因此先给大家普及一下背景:动态规划法是20世纪50年代由贝尔曼(
- 动态规划DP ---- 硬币问题(4) ---- 排列方案数
记得开心一点嘛
动态规划算法
欢迎收看本篇博客,今天给大家带来了排列组合输出方案数。在此之前,博主写过动态规划的硬币问题,给五种硬币(一分,五分,十分,二十五分,五十分)且不限制数量,请你输出其全部组合方案数。如果我们想要6分的话,其组成为1+1+1+1+1+1和5+1;那么如果咱们要输出其全部排列式方案数呢?其实改动不算太大,无非是将其遍历顺序颠倒:for(intj=0;i=w[i]){dp[j]+=dp[j-w[i]];}
- 代码随想录第第五十七天—回文子串,最长回文子序列
荒野饮冰室
动态规划数据结构算法
leetcode647.回文子串题目链接:回文子串版本一:动态规划dp数组及下标的含义dp[i][j]:区间范围[i,j](左闭右闭)的子串是否是回文子串,如果是dp[i][j]为true,否则为false。确定递推公式(1)当s[i]与s[j]不相等时,dp[i][j]=false(2)当s[i]与s[j]相等时,有如下三种情况:情况一:下标i与j相同,同一个字符,是回文子串情况二:下标i与j相
- dp专题10 目标和
Angindem
算法笔记算法数据结构
本题链接:.-力扣(LeetCode)题目:思路:根据这道题,可以通过暴力的方法进行取+号或者-号两个操作,通过当刚好得到target的时候答案+1,但是通过长度是20,操作状态为2个,随后的回溯暴力递归,最坏的情况时间复杂度大约是20^20^2,肯定会TLE了。这时候就用到了动态规划dp,这里我们可以知道有两个操作+-,我们分成两个子集,一些放正号子集left,另一些放负号子集righ。最后得到
- LeetCode(32):最长有效括号 Longest Valid Parentheses(Java)
NJU_ChopinXBP
JAVALeetCode数据结构与算法数据结构与算法LeetCode栈动态规划括号
2019.10.9#程序员笔试必备#LeetCode从零单刷个人笔记整理(持续更新)github:https://github.com/ChopinXBP/LeetCode-Babel括号题离不开栈,字符串题一般可以走动态规划,这道题主要有三种思路:1.动态规划dp[i]代表以第i个括号结尾的最长有效字符串长度。第一个子串必无效,dp[1]=0。顺序遍历,如果第i个括号为’(’,则字符串必无效,d
- labuladong日常刷题-前缀和数组 | LeetCode 303区域和检索-数组不可变 304二维区域和检索-矩阵不可变 | 差分数组 1094拼车
精彩文帅。
labuladong日常刷题leetcode矩阵算法数据结构c++
前缀和数组—动态规划的一种LeetCode303区域和检索-数组不可变2023.12.30题目链接labuladong讲解[链接]classNumArray{public:NumArray(vector&nums){//num=nums;//暴力求解//简单动态规划dp.resize(nums.size());dp[0]=nums[0];for(inti=1;inum;//暴力求解vectordp
- 192.【2023年华为OD机试真题(C卷)】Wonderland(动态规划DP—Java&Python&C++&JS实现)
一见已难忘
算法之翼—华为OD机试专栏华为odc语言动态规划WonderlandjavapythonJavaScript
请到本专栏顶置查阅最新的华为OD机试宝典点击跳转到本专栏-算法之翼:华为OD机试你的旅程将在这里启航!本专栏所有题目均包含优质解题思路,高质量解题代码,详细代码讲解,助你深入学习,深度掌握!文章目录【2023年华为OD机试真题(C卷)】Wonderland(动态规划DP—Java&Python&C++&JS实现)
- 183.【2023年华为OD机试真题(C卷)】虚拟游戏理财(动态规划dp实现Java&Python&C++&JS)
一见已难忘
算法之翼—华为OD机试专栏华为odc语言动态规划pythonjavajs虚拟游戏理财
请到本专栏顶置查阅最新的华为OD机试宝典点击跳转到本专栏-算法之翼:华为OD机试你的旅程将在这里启航!本专栏所有题目均包含优质解题思路,高质量解题代码,详细代码讲解,助你深入学习,深度掌握!文章目录183.【2023年华为OD机试真题(C卷)】虚拟游戏理财(动态规划dp实现Java&Python&C++&JS)
- 279. 完全平方数
莫小鹏
题目描述给定正整数n,找到若干个完全平方数(比如1,4,9,16,...)使得它们的和等于n。你需要让组成和的完全平方数的个数最少。示例1:输入:n=12输出:3解释:12=4+4+4.示例2:输入:n=13输出:2解释:13=4+9.分析使用动态规划dp[i]=min{dp[i-j*j]},1dp(n+1,0);dp[1]=1;for(inti=2;i<=n;i++){intmin=INT_MA
- 数据结构学习 Leetcode1143最长公共子序列
_Rindo
数据结构学习学习
动态规划最长公共子序列LCS这是我在看动态规划学习的时候做的。这是一篇LCS。LCS是两个数组进行比较。题目:思路:我觉得这个总结挺好的:求两个数组或者字符串的最长公共子序列问题,肯定是要用动态规划的。首先,区分两个概念:子序列可以是不连续的;子数组(子字符串)需要是连续的;另外,动态规划也是有套路的:单个数组或者字符串要用动态规划时,可以把动态规划dp[i]定义为nums[0:i]中想要求的结果
- 数据采集高并发的架构应用
3golden
.net
问题的出发点:
最近公司为了发展需要,要扩大对用户的信息采集,每个用户的采集量估计约2W。如果用户量增加的话,将会大量照成采集量成3W倍的增长,但是又要满足日常业务需要,特别是指令要及时得到响应的频率次数远大于预期。
&n
- 不停止 MySQL 服务增加从库的两种方式
brotherlamp
linuxlinux视频linux资料linux教程linux自学
现在生产环境MySQL数据库是一主一从,由于业务量访问不断增大,故再增加一台从库。前提是不能影响线上业务使用,也就是说不能重启MySQL服务,为了避免出现其他情况,选择在网站访问量低峰期时间段操作。
一般在线增加从库有两种方式,一种是通过mysqldump备份主库,恢复到从库,mysqldump是逻辑备份,数据量大时,备份速度会很慢,锁表的时间也会很长。另一种是通过xtrabacku
- Quartz——SimpleTrigger触发器
eksliang
SimpleTriggerTriggerUtilsquartz
转载请出自出处:http://eksliang.iteye.com/blog/2208166 一.概述
SimpleTrigger触发器,当且仅需触发一次或者以固定时间间隔周期触发执行;
二.SimpleTrigger的构造函数
SimpleTrigger(String name, String group):通过该构造函数指定Trigger所属组和名称;
Simpl
- Informatica应用(1)
18289753290
sqlworkflowlookup组件Informatica
1.如果要在workflow中调用shell脚本有一个command组件,在里面设置shell的路径;调度wf可以右键出现schedule,现在用的是HP的tidal调度wf的执行。
2.designer里面的router类似于SSIS中的broadcast(多播组件);Reset_Workflow_Var:参数重置 (比如说我这个参数初始是1在workflow跑得过程中变成了3我要在结束时还要
- python 获取图片验证码中文字
酷的飞上天空
python
根据现成的开源项目 http://code.google.com/p/pytesser/改写
在window上用easy_install安装不上 看了下源码发现代码很少 于是就想自己改写一下
添加支持网络图片的直接解析
#coding:utf-8
#import sys
#reload(sys)
#sys.s
- AJAX
永夜-极光
Ajax
1.AJAX功能:动态更新页面,减少流量消耗,减轻服务器负担
2.代码结构:
<html>
<head>
<script type="text/javascript">
function loadXMLDoc()
{
.... AJAX script goes here ...
- 创业OR读研
随便小屋
创业
现在研一,有种想创业的想法,不知道该不该去实施。因为对于的我情况这两者是矛盾的,可能就是鱼与熊掌不能兼得。
研一的生活刚刚过去两个月,我们学校主要的是
- 需求做得好与坏直接关系着程序员生活质量
aijuans
IT 生活
这个故事还得从去年换工作的事情说起,由于自己不太喜欢第一家公司的环境我选择了换一份工作。去年九月份我入职现在的这家公司,专门从事金融业内软件的开发。十一月份我们整个项目组前往北京做现场开发,从此苦逼的日子开始了。
系统背景:五月份就有同事前往甲方了解需求一直到6月份,后续几个月也完
- 如何定义和区分高级软件开发工程师
aoyouzi
在软件开发领域,高级开发工程师通常是指那些编写代码超过 3 年的人。这些人可能会被放到领导的位置,但经常会产生非常糟糕的结果。Matt Briggs 是一名高级开发工程师兼 Scrum 管理员。他认为,单纯使用年限来划分开发人员存在问题,两个同样具有 10 年开发经验的开发人员可能大不相同。近日,他发表了一篇博文,根据开发者所能发挥的作用划分软件开发工程师的成长阶段。
初
- Servlet的请求与响应
百合不是茶
servletget提交java处理post提交
Servlet是tomcat中的一个重要组成,也是负责客户端和服务端的中介
1,Http的请求方式(get ,post);
客户端的请求一般都会都是Servlet来接受的,在接收之前怎么来确定是那种方式提交的,以及如何反馈,Servlet中有相应的方法, http的get方式 servlet就是都doGet(
- web.xml配置详解之listener
bijian1013
javaweb.xmllistener
一.定义
<listener>
<listen-class>com.myapp.MyListener</listen-class>
</listener>
二.作用 该元素用来注册一个监听器类。可以收到事件什么时候发生以及用什么作为响
- Web页面性能优化(yahoo技术)
Bill_chen
JavaScriptAjaxWebcssYahoo
1.尽可能的减少HTTP请求数 content
2.使用CDN server
3.添加Expires头(或者 Cache-control) server
4.Gzip 组件 server
5.把CSS样式放在页面的上方。 css
6.将脚本放在底部(包括内联的) javascript
7.避免在CSS中使用Expressions css
8.将javascript和css独立成外部文
- 【MongoDB学习笔记八】MongoDB游标、分页查询、查询结果排序
bit1129
mongodb
游标
游标,简单的说就是一个查询结果的指针。游标作为数据库的一个对象,使用它是包括
声明
打开
循环抓去一定数目的文档直到结果集中的所有文档已经抓取完
关闭游标
游标的基本用法,类似于JDBC的ResultSet(hasNext判断是否抓去完,next移动游标到下一条文档),在获取一个文档集时,可以提供一个类似JDBC的FetchSize
- ORA-12514 TNS 监听程序当前无法识别连接描述符中请求服务 的解决方法
白糖_
ORA-12514
今天通过Oracle SQL*Plus连接远端服务器的时候提示“监听程序当前无法识别连接描述符中请求服务”,遂在网上找到了解决方案:
①打开Oracle服务器安装目录\NETWORK\ADMIN\listener.ora文件,你会看到如下信息:
# listener.ora Network Configuration File: D:\database\Oracle\net
- Eclipse 问题 A resource exists with a different case
bozch
eclipse
在使用Eclipse进行开发的时候,出现了如下的问题:
Description Resource Path Location TypeThe project was not built due to "A resource exists with a different case: '/SeenTaoImp_zhV2/bin/seentao'.&
- 编程之美-小飞的电梯调度算法
bylijinnan
编程之美
public class AptElevator {
/**
* 编程之美 小飞 电梯调度算法
* 在繁忙的时间,每次电梯从一层往上走时,我们只允许电梯停在其中的某一层。
* 所有乘客都从一楼上电梯,到达某层楼后,电梯听下来,所有乘客再从这里爬楼梯到自己的目的层。
* 在一楼时,每个乘客选择自己的目的层,电梯则自动计算出应停的楼层。
* 问:电梯停在哪
- SQL注入相关概念
chenbowen00
sqlWeb安全
SQL Injection:就是通过把SQL命令插入到Web表单递交或输入域名或页面请求的查询字符串,最终达到欺骗服务器执行恶意的SQL命令。
具体来说,它是利用现有应用程序,将(恶意)的SQL命令注入到后台数据库引擎执行的能力,它可以通过在Web表单中输入(恶意)SQL语句得到一个存在安全漏洞的网站上的数据库,而不是按照设计者意图去执行SQL语句。
首先让我们了解什么时候可能发生SQ
- [光与电]光子信号战防御原理
comsci
原理
无论是在战场上,还是在后方,敌人都有可能用光子信号对人体进行控制和攻击,那么采取什么样的防御方法,最简单,最有效呢?
我们这里有几个山寨的办法,可能有些作用,大家如果有兴趣可以去实验一下
根据光
- oracle 11g新特性:Pending Statistics
daizj
oracledbms_stats
oracle 11g新特性:Pending Statistics 转
从11g开始,表与索引的统计信息收集完毕后,可以选择收集的统信息立即发布,也可以选择使新收集的统计信息处于pending状态,待确定处于pending状态的统计信息是安全的,再使处于pending状态的统计信息发布,这样就会避免一些因为收集统计信息立即发布而导致SQL执行计划走错的灾难。
在 11g 之前的版本中,D
- 快速理解RequireJs
dengkane
jqueryrequirejs
RequireJs已经流行很久了,我们在项目中也打算使用它。它提供了以下功能:
声明不同js文件之间的依赖
可以按需、并行、延时载入js库
可以让我们的代码以模块化的方式组织
初看起来并不复杂。 在html中引入requirejs
在HTML中,添加这样的 <script> 标签:
<script src="/path/to
- C语言学习四流程控制if条件选择、for循环和强制类型转换
dcj3sjt126com
c
# include <stdio.h>
int main(void)
{
int i, j;
scanf("%d %d", &i, &j);
if (i > j)
printf("i大于j\n");
else
printf("i小于j\n");
retu
- dictionary的使用要注意
dcj3sjt126com
IO
NSDictionary *dict = [NSDictionary dictionaryWithObjectsAndKeys:
user.user_id , @"id",
user.username , @"username",
- Android 中的资源访问(Resource)
finally_m
xmlandroidStringdrawablecolor
简单的说,Android中的资源是指非代码部分。例如,在我们的Android程序中要使用一些图片来设置界面,要使用一些音频文件来设置铃声,要使用一些动画来显示特效,要使用一些字符串来显示提示信息。那么,这些图片、音频、动画和字符串等叫做Android中的资源文件。
在Eclipse创建的工程中,我们可以看到res和assets两个文件夹,是用来保存资源文件的,在assets中保存的一般是原生
- Spring使用Cache、整合Ehcache
234390216
springcacheehcache@Cacheable
Spring使用Cache
从3.1开始,Spring引入了对Cache的支持。其使用方法和原理都类似于Spring对事务管理的支持。Spring Cache是作用在方法上的,其核心思想是这样的:当我们在调用一个缓存方法时会把该方法参数和返回结果作为一个键值对存放在缓存中,等到下次利用同样的
- 当druid遇上oracle blob(clob)
jackyrong
oracle
http://blog.csdn.net/renfufei/article/details/44887371
众所周知,Oracle有很多坑, 所以才有了去IOE。
在使用Druid做数据库连接池后,其实偶尔也会碰到小坑,这就是使用开源项目所必须去填平的。【如果使用不开源的产品,那就不是坑,而是陷阱了,你都不知道怎么去填坑】
用Druid连接池,通过JDBC往Oracle数据库的
- easyui datagrid pagination获得分页页码、总页数等信息
ldzyz007
var grid = $('#datagrid');
var options = grid.datagrid('getPager').data("pagination").options;
var curr = options.pageNumber;
var total = options.total;
var max =
- 浅析awk里的数组
nigelzeng
二维数组array数组awk
awk绝对是文本处理中的神器,它本身也是一门编程语言,还有许多功能本人没有使用到。这篇文章就单单针对awk里的数组来进行讨论,如何利用数组来帮助完成文本分析。
有这么一组数据:
abcd,91#31#2012-12-31 11:24:00
case_a,136#19#2012-12-31 11:24:00
case_a,136#23#2012-12-31 1
- 搭建 CentOS 6 服务器(6) - TigerVNC
rensanning
centos
安装GNOME桌面环境
# yum groupinstall "X Window System" "Desktop"
安装TigerVNC
# yum -y install tigervnc-server tigervnc
启动VNC服务
# /etc/init.d/vncserver restart
# vncser
- Spring 数据库连接整理
tomcat_oracle
springbeanjdbc
1、数据库连接jdbc.properties配置详解 jdbc.url=jdbc:hsqldb:hsql://localhost/xdb jdbc.username=sa jdbc.password= jdbc.driver=不同的数据库厂商驱动,此处不一一列举 接下来,详细配置代码如下:
Spring连接池
- Dom4J解析使用xpath java.lang.NoClassDefFoundError: org/jaxen/JaxenException异常
xp9802
用Dom4J解析xml,以前没注意,今天使用dom4j包解析xml时在xpath使用处报错
异常栈:java.lang.NoClassDefFoundError: org/jaxen/JaxenException异常
导入包 jaxen-1.1-beta-6.jar 解决;
&nb