- 【数据标注师】关键词标注
试着
数据标注师数据标注师关键词标注
目录一、**理解关键词标注的核心逻辑**1.**三大标注原则**2.**关键词类型体系**二、**四阶训练体系**▶**阶段1:基础规则内化**▶**阶段2:语义浓缩训练**▶**阶段3:场景化标注策略**▶**阶段4:工具效率提升**三、**五大高频错误防御指南**四、**复杂场景突破策略**1.**隐喻处理方案**2.**多义词消歧流程**3.**跨语言混合标注**五、**持续进阶体系**1.
- Lucence 和 Elasticsearch 的区别?
码出财富
elasticsearch大数据搜索引擎
Lucene和Elasticsearch都是在信息检索和文本处理领域中广泛使用的工具,它们的主要区别如下:概念和定位Lucene:是一个基于Java的全文检索库,它提供了一套强大的底层索引和搜索功能的API。Lucene更像是一个工具包,开发人员可以基于它来构建自己的搜索应用程序,需要深入了解搜索的底层原理和算法,对开发者的技术要求较高。Elasticsearch:是一个基于Lucene的分布式搜
- 8、 探讨排序算法及其实际应用
侯昂
排序算法插入排序快速排序
探讨排序算法及其实际应用1.排序算法的重要性排序算法在计算机科学中扮演着至关重要的角色。无论是日常生活中常见的任务,还是复杂的数据处理工作,排序算法都能帮助我们更有效地管理和检索信息。以下是几个实际应用场景:字典中的单词:字典中的单词按顺序排列,忽略大小写差异。这使得查找特定单词变得非常容易。目录中的文件:目录中的文件通常按排序顺序列出,方便用户快速找到所需文件。书籍索引:一本书的索引是排序过的,
- X86和ARM架构的优缺点?
心对元&鑫鑫
arm开发架构
x86和ARM是当前主流的两种处理器架构,分别主导不同领域(如PC/服务器和移动/嵌入式设备)。它们的优缺点对比如下:1.x86架构(Intel/AMD)优点:高性能:复杂指令集(CISC)设计,单条指令功能强大,适合高吞吐量计算。强大的单线程性能,尤其在浮点运算和多媒体处理(如视频编辑、游戏)。软件生态成熟:主导PC和服务器市场,兼容Windows、Linux等主流操作系统。支持大量专业软件(如
- MySQL对CPU的占用率很高怎么处理
半桶水专家
mysqlmysql数据库
一、确认与定位确认整体CPU使用情况top-b-n1|head-n15观察MySQL(mysqld)进程所占的%CPU。如果是多核系统,关注总和以及单核是否满载。查看系统负载uptimeLoadAverage长期高于CPU核数,说明系统压力大。查看其它进程情况psaux--sort=-%cpu|head-n10确认是否仅MySQL占用高,或与其它进程有关。二、操作系统层面排查磁盘I/O瓶颈iost
- Spring Boot 中使用 Jackson 实现全局时间格式处理(支持多格式反序列化)
喵行星
Jacksping相关java
好的,以下是整理好的CSDN博文格式内容,适合你发布为一篇讲解Jackson全局时间配置的文章:SpringBoot中使用Jackson实现全局时间格式处理(支持多格式反序列化)在实际开发中,我们经常需要处理前后端交互中的时间字段。为了统一时间格式,通常会在字段上添加@JsonFormat注解,但如果项目中字段很多,这种方式会显得重复且难以维护。本文将介绍如何通过配置Jackson的全局时间格式,
- JVM调优实战 Day 14 :大数据处理中的JVM调优
在未来等你
JVM调优实战JVMJava性能优化调优虚拟机
【JVM调优实战Day14】大数据处理中的JVM调优文章标签jvm调优,大数据处理,Java性能优化,JVM参数配置,JVMGC调优,Java开发,大数据架构,Jvm实战文章简述在大数据处理场景中,Java应用通常面临内存占用高、GC频率频繁、堆内存不足等挑战。本文作为“JVM调优实战”系列的第14天,深入探讨了大数据处理中的JVM调优策略。文章从概念解析、技术原理、常见问题、诊断方法、调优策略到
- Python的内存管理
星辰灬
Pythonpythonpycharm
Python的内存管理在Python中,内存管理涉及到一个包含所有Python对象和数据结构的私有堆(heap)。这个私有堆的管理由内部的Python内存管理器(Pythonmemorymanager)保证。Python内存管理器有不同的组件来处理各种动态存储管理方面的问题,如共享、分割、预分配或缓存。内存管理机制动态内存分配:Python使用动态内存分配,这意味着它在运行时动态分配和管理内存,而
- 数据库必知必会系列:数据库连接池与连接管理
AI天才研究院
AI大模型企业级应用开发实战大数据人工智能语言模型JavaPython架构设计
作者:禅与计算机程序设计艺术1.简介随着互联网技术的飞速发展,网站流量呈爆炸性增长。对于后端服务器而言,处理大量请求通常需要依赖于高性能、高并发、海量数据等优秀的服务器硬件资源。这种情况下,如何有效地分配和管理服务器资源显得尤为重要。一个有效的方式就是采用数据库连接池技术。数据库连接池,是一种用于提升数据库访问性能的技术。它主要解决了如下两个方面的问题:由于不同线程或用户对同一个数据库的频繁访问,
- Spring Framework 5.0新增的WebClient
svygh123
编程springJava学习springlog4jjavaWebClient
SpringFramework5.0引入了WebClient,这是一个新的非阻塞、响应式Web客户端API,旨在为构建响应式微服务提供更好的支持。WebClient是基于ProjectReactor的响应式流API构建的,它可以高效地处理大量的并发请求,非常适合现代微服务架构。WebClient的主要特性响应式:WebClient基于非阻塞I/O,可以处理大量的并发请求而不会阻塞线程。类型安全:A
- MybatisPlus操作Oracle日期时间时TO_DATE处理
一朵梨花压海棠go
javamybatis开发语言
问题描述:在使用mybatis-plus构建查询语句对oracle数据进行查询时会报错,这是因为在ORACLE涉及日期查询时需要使用TO_DATE,所以,mybatis-plus构建查询语句时需要特别注意。mybatis-plus构建查询语句时,怎么处理oracle的日期呢?QueryWrapperwrapper=newQueryWrapper=TO_DATE({0},'yyyy-MM-dd')"
- SpringBoot返回文件让前端下载的几种方式
一朵梨花压海棠go
springboot前端后端
01背景在后端开发中,通常会有文件下载的需求,常用的解决方案有两种:不通过后端应用,直接使用nginx直接转发文件地址下载(适用于一些公开的文件,因为这里不需要授权)通过后端进行下载,同时进行一些业务处理本篇主要以方法2进行介绍,方法2的原理步骤如下:读取文件,得到文件的字节流将字节流写入到响应输出流中02一次性读取到内存,通过响应输出流输出到前端@GetMapping("/file/downlo
- python键盘输入转换为列表_Python键盘输入转换为列表的实例
云云众生w
python键盘输入转换为列表
Python键盘输入转换为列表的实例发布时间:2020-08-1912:58:38来源:脚本之家阅读:92作者:清泉影月Python输入字符串转列表是为了方便后续处理,这种操作在考试的时候比较多见。1.在Python3.0以后,键盘输入使用input函数eg1.>>>x=input>>>123123在命令行没有任何显示,输入123后直接赋值给x,并打印。eg2.>>>x=input("请输入...
- git 总结+场景应用
放逐者-保持本心,方可放逐
工具配置gitgit远程连接git标签git应用git打包迁移git版本控制git新手应用
文章目录概要(git)git冲突经验之谈git相关操作后续git具体应用回退到指定版本git校验忽略git版本标签管理git代码仓库迁移gitbundle后续git新手应用指南概要(git)一、Git简介Git是一个分布式版本控制系统,用于高效地处理从非常小到非常大的项目版本管理。它允许开发者跟踪文件的更改历史,方便团队协作开发,并且可以在不同分支上进行并行开发。二、基础指令连接(配置)gitco
- Swift concurrency 10 — AsyncStream 和 AsyncThrowingStream:用异步流优雅处理事件
技术拾光
SwiftConcurrencyswiftiosAsyncStream
SwiftConcurrency带来了现代化的异步编程体验。在处理异步事件流时,AsyncStream和AsyncThrowingStream提供了优雅的方式来消费和控制异步值序列。本文将全面讲解这两个API的用途、用法、底层机制和实战场景。什么是AsyncStream与AsyncThrowingStream?类型描述AsyncStream产生异步值序列,不支持抛出错误AsyncThrowingS
- JSON数据格式的序列化和反序列化jackson针对首字母小学的字段返回序列化后第2个大写字母也变成小写的问题处理
会飞的哈士奇
json
SpringBoot类属性”第二个字母大写“反序列化问题。key第二个字母大写会被转成小写,这个为jackson的bug。后台:StringpName;publicStringgetPName(){returnpName;}前台:{pname:'xxx'}解决方案:使用@JsonProperty("pName")注解类属性,指定错误反序列化后的属性名后台:StringpName;@JsonProp
- 使用虚幻引擎5(UE5)开发游戏的最低配置推荐
知1而N
UE5-游戏引擎虚幻5最佳实践ue5游戏
一、最低系统配置(可运行引擎)组件要求说明来源操作系统Windows1064位(版本20H2或更高)处理器4核CPU(如Inteli5-4590或AMDRyzen51600),主频≥3.0GHz内存8GBRAM(推荐32GB以上,复杂场景需64GB)显卡NVIDIAGTX970(4GB显存)或AMDRadeonR9290(4GB显存)存储100GBSSD(项目文件占用空间随复杂度增长)Direct
- 116-基于5VLX110T FPGA FMC接口功能验证6U CPCI平台
Anin蓝天(北京太速科技-陈)
fpga开发嵌入式硬件图像处理
一、板卡概述本板卡是Xilinx公司芯片V5系列芯片设计信号处理板卡。由一片Xilinx公司的XC5VLX110T-1FF1136/XC5VSX95T-1FF1136/XC5VFX70T-1FF1136芯片组成。FPGA接1片DDR2内存条2GB,32MBNorflash存储器,用于存储程序。外扩SATA、PCI、PCIexpress、千兆网络接口、SFP接口,自定义总线支持最大到266个IO。该
- Swift 小技巧:用单边区间优雅处理模糊范围
狼_夏天
SwiftTipsswift开发语言ios
进入正题之前先科普一下Swift区间的知识。Swift中的区间有两种类型:闭区间和半开区间。闭区间:用a...b表示,包含a和b。半开区间:用a..=0&&number=2.0&&score=3.5&&score<=5.0{print("好评")}else{print("评分超出范围")}3.用单边区间优雅处理letscore=4.2guard(0.0...5.0).contains(score)
- 「Go框架」gin框架是如何处理panic的?
-睡到自然醒~
golanggin开发语言后端springbootjava
本文我们介绍下recover在gin框架中的应用。首先,在golang中,如果在子协程中遇到了panic,那么主协程也会被终止。如下:packagemainimport("github.com/gin-gonic/gin")funcmain(){r:=gin.Default()//在子协程中引起panic,主协程也会退出gofunc(){panic("helloworld")}()//Listen
- Go语言中的defer关键字
在Go语言中,defer关键字是一个独特而强大的特性,它可以将代码块推迟到函数返回之前执行。这种机制可以用于资源的释放、错误处理、性能优化等多种场景。本文将详细介绍defer的用法和工作原理,并通过实际示例来展示其在不同情况下的应用。defer的基本用法在Go语言中,使用defer关键字可以将一个函数调用推迟到当前函数执行结束前执行。defer语句由关键字defer和一个函数在上述代码中,当函数d
- 折线图多数据处理
lifelalala
后端java
前言:skline1有年份和新申请单位数,skline2有年份和有效期内单位数,我想要把1和2的年份放在一起从小到大放,没有重复的,新申请单位数和有效期内单位数和年份的排列顺序一致实现://获取原始数据List>skLine1=bmzgConfidentQualifyManageService.getSkLine1();List>skLine2=bmzgConfidentQualifyManage
- 大模型学习 (Datawhale_Happy-LLM)笔记7: Encoder-Decoder PLM
lxltom
学习笔记languagemodel自然语言处理神经网络人工智能深度学习
大模型学习(Datawhale_Happy-LLM)笔记7:Encoder-DecoderPLM1.Encoder-Decoder架构概述1.1架构基础Encoder-DecoderPLM是基于原始Transformer架构的完整实现,它同时保留了编码器(Encoder)和解码器(Decoder)两个核心组件。这种设计使得模型能够兼具文本理解和生成的双重能力,特别适合处理序列到序列(Seq2Seq
- MyBatis SQL 执行过程原理分析(附源码) 代理层:Mapper 接口动态代理 路由层:MapperMethod 分发 核心引擎:SqlSession 执行
夜雨hiyeyu.com
mybatissql数据库数据库架构javaspringbootdb
MyBatisSQL执行过程原理分析(附源码)1.代理层:Mapper接口动态代理2.路由层:MapperMethod分发3.核心引擎:SqlSession执行4.执行器:Executor调度5.处理器层:StatementHandler执行6.结果映射:ResultSetHandler转换核心执行流程图关键设计亮点性能优化建议MyBatis的SQL执行过程可以分为6个核心阶段,我们将通过源码逐层
- 【Python基础】07 实战:批量视频压缩的实现
智算菩萨
python服务器开发语言
前言在数字化时代,视频内容已成为信息传播的主要载体。无论是个人用户还是企业,都面临着大量视频文件存储和传输的挑战。视频文件通常体积庞大,占用大量存储空间,同时在网络传输时也会消耗大量带宽。因此,一个高效、易用的视频压缩工具变得尤为重要。本文将详细介绍一个基于Python开发的批量视频压缩工具,该工具结合了现代图形界面设计和强大的FFmpeg视频处理能力,为用户提供了一站式的视频压缩解决方案。通过本
- # 国产高性能VPX6U模块:飞腾4核/8核处理器助力数据处理与通信
#产品概述今天为大家介绍一款高性能国产VPX6U模块——飞腾4核/8核VPX6U模块。这款产品采用国产飞腾处理器,具备强大的数据处理能力和丰富的接口配置,是军工、通信、存储等领域的理想选择。##核心特点###1.国产飞腾处理器,性能灵活可选-模块兼容FT2000-4或D2000-8两种处理器-用户可根据实际性能需求自由选择-完全国产化方案,安全可控###2.丰富接口配置,多功能应用-万兆以太网、千
- 【翻译】Part4: Texture samplers.
AtripthroughtheGraphicsPipeline2011,part4|Therygblog欢迎回来。上一部分讲的是顶点着色器,还大致介绍了通用的GPU着色器单元。总的来说,它们只是向量处理器,但它们可以访问一种在其他向量处理架构中不存在的资源:纹理采样器。纹理采样器是GPU流水线不可或缺的一部分,其复杂程度(以及趣味性!)足以单独写一篇文章来介绍,那接下来就开始吧。纹理状态在开始实际
- Spring Boot 过滤器 拦截器 监听器
后端springboot
Filter过滤器SpringBoot的过滤器用于对数据进行过滤处理。通过SpringBoot的过滤器,程序开发人员不仅可以对用户通过URL地址发送的请求进行过滤处理(例如,过滤一些错误的请求或者请求中的敏感词等),而且可以对服务器返回的数据进行过滤处理(例如,压缩响应信息等)。使用FilterRegistrationBean类实现过滤器实现过滤器类如果一个类实现了一个继承Filter接口的类,那
- Prompt Engineering 指南教程
班磊闯Andrea
PromptEngineering指南教程Prompt-Engineering-Guidedair-ai/Prompt-Engineering-Guide:是一个用于指导对话人工智能开发的文档。适合用于学习对话人工智能开发和自然语言处理。特点是提供了详细的指南和参考资料,涵盖了多种对话人工智能技术和算法,并且可以自定义学习路径和行为。项目地址:https://gitcode.com/gh_mirr
- 数据结构与算法 第一章 绪论
noruta
408#数据结构与算法数据结构
1.1.数据结构的基本概念数据:对计算机来说,能被计算机程序识别和处理的符号的集合。(比如二进制0和1)数据元素:数据的基本单位,通常作为一个整体进行考虑和处理。(比如一个学生的信息是一个数据元素)数据项:构成数据元素的最小单位。(学生的学号,姓名,班级构成一个学生信息)要根据实际的业务需求来确定什么是数据元素、什么是数据项。数据结构:相互之间存在一种或多种特定关系的数据元素的集合。比如汉字有左右
- 解线性方程组
qiuwanchi
package gaodai.matrix;
import java.util.ArrayList;
import java.util.List;
import java.util.Scanner;
public class Test {
public static void main(String[] args) {
Scanner scanner = new Sc
- 在mysql内部存储代码
annan211
性能mysql存储过程触发器
在mysql内部存储代码
在mysql内部存储代码,既有优点也有缺点,而且有人倡导有人反对。
先看优点:
1 她在服务器内部执行,离数据最近,另外在服务器上执行还可以节省带宽和网络延迟。
2 这是一种代码重用。可以方便的统一业务规则,保证某些行为的一致性,所以也可以提供一定的安全性。
3 可以简化代码的维护和版本更新。
4 可以帮助提升安全,比如提供更细
- Android使用Asynchronous Http Client完成登录保存cookie的问题
hotsunshine
android
Asynchronous Http Client是android中非常好的异步请求工具
除了异步之外还有很多封装比如json的处理,cookie的处理
引用
Persistent Cookie Storage with PersistentCookieStore
This library also includes a PersistentCookieStore whi
- java面试题
Array_06
java面试
java面试题
第一,谈谈final, finally, finalize的区别。
final-修饰符(关键字)如果一个类被声明为final,意味着它不能再派生出新的子类,不能作为父类被继承。因此一个类不能既被声明为 abstract的,又被声明为final的。将变量或方法声明为final,可以保证它们在使用中不被改变。被声明为final的变量必须在声明时给定初值,而在以后的引用中只能
- 网站加速
oloz
网站加速
前序:本人菜鸟,此文研究总结来源于互联网上的资料,大牛请勿喷!本人虚心学习,多指教.
1、减小网页体积的大小,尽量采用div+css模式,尽量避免复杂的页面结构,能简约就简约。
2、采用Gzip对网页进行压缩;
GZIP最早由Jean-loup Gailly和Mark Adler创建,用于UNⅨ系统的文件压缩。我们在Linux中经常会用到后缀为.gz
- 正确书写单例模式
随意而生
java 设计模式 单例
单例模式算是设计模式中最容易理解,也是最容易手写代码的模式了吧。但是其中的坑却不少,所以也常作为面试题来考。本文主要对几种单例写法的整理,并分析其优缺点。很多都是一些老生常谈的问题,但如果你不知道如何创建一个线程安全的单例,不知道什么是双检锁,那这篇文章可能会帮助到你。
懒汉式,线程不安全
当被问到要实现一个单例模式时,很多人的第一反应是写出如下的代码,包括教科书上也是这样
- 单例模式
香水浓
java
懒汉 调用getInstance方法时实例化
public class Singleton {
private static Singleton instance;
private Singleton() {}
public static synchronized Singleton getInstance() {
if(null == ins
- 安装Apache问题:系统找不到指定的文件 No installed service named "Apache2"
AdyZhang
apachehttp server
安装Apache问题:系统找不到指定的文件 No installed service named "Apache2"
每次到这一步都很小心防它的端口冲突问题,结果,特意留出来的80端口就是不能用,烦。
解决方法确保几处:
1、停止IIS启动
2、把端口80改成其它 (譬如90,800,,,什么数字都好)
3、防火墙(关掉试试)
在运行处输入 cmd 回车,转到apa
- 如何在android 文件选择器中选择多个图片或者视频?
aijuans
android
我的android app有这样的需求,在进行照片和视频上传的时候,需要一次性的从照片/视频库选择多条进行上传
但是android原生态的sdk中,只能一个一个的进行选择和上传。
我想知道是否有其他的android上传库可以解决这个问题,提供一个多选的功能,可以使checkbox之类的,一次选择多个 处理方法
官方的图片选择器(但是不支持所有版本的androi,只支持API Level
- mysql中查询生日提醒的日期相关的sql
baalwolf
mysql
SELECT sysid,user_name,birthday,listid,userhead_50,CONCAT(YEAR(CURDATE()),DATE_FORMAT(birthday,'-%m-%d')),CURDATE(), dayofyear( CONCAT(YEAR(CURDATE()),DATE_FORMAT(birthday,'-%m-%d')))-dayofyear(
- MongoDB索引文件破坏后导致查询错误的问题
BigBird2012
mongodb
问题描述:
MongoDB在非正常情况下关闭时,可能会导致索引文件破坏,造成数据在更新时没有反映到索引上。
解决方案:
使用脚本,重建MongoDB所有表的索引。
var names = db.getCollectionNames();
for( var i in names ){
var name = names[i];
print(name);
- Javascript Promise
bijian1013
JavaScriptPromise
Parse JavaScript SDK现在提供了支持大多数异步方法的兼容jquery的Promises模式,那么这意味着什么呢,读完下文你就了解了。
一.认识Promises
“Promises”代表着在javascript程序里下一个伟大的范式,但是理解他们为什么如此伟大不是件简
- [Zookeeper学习笔记九]Zookeeper源代码分析之Zookeeper构造过程
bit1129
zookeeper
Zookeeper重载了几个构造函数,其中构造者可以提供参数最多,可定制性最多的构造函数是
public ZooKeeper(String connectString, int sessionTimeout, Watcher watcher, long sessionId, byte[] sessionPasswd, boolea
- 【Java命令三】jstack
bit1129
jstack
jstack是用于获得当前运行的Java程序所有的线程的运行情况(thread dump),不同于jmap用于获得memory dump
[hadoop@hadoop sbin]$ jstack
Usage:
jstack [-l] <pid>
(to connect to running process)
jstack -F
- jboss 5.1启停脚本 动静分离部署
ronin47
以前启动jboss,往各种xml配置文件,现只要运行一句脚本即可。start nohup sh /**/run.sh -c servicename -b ip -g clustername -u broatcast jboss.messaging.ServerPeerID=int -Djboss.service.binding.set=p
- UI之如何打磨设计能力?
brotherlamp
UIui教程ui自学ui资料ui视频
在越来越拥挤的初创企业世界里,视觉设计的重要性往往可以与杀手级用户体验比肩。在许多情况下,尤其对于 Web 初创企业而言,这两者都是不可或缺的。前不久我们在《右脑革命:别学编程了,学艺术吧》中也曾发出过重视设计的呼吁。如何才能提高初创企业的设计能力呢?以下是 9 位创始人的体会。
1.找到自己的方式
如果你是设计师,要想提高技能可以去设计博客和展示好设计的网站如D-lists或
- 三色旗算法
bylijinnan
java算法
import java.util.Arrays;
/**
问题:
假设有一条绳子,上面有红、白、蓝三种颜色的旗子,起初绳子上的旗子颜色并没有顺序,
您希望将之分类,并排列为蓝、白、红的顺序,要如何移动次数才会最少,注意您只能在绳
子上进行这个动作,而且一次只能调换两个旗子。
网上的解法大多类似:
在一条绳子上移动,在程式中也就意味只能使用一个阵列,而不使用其它的阵列来
- 警告:No configuration found for the specified action: \'s
chiangfai
configuration
1.index.jsp页面form标签未指定namespace属性。
<!--index.jsp代码-->
<%@taglib prefix="s" uri="/struts-tags"%>
...
<s:form action="submit" method="post"&g
- redis -- hash_max_zipmap_entries设置过大有问题
chenchao051
redishash
使用redis时为了使用hash追求更高的内存使用率,我们一般都用hash结构,并且有时候会把hash_max_zipmap_entries这个值设置的很大,很多资料也推荐设置到1000,默认设置为了512,但是这里有个坑
#define ZIPMAP_BIGLEN 254
#define ZIPMAP_END 255
/* Return th
- select into outfile access deny问题
daizj
mysqltxt导出数据到文件
本文转自:http://hatemysql.com/2010/06/29/select-into-outfile-access-deny%E9%97%AE%E9%A2%98/
为应用建立了rnd的帐号,专门为他们查询线上数据库用的,当然,只有他们上了生产网络以后才能连上数据库,安全方面我们还是很注意的,呵呵。
授权的语句如下:
grant select on armory.* to rn
- phpexcel导出excel表简单入门示例
dcj3sjt126com
PHPExcelphpexcel
<?php
error_reporting(E_ALL);
ini_set('display_errors', TRUE);
ini_set('display_startup_errors', TRUE);
if (PHP_SAPI == 'cli')
die('This example should only be run from a Web Brows
- 美国电影超短200句
dcj3sjt126com
电影
1. I see. 我明白了。2. I quit! 我不干了!3. Let go! 放手!4. Me too. 我也是。5. My god! 天哪!6. No way! 不行!7. Come on. 来吧(赶快)8. Hold on. 等一等。9. I agree。 我同意。10. Not bad. 还不错。11. Not yet. 还没。12. See you. 再见。13. Shut up!
- Java访问远程服务
dyy_gusi
httpclientwebservicegetpost
随着webService的崛起,我们开始中会越来越多的使用到访问远程webService服务。当然对于不同的webService框架一般都有自己的client包供使用,但是如果使用webService框架自己的client包,那么必然需要在自己的代码中引入它的包,如果同时调运了多个不同框架的webService,那么就需要同时引入多个不同的clien
- Maven的settings.xml配置
geeksun
settings.xml
settings.xml是Maven的配置文件,下面解释一下其中的配置含义:
settings.xml存在于两个地方:
1.安装的地方:$M2_HOME/conf/settings.xml
2.用户的目录:${user.home}/.m2/settings.xml
前者又被叫做全局配置,后者被称为用户配置。如果两者都存在,它们的内容将被合并,并且用户范围的settings.xml优先。
- ubuntu的init与系统服务设置
hongtoushizi
ubuntu
转载自:
http://iysm.net/?p=178 init
Init是位于/sbin/init的一个程序,它是在linux下,在系统启动过程中,初始化所有的设备驱动程序和数据结构等之后,由内核启动的一个用户级程序,并由此init程序进而完成系统的启动过程。
ubuntu与传统的linux略有不同,使用upstart完成系统的启动,但表面上仍维持init程序的形式。
运行
- 跟我学Nginx+Lua开发目录贴
jinnianshilongnian
nginxlua
使用Nginx+Lua开发近一年的时间,学习和实践了一些Nginx+Lua开发的架构,为了让更多人使用Nginx+Lua架构开发,利用春节期间总结了一份基本的学习教程,希望对大家有用。也欢迎谈探讨学习一些经验。
目录
第一章 安装Nginx+Lua开发环境
第二章 Nginx+Lua开发入门
第三章 Redis/SSDB+Twemproxy安装与使用
第四章 L
- php位运算符注意事项
home198979
位运算PHP&
$a = $b = $c = 0;
$a & $b = 1;
$b | $c = 1
问a,b,c最终为多少?
当看到这题时,我犯了一个低级错误,误 以为位运算符会改变变量的值。所以得出结果是1 1 0
但是位运算符是不会改变变量的值的,例如:
$a=1;$b=2;
$a&$b;
这样a,b的值不会有任何改变
- Linux shell数组建立和使用技巧
pda158
linux
1.数组定义 [chengmo@centos5 ~]$ a=(1 2 3 4 5) [chengmo@centos5 ~]$ echo $a 1 一对括号表示是数组,数组元素用“空格”符号分割开。
2.数组读取与赋值 得到长度: [chengmo@centos5 ~]$ echo ${#a[@]} 5 用${#数组名[@或
- hotspot源码(JDK7)
ol_beta
javaHotSpotjvm
源码结构图,方便理解:
├─agent Serviceab
- Oracle基本事务和ForAll执行批量DML练习
vipbooks
oraclesql
基本事务的使用:
从账户一的余额中转100到账户二的余额中去,如果账户二不存在或账户一中的余额不足100则整笔交易回滚
select * from account;
-- 创建一张账户表
create table account(
-- 账户ID
id number(3) not null,
-- 账户名称
nam
![]() , S[i..j] and S[j+1..k] are all palindrome strings. It's easy for her. She wants to know the sum of i*k of all required three tuples. She can't solve it. So can you help her? The answer may be very large, please output the answer mod 1000000007.
, S[i..j] and S[j+1..k] are all palindrome strings. It's easy for her. She wants to know the sum of i*k of all required three tuples. She can't solve it. So can you help her? The answer may be very large, please output the answer mod 1000000007.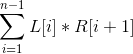 。接下来要求的就是L【i】和R【i】。以L[i]为例,一个中心为i半径为l的回文串对属于【i−l+1,i】的
L
[
i
]
值都是有贡献的,且对于
。接下来要求的就是L【i】和R【i】。以L[i]为例,一个中心为i半径为l的回文串对属于【i−l+1,i】的
L
[
i
]
值都是有贡献的,且对于 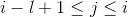 的贡献为 i +(i - j), 那么,
的贡献为 i +(i - j), 那么,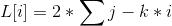 。
。
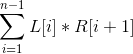 。接下来要求的就是L【i】和R【i】。以L[i]为例,一个中心为i半径为l的回文串对属于【i−l+1,i】的
L
[
i
]
值都是有贡献的,且对于
。接下来要求的就是L【i】和R【i】。以L[i]为例,一个中心为i半径为l的回文串对属于【i−l+1,i】的
L
[
i
]
值都是有贡献的,且对于