泰勒公式、等价无穷小代换式总结
泰勒公式是极限计算的重要工具,常用重要函数的泰勒展开式有:
- s i n x = x − x 3 3 ! + x 5 5 ! − x 7 7 ! + o ( x 7 ) \bm{sinx=x-\frac{x^3}{3!}+\frac{x^5}{5!}-\frac{x^7}{7!}+o(x^7)} sinx=x−3!x3+5!x5−7!x7+o(x7)
s i n x sinx sinx是奇函数,展开式也绝不会有偶函数的形式,其中o(·)为佩亚诺余项;
一般用到余项为3次方即可 s i n x = x − x 3 3 ! + o ( x 3 ) sinx=x-\frac{x^3}{3!}+o(x^3) sinx=x−3!x3+o(x3)
- c o s x = 1 − x 2 2 ! + x 4 4 ! − x 6 6 ! + o ( x 6 ) \bm{cosx=1-\frac{x^2}{2!}+\frac{x^4}{4!}-\frac{x^6}{6!}+o(x^6)} cosx=1−2!x2+4!x4−6!x6+o(x6)
c o s x cosx cosx是偶函数,展开式也绝不会有奇函数的形式.
-
a r c s i n x = x + x 3 3 ! + o ( x 3 ) \bm{arcsinx=x+\frac{x^3}{3!}+o(x^3)} arcsinx=x+3!x3+o(x3)
-
t a n x = x + x 3 3 + o ( x 3 ) \bm{tanx=x+\frac{x^3}{3}+o(x^3)} tanx=x+3x3+o(x3)
-
a r c t a n x = x − x 3 3 + o ( x 3 ) \bm{arctanx=x-\frac{x^3}{3}+o(x^3)} arctanx=x−3x3+o(x3)
-
l n ( 1 + x ) = x − x 2 2 + x 3 3 + o ( x 3 ) \bm{ln(1+x)=x-\frac{x^2}{2}+\frac{x^3}{3}+o(x^3)} ln(1+x)=x−2x2+3x3+o(x3)
-
e x = 1 + x + x 2 2 ! + x 3 3 ! + o ( x 3 ) \bm{e^x=1+x+\frac{x^2}{2!}+\frac{x^3}{3!}+o(x^3)} ex=1+x+2!x2+3!x3+o(x3)
-
( 1 + x ) α = 1 + α x + α ( α − 1 ) 2 ! x 2 + o ( x 2 ) \bm{(1+x)^\alpha=1+\alpha x +\frac{\alpha (\alpha -1)}{2!}x^2+o(x^2)} (1+x)α=1+αx+2!α(α−1)x2+o(x2)
由泰勒展开式作差,可得到一组“差函数”的等价无穷小代换式,
当 x → 0 x \to 0 x→0时:
- x − s i n x ∼ x 3 3 ! = x 3 6 \bm{x-sinx \sim \frac{x^3}{3!}=\frac{x^3}{6}} x−sinx∼3!x3=6x3
其中 x x x可任意替换, x − s i n ( g ( x ) ) ∼ ( g ( x ) ) 3 6 x-sin(g(x)) \sim \frac{(g(x))^3}{6} x−sin(g(x))∼6(g(x))3,写成狗也可以, 狗 − s i n ( 狗 ) ∼ ( 狗 ) 3 6 狗-sin(狗) \sim \frac{(狗)^3}{6} 狗−sin(狗)∼6(狗)3
-
a r c s i n x − x ∼ x 3 6 \bm{arcsinx-x \sim \frac{x^3}{6}} arcsinx−x∼6x3
-
t a n x − x ∼ x 3 3 \bm{tanx-x \sim \frac{x^3}{3}} tanx−x∼3x3
-
x − a r c t a n x ∼ x 3 3 \bm{x-arctanx \sim \frac{x^3}{3}} x−arctanx∼3x3
常用等价无穷小还包括:
s i n x ∼ x , t a n x ∼ x , a r c s i n x ∼ x , a r c t a n x ∼ x , l n ( 1 + x ) ∼ x , e x − 1 ∼ x , a x − 1 ∼ x l n a , 1 − c o s x ∼ x 2 2 , ( 1 + x ) α − 1 ∼ α x sinx \sim x,tanx \sim x,arcsinx \sim x,arctanx\sim x,ln(1+x)\sim x,e^x-1\sim x,a^x-1\sim xlna,1-cosx\sim \frac{x^2}{2},(1+x)^\alpha -1\sim \alpha x sinx∼x,tanx∼x,arcsinx∼x,arctanx∼x,ln(1+x)∼x,ex−1∼x,ax−1∼xlna,1−cosx∼2x2,(1+x)α−1∼αx
其中 x x x也可任意替换,灵活使用
例题:
- A B \frac{A}{B} BA型
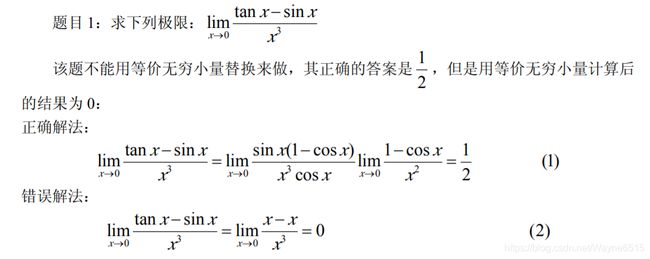
计算 lim x → 0 x − s i n x x 3 \lim\limits_{x \to 0}\frac{x-sinx}{x^3} x→0limx3x−sinx,这种类型要尽可能避免使用洛必达法则,考点往往是利用泰勒公式解决.
将分子代换为与上下同阶的形式: lim x → 0 x 3 6 x 3 = 1 6 \lim\limits_{x \to 0}\frac{\frac{x^3}{6}}{x^3}=\frac{1}{6} x→0limx36x3=61.
- A − B A-B A−B型
x → 0 x \to 0 x→0时, c o s x − e − x 2 x cosx-e^{-\frac{x^2}{x}} cosx−e−xx2与 a x b ax^b axb为等价无穷小,计算a,b,利用泰勒公式,将 A 和 B A和B A和B分别展开到他们的系数不相等的 x x x的最低幂次.
c o s x − e − x 2 x = [ 1 − x 2 2 ! + x 4 4 ! + o ( x 4 ) ] − [ 1 − x 2 2 + x 4 4 2 ! + o ( x 4 ) ] ∼ − x 4 12 cosx-e^{-\frac{x^2}{x}}=[1-\frac{x^2}{2!}+\frac{x^4}{4!}+o(x^4)]-[1-\frac{x^2}{2}+\frac{\frac{x^4}{4}}{2!}+o(x^4)]\sim-\frac{x^4}{12} cosx−e−xx2=[1−2!x2+4!x4+o(x4)]−[1−2x2+2!4x4+o(x4)]∼−12x4
故a= − 1 12 \frac{-1}{12} 12−1,b=4.
但须注意,有些情况下不能随便用等价无穷小代换:

注意:此题中提到的等价替换表达式其实为 lim x → 0 s i n f ( x ) x ≠ 1 \lim\limits_{x \to 0}\frac{sinf(x)}{x}\neq 1 x→0limxsinf(x)=1,其中x是自变量, x x x在趋于0的过程中,当 x n = 1 n π , f ( x ) x_n=\frac{1}{n\pi},f(x) xn=nπ1,f(x)会=0,无穷小量为0,不能使用等价无穷小;但若表达式写作
lim 狗 → 0 s i n 狗 狗 = 1 \lim\limits_{狗 \to 0}\frac{sin狗}{狗}=1 狗→0lim狗sin狗=1(此题中并未用到),
狗趋近于0,但不会取到0,三者都为狗,所以不会存在无穷小量为0的情况,因此在此前提下将 s i n 狗 ∼ 狗 sin狗\sim 狗 sin狗∼狗放心地广义化,对比上一个表达式,可将此种类型的题理解为 s i n sin sin狗不等价狗的案例。
总结:
实际计算数列极限的问题更为繁琐,还包括用定义法证明数列极限,并用性质(唯一性、有界性、保号性)求极限,以及运用极限的四则运算规则、夹逼准则、单调有界限准则(单调有界必有极限)、放缩后裂项相消等方法求极限,学会运用泰勒公式以及正确前提下使用等价无穷小替换也极为重要。