- 源码地址请点击此处
链表也是一种常见的数据结构,从数据结构类型上区分,链表属于存储结构的一种:链式存储结构。
和顺序存储结构一样,链式存储结构也可以用来作为实现其他数据结构的基础,比如前面介绍的线性表、栈、队列等。
顺序存储结构和链式存储结构的区别
使用顺序存储结构存放数据时,使用的是内存空间中连续的内存,如下图所示:
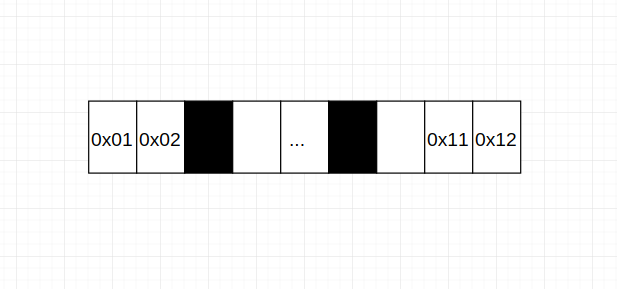
使用顺序存储结构时,数据需要存放在连续的内存空间中。这种存储结构的优点在于读取速度很快,缺点在于无法有效利用内存空间。怎么理解呢?举个例子,如果只使用顺序存储结构,在程序的运行过程中,会产生很多的临时变量,这些变量在不用时会被垃圾回收,当变量被回收后,内存中就出现了不连续的情况,也可以叫做内存空洞。如下图所示,黑色区域表示内存空洞:
随着程序的运行,这样的内存空洞会越来越多,此时如果程序中需要用到较大的内存空间,而剩余的连续内存不够的情况下,将无法成功分配内存,但内存中又有很多的内存空洞,却无法得到有效利用。
要解决这个问题,有两个解决方案:
- 将内存空洞后面的数据往前移
第一种方式就是每隔一段时间,将内存空洞后面的数据向前移,以达到“挤压”内存空洞的目的。这种方式的缺点在于性能浪费严重。 -
使用链式存储结构
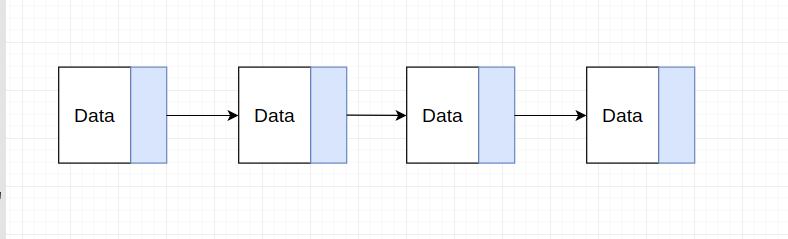
第二种方式就是使用链式存储结构:在某些内存空洞中存放一部分数据,再存放下一块可用内存空洞的地址,通过地址把这些可用的内存空洞连接起来,实现一个链式的结构,这就是链表。下面是链表的基本结构:
上图展示了链表的基本结构,对于链表,其中的每个元素我们称之为节点。可见每个节点至少需要包含两部分内容:
- 数据区:用来存放数据
- 地址区:用来存放下一个节点的地址
链表的分类
通常我们说的链表也叫作单向链表,此外,链表还有两个常用的衍生结构:双向链表和循环链表。本文主要介绍单向链表的实现,双向链表和循环链表在后文介绍。
链表的代码实现
下面是链表的代码实现,首先来定义一个 ISingleNode 接口和 SingleNode 类,用来实现链表中的节点。
节点类和接口的代码实现
interface ISingleNode{
// 数据区
data:T;
// 地址区
next:ISingleNode;
}
class SingleNode implements ISingleNode{
data:T = null;
next:ISingleNode = null;
constructor(data?:T){
this.data = data;
}
}
链表类的接口和代码实现
首先定义 ISingleLinkedList 接口,规范链表类中的基本操作方法:
interface ISingleLinkedList{
// 获取链表的长度
size():number;
// 获取链表头
head():ISingleNode;
// 增加节点
append(item:T):ISingleNode;
// 删除节点
remove(item:T):void;
// 根据位置删除
removeAt(pos:number):void;
// 插入节点
insert(newItem:T,oldItem:T):ISingleNode;
// 在具体的位置插入
insertAt(newItem:T,pos:number):ISingleNode;
// 清空链表
clear():void;
// 判断链表是否为空
isEmpty():boolean;
// 查找节点和其前驱
find(item:T):{
previous:ISingleNode,
current:ISingleNode,
currentPos:number,
previousPos:number
};
// 根据位置查找节点和其前驱
findAt(pos:number):{
previous:ISingleNode,
current:ISingleNode,
currentPos:number,
previousPos:number
};
// 获取链表中的元素
toString():ISingleNode[];
}
定义链表类 SingleLinkedList:
class SingleLinkedList implements ISingleLinkedList{
private _size:number = 0;
private _head:ISingleNode = new SingleNode();
}
下面逐个讲解链表类中的几个关键方法。
- 实现
find()方法
首先需要实现find()方法,该方法用来从链表中查找节点,以供后面的添加和删除方法使用。
find(item:T):{
previous:ISingleNode,
current:ISingleNode,
currentPos:number,
previousPos:number,
}{
if(!item){
throw new Error("参数错误!")
}
let
previous:ISingleNode = null,
current:ISingleNode = this._head,
index:number = -1;
while(current){
// 更新索引值
index++;
// 判断当前节点中的数据和传入的是否匹配
if(current.data === item){
break;
}
// 将 current 赋值给 previous
// 将 current.next 赋值给 current
// 在下一次迭代中使用
previous = current;
current = current.next;
}
// HACK 在前面的循环中找不到对于的元素时,会获取到尾节点
// 这里进行一次二次验证
if(current.data !== item){
index = -1;
}
// 处理未找到的情况
if(index === -1){
return{
previous:null,
current:null,
previousPos:-1,
currentPos:-1
}
}
return{
previous,
current,
currentPos:index,
// 前驱的位置在当前节点之前
previousPos:index - 1
}
}
这个方法(和配套的 findAt())是链表类中核心方法,再进行链表的添加、插入或删除操作时,首先需要查找链表中的某个节点和其前驱节点,查找总是从链表的头部开始的。
- 实现
append()方法
该方法用来向链表中添加节点,在添加时有两种情况:
- 链表为空时,将头节点替换为新节点
- 链表不为空时,将尾结点替换为新节点
append(item:T):ISingleNode{
const newNode = new SingleNode(item);
// 链表中没有节点
if(!this._size){
this._head = newNode;
}else{
const {current} = this.findAt(this._size - 1);
current.next = newNode
}
this._size++;
return newNode;
}
3.实现 insert() 方法
该方法用来向链表中插入元素,也有两种情况:
- 插入的位置是头节点,将头节点替换为新节点
- 插入的位置是其他节点,将原始节点的位置插入新节点,同时将原始节点向后移,这时就需要用到原始节点的前驱节点
insert(newItem:T,oldItem:T):ISingleNode{
// 创建新节点
const newNode = new SingleNode(newItem);
// 查找旧节点及其前驱节点
const {current,previous} = this.find(oldItem);
// 没有查找到旧节点,直接返回
if(!current) return null;
// 当 previous 为 null 时,说明是头节点
if(!previous){
newNode.next = current;
this._head = newNode;
}else{
// 将新建节点的 next 指向旧节点
newNode.next = current;
// 将旧节点前驱的 next 指向新建的节点
previous.next = newNode;
}
this._size++;
return newNode;
}
- 实现
remove()方法
该方法用来从链表中移除节点。
remove(item:T):void{
// 获取当前节点和其的前驱
let { current,previous } = this.find(item);
// 还没有添加节点的情况
if(!current) return;
// 没有前驱节点,说明是头节点
if(!previous){
this._head = current.next;
}else{
// 将当前节点的前驱的 next 指向当前节点的后继
previous.next = current.next;
}
// 移除当前节点
current = null;
// 更新链表长度
this._size--;
}
下面是 SingleLinkedList 类的完整代码实现:
class SingleLinkedList implements ISingleLinkedList{
private _size:number = 0;
private _head:ISingleNode = new SingleNode();
size():number{
return this._size;
}
head():ISingleNode{
return this._head;
}
clear():void{
this._head = null;
this._head = new SingleNode();
this._size = 0;
}
isEmpty():boolean{
return !this._size;
}
append(item:T):ISingleNode{
const newNode = new SingleNode(item);
// 链表中没有节点
if(!this._size){
this._head = newNode;
}else{
const {current} = this.findAt(this._size - 1);
current.next = newNode
}
this._size++;
return newNode;
}
find(item:T):{
previous:ISingleNode,
current:ISingleNode,
currentPos:number,
previousPos:number,
}{
if(!item){
throw new Error("参数错误!")
}
let
previous:ISingleNode = null,
current:ISingleNode = this._head,
index:number = -1;
while(current){
// 更新索引值
index++;
// 判断当前节点中的数据和传入的是否匹配
if(current.data === item){
break;
}
// 将 current 赋值给 previous
// 将 current.next 赋值给 current
// 在下一次迭代中使用
previous = current;
current = current.next;
}
// HACK 在前面的循环中找不到对于的元素时,会获取到尾节点
// 这里进行一次二次验证
if(current.data !== item){
index = -1;
}
// 处理未找到的情况
if(index === -1){
return{
previous:null,
current:null,
previousPos:-1,
currentPos:-1
}
}
return{
previous,
current,
currentPos:index,
// 前驱的位置在当前节点之前
previousPos:index - 1
}
}
findAt(pos:number):{
previous:ISingleNode,
current:ISingleNode,
currentPos:number,
previousPos:number,
}{
let
previous:ISingleNode = null,
current:ISingleNode = this._head,
index:number = -1;
if(pos < 0 || pos > this._size - 1){
throw new Error("索引越界!");
}
while(current){
index++;
if(index === pos){
break;
}
previous = current;
current = current.next;
}
// 处理未找到的情况
if(index === -1){
return{
previous:null,
current:null,
previousPos:-1,
currentPos:-1
}
}
return{
previous,
current,
currentPos:index,
// 前驱的位置在当前节点之前
previousPos:index - 1
}
}
remove(item:T):void{
// 获取当前节点和其的前驱
let { current,previous } = this.find(item);
// 还没有添加节点的情况
if(!current) return;
// 没有前驱节点,说明是头节点
if(!previous){
this._head = current.next;
}else{
// 将当前节点的前驱的 next 指向当前节点的后继
previous.next = current.next;
}
// 移除当前节点
current = null;
// 更新链表长度
this._size--;
}
removeAt(pos:number):void{
// 获取当前节点和其的前驱
let { current,previous } = this.findAt(pos);
// 还没有添加节点的情况
if(!current) return;
// 没有前驱节点,说明是头节点
if(!previous){
this._head = current.next;
}else{
// 将当前节点的前驱的 next 指向当前节点的后继
previous.next = current.next;
}
// 移除当前节点
current = null;
// 更新链表长度
this._size--;
}
insert(newItem:T,oldItem:T):ISingleNode{
// 创建新节点
const newNode = new SingleNode(newItem);
// 查找旧节点及其前驱节点
const {current,previous} = this.find(oldItem);
// 没有查找到旧节点,直接返回
if(!current) return null;
// 当 previous 为 null 时,说明是头节点
if(!previous){
newNode.next = current;
this._head = newNode;
}else{
// 将新建节点的 next 指向旧节点
newNode.next = current;
// 将旧节点前驱的 next 指向新建的节点
previous.next = newNode;
}
this._size++;
return newNode;
}
insertAt(newItem:T,pos:number):ISingleNode{
// 创建新节点
const newNode = new SingleNode(newItem);
// 查找旧节点及其前驱节点
const {current,previous} = this.findAt(pos);
if(!current) return null;
// 当 previous 为 null 时,说明是头节点
if(!previous){
newNode.next = current;
this._head = newNode;
}else{
// 将新建节点的 next 指向旧节点
newNode.next = current;
// 将旧节点前驱的 next 指向新建的节点
previous.next = newNode;
}
this._size++;
return newNode;
}
toString():ISingleNode[]{
const tmp:ISingleNode[] = [];
let current:ISingleNode = this._head;
while(current){
tmp.push(current);
current = current.next;
}
return tmp;
}
}
总结
链表在实现上比前面几个数据结构要复杂一点,这是因为在实现链表的添加、插入和删除操作时还需要修复节点地址区的指向问题。通常,链表操作需要考虑两种情况:
- 在头节点进行添加、插入和删除操作
- 在其他位置进行添加、插入和删除操作
当在头节点进行操作时,不需要使用头节点的前驱节点,因为链表的头节点没有前驱节点,只需要修正头节点的 next 属性,或者使用新的节点替换头节点。
在其他位置进行操作时,需要使用到该节点的前驱节点,并修正前驱节点的 next 属性。同时,如果是删除操作,还需要使用到该节点的后继节点,在修正前驱节点和后继节点的地址区指向后,还需要将被删除的节点置为 null,防止内存泄露。
完。