形式语言与自动机 04 Regular Expressions
Regular Expressions
Definition
RE’ s: Introduction
- Regular expressions describe languages by an algebra
- They describe exactly the regular languages
- If E is a regular expression, then L(E) is the language it defines
- We’ll describe RE’ s and their languages recursively
Operations on Languages
Union
{ 01 , 111 , 10 } ∪ { 00 , 01 } = { 01 , 111 , 10 , 00 } \{01,111,10\} \cup \{00,01\} = \{01,111,10,00\} {01,111,10}∪{00,01}={01,111,10,00}
Concatenation
- The concatenation of languages L L L and M M M is denoted L M LM LM
- It contains every string w x wx wx such that w w w is in L L L and x x x is in M M M
{ 01 , 111 , 10 } { 00 , 01 } = { 0100 , 0101 , 11100 , 11101 , 1000 , 1001 } \{ 01,111,10\} \{00,01\} = \{0100,0101,11100,11101,1000,1001\} {01,111,10}{00,01}={0100,0101,11100,11101,1000,1001}
Kleene Star
- L ∗ = { ϵ } ∪ L ∪ L L ∪ L L L . . . } L^* = \{\epsilon \} \cup L \cup LL \cup LLL ...\} L∗={ϵ}∪L∪LL∪LLL...}
{ 0 , 10 } ∗ = ϵ ∪ { 0 , 10 , 00 , 010 , 100 , 1010 , . . . } \{0,10\}^* = \epsilon \cup \{0,10,00,010,100,1010,...\} {0,10}∗=ϵ∪{0,10,00,010,100,1010,...}
RE’ s Definition
-
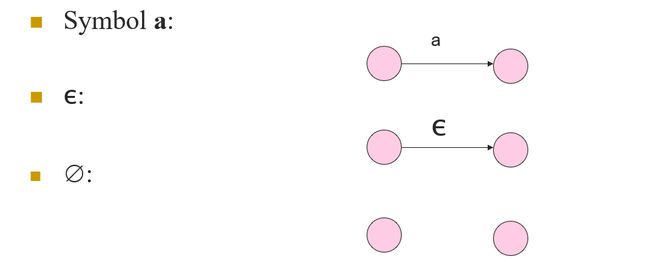
Basis 1: If a a a is any symbol. then a a a is a RE, and L( a a a) = {a}
- Note: {a} is the language containing one string, and that string is of length 1
-
Basic 2: ϵ \epsilon ϵ is a RE, and L( ϵ \epsilon ϵ) = { ϵ \epsilon ϵ}
-
Basic 3: ∅ \emptyset ∅ is a RE, and L( ∅ \emptyset ∅) = ∅ \emptyset ∅
-
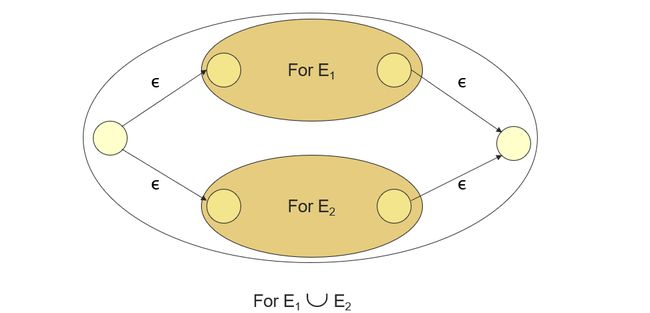
Induction 1: If E 1 E_1 E1 and E 2 E_2 E2 are regular expressions, then E 1 + E 2 E_1 + E_2 E1+E2 is a regular expression, and L( E 1 + E 2 E_1+E_2 E1+E2) = L( E 1 E_1 E1) + L( E 2 E_2 E2)
-
Induction 2: If E 1 E_1 E1 and E 2 E_2 E2 are regular expressions, then E 1 E 2 E_1E_2 E1E2 is a regular expression, and L( E 1 E 2 E_1E_2 E1E2) = L( E 1 E_1 E1)L( E 2 E_2 E2)
-
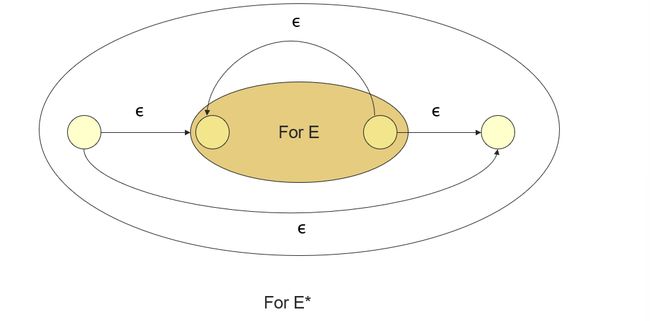
Induction 3: If E E E is a R E RE RE, then E ∗ E^* E∗ is a R E RE RE, and L( E ∗ E^* E∗) = (L( E E E)) ∗ ^* ∗
Precedence of Operators
- L(01) = {01}
- L(01+0) = {01,0}
- L(0(1+0)) = {01,00}
- L( 0 ∗ 0^* 0∗) = { ϵ , 0 , 00 , 000 , . . . \epsilon,0,00,000,... ϵ,0,00,000,...}
- L((0+10) ∗ ^* ∗ ( ϵ \epsilon ϵ + 1)) = all strings of 0’ s and 1’ s without two consecutive 1’ s
Equivalence to Finite Automata
We need to show that for every RE, there is a finite automaton that accepts
And for every finite automaton, there is a RE defining its language
Converting a RE to an ϵ \epsilon ϵ-NFA
- Proof is an induction on the number of operators(+,concatenation,*) in RE
Basic
Union
Concatenation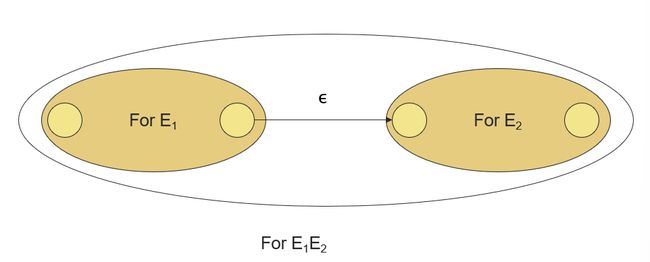
Closure
DFA to RE
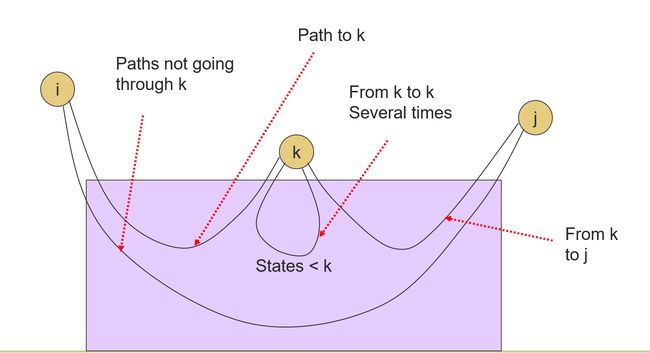
k-Paths
- A k-path is a path through the graph of the DFA that goes through no state numbered higher than k
- n-paths are unrestricted
RE is the union of RE’ s for the n-paths from the start state to each final state
- **Basis: ** k=0; only arcs or a node by itself
- **Induction: ** construct RE’ s for paths allowed to pass through state k from paths allowed only up to k-1
k-Path Induction
- Let R i j k R_{ij}^k Rijk be the regular expression for the set of labels of k-paths from state i to state j
- Basis: k=0. R i j 0 R_{ij}^0 Rij0 = sum of labels of arc from i to j
- ∅ \emptyset ∅ if no such arc
- But add ∈ \in ∈ if i=j
k-Path Inductive Case
R i j k = R i j k − 1 + R i k k − 1 ( R k k k − 1 ) ∗ R k j k − 1 R_{ij}^k = R_{ij}^{k-1} + R_{ik}^{k-1}(R_{kk}^{k-1})^*R_{kj}^{k-1} Rijk=Rijk−1+Rikk−1(Rkkk−1)∗Rkjk−1
Final Step
- The RE with the same language as the DFA is the sum (union) of R i j n , R_{ij}^n, Rijn, where:
- n is the number of states; i.e., paths are unconstrained
- i is the start state
- j is one of the final states
Summary
Each of the three types of automata (DFA,NFA, ϵ \epsilon ϵ-NFA) we discussed, and regular expressions as well, define exactly the same set of languages: the regular languages
Algebraic Laws for RE’ s
Identities and Annihilators
- ∅ \empty ∅ is the identity for +
- R + ∅ \empty ∅ = R
- ϵ \epsilon ϵ is the identity for concatenation
- ϵ R = R ϵ = R \epsilon R = R \epsilon = R ϵR=Rϵ=R
- ∅ \emptyset ∅ is the annihilator for concatenation
- ∅ R = R ∅ = ∅ \emptyset R = R \emptyset = \emptyset ∅R=R∅=∅
Decision Properties of Regular Languages
General Discussion of “Properties”
Properties of Language Classes
- A language class is a set of languages
- Language classes have two important kinds of properties
- Decision properties
- Closure properties
Closure Properties
A closure property of a language class says that given languages in the class, an operation(e.g) produces another language in the same class
example: the regular language are closed under union, concatenation and Kleene closure
Representation of Languages
formal or informal
Decision Properties
- A decision property for a class of languages corresponds an algorithm that takes a formal description of a language and tell whether or not some property holds
- Example: Is language L empty
Why Decision Properties
We might want a “smallest” representation for a language, a minimum-state DFA or a shortest RE
The Emptiness Problem
The Infiniteness Problem
-
Is a given regular language infinite?
-
**Key idea: ** if the DFA has n states, and the language contains any string of length n or more, then the language is inifinite
-
Otherwise the language is surely finite
-
**Second key idea: ** if there is a string of length ≥ \ge ≥ n (= number of states) in L, then there is a string of length between n and 2n - 1
Proof
- Test for membership all strings of length between n and 2n -1
- If any are accepted, then infinite, else finite
- A terrible algorithm
- **Better: ** find cycles between the start state and a final state
Finding Cycles
- Eliminate states not reachable from the start state
- Eliminate states that do not reach a final state
- Test if then remaining transition graph has any cycle
The Pumping Lemma
泵引理
Statement of the Pumping Lemma
For every regular language L,
There is an integer n, such that
For every string w in L of length ≥ \ge ≥ n
We can write w = xyz such that:
- |xy| ≤ \le ≤ n
- |y| > 0
- For all i ≥ \ge ≥ 0, xy i ^i iz is in L
Example: Use of Pumping Lemma
泵引理帮助我们判断一些无穷语言是否为正则语言
{ 0 n 1 n ∣ k ≥ 1 } \{0^n 1^n | k \ge 1 \} {0n1n∣k≥1} is not a regular language
Proof
-
Let w = 0 n 1 n 0^n1^n 0n1n, then write x = xyz, and y consists of 0’ s, y ≠ ϵ \ne \epsilon =ϵ
-
But xyyz would be in L ,thus impossible
Decision Property: Equivalence
DFA L and M
-
Let these DFA has sets of states Q and R
-
Product DFA has set of states Q x R
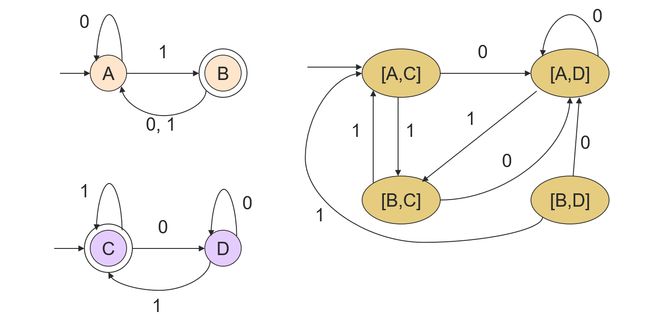
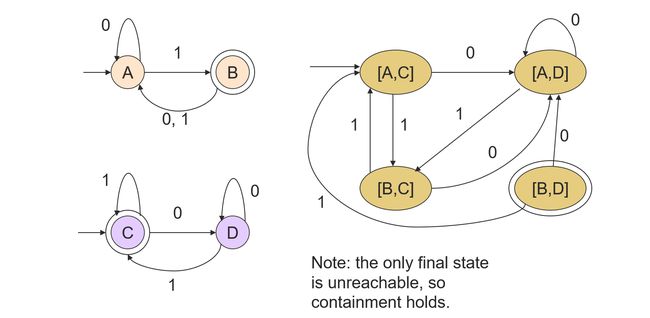
Decision Property: Containment
How do you define the final states [q.r] of the product so its language is empty iff L ⊆ \subseteq ⊆ M
**Answer: ** q is final; r is not
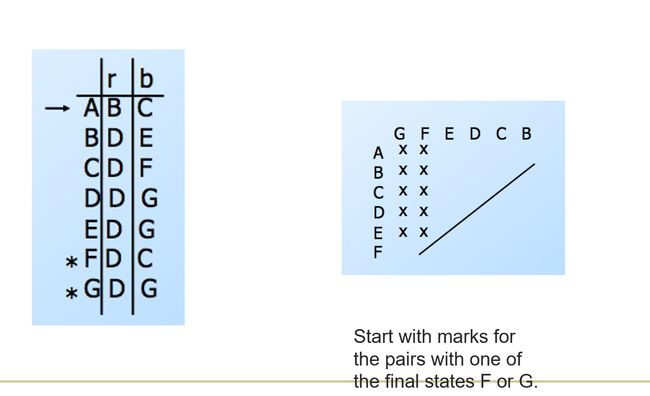
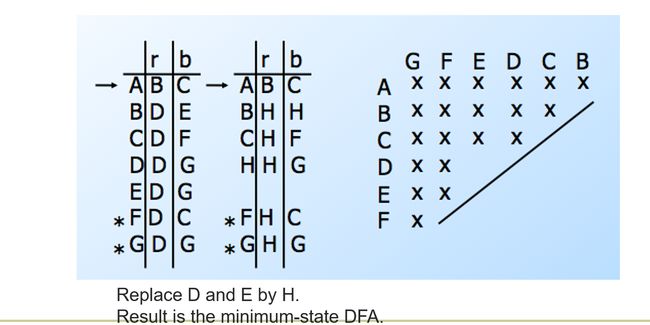
The Minimum-State DFA for a Regular Language
**Basis: ** Mark pairs with exactly one final state
**Induction: ** mark [q,r] if for some input symbol a, [ δ ( q , a ) , δ ( r , a ) \delta(q,a),\delta(r,a) δ(q,a),δ(r,a)] is marked
After no more marks are possible, the unmarked pairs are equivalent and can be into one state
Constructing the Minimum-State DFA
- Suppose q 1 , . . . , q k q_1,...,q_k q1,...,qk are indistinguishable states
- Replace them by one representative state q
- Then δ ( q 1 , a ) , . . . δ ( q k , a ) \delta(q_1,a),...\delta(q_k,a) δ(q1,a),...δ(qk,a) are all indistinguishable states.
Example
Eliminating Unreachable States
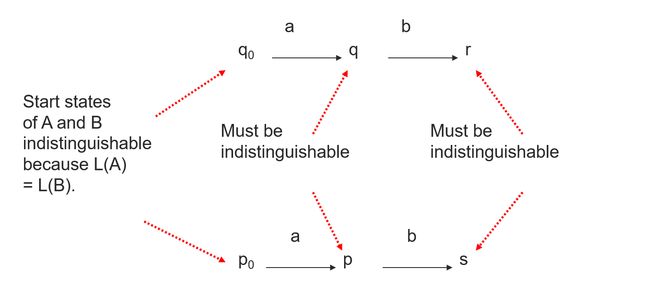
The proof involves minimizing the DFA we derived with the hypothetical better DFA
Proof: No unrelated, smaller DFA
IH: every state q of A is indistinguishable from some state of B
Proof
**Basis: ** Start states of A and B are indistinguishable
**Induction: ** Suppose w = xa, is a shortest string getting A to q
By IH, x gets to A to some state r that is indistinguishable from some state of B
Then δ A ( r , a ) = q \delta_A(r,a) = q δA(r,a)=q is indistinguishable from δ B ( p , a ) \delta_B(p,a) δB(p,a)
However, two states of A cannot be indistingruishable from the same state of B, thus, B has at least as many states as A
Closure Properties of Regular Languages
Union
- If L and M are regular languages, so is L ∪ \cup ∪ M
Intersection
- If L and M are regular languages, then so is L ∩ \cap ∩ M
- Proof: Construct C, the product automaton of A and B
Difference
- If L and M are regular languages, then so is L - M
- Proof: Construct product automaton
Reversal
Proof: Let E be a regular expression for L, We show how to reverse E, to provide a regular expression E R ^R R for L R ^R R
**Basis: ** If E is a symbol a, ϵ \epsilon ϵ, or ∅ \emptyset ∅, then E R ^R R = E
**Induction: ** If E is
- F + G, then E^R = F^R + G^R
- FG, then E^R = G R ^R R F R ^R R
- F ∗ ^* ∗, then E^R = (F R ^R R) ∗ ^* ∗
Homomorphisms
同态
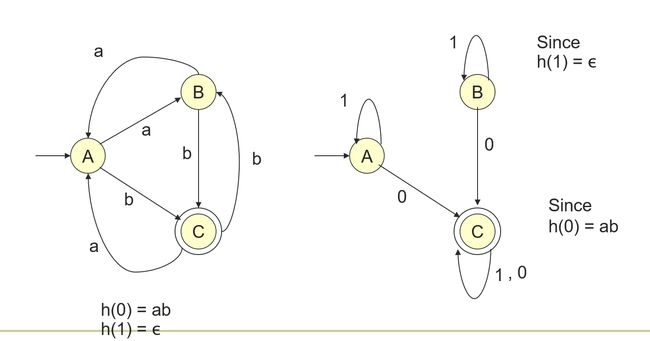
- A homomorphism on an alphabet is a function that gives a string for each symbol in that alphabet
- Example: h(0) = ab; h(1) = ϵ \epsilon ϵ
- Extend to strings by h(a 1 . . . _1... 1...a n _n n) = h(a 1 _1 1)…h(a n _n n)
- Example: h(01010) = ababab
Closure Under Homomorphism
- If L is a regular language, and h is a homomorphism on its alphabet, then h(L) = {h(w)|w is in L} is also a regular language
- Proof: Let E be a regular language expression for L
- Apply h to each symbol in E
- Language of resulting RE is h(L)
Inverse Homomorphisms
- h$^{-1} = $ {w | h(w) is in L}
Example Inverse Homomorphisms
- Let h(0) = ab; h(1) = ϵ \epsilon ϵ
- Let L = {abab,baba}
- h − 1 ^{-1} −1(L) = L( 1 ∗ 0 1 ∗ 0 1 ∗ 1^*01^*01^* 1∗01∗01∗)
Closure Proof
- Start with a DFA A for L
- Construct a DFA B for h − 1 ^{-1} −1(L) with
- the same set of states
- the same start
- the same final
- Input alphabet = the symbols to which homomorphism h applies
δ B ( q , a ) = δ A ( q , h ( a ) ) \delta_B(q,a) = \delta_A(q,h(a)) δB(q,a)=δA(q,h(a))
- An induction on |w| (omitted) shows that δ ( q 0 , w ) = δ A ( q 0 , h ( w ) ) \delta(q_0,w) = \delta_A(q_0,h(w)) δ(q0,w)=δA(q0,h(w))
- Thus, B accepts w if and only if A accepts h(w)