李群李代数:SO(3)和SE(3)
群论
定义
群(Group)是一种集合加上一个二元运算。
设 G G G是一个非空集合, ⋅ \cdot ⋅是一个二元运算,若满足如下条件:
- 封闭性: ∀ a , b ∈ G , a ⋅ b ∈ G \forall a,b\in G, a\cdot b \in G ∀a,b∈G,a⋅b∈G
- 结合律: ∀ a , b , c ∈ G , ( a ⋅ b ) ⋅ c = a ⋅ ( b ⋅ c ) \forall a,b,c\in G, (a\cdot b)\cdot c=a\cdot(b\cdot c) ∀a,b,c∈G,(a⋅b)⋅c=a⋅(b⋅c)
- 单位元(幺元): ∃ e ∈ G , s . t . ∀ a ∈ G a ⋅ e = e ⋅ a = a \exists e\in G,\enspace s.t.\enspace \forall a\in G\enspace a\cdot e=e\cdot a=a ∃e∈G,s.t.∀a∈Ga⋅e=e⋅a=a
- 逆元: ∀ a ∈ G , ∃ a − 1 ∈ G , s . t . a ⋅ a − 1 = a − 1 ⋅ a = e \forall a\in G,\exist a^{-1}\in G,\enspace s.t.\enspace a\cdot a^{-1}=a^{-1}\cdot a=e ∀a∈G,∃a−1∈G,s.t.a⋅a−1=a−1⋅a=e
则称 G G G对 ⋅ \cdot ⋅构成一个群,记作 ( G , ⋅ ) (G,\cdot) (G,⋅)。
- 称 G G G上二元运算 ⋅ \cdot ⋅为“乘法”
- a ⋅ b a\cdot b a⋅b为 a a a与 b b b的积,简写 a b ab ab
- 若群 G G G中元素有限,则称其为有限群,反之则称其为无限群
- 有限群的元素个数称为有限群的阶
子群
对于群 ( G , ⋅ ) (G,\cdot) (G,⋅)存在 H ⊂ G H\subset G H⊂G且 H ≠ ∅ H\neq \varnothing H=∅,若 ( H , ⋅ ) (H,\cdot) (H,⋅)也是一个群,则称 H H H为 G G G的子群。子群的充要条件如下:
H H = H 且 H − 1 = H HH=H且H^{-1}=H HH=H且H−1=H
李群
李群是一种具有连续(光滑)性质的群,它既是群也是流形。
刚体能够连续地在空间中运动,故而特殊正交群 S O ( n ) SO(n) SO(n)和特殊欧式群 S E ( n ) SE(n) SE(n)为李群。
李代数
定义
每个李群都有与之对应的李代数,李代数描述了李群单位元附近的正切空间性质。
李代数由一个集合 V V V,一个数域 F F F以及一个二元运算 [ , ] [,] [,]组成,记为 ( V , F , [ , ] ) (V,F,[,]) (V,F,[,])。其中二元运算 [ , ] [,] [,]被称为李括号,表示了两元素间的差异。
李代数满足如下条件:
-
封闭性: ∀ X , Y ∈ V , [ X , Y ] ∈ V \forall X,Y\in V,\enspace [X,Y]\in V ∀X,Y∈V,[X,Y]∈V
-
双线性: ∀ X , Y , Z ∈ V , a , b ∈ F \forall X,Y,Z\in V,\enspace a,b\in F ∀X,Y,Z∈V,a,b∈F有:
[ a X + b Y , Z ] = a [ X , Z ] + b [ Y , Z ] [ Z , a X + b Y ] = a [ Z , X ] + b [ Z , Y ] [aX+bY,Z]=a[X,Z]+b[Y,Z]\\ [Z,aX+bY]=a[Z,X]+b[Z,Y] [aX+bY,Z]=a[X,Z]+b[Y,Z][Z,aX+bY]=a[Z,X]+b[Z,Y] -
自反性: ∀ X ∈ V , [ X , X ] = 0 \forall X\in V,[X,X]=0 ∀X∈V,[X,X]=0
-
雅可比等价: ∀ X , Y , Z ∈ V , [ X , [ Y , Z ] ] + [ Y , [ Z , X ] ] + [ Z , [ X , Y ] ] = 0 \forall X,Y,Z\in V,[X,[Y,Z]]+[Y,[Z,X]]+[Z,[X,Y]]=0 ∀X,Y,Z∈V,[X,[Y,Z]]+[Y,[Z,X]]+[Z,[X,Y]]=0
-
反对称性: ∀ X , Y ∈ V , [ X , Y ] = − [ Y , X ] \forall X,Y\in V, [X,Y]=-[Y,X] ∀X,Y∈V,[X,Y]=−[Y,X]
推导(SO(3)李代数)
对于任意旋转矩阵 R R R,满足 R R T = I RR^T=I RRT=I。刚体在三维空间中的运动是连续的,随时间而变换,也即:
R ( t ) R ( t ) T = I R(t)R(t)^T=I R(t)R(t)T=I
两侧对时间 t t t进行求导:
R ˙ ( t ) R ( t ) T + R ( t ) R ˙ ( t ) T = 0 R ˙ ( t ) R ( t ) T = − R ( t ) R ˙ ( t ) T R ˙ ( t ) R ( t ) T = − ( R ˙ ( t ) R ( t ) T ) T \begin{aligned} \dot R(t)R(t)^T&+R(t)\dot R(t)^T=0\\ \dot R(t)R(t)^T&=-R(t)\dot R(t)^T\\ \dot R(t)R(t)^T&=-\Bigl(\dot R(t)R(t)^T\Bigr)^T \end{aligned} R˙(t)R(t)TR˙(t)R(t)TR˙(t)R(t)T+R(t)R˙(t)T=0=−R(t)R˙(t)T=−(R˙(t)R(t)T)T
由此,可知 R ˙ ( t ) R ( t ) T \dot R(t)R(t)^T R˙(t)R(t)T为反对称矩阵,记 R ˙ ( t ) R ( t ) T = ϕ ( t ) ∧ \dot R(t)R(t)^T=\phi(t)^\wedge R˙(t)R(t)T=ϕ(t)∧,等式两侧右乘 R ( t ) R(t) R(t):
R ˙ ( t ) = ϕ ( t ) ∧ R ( t ) R ˙ ( t ) = [ 0 − ϕ 3 ϕ 2 ϕ 3 0 − ϕ 1 − ϕ 2 ϕ 1 0 ] R ( t ) \begin{aligned} \dot R(t)&=\phi(t)^\wedge R(t)\\ \dot R(t)&=\begin{bmatrix}0&-\phi_3&\phi_2\\\phi_3&0&-\phi_1\\-\phi_2&\phi_1&0\end{bmatrix}R(t) \end{aligned} R˙(t)R˙(t)=ϕ(t)∧R(t)=⎣⎡0ϕ3−ϕ2−ϕ30ϕ1ϕ2−ϕ10⎦⎤R(t)
由上式可知,每对旋转矩阵求一次导数,只需对其左乘一个 ϕ ( t ) ∧ \phi(t)^\wedge ϕ(t)∧。
S O ( 3 ) SO(3) SO(3)的单位元为: t 0 = 0 , R ( 0 ) = I t_0=0,R(0)=I t0=0,R(0)=I,将 R ( t ) R(t) R(t)在单位元附近进行一阶泰勒展开:
R ( t ) ≈ R ( t 0 ) + R ˙ ( t 0 ) ( t − t 0 ) = I + ϕ ( t 0 ) ∧ R ( t 0 ) ( t − t 0 ) = I + ϕ ( t 0 ) ∧ t \begin{aligned} R(t)&\approx R(t_0)+\dot R(t_0)(t-t_0)\\ &=I+\phi(t_0)^\wedge R(t_0)(t-t_0)\\ &=I+\phi(t_0)^\wedge t \end{aligned} R(t)≈R(t0)+R˙(t0)(t−t0)=I+ϕ(t0)∧R(t0)(t−t0)=I+ϕ(t0)∧t
ϕ \phi ϕ反应了旋转矩阵 R R R的导数性质,称其在 S O ( 3 ) SO(3) SO(3)原点附近的正切空间上。
设 t 0 t_0 t0附近 ϕ \phi ϕ为常数不变: ϕ ( t 0 ) = ϕ 0 \phi(t_0)=\phi_0 ϕ(t0)=ϕ0,则有:
R ˙ ( t ) = ϕ 0 ∧ R ( t ) \dot R(t)=\phi_0^\wedge R(t) R˙(t)=ϕ0∧R(t)
在初始条件 R ( 0 ) = I R(0)=I R(0)=I下解微分方程,得到:
R ( t ) = e x p ( ϕ 0 ∧ t ) R(t)=exp(\phi_0^\wedge t) R(t)=exp(ϕ0∧t)
上式称为李代数 s o ( 3 ) so(3) so(3)的指数映射关系。
李代数so(3)
向量空间: s o ( 3 ) = { Φ = ϕ ∧ ∈ ℜ 3 × 3 ∣ ϕ ∈ ℜ 3 } so(3)=\Bigl\{\Phi=\phi^\wedge\in\Re^{3\times3}\enspace\Big\vert \phi\in\Re^3\Bigr\} so(3)={Φ=ϕ∧∈ℜ3×3∣∣∣ϕ∈ℜ3}
数域: ℜ \Re ℜ
李括号: [ ϕ a , ϕ b ] = Φ a Φ b − Φ b Φ a [\phi_a,\phi_b]=\Phi_a\Phi_b-\Phi_b\Phi_a [ϕa,ϕb]=ΦaΦb−ΦbΦa
[ ϕ a , ϕ b ] = Φ a Φ b − Φ b Φ a = ϕ a ∧ ϕ b ∧ − ϕ b ∧ ϕ a ∧ = ( ϕ a ∧ ϕ b ) ∧ ∈ s o ( 3 ) \begin{aligned} {[}\phi_a,\phi_b]&=\Phi_a\Phi_b-\Phi_b\Phi_a\\ &=\phi_a^\land\phi_b^\land-\phi_b^\land\phi_a^\land\\ &=\bigl(\phi_a^\land\phi_b\bigr)^\land\in so(3) \end{aligned} [ϕa,ϕb]=ΦaΦb−ΦbΦa=ϕa∧ϕb∧−ϕb∧ϕa∧=(ϕa∧ϕb)∧∈so(3)
其中 Φ = ϕ ∧ \Phi=\phi^\land Φ=ϕ∧,为反对称矩阵:
Φ = [ 0 − ϕ 3 ϕ 2 ϕ 3 0 − ϕ 1 − ϕ 2 ϕ 1 0 ] ∈ ℜ 3 × 3 \Phi=\begin{bmatrix}0&-\phi_3&\phi_2\\\phi_3&0&-\phi_1\\-\phi_2&\phi_1&0\end{bmatrix}\in \Re^{3\times3} Φ=⎣⎡0ϕ3−ϕ2−ϕ30ϕ1ϕ2−ϕ10⎦⎤∈ℜ3×3
视集合 ℜ 3 \Re^3 ℜ3和叉乘运算作为 s e ( 3 ) se(3) se(3)
李代数se(3)
向量空间: s e ( 3 ) = { Ξ = ξ ∧ ∈ ℜ 4 × 4 ∣ ξ ∈ ℜ 6 } se(3)=\Biggl\{\Xi=\xi^\land\in\Re^{4\times4} \Bigg\vert\xi\in\Re^{6}\Biggr\} se(3)={Ξ=ξ∧∈ℜ4×4∣∣∣∣∣ξ∈ℜ6}
数域: ℜ \Re ℜ
李括号 [ Ξ 1 , Ξ 2 ] = Ξ 1 Ξ 2 − Ξ 2 Ξ 1 [\Xi_1,\Xi_2]=\Xi_1\Xi_2-\Xi_2\Xi_1 [Ξ1,Ξ2]=Ξ1Ξ2−Ξ2Ξ1
[ Ξ a , Ξ b ] = Ξ a Ξ b − Ξ a Ξ b = ξ a ∧ ξ b ∧ − ξ b ∧ ξ a ∧ = ( ξ 1 ⋆ ξ 2 ) ∧ ∈ s e ( 3 ) \begin{aligned} {[}\Xi_a,\Xi_b]&=\Xi_a\Xi_b-\Xi_a\Xi_b\\ &=\xi_a^\land\xi_b^\land-\xi_b^\land\xi_a^\land\\ &=\bigl(\xi_1^{\star}\xi_2\bigr)^\land\in se(3) \end{aligned} [Ξa,Ξb]=ΞaΞb−ΞaΞb=ξa∧ξb∧−ξb∧ξa∧=(ξ1⋆ξ2)∧∈se(3)
其中,参量 ξ \xi ξ用于表示平移加旋转:
ξ = [ ρ ϕ ] , ρ ∈ ℜ 3 , ϕ ∈ s o ( 3 ) \xi=\begin{bmatrix}\rho\\\phi\end{bmatrix},\rho\in\Re^3,\phi\in so(3) ξ=[ρϕ],ρ∈ℜ3,ϕ∈so(3)
ξ ∧ \xi^\land ξ∧非反对称矩阵,但仍保留记法:
ξ ∧ = [ ϕ ∧ ρ 0 T 0 ] ∈ ℜ 4 × 4 \xi^\land=\begin{bmatrix}\phi^\land&\rho\\0^T&0\end{bmatrix}\in\Re^{4\times4} ξ∧=[ϕ∧0Tρ0]∈ℜ4×4
星计算 ξ ⋆ \xi^\star ξ⋆定义为:
ξ ⋆ = [ ρ ϕ ] ⋆ = [ ϕ ∧ ρ ∧ 0 ϕ ∧ ] ∈ ℜ 6 × 6 \xi^\star=\begin{bmatrix}\rho\\\phi\end{bmatrix}^\star=\begin{bmatrix}\phi^\land&\rho^\land\\0&\phi^\land\end{bmatrix}\in\Re^{6\times6} ξ⋆=[ρϕ]⋆=[ϕ∧0ρ∧ϕ∧]∈ℜ6×6
视集合 ℜ 6 \Re^6 ℜ6和星计算为 s e ( 3 ) se(3) se(3)
指数映射与对数映射
指数映射反应了从李代数到李群的转换,任意矩阵的指数映射可写成一个泰勒展开,其结果仍是一个矩阵:
e x p ( G ) = ∑ n = 0 ∞ 1 n ! G n exp(G)=\sum_{n=0}^\infty\frac{1}{n!}G^n exp(G)=n=0∑∞n!1Gn
对数映射则反应了从李群到李代数的转换:
ln ( G ) = ∑ n = 0 ∞ ( − 1 ) n n + 1 ( G − I ) n + 1 \ln(G)=\sum_{n=0}^\infty\frac{(-1)^{n}}{n+1}(G-I)^{n+1} ln(G)=n=0∑∞n+1(−1)n(G−I)n+1
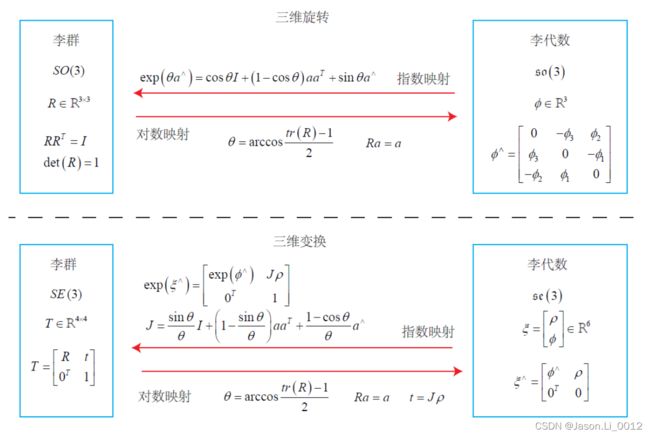
李群 S O ( 3 ) SO(3) SO(3)李代数 s o ( 3 ) so(3) so(3)
ϕ \phi ϕ是一个向量,定义其模长为 θ \theta θ方向为 a a a, ϕ = θ a \phi=\theta a ϕ=θa。其中, a a a为一个模长为1的单位向量: ∥ a ∥ = 1 \begin{Vmatrix}a\end{Vmatrix}=1 ∥∥a∥∥=1。
对于反对称矩阵 a ∧ a^\land a∧,易得:
式 1 : a ∧ a ∧ = a a T − I 式 2 : a ∧ a ∧ a ∧ = a ∧ ( a a T − I ) = − a ∧ \begin{aligned} 式1:\qquad&a^\land a^\land=aa^T-I\\ 式2:\qquad&a^\land a^\land a^\land=a^\land (aa^T-I)=-a^\land \end{aligned} 式1:式2:a∧a∧=aaT−Ia∧a∧a∧=a∧(aaT−I)=−a∧
指数映射
对指数映射进行Taylor展开并将上述等式代入:
e x p ( ϕ ∧ ) = e x p ( θ a ∧ ) = ∑ n = 0 ∞ 1 n ! ( θ a ∧ ) n 1 : = I + θ a ∧ + 1 2 ! θ 2 a ∧ a ∧ + 1 3 ! θ 3 a ∧ a ∧ a ∧ + 1 4 ! θ 4 ( a ∧ ) 4 + ⋯ 2 : = ( a a T − a ∧ a ∧ ) + θ a ∧ + 1 2 ! θ 2 a ∧ a ∧ − 1 3 ! θ 3 a ∧ − 1 4 ! θ 4 a ∧ a ∧ + ⋯ 3 : = a a T + ( θ − 1 3 ! θ 3 + 1 5 ! θ 5 − ⋯ ) a ∧ − ( 1 − 1 2 ! θ 2 + 1 4 ! θ 4 − ⋯ ) a ∧ a ∧ 4 : = ( I + a ∧ a ∧ ) + sin θ a ∧ − cos θ a ∧ a ∧ 5 : = ( 1 − cos θ ) a ∧ a ∧ + I + sin θ a ∧ 6 : = ( 1 − cos θ ) ( a a T − I ) + I + sin θ a ∧ 7 : = cos θ I + ( 1 − cos θ ) a a T + sin θ a ∧ \begin{aligned} exp(\phi^\land)&=exp(\theta a^\land)=\sum^\infty_{n=0}\frac{1}{n!}(\theta a^\land)^n\\ 1:\qquad &=I+\theta a^\land + \frac{1}{2!}\theta^2a^\land a^\land +\frac{1}{3!}\theta^3 a^\land a^\land a^\land+\frac{1}{4!}\theta^4(a^\land)^4+\cdots\\ 2:\qquad &=(aa^T-a^\land a^\land)+\theta a^\land+\frac{1}{2!}\theta^2a^\land a^\land -\frac{1}{3!}\theta^3 a^\land-\frac{1}{4!}\theta^4a^\land a^\land+\cdots\\ 3:\qquad &=aa^T+\Bigl(\theta-\frac{1}{3!}\theta^3+\frac{1}{5!}\theta^5-\cdots\Bigr)a^\land-\Bigl(1-\frac{1}{2!}\theta^2+\frac{1}{4!}\theta^4-\cdots\Bigr)a^\land a^\land\\ 4:\qquad &=(I+a^\land a^\land) +\sin\theta a^\land -\cos\theta a^\land a^\land\\ 5:\qquad &=(1-\cos\theta)a^\land a^\land+I+\sin\theta a^\land\\ 6:\qquad &=(1-\cos\theta)(aa^T-I)+I+\sin\theta a^\land\\ 7:\qquad &=\cos\theta I+(1-\cos\theta)aa^T+\sin\theta a^\land \end{aligned} exp(ϕ∧)1:2:3:4:5:6:7:=exp(θa∧)=n=0∑∞n!1(θa∧)n=I+θa∧+2!1θ2a∧a∧+3!1θ3a∧a∧a∧+4!1θ4(a∧)4+⋯=(aaT−a∧a∧)+θa∧+2!1θ2a∧a∧−3!1θ3a∧−4!1θ4a∧a∧+⋯=aaT+(θ−3!1θ3+5!1θ5−⋯)a∧−(1−2!1θ2+4!1θ4−⋯)a∧a∧=(I+a∧a∧)+sinθa∧−cosθa∧a∧=(1−cosθ)a∧a∧+I+sinθa∧=(1−cosθ)(aaT−I)+I+sinθa∧=cosθI+(1−cosθ)aaT+sinθa∧
上述计算过程的处理思路如下:
-
第一步:泰勒展开
-
第二步:将式1代入替换第一步中的 I I I;将式2代入替换 a ∧ a ∧ a ∧ a^\land a^\land a^\land a∧a∧a∧
-
第三步:对第二步进行整理
-
第四步:将式1代入替换第三步中的 a a T aa^T aaT;将三角函数的泰勒展开形式写回三角函数形式:
sin θ = θ − 1 3 ! θ 3 + 1 5 ! θ 5 − ⋯ cos θ = 1 − 1 2 ! θ 2 + 1 4 ! θ 4 − ⋯ \begin{aligned} \sin\theta &=\theta-\frac{1}{3!}\theta^3+\frac{1}{5!}\theta^5-\cdots\\ \cos\theta &=1-\frac{1}{2!}\theta^2+\frac{1}{4!}\theta^4-\cdots \end{aligned} sinθcosθ=θ−3!1θ3+5!1θ5−⋯=1−2!1θ2+4!1θ4−⋯ -
第五步:对第四步进行整理
-
第六步:将式1代入替换第五步中 a ∧ a ∧ a^\land a^\land a∧a∧
-
第七步:对第六步进行整理
最终得到李代数 s o ( 3 ) so(3) so(3)到李群 S O ( 3 ) SO(3) SO(3)的指数映射:
e x p ( ϕ ∧ ) = e x p ( θ a ∧ ) = cos θ I + ( 1 − cos θ ) a a T + sin θ a ∧ exp(\phi^\land)=exp(\theta a^\land)=\cos\theta I+(1-\cos\theta)aa^T+\sin\theta a^\land exp(ϕ∧)=exp(θa∧)=cosθI+(1−cosθ)aaT+sinθa∧
该式被称为罗德里格斯公式,李代数 s o ( 3 ) so(3) so(3)的物理意义就是旋转向量。
对数映射
由 S O ( 3 ) SO(3) SO(3)到 s o ( 3 ) so(3) so(3)的对数映射如下:
ϕ = ln ( R ) ∨ = ( ∑ n = 0 ∞ ( − 1 ) n n + 1 ( R − I ) n + 1 ) ∨ \phi=\ln(R)^\lor=\Biggl(\sum_{n=0}^\infty\frac{(-1)^{n}}{n+1}(R-I)^{n+1}\Biggr)^\lor ϕ=ln(R)∨=(n=0∑∞n+1(−1)n(R−I)n+1)∨
对数映射实现由旋转矩阵到旋转向量的转换,可使用刚体变换中计算方式,对指数映射两侧求迹从而简化计算:
θ = arccos t r ( R ) − 1 2 R n = n \theta=\arccos\frac{tr(R)-1}{2}\qquad\qquad\qquad\qquad Rn=n θ=arccos2tr(R)−1Rn=n
注意点
指数映射是一个满射,不是一个单射。也即每个 S O ( 3 ) SO(3) SO(3)内的元素,都可以找到一个 s o ( 3 ) so(3) so(3)元素与之一一对应;但可能存在多个 s o ( 3 ) so(3) so(3)中的元素,对应到一个 S O ( 3 ) SO(3) SO(3)中元素。
若将旋转角度固定至 ± π \pm\pi ±π中,则可认为李群、李代数中元素一一对应。
李群 S E ( 3 ) SE(3) SE(3)李代数 s e ( 3 ) se(3) se(3)
指数映射
由李代数 s e ( 3 ) se(3) se(3)到李群 S E ( 3 ) SE(3) SE(3)的转换如下:
e x p ( ξ ∧ ) = [ ∑ n = 0 ∞ 1 n ! ( ϕ ∧ ) n ∑ n = 0 ∞ 1 ( n + 1 ) ! ( ϕ ∧ ) n ρ 0 T 1 ] ≜ [ R J ρ 0 T 1 ] = T \begin{aligned} exp(\xi^\land)&=\begin{bmatrix}\sum^\infty_{n=0}\frac{1}{n!}(\phi^\land)^n&\sum_{n=0}^\infty\frac{1}{(n+1)!}(\phi^\land)^n\rho\\0^T&1\end{bmatrix}\\ &\triangleq \begin{bmatrix}R&J\rho\\0^T&1\end{bmatrix}\\ &=T \end{aligned} exp(ξ∧)=[∑n=0∞n!1(ϕ∧)n0T∑n=0∞(n+1)!1(ϕ∧)nρ1]≜[R0TJρ1]=T
式中 J J J称为雅可比矩阵:
J = ∑ n = 0 ∞ 1 ( n + 1 ) ! ( ϕ ∧ ) n = ∑ n = 0 ∞ 1 ( n + 1 ) ! ( θ a ∧ ) n 1 : = I + 1 2 ! θ a ∧ + 1 3 ! θ 2 ( a ∧ ) 2 + 1 4 ! θ 3 ( a ∧ ) 3 + 1 5 ! θ 4 ( a ∧ ) 4 + ⋯ 2 : = I + 1 θ ( 1 2 ! θ 2 a ∧ + 1 3 ! θ 3 ( a ∧ ) 2 + 1 4 ! θ 4 ( a ∧ ) 3 + 1 5 ! θ 5 ( a ∧ ) 4 + ⋯ ) 3 : = I + 1 θ ( 1 2 ! θ 2 a ∧ + 1 3 ! θ 3 ( a ∧ ) 2 − 1 4 ! θ 4 a ∧ − 1 5 ! θ 5 ( a ∧ ) 2 + ⋯ ) 4 : = I + 1 θ ( 1 2 ! θ 2 − 1 4 ! θ 4 + ⋯ ) a ∧ + 1 θ ( 1 3 ! θ 3 − 1 5 ! θ 5 + ⋯ ) ( a ∧ ) 2 5 : = I + 1 θ ( 1 − cos θ ) a ∧ + 1 θ ( θ − sin θ ) ( a ∧ ) 2 6 : = I + 1 θ ( 1 − cos θ ) a ∧ + 1 θ ( θ − sin θ ) ( a a T − I ) 7 : = sin θ θ I + ( 1 − sin θ θ ) a a T + 1 − cos θ θ a ∧ \begin{aligned} J=&\sum_{n=0}^\infty\frac{1}{(n+1)!}(\phi^\land)^n=\sum_{n=0}^\infty\frac{1}{(n+1)!}(\theta a^\land)^n\\ 1:\qquad =&I+\frac{1}{2!}\theta a^\land+\frac{1}{3!}\theta^2 (a^\land)^2+\frac{1}{4!}\theta^3 (a^\land)^3+\frac{1}{5!}\theta^4 (a^\land)^4+\cdots\\ 2:\qquad =&I+\frac{1}{\theta}\Bigl(\frac{1}{2!}\theta^2 a^\land+\frac{1}{3!}\theta^3 (a^\land)^2+\frac{1}{4!}\theta^4 (a^\land)^3+\frac{1}{5!}\theta^5 (a^\land)^4+\cdots\Bigr) \\ 3:\qquad =&I+\frac{1}{\theta}\Bigl(\frac{1}{2!}\theta^2 a^\land+\frac{1}{3!}\theta^3 (a^\land)^2-\frac{1}{4!}\theta^4 a^\land-\frac{1}{5!}\theta^5 (a^\land)^2+\cdots\Bigr)\\ 4:\qquad =& I+\frac{1}{\theta}\Bigl(\frac{1}{2!}\theta^2-\frac{1}{4!}\theta^4+\cdots\Bigr)a^\land + \frac{1}{\theta}\Bigl(\frac{1}{3!}\theta^3-\frac{1}{5!}\theta^5+\cdots\Bigr)(a^\land)^2 \\ 5:\qquad =& I+\frac{1}{\theta}(1-\cos\theta)a^\land+\frac{1}{\theta}(\theta-\sin\theta)(a^\land)^2\\ 6:\qquad =& I+\frac{1}{\theta}(1-\cos\theta)a^\land+\frac{1}{\theta}(\theta-\sin\theta)(aa^T-I)\\ 7:\qquad =& \frac{\sin\theta}{\theta}I+\Bigl(1-\frac{\sin\theta}{\theta}\Bigr)aa^T+\frac{1-\cos\theta}{\theta}a^\land\\ \end{aligned} J=1:=2:=3:=4:=5:=6:=7:=n=0∑∞(n+1)!1(ϕ∧)n=n=0∑∞(n+1)!1(θa∧)nI+2!1θa∧+3!1θ2(a∧)2+4!1θ3(a∧)3+5!1θ4(a∧)4+⋯I+θ1(2!1θ2a∧+3!1θ3(a∧)2+4!1θ4(a∧)3+5!1θ5(a∧)4+⋯)I+θ1(2!1θ2a∧+3!1θ3(a∧)2−4!1θ4a∧−5!1θ5(a∧)2+⋯)I+θ1(2!1θ2−4!1θ4+⋯)a∧+θ1(3!1θ3−5!1θ5+⋯)(a∧)2I+θ1(1−cosθ)a∧+θ1(θ−sinθ)(a∧)2I+θ1(1−cosθ)a∧+θ1(θ−sinθ)(aaT−I)θsinθI+(1−θsinθ)aaT+θ1−cosθa∧
上述计算过程的处理思路如下:
-
第一步:泰勒展开
-
第二步:除单位阵 I I I外其余项提出一个因子 1 θ \frac{1}{\theta} θ1
-
第三步:将式2代入代替 ( a ∧ ) 3 (a^\land)^3 (a∧)3
-
第四步:对第三步进行整理,将有共同项的写在一起
-
第五步:将三角函数的泰勒展开进行整理后代入上式:
cos θ = 1 − 1 2 ! θ 2 + 1 4 ! θ 4 − ⋯ 1 − cos θ = 1 2 ! θ 2 − 1 4 ! θ 4 + ⋯ sin θ = θ − 1 3 ! θ 3 + 1 5 ! θ 5 − ⋯ θ − sin θ = 1 3 ! θ 3 − 1 5 ! θ 5 + ⋯ \begin{aligned} \cos\theta &=1-\frac{1}{2!}\theta^2+\frac{1}{4!}\theta^4-\cdots\\ 1-\cos\theta&=\frac{1}{2!}\theta^2-\frac{1}{4!}\theta^4+\cdots\\ \\ \sin\theta &=\theta-\frac{1}{3!}\theta^3+\frac{1}{5!}\theta^5-\cdots\\ \theta-\sin\theta&=\frac{1}{3!}\theta^3-\frac{1}{5!}\theta^5+\cdots \end{aligned} cosθ1−cosθsinθθ−sinθ=1−2!1θ2+4!1θ4−⋯=2!1θ2−4!1θ4+⋯=θ−3!1θ3+5!1θ5−⋯=3!1θ3−5!1θ5+⋯ -
第六步:将式1代入代替 ( a ∧ ) 2 (a^\land)^2 (a∧)2
-
第七步:对第六步进行整理
由此得到雅可比矩阵的表达式:
J = sin θ θ I + ( 1 − sin θ θ ) a a T + 1 − cos θ θ a ∧ J=\frac{\sin\theta}{\theta}I+\Bigl(1-\frac{\sin\theta}{\theta}\Bigr)aa^T+\frac{1-\cos\theta}{\theta}a^\land J=θsinθI+(1−θsinθ)aaT+θ1−cosθa∧
对数映射
从 S E ( 3 ) SE(3) SE(3)到 s e ( 3 ) se(3) se(3)的转换,如下:
ξ = ln ( T ) ∨ \xi=\ln(T)^\lor ξ=ln(T)∨
在进行实际转换时,使用对数映射较为复杂。一般用 T T T左上角旋转矩阵 R R R求解旋转向量;再由右侧平移向量计算平移部分:
t = J ρ t=J\rho t=Jρ