一、题目658.找到K个最接近的元素给定一个排序好的数组arr,两个整数k和x,从数组中找到最靠近x(两数之差最小)的k个数。返回的结果必须要是按升序排好的。整数a比整数b更接近x需要满足:|a-x|<|b-x|或者|a-x|==|b-x|且a
leetcode 658. 找到 K 个最接近的元素
leetcode658.找到K个最接近的元素题目描述:给定一个排序好的数组arr,两个整数k和x,从数组中找到最靠近x(两数之差最小)的k个数。返回的结果必须要是按升序排好的。整数a比整数b更接近x需要满足:|a-x|findClosestElements(vector&arr,intk,intx){intn=arr.size()-1;intl=0,r=n;k--;while(r-l>k){if(
【二分查找】leetcode 658. 找到 K 个最接近的元素
lele_ne
#双指针#二分查找算法之路leetcode算法数据结构
658.找到K个最接近的元素题目描述给定一个排序好的数组arr,两个整数k和x,从数组中找到最靠近x(两数之差最小)的k个数。返回的结果必须要是按升序排好的。整数a比整数b更接近x需要满足:|a-x|arr[right]−xx-arr[left]>arr[right]-xx−arr[left]>arr[right]−x,右指针上的元素存入数组,并向右移动;如果右指针已经移动到超过数组arr的长度,
Leetcode 658. 找到k个最接近的元素
皮卡猴
leetcode刷题leetcode算法排序算法
Leetcode658.找到k个最接近的元素1.问题描述2.思路 按照递增序列,找到x应该插入的位置index。以此为中心,向两侧扩展长度为k的空间,该空间内的元素就是我们想要的元素。1二分查找,找到满足=0,且jlen(arr)-1,向左移动i3.代码3.1第一次运行成功funcfindClosestElements(arr[]int,kint,xint)[]int{varres[]intin
LeetCode 25. K 个一组翻转链表 java题解
反转链表进阶版题目https://leetcode-cn.com/problems/reverse-nodes-in-k-group/代码/***Definitionforsingly-linkedlist.*publicclassListNode{*intval;*ListNodenext;*ListNode(){}*ListNode(intval){this.val=val;}*ListNod
LeetCode 1471.数组中的k个最强值
吃着火锅x唱着歌
LeetCodeleetcode算法数据结构
给你一个整数数组arr和一个整数k。设m为数组的中位数,只要满足下述两个前提之一,就可以判定arr[i]的值比arr[j]的值更强:|arr[i]-m|>|arr[j]-m||arr[i]-m|==|arr[j]-m|,且arr[i]>arr[j]请返回由数组中最强的k个值组成的列表。答案可以以任意顺序返回。中位数是一个有序整数列表中处于中间位置的值。形式上,如果列表的长度为n,那么中位数就是该有
LeetCode 633.平方数之和(python题解)
wendong97
LeetCode从零起步#双指针leetcodepython算法
LeetCode633.平方数之和(python题解)题目示例分析题解用到的基础知识计算平方和平方根取整参考题目给定一个非负整数c,你要判断是否存在两个整数a和b,使得a2+b2=c示例输入:5输出:True解释:1*1+2*2=5分析本题与上一题LeetCode167.两数之和II非常相似,把两数之和变成了平方数之和,利用上一题答案的思路,同样设置两个一左一右的指针,很容易就可以解答出来。这里要
Leetcode—347. 前 K 个高频元素【中等】(桶排序)
源代码•宸
LeetCode刷题leetcode算法经验分享c++桶排序
2024每日刷题(178)Leetcode—347.前K个高频元素桶排序实现代码classSolution{public:vectortopKFrequent(vector&<
Leetcode刷题笔记——哈希表篇
code_lover_forever
Leetcode刷题笔记leetcode笔记散列表python
Leetcode刷题笔记——哈希表篇一、哈希表在面试中的高频考题第一题:两数之和Leetcode1:两数之和:中等题(详情点击链接见原题)给定一个整数数组nums和一个整数目标值target,请你在该数组中找出和为目标值target的那两个整数,并返回它们的数组下标python代码解法classSolution:deftwoSum(self,nums:List[int],target:int)->
138. 复制带随机指针的链表
秃头哥编程
2021-07-22LeetCode每日一题链接:https://leetcode-cn.com/problems/copy-list-with-random-pointer/标签:哈希表、链表题目给你一个长度为n的链表,每个节点包含一个额外增加的随机指针random,该指针可以指向链表中的任何节点或空节点。构造这个链表的深拷贝。深拷贝应该正好由n个全新节点组成,其中每个新节点的值都设为其对应的原
LeetCode 每日一题 2024/10/21-2024/10/27
alphaTao
Exerciseleetcode算法
记录了初步解题思路以及本地实现代码;并不一定为最优也希望大家能一起探讨一起进步目录10/21910.最小差值II10/223184.构成整天的下标对数目I10/233185.构成整天的下标对数目II10/243175.找到连续赢K场比赛的第一位玩家10/253180.执行操作可获得的最大总奖励I10/263181.执行操作可获得的最大总奖励II10/27684.冗余连接10/21910.最小差值I
leetcode-5. 最长回文子串(c++)
应技大学子
力扣—字符串pycharmpythonide
题目:给你一个字符串s,找到s中最长的回文子串。示例:输入:s="babad"输出:"bab"、"aba"同样是符合题意的答案。解法1:中心扩散法从每一个位置出发,向两边扩散即可。遇到不是回文的时候结束。例str=acdbbdaa我们需要寻找从第一个b。首先往左寻找与当期位置相同的字符,直到遇到不相等为止。然后往右寻找与当期位置相同的字符,直到遇到不相等为止。最后左右双向扩散,直到左和右不相等。如
【c++】leetcode5 最长回文子串
1.题目5.最长回文子串-力扣(LeetCode)2.codeclassSolution{public:stringlongestPalindrome(strings){stringres="";for(autoi=0U;ires.length()?s1:res;res=s2.length()>res.length()?s2:res;}returnres;}stringpalindrome(str
java学习day6 + leetcode31 下一个排列
冬夜戏雪
java学习算法
1.消息队列和一些功能P74P75P76基于stream的消息队列单消费模式消费者组P77基于消息队列的异步秒杀下单shift2提及,插入已知笔记P78探店笔记P79查看探店笔记p80点赞功能一人一赞这里也有并发P81点赞排行榜sortedsetset集合的选择redis里面的zsetmybatis改sql排序语句p82好友关注关注和取关p83共同关注redis里的set交集功能解析id集合没看懂
java学习 leetcode31 下一个排列
冬夜戏雪
java学习leetcode
1.排列方法(按照全排列,数组,整数来回转换的思路)packagecom.hmdp.leetcode;importjava.util.*;publicclassbacktracking31{publicvoidnextPermutation(int[]nums){//1.将当前数组转为字符串表示StringBuildersb=newStringBuilder();for(intnum:nums){
【数据结构】详解堆排序当中的topk问题(leetcode例题)
ylfxw
数据结构leetcode算法
文章目录前言如何理解topk问题代码逻辑代码实现前言Leetcode相关题目:215.数组中的第K个最大元素如何理解topk问题**TopK问题是一个经典的问题,在计算机科学中,它的目标是在一组数据中找到前K个最大或最小的元素。**这个问题在许多场景下都很重要,比如搜索引擎的搜索结果排名、数据分析中的热门元素筛选等。.在最简单的形式中,给定一个数组(或列表)和一个整数K,TopK问题要求返回数组中
2163. 删除元素后和的最小差值
咔咔咔的
leetcodec++
2163.删除元素后和的最小差值题目链接:2163.删除元素后和的最小差值代码如下://参考链接:https://leetcode.cn/problems/minimum-difference-in-sums-after-removal-of-elements/solutions/1247074/qian-zhui-zui-xiao-he-hou-zhui-zui-da-he-yz3dclassS
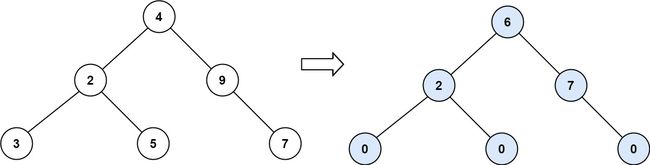
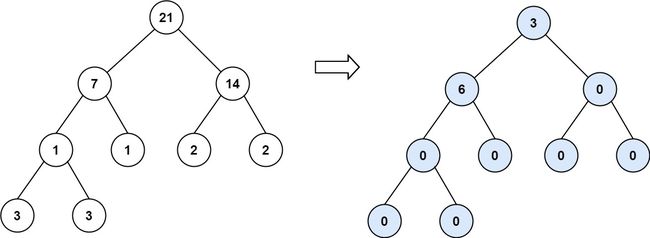
104. 二叉树的最大深度
间歇性发呆
给定一个二叉树,找出其最大深度。二叉树的深度为根节点到最远叶子节点的最长路径上的节点数。说明:叶子节点是指没有子节点的节点。示例:给定二叉树[3,9,20,null,null,15,7],3/\920/\157返回它的最大深度3。来源:力扣(LeetCode)链接:https://leetcode-cn.com/problems/maximum-depth-of-binary-tree著作权归领扣
LeetCode第860题解析
在柠檬水摊上,每一杯柠檬水的售价为5美元。顾客排队购买你的产品,(按账单bills支付的顺序)一次购买一杯。每位顾客只买一杯柠檬水,然后向你付5美元、10美元或20美元。你必须给每个顾客正确找零,也就是说净交易是每位顾客向你支付5美元。注意,一开始你手头没有任何零钱。如果你能给每位顾客正确找零,返回true,否则返回false。示例1:输入:[5,5,5,10,20]输出:true解释:前3位顾客
【leetcode-字符串】单词搜索 II
程序员小2
【leetcode-字符串】单词搜索II题目:给定一个二维网格board和一个字典中的单词列表words,找出所有同时在二维网格和字典中出现的单词。单词必须按照字母顺序,通过相邻的单元格内的字母构成,其中“相邻”单元格是那些水平相邻或垂直相邻的单元格。同一个单元格内的字母在一个单词中不允许被重复使用。示例:输入:words=["oath","pea","eat","rain"]andboard=[
【力扣】第42题:接雨水
jstart千语
力扣算法leetcode算法职场和发展
原文链接:42.接雨水-力扣(LeetCode)1、题目解析解读:给定一个数组,使数组的值为高形成柱子,按照短板效应原理能剩多少水。核心思想:每一个坐标位置可以承装的水=min(左边最高柱子,右边最高柱子)-该坐标值2、编码实现方法一我们可以用两个数组,一个用来记录每一个坐标值的左边中柱子的最高值,一个用来记录每一个坐标值右边中柱子的最高值。当我们要记录某一个坐标值能盛装多少水时,根据上面提供的公
LeetCode - 3274. Check if Two Chessboard Squares Have the Same Color
阿蒙Armon
LeetCodeleetcode算法职场和发展
LeetCode-3274.CheckifTwoChessboardSquaresHavetheSameColor在LeetCode的算法题库中,有许多有趣的题目将实际场景与编程逻辑相结合,LeetCode3274题CheckifTwoChessboardSquaresHavetheSameColor便是其中之一。这道题以国际象棋棋盘为背景,要求我们判断给定的两个方格颜色是否相同。通过解决这道题,
【Python LeetCode 专题】热题 100,重在思路
一杯水果茶!
人生苦短我用Pythonpythonleetcode
哈希1.两数之和49.字母异位词分组128.最长连续序列双指针283.移动零11.盛最多水的容器15.三数之和42.接雨水滑动窗口3.无重复字符的最长子串438.找到字符串中所有字母异位词子串560.和为K的子数组239.滑动窗口最大值普通数组53.最大子数组和56.合并区间189.轮转数组238.除自身以外数组的乘积矩阵73.矩阵置零链表160.相交链表206.反转链表234.回文链表141.环
JAVA刷题记录: 专题十五 BFS解决FloodFill算法
用屁屁笑
宽度优先算法
733.图像渲染-力扣(LeetCode)classSolution{int[]dx={0,0,-1,1};int[]dy={1,-1,0,0};publicint[][]floodFill(int[][]image,intsr,intsc,intcolor){intprev=image[sr][sc];if(color==prev)returnimage;Queueq=newLinkedList
BFS-FloodFill 算法 解决最短路问题 多源 解决拓扑排序
penguin_bark
#BFS算法宽度优先leetcode
文章目录一、FloodFill算法[733.图像渲染](https://leetcode.cn/problems/flood-fill/description/)2.思路3.代码[200.岛屿数量](https://leetcode.cn/problems/number-of-islands/description/)2.思路3.代码[LCR105.岛屿的最大面积](https://leetcod
LeetCode 72. 编辑距离(Edit Distance)| 动态规划详解
72.编辑距离题目描述给你两个单词word1和word2,请计算将word1转换为word2所需的最少操作数。你可以对一个单词进行以下三种操作:插入一个字符删除一个字符替换一个字符✅示例输入:word1="horse",word2="ros"输出:3解释:horse->rorse(替换h为r)rorse->rose(删除r)rose->ros(删除e)解题思路:动态规划(DP)✅状态定义dp[i]
Leetcode703. 数据流中的第K大元素
LonnieQ
题目设计一个找到数据流中第K大元素的类(class)。注意是排序后的第K大元素,不是第K个不同的元素。你的KthLargest类需要一个同时接收整数k和整数数组nums的构造器,它包含数据流中的初始元素。每次调用KthLargest.add,返回当前数据流中第K大的元素。示例:intk=3;int[]arr=[4,5,8,2];KthLargestkthLargest=newKthLargest(
1948. 删除系统中的重复文件夹
追逐此刻
力扣pythonlinux开发语言
1948.删除系统中的重复文件夹-力扣(LeetCode)classTrieNode:__slots__='son','name','deleted'def__init__(self):self.son={}self.name=''self.deleted=FalseclassSolution:defdeleteDuplicateFolder(self,paths:List[List[str]])
LeetCode第337题_打家劫舍III
@蓝莓果粒茶
算法leetcode算法职场和发展c#学习
LeetCode第337题:打家劫舍III文章摘要本文详细解析LeetCode第337题"打家劫舍III",这是一道中等难度的二叉树动态规划问题。文章提供了基于深度优先搜索和动态规划的解法,包含C#、Python、C++三种语言实现,配有详细的算法分析和性能对比。适合想要提升二叉树和动态规划能力的程序员。核心知识点:二叉树、动态规划、深度优先搜索难度等级:中等推荐人群:具有基础数据结构知识,想要提
apache 安装linux windows
墙头上一根草
apacheinuxwindows
linux安装Apache 有两种方式一种是手动安装通过二进制的文件进行安装,另外一种就是通过yum 安装,此中安装方式,需要物理机联网。以下分别介绍两种的安装方式
通过二进制文件安装Apache需要的软件有apr,apr-util,pcre
1,安装 apr 下载地址:htt
fill_parent、wrap_content和match_parent的区别
Cb123456
match_parentfill_parent
fill_parent、wrap_content和match_parent的区别:
1)fill_parent
设置一个构件的布局为fill_parent将强制性地使构件扩展,以填充布局单元内尽可能多的空间。这跟Windows控件的dockstyle属性大体一致。设置一个顶部布局或控件为fill_parent将强制性让它布满整个屏幕。
2) wrap_conte
网页自适应设计
天子之骄
htmlcss响应式设计页面自适应
网页自适应设计
网页对浏览器窗口的自适应支持变得越来越重要了。自适应响应设计更是异常火爆。再加上移动端的崛起,更是如日中天。以前为了适应不同屏幕分布率和浏览器窗口的扩大和缩小,需要设计几套css样式,用js脚本判断窗口大小,选择加载。结构臃肿,加载负担较大。现笔者经过一定时间的学习,有所心得,故分享于此,加强交流,共同进步。同时希望对大家有所
[sql server] 分组取最大最小常用sql
一炮送你回车库
SQL Server
--分组取最大最小常用sql--测试环境if OBJECT_ID('tb') is not null drop table tb;gocreate table tb( col1 int, col2 int, Fcount int)insert into tbselect 11,20,1 union allselect 11,22,1 union allselect 1
ImageIO写图片输出到硬盘
3213213333332132
javaimage
package awt;
import java.awt.Color;
import java.awt.Font;
import java.awt.Graphics;
import java.awt.image.BufferedImage;
import java.io.File;
import java.io.IOException;
import javax.imagei
自己的String动态数组
宝剑锋梅花香
java动态数组数组
数组还是好说,学过一两门编程语言的就知道,需要注意的是数组声明时需要把大小给它定下来,比如声明一个字符串类型的数组:String str[]=new String[10]; 但是问题就来了,每次都是大小确定的数组,我需要数组大小不固定随时变化怎么办呢? 动态数组就这样应运而生,龙哥给我们讲的是自己用代码写动态数组,并非用的ArrayList 看看字符
pinyin4j工具类
darkranger
.net
pinyin4j工具类Java工具类 2010-04-24 00:47:00 阅读69 评论0 字号:大中小
引入pinyin4j-2.5.0.jar包:
pinyin4j是一个功能强悍的汉语拼音工具包,主要是从汉语获取各种格式和需求的拼音,功能强悍,下面看看如何使用pinyin4j。
本人以前用AscII编码提取工具,效果不理想,现在用pinyin4j简单实现了一个。功能还不是很完美,
StarUML学习笔记----基本概念
aijuans
UML建模
介绍StarUML的基本概念,这些都是有效运用StarUML?所需要的。包括对模型、视图、图、项目、单元、方法、框架、模型块及其差异以及UML轮廓。
模型、视与图(Model, View and Diagram)
&
Activiti最终总结
avords
Activiti id 工作流
1、流程定义ID:ProcessDefinitionId,当定义一个流程就会产生。
2、流程实例ID:ProcessInstanceId,当开始一个具体的流程时就会产生,也就是不同的流程实例ID可能有相同的流程定义ID。
3、TaskId,每一个userTask都会有一个Id这个是存在于流程实例上的。
4、TaskDefinitionKey和(ActivityImpl activityId
从省市区多重级联想到的,react和jquery的差别
bee1314
jqueryUIreact
在我们的前端项目里经常会用到级联的select,比如省市区这样。通常这种级联大多是动态的。比如先加载了省,点击省加载市,点击市加载区。然后数据通常ajax返回。如果没有数据则说明到了叶子节点。 针对这种场景,如果我们使用jquery来实现,要考虑很多的问题,数据部分,以及大量的dom操作。比如这个页面上显示了某个区,这时候我切换省,要把市重新初始化数据,然后区域的部分要从页面
Eclipse快捷键大全
bijian1013
javaeclipse快捷键
Ctrl+1 快速修复(最经典的快捷键,就不用多说了)Ctrl+D: 删除当前行 Ctrl+Alt+↓ 复制当前行到下一行(复制增加)Ctrl+Alt+↑ 复制当前行到上一行(复制增加)Alt+↓ 当前行和下面一行交互位置(特别实用,可以省去先剪切,再粘贴了)Alt+↑ 当前行和上面一行交互位置(同上)Alt+← 前一个编辑的页面Alt+→ 下一个编辑的页面(当然是针对上面那条来说了)Alt+En
js 笔记 函数
征客丶
JavaScript
一、函数的使用
1.1、定义函数变量
var vName = funcation(params){
}
1.2、函数的调用
函数变量的调用: vName(params);
函数定义时自发调用:(function(params){})(params);
1.3、函数中变量赋值
var a = 'a';
var ff
【Scala四】分析Spark源代码总结的Scala语法二
bit1129
scala
1. Some操作
在下面的代码中,使用了Some操作:if (self.partitioner == Some(partitioner)),那么Some(partitioner)表示什么含义?首先partitioner是方法combineByKey传入的变量,
Some的文档说明:
/** Class `Some[A]` represents existin
java 匿名内部类
BlueSkator
java匿名内部类
组合优先于继承
Java的匿名类,就是提供了一个快捷方便的手段,令继承关系可以方便地变成组合关系
继承只有一个时候才能用,当你要求子类的实例可以替代父类实例的位置时才可以用继承。
在Java中内部类主要分为成员内部类、局部内部类、匿名内部类、静态内部类。
内部类不是很好理解,但说白了其实也就是一个类中还包含着另外一个类如同一个人是由大脑、肢体、器官等身体结果组成,而内部类相
盗版win装在MAC有害发热,苹果的东西不值得买,win应该不用
ljy325
游戏applewindowsXPOS
Mac mini 型号: MC270CH-A RMB:5,688
Apple 对windows的产品支持不好,有以下问题:
1.装完了xp,发现机身很热虽然没有运行任何程序!貌似显卡跑游戏发热一样,按照那样的发热量,那部机子损耗很大,使用寿命受到严重的影响!
2.反观安装了Mac os的展示机,发热量很小,运行了1天温度也没有那么高
&nbs
读《研磨设计模式》-代码笔记-生成器模式-Builder
bylijinnan
java设计模式
声明: 本文只为方便我个人查阅和理解,详细的分析以及源代码请移步 原作者的博客http://chjavach.iteye.com/
/**
* 生成器模式的意图在于将一个复杂的构建与其表示相分离,使得同样的构建过程可以创建不同的表示(GoF)
* 个人理解:
* 构建一个复杂的对象,对于创建者(Builder)来说,一是要有数据来源(rawData),二是要返回构
JIRA与SVN插件安装
chenyu19891124
SVNjira
JIRA安装好后提交代码并要显示在JIRA上,这得需要用SVN的插件才能看见开发人员提交的代码。
1.下载svn与jira插件安装包,解压后在安装包(atlassian-jira-subversion-plugin-0.10.1)
2.解压出来的包里下的lib文件夹下的jar拷贝到(C:\Program Files\Atlassian\JIRA 4.3.4\atlassian-jira\WEB
常用数学思想方法
comsci
工作
对于搞工程和技术的朋友来讲,在工作中常常遇到一些实际问题,而采用常规的思维方式无法很好的解决这些问题,那么这个时候我们就需要用数学语言和数学工具,而使用数学工具的前提却是用数学思想的方法来描述问题。。下面转帖几种常用的数学思想方法,仅供学习和参考
函数思想
把某一数学问题用函数表示出来,并且利用函数探究这个问题的一般规律。这是最基本、最常用的数学方法
pl/sql集合类型
daizj
oracle集合typepl/sql
--集合类型
/*
单行单列的数据,使用标量变量
单行多列数据,使用记录
单列多行数据,使用集合(。。。)
*集合:类似于数组也就是。pl/sql集合类型包括索引表(pl/sql table)、嵌套表(Nested Table)、变长数组(VARRAY)等
*/
/*
--集合方法
&n
[Ofbiz]ofbiz初用
dinguangx
电商ofbiz
从github下载最新的ofbiz(截止2015-7-13),从源码进行ofbiz的试用
1. 加载测试库
ofbiz内置derby,通过下面的命令初始化测试库
./ant load-demo (与load-seed有一些区别)
2. 启动内置tomcat
./ant start
或
./startofbiz.sh
或
java -jar ofbiz.jar
&
结构体中最后一个元素是长度为0的数组
dcj3sjt126com
cgcc
在Linux源代码中,有很多的结构体最后都定义了一个元素个数为0个的数组,如/usr/include/linux/if_pppox.h中有这样一个结构体: struct pppoe_tag { __u16 tag_type; __u16 tag_len; &n
Linux cp 实现强行覆盖
dcj3sjt126com
linux
发现在Fedora 10 /ubutun 里面用cp -fr src dest,即使加了-f也是不能强行覆盖的,这时怎么回事的呢?一两个文件还好说,就输几个yes吧,但是要是n多文件怎么办,那还不输死人呢?下面提供三种解决办法。 方法一
我们输入alias命令,看看系统给cp起了一个什么别名。
[root@localhost ~]# aliasalias cp=’cp -i’a
Memcached(一)、HelloWorld
frank1234
memcached
一、简介
高性能的架构离不开缓存,分布式缓存中的佼佼者当属memcached,它通过客户端将不同的key hash到不同的memcached服务器中,而获取的时候也到相同的服务器中获取,由于不需要做集群同步,也就省去了集群间同步的开销和延迟,所以它相对于ehcache等缓存来说能更好的支持分布式应用,具有更强的横向伸缩能力。
二、客户端
选择一个memcached客户端,我这里用的是memc
Search in Rotated Sorted Array II
hcx2013
search
Follow up for "Search in Rotated Sorted Array":What if duplicates are allowed?
Would this affect the run-time complexity? How and why?
Write a function to determine if a given ta
Spring4新特性——更好的Java泛型操作API
jinnianshilongnian
spring4generic type
Spring4新特性——泛型限定式依赖注入
Spring4新特性——核心容器的其他改进
Spring4新特性——Web开发的增强
Spring4新特性——集成Bean Validation 1.1(JSR-349)到SpringMVC
Spring4新特性——Groovy Bean定义DSL
Spring4新特性——更好的Java泛型操作API
Spring4新
CentOS安装JDK
liuxingguome
centos
1、行卸载原来的:
[root@localhost opt]# rpm -qa | grep java
tzdata-java-2014g-1.el6.noarch
java-1.7.0-openjdk-1.7.0.65-2.5.1.2.el6_5.x86_64
java-1.6.0-openjdk-1.6.0.0-11.1.13.4.el6.x86_64
[root@localhost
二分搜索专题2-在有序二维数组中搜索一个元素
OpenMind
二维数组算法二分搜索
1,设二维数组p的每行每列都按照下标递增的顺序递增。
用数学语言描述如下:p满足
(1),对任意的x1,x2,y,如果x1<x2,则p(x1,y)<p(x2,y);
(2),对任意的x,y1,y2, 如果y1<y2,则p(x,y1)<p(x,y2);
2,问题:
给定满足1的数组p和一个整数k,求是否存在x0,y0使得p(x0,y0)=k?
3,算法分析:
(
java 随机数 Math与Random
SaraWon
javaMathRandom
今天需要在程序中产生随机数,知道有两种方法可以使用,但是使用Math和Random的区别还不是特别清楚,看到一篇文章是关于的,觉得写的还挺不错的,原文地址是
http://www.oschina.net/question/157182_45274?sort=default&p=1#answers
产生1到10之间的随机数的两种实现方式:
//Math
Math.roun
oracle创建表空间
tugn
oracle
create temporary tablespace TXSJ_TEMP
tempfile 'E:\Oracle\oradata\TXSJ_TEMP.dbf'
size 32m
autoextend on
next 32m maxsize 2048m
extent m
使用Java8实现自己的个性化搜索引擎
yangshangchuan
javasuperword搜索引擎java8全文检索
需要对249本软件著作实现句子级别全文检索,这些著作均为PDF文件,不使用现有的框架如lucene,自己实现的方法如下:
1、从PDF文件中提取文本,这里的重点是如何最大可能地还原文本。提取之后的文本,一个句子一行保存为文本文件。
2、将所有文本文件合并为一个单一的文本文件,这样,每一个句子就有一个唯一行号。
3、对每一行文本进行分词,建立倒排表,倒排表的格式为:词=包含该词的总行数N=行号