【算法题解】22. 接雨水
这是一道 困难 题
题目来自: https://leetcode.cn/problems/trapping-rain-water/
题目
给定 n 个非负整数表示每个宽度为 1 的柱子的高度图,计算按此排列的柱子,下雨之后能接多少雨水。
示例 1:
输入:height = [0,1,0,2,1,0,1,3,2,1,2,1]
输出:6
解释:上面是由数组 [0,1,0,2,1,0,1,3,2,1,2,1] 表示的高度图,在这种情况下,可以接 6 个单位的雨水(蓝色部分表示雨水)。
示例 2:
输入:height = [4,2,0,3,2,5]
输出:9
提示:
- n = = h e i g h t . l e n g t h n == height.length n==height.length
- 1 < = n < = 2 ∗ 1 0 4 1 <= n <= 2 * 10^4 1<=n<=2∗104
- 0 < = h e i g h t [ i ] < = 1 0 5 0 <= height[i] <= 10^5 0<=height[i]<=105
解题思路
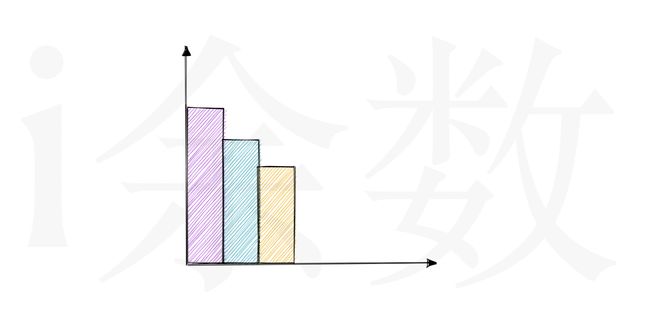
使用单调栈的思路,假如给定的每个柱子是逐个变矮的,那么可以接的雨水就是0。
如果这时候突然来了一个变高的柱子 4 ,那么这个柱子就会使得当前柱状图可以接雨水了。
如上图所示:
- 先将栈顶的柱子取出(柱子3),使用(其左边柱子的高度和新增柱子高度的最小值 - 其本身高度)* 其宽度,得出当前柱子的接水量,累加到答案中。(注意:如果栈中目前只有一个柱子的话,其左边高度设为
0,即不可以接水。) - 这时如果新的栈顶柱子(柱子2)依然比新增的柱子矮,继续取出栈顶柱子并计算其接水量,注意这是宽度应该是柱子3的宽度 + 柱子2的宽度。
- 这时栈顶柱子1的高度 大于 新增的柱子4 的高度,柱子4入栈,注意:这时柱子4的宽度 = 柱子2 + 柱子3 + 柱子4 的总宽度,即前面所有出栈的柱子的宽度都需要保留下来,因为如果再来一个第5个柱子的高度 > 柱子4的高度的话,前面那么宽度还是有用的。
代码实现
Java 代码实现
class Solution {
private Deque<int[]> stack = new LinkedList<>();
public int trap(int[] height) {
int n = height.length ;
if(n <= 1){
return 0;
}
int ans = 0;
for(int h : height){
int w = 0;
while(!stack.isEmpty() && h > stack.peek()[1]){
int[] top = stack.pop();
w += top[0];
if(!stack.isEmpty()){
ans += w * (Math.min(stack.peek()[1], h) - top[1]);
}
}
stack.push(new int[]{w + 1, h});
}
return ans;
}
}
Go 代码实现
func trap(height []int) int {
n := len(height)
if n <= 1 {
return 0
}
stack := [][]int{}
ans := 0
for _, h := range height {
if len(stack) == 0 {
stack = append(stack, []int{1, h})
continue
}
w := 0
for len(stack) > 0 && h > stack[len(stack) - 1][1] {
top := stack[len(stack) - 1]
stack = stack[:len(stack) - 1]
w += top[0]
if len(stack) > 0 {
ans += w * (min(stack[len(stack) - 1][1], h) - top[1])
}
}
stack = append(stack, []int{w + 1, h})
}
return ans
}
func min(a int, b int) int {
if a > b {
return b
}
return a
}
复杂度分析
时间复杂度: O ( n ) O(n) O(n)
空间复杂度: O ( n ) O(n) O(n)