栈（stack）入门详解之C语言版

一、栈

1）基本概念
栈(Stack)：是一种受限的线性表，即限制在表的一端进行插入和删除操作。栈也称为后进先出LIFO (Last In First Out)或先进后出FILO (First In Last Out)线性表。
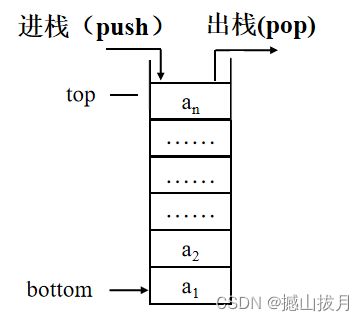
栈顶(top)：允许进行插入、删除操作的一端称为栈的栈顶(top)，也称为表尾。
栈底（bottom）：固定不动的一端，称为栈底（bottom），也称为表头。
2）栈的示意图如下：

3）进栈和出栈的示意图：

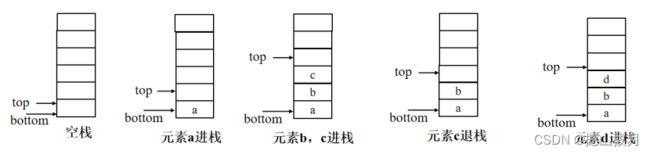
当元素进栈时，栈顶指针指向第一个为空的元素地址。
当元素出栈时，栈顶指针向下移动一个存储单元。
4）进栈和出栈序列
如果序列{1,2,3}依次进栈，则出栈的全部可能序列为：
{3,2,1}、
{2,3,1}、{2,1,3}
{1,2,3}、{1,3,2}
当进栈序列为1,2,…,n时，出栈序列的个数为尤.卡塔南数：

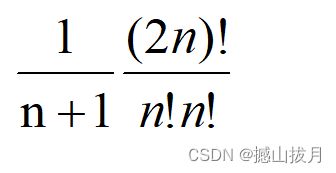
二、动态栈的基本操作

1、栈的类型定义：

typedef  int  ElemType ;
typedef struct stack
{   
      ElemType  *bottom;   //栈底指针 
      ElemType  *top;      //栈顶指针 
      int   stacksize ;       //当前栈的容量 
}Stack ;

2、栈的初始化
假设初始时给栈分配存储空间大小为STACK_SIZE，栈的容量也赋值为STACK_SIZE。
栈初始化算法的主要过程：
1）为栈分配存储空间（也就是给栈底指针分配空间，这是因为栈底是固定不动的）；
2）让栈顶指针指向栈底（表示栈是空的）。

Status Init_Stack( Stack  &S  )
{
	S.bottom=(ElemType *)malloc(STACK_SIZE *sizeof(ElemType));
	if ( !S.bottom ) //判断分配空间是否成功，如果失败则返回错误
	      return  ERROR;
	S.top = S.bottom ;    //初始化时让栈顶指针指向栈底
	S. stacksize = STACK_SIZE; //初始化时
	return OK ;
}//Init_Stack

3、进栈（也称压栈、入栈）
元素进栈算法的主要过程：
1）首先判断栈的容量是否已经达到最大，如果达到，则追加存储空间，并重新定位栈顶指针的位置（其实就是指向实际的栈顶），然后更新栈的容量；
2）把入栈元素存入栈顶；
3）栈顶指针指向新栈顶。

Status Push(Stack &S , ElemType  e)
{
	//如果栈满，则使用realloc追加存储空间
	if( S.top-S.bottom >= S. stacksize-1 )      
    {
		S.bottom=(ElemType *)realloc( S.bottom, (S.stacksize+STACK_SIZE) * sizeof(ElemType));  
        if( !S.bottom )//如果重新分配存储空间失败，则返回错误     
            return  ERROR; 
		S.top = S.bottom + S.stacksize;//重新定位栈顶 
		S.stacksize += STACKINCREMENT;//更新栈的容量 
	}
	*S.top = e;//把元素存入栈顶  
	S.top++; //栈顶指针加1（即向上移动一个存储单元），e成为新的栈顶   
	return OK;
}//Push

4、出栈（也称弹栈）
元素出栈算法的主要过程：
1）判断栈是否是控制，如果是则返回出栈失败；
2）栈顶指针下移一个存储单元；
3）把栈顶指针指向位置的元素存入到目标变量中。

Status Pop( Stack  &S, ElemType  &e )      
{   
	if( S.top == S.bottom ) //栈空，返回失败  
		return ERROR ;  
	S.top--;//栈顶下移
	e = *S.top;//用e缓存栈顶元素
	return  OK; 
}// Pop 

5、取栈顶元素
取栈顶元素的主要步骤：
1）判断栈是否为空，为空则返回失败
2）取栈顶元素并存储到相应的变量中，需要注意的是栈顶元素实际存储在栈顶指针的下一个存储空间中。

Status GetTopElement( Stack S, ElemType  &e )      
{   
	if ( S.top == S.bottom ) //如果栈为空，则返回失败
		return ERROR ;       
	e = *(S. top – 1) ;      //用e缓存栈顶元素
	return  OK ; 
}//GetTopElement

6、把栈置为空栈
把栈置为空栈的主要步骤：
1）把栈顶指针指向栈底即可。

Status ClearStack( Stack &S )
{   
	S.top = S.bottom; 
	return  OK ; 
}// ClearStack

7、销毁栈
销毁栈的主要步骤：
1）释放栈底指针（因为栈的存储空间是通过给栈底指针分配空间得到的）
2）把栈底指针和栈顶指针均赋值为NULL
3）把栈的容量赋值为0

Status DestroyStack( Stack &S )      
{   
	free( S.bottom );
	S.top =  NULL;
	S.bottom  = NULL;
	S. stacksize = 0;
	return  OK ; 
}// DestroyStack

三、利用动态栈实现进制转换
问题描述：将十进制整数n分别转换为16进制数、8进制数、2进制数。
算法主要步骤（ base表示进制）：
1、初始化栈
2、当n大于0时：
1）求n对base求余数
2）将余数压入栈
3）更新n为n/base
3、当栈非空时：
1）栈顶元素出栈
2）输出栈顶元素
对应的代码：

 void BaseConversion( unsigned n, unsigned base ) 
{
	Stack S;
	int k, e;
	InitStack( S );
	while( n > 0 )
	{
		k = n % base;
		Push( S, k );
		n = n / base;
	}
	while( S.top != S.bottom )
	{
		Pop( S, e );
		char format[3]={ '%' };
		if( base == 16 ) 
		{
			format[1] = 'x';
		}
		else
		{
			format[1] = 'u';
		}
		printf( format, e );
	}
	free( S.bottom );
}

完整的测试代码：

//利用栈实现进制转换
#include"stdio.h"
#include"malloc.h"
#include"String.h"
#define OK    0
#define ERROR 1
#define STACKSIZE 100
#define STACKINCREMENT 10
typedef int Status;
typedef int Etype;
typedef struct stack
{   
      Etype  *bottom;   //栈底指针 
      Etype  *top;      //栈顶指针 
      int    stacksize; //当前栈的容量 
}Stack; 

Status InitStack( Stack &S );
Status Push( Stack &S , Etype e );
Status Pop( Stack  &S, Etype  &e );
void BaseConversion( unsigned n, unsigned base );

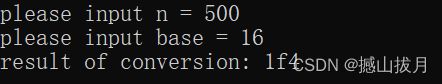
int main()
{
	unsigned n, base;
	printf( "please input n = " );
	scanf( "%ud", &n );
	printf( "please input base = " );
	scanf( "%ud", &base );
	printf( "result of conversion: " );
	BaseConversion( n, base );
}

//栈的初始化 
Status InitStack( Stack &S )
{
	S.bottom=(Etype *)malloc(STACKSIZE *sizeof(Etype));
	if ( !S.bottom ) //判断分配空间是否成功，如果失败则返回错误
	{
		return  ERROR;
	}
	S.top = S.bottom ;    //初始化时让栈顶指针指向栈底
	S.stacksize = STACKSIZE; //初始化时
	return OK ;
}//InitStack
//压栈（进栈、入栈） 
Status Push( Stack &S , Etype e )
{
	//如果栈满，则使用realloc追加存储空间
	if( S.top - S.bottom >= S.stacksize-1 )      
    {
		S.bottom=(Etype *)realloc( S.bottom, (S.stacksize+STACKSIZE) * sizeof(Etype) );  
        if( !S.bottom )//如果重新分配存储空间失败，则返回错误     
        {
        	return  ERROR; 
		}
		S.top = S.bottom + S.stacksize;//重新定位栈顶 
		S.stacksize += STACKINCREMENT;//更新栈的容量 
	}
	*S.top = e;//把元素存入栈顶  
	S.top++; //栈顶指针加1（即向上移动一个存储单元），e成为新的栈顶   
	return OK;
}//Push

Status Pop( Stack  &S, Etype  &e )      
{   
	if( S.top == S.bottom ) //栈空，返回失败  
	{
		return ERROR; 
	}	
	S.top--;   //栈顶下移
	e = *S.top;//用e缓存栈顶元素
	return  OK; 
}// Pop 

void BaseConversion( unsigned n, unsigned base ) 
{
	Stack S;
	int k, e;
	InitStack( S );
	while( n > 0 )
	{
		k = n % base;
		Push( S, k );
		n = n / base;
	}
	while( S.top != S.bottom )
	{
		Pop( S, e );
		char format[3]={ '%' };
		if( base == 16 ) 
		{
			format[1] = 'x';
		}
		else
		{
			format[1] = 'u';
		}
		printf( format, e );
	}
	free( S.bottom );
}

运行结果：