二叉树题目:从前序遍历还原二叉树
文章目录
- 题目
-
- 标题和出处
- 难度
- 题目描述
-
- 要求
- 示例
- 数据范围
- 解法
-
- 思路和算法
- 代码
- 复杂度分析
题目
标题和出处
标题:从前序遍历还原二叉树
出处:1028. 从前序遍历还原二叉树
难度
6 级
题目描述
要求
我们从二叉树的根结点 root \texttt{root} root 开始深度优先搜索。
在遍历中的每个结点处,我们输出 D \texttt{D} D 条短划线(其中 D \texttt{D} D 是该结点的深度),然后输出该结点的值。如果结点的深度为 D \texttt{D} D,则其子结点的深度为 D + 1 \texttt{D + 1} D + 1。根结点的深度为 0 \texttt{0} 0。
如果结点只有一个子结点,那么保证该子结点为左子结点。
给出遍历的输出 traversal \texttt{traversal} traversal,还原树并返回其根结点 root \texttt{root} root。
示例
示例 1:
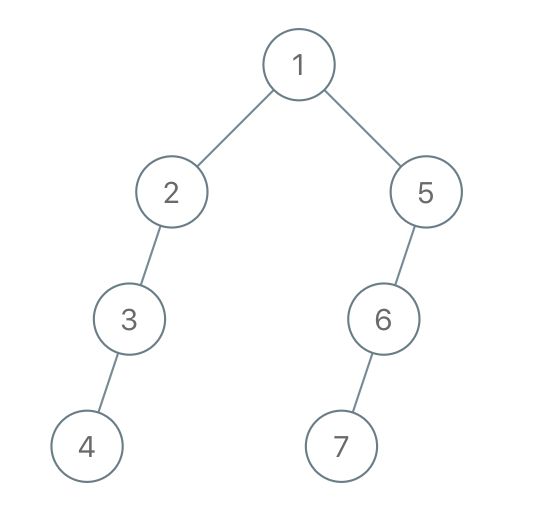
输入: traversal = "1-2--3--4-5--6--7" \texttt{traversal = "1-2--3--4-5--6--7"} traversal = "1-2--3--4-5--6--7"
输出: [1,2,5,3,4,6,7] \texttt{[1,2,5,3,4,6,7]} [1,2,5,3,4,6,7]
示例 2:
输入: traversal = "1-2--3---4-5--6---7" \texttt{traversal = "1-2--3---4-5--6---7"} traversal = "1-2--3---4-5--6---7"
输出: [1,2,5,3,null,6,null,4,null,7] \texttt{[1,2,5,3,null,6,null,4,null,7]} [1,2,5,3,null,6,null,4,null,7]
示例 3:
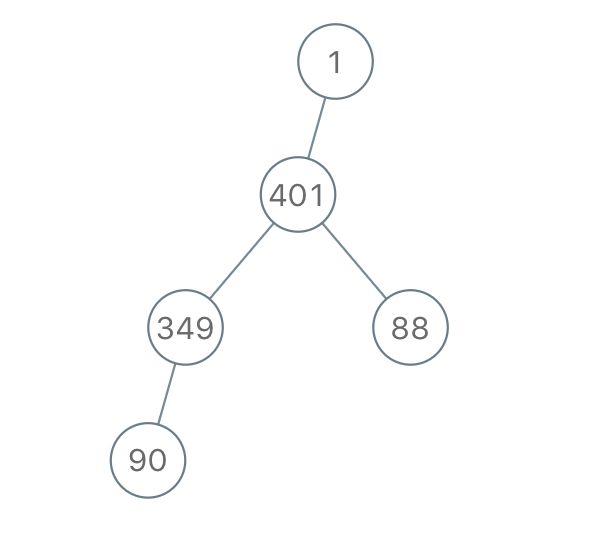
输入: traversal = "1-401--349---90--88" \texttt{traversal = "1-401--349---90--88"} traversal = "1-401--349---90--88"
输出: [1,401,null,349,88,90] \texttt{[1,401,null,349,88,90]} [1,401,null,349,88,90]
数据范围
- 树中结点数目在范围 [1, 1000] \texttt{[1, 1000]} [1, 1000] 内
- 1 ≤ Node.val ≤ 10 9 \texttt{1} \le \texttt{Node.val} \le \texttt{10}^\texttt{9} 1≤Node.val≤109
解法
思路和算法
给定的字符串包含每个结点的值和结点所在深度。根结点所在深度为 0 0 0,其余结点所在深度都大于 0 0 0。
由于给定的字符串是二叉树的前序遍历序列,因此对于遍历到的每个结点,如果其层数为 depth \textit{depth} depth( depth > 0 \textit{depth} > 0 depth>0),其父结点一定是已经遍历的结点中的最后一个访问过的层数为 depth − 1 \textit{depth} - 1 depth−1 的结点。如果父结点的左子结点为空,则当前结点作为父结点的左子结点,否则当前结点作为父结点的右子结点。
为了定位到最后一个访问过的上一层结点,需要使用栈存储结点。栈内结点从栈底到栈顶的深度依次递增,根结点位于栈底。
首先从给定的字符串中得到根结点值,创建根结点,并将根结点入栈。继续遍历字符串的其余部分,对于每个结点,执行如下操作。
-
根据短划线数量得到结点所在深度 depth \textit{depth} depth,根据短划线后的数值得到结点值 val \textit{val} val,使用结点值 val \textit{val} val 创建当前结点。
-
当前结点的父结点的深度为 depth − 1 \textit{depth} - 1 depth−1。如果栈内元素个数大于 depth \textit{depth} depth,则栈顶结点的深度与父结点的深度不同,因此将栈顶结点出栈。重复出栈操作直到栈内元素个数等于 depth \textit{depth} depth,此时栈顶结点的深度为 depth − 1 \textit{depth} - 1 depth−1。
-
此时栈顶结点为当前结点的父结点。判断父结点的左子结点是否为空,如果父结点的左子结点为空则将当前结点设为父结点的左子结点,否则将当前结点设为父结点的右子结点。
-
将当前结点入栈。
重复上述操作,直到字符串遍历结束。遍历结束之后返回根结点,即为还原的二叉树。
以下是示例 1 的计算过程。
-
创建根结点 1 1 1,深度为 0 0 0。将结点 1 1 1 入栈, stack = [ 1 ] \textit{stack} = [1] stack=[1],其中左边为栈底,右边为栈顶,栈内元素为结点,此处用数字表示结点且省略父结点和子结点的关系。
-
创建结点 2 2 2,深度为 1 1 1。由于栈内元素个数等于 1 1 1,因此将结点 2 2 2 作为栈顶结点 1 1 1 的左子结点,将结点 2 2 2 入栈, stack = [ 1 , 2 ] \textit{stack} = [1, 2] stack=[1,2]。
-
创建结点 3 3 3,深度为 2 2 2。由于栈内元素个数等于 2 2 2,因此将结点 3 3 3 作为栈顶结点 2 2 2 的左子结点,将结点 3 3 3 入栈, stack = [ 1 , 2 , 3 ] \textit{stack} = [1, 2, 3] stack=[1,2,3]。
-
创建结点 4 4 4,深度为 2 2 2。由于栈内元素个数大于 2 2 2,因此将结点 3 3 3 出栈,此时栈内元素个数等于 2 2 2,将结点 4 4 4 作为栈顶结点 2 2 2 的右子结点,将结点 4 4 4 入栈, stack = [ 1 , 2 , 4 ] \textit{stack} = [1, 2, 4] stack=[1,2,4]。
-
创建结点 5 5 5,深度为 1 1 1。由于栈内元素个数大于 1 1 1,因此将结点 4 4 4 和 2 2 2 出栈,此时栈内元素个数等于 1 1 1,将结点 5 5 5 作为栈顶结点 1 1 1 的右子结点,将结点 5 5 5 入栈, stack = [ 1 , 5 ] \textit{stack} = [1, 5] stack=[1,5]。
-
创建结点 6 6 6,深度为 2 2 2。由于栈内元素个数等于 2 2 2,因此将结点 6 6 6 作为栈顶结点 5 5 5 的左子结点,将结点 6 6 6 入栈, stack = [ 1 , 5 , 6 ] \textit{stack} = [1, 5, 6] stack=[1,5,6]。
-
创建结点 7 7 7,深度为 2 2 2。由于栈内元素个数大于 2 2 2,因此将结点 6 6 6 出栈,此时栈内元素个数等于 2 2 2,将结点 7 7 7 作为栈顶结点 5 5 5 的右子结点,将结点 7 7 7 入栈, stack = [ 1 , 5 , 7 ] \textit{stack} = [1, 5, 7] stack=[1,5,7]。
-
遍历结束,返回根结点 1 1 1。
代码
class Solution {
public TreeNode recoverFromPreorder(String traversal) {
Deque<TreeNode> stack = new ArrayDeque<TreeNode>();
int rootVal = 0;
int length = traversal.length();
int index = 0;
while (index < length && Character.isDigit(traversal.charAt(index))) {
rootVal = rootVal * 10 + traversal.charAt(index) - '0';
index++;
}
TreeNode root = new TreeNode(rootVal);
stack.push(root);
while (index < length) {
int depth = 0;
while (traversal.charAt(index) == '-') {
depth++;
index++;
}
int val = 0;
while (index < length && Character.isDigit(traversal.charAt(index))) {
val = val * 10 + traversal.charAt(index) - '0';
index++;
}
TreeNode node = new TreeNode(val);
while (stack.size() > depth) {
stack.pop();
}
TreeNode parent = stack.peek();
if (parent.left == null) {
parent.left = node;
} else {
parent.right = node;
}
stack.push(node);
}
return root;
}
}
复杂度分析
-
时间复杂度: O ( m ) O(m) O(m),其中 m m m 是字符串 traversal \textit{traversal} traversal 的长度。需要遍历字符串一次还原二叉树,对于二叉树中的每个结点,最多入栈和出栈各一次。由于二叉树的结点数 n n n 一定不超过字符串的长度 m m m,因此总时间复杂度是 O ( m ) O(m) O(m)。
-
空间复杂度: O ( n ) O(n) O(n),其中 n n n 是二叉树的结点数。空间复杂度主要是栈空间,取决于二叉树的高度,最坏情况下二叉树的高度是 O ( n ) O(n) O(n)。