《堆排序》与《Top—k》
目录
编辑
前言:
关于《堆排序》:
第一步:建堆
第二步:排序
《Top—K问题》
关于Top—k问题:
前言:
我们在前面的blog中,对于《堆》已经有了初步的概念,那么接下来我们可以利用《堆》来解决我们日常生活中存在的问题,本篇我们给出两个常用的应用场景,分别是《排序》以及《Top—k问题》,上一篇blog在:《堆》的模拟实现-CSDN博客
关于《堆排序》:
#define _CRT_SECURE_NO_WARNINGS 1
#include
void swap(int* a, int* b)
{
int tmp = *a;
*a = *b;
*b = tmp;
}
void AdjustDown(int* arr, int sz, int parent)
{
int child = parent * 2 + 1;
while (child < sz)
{
if (child + 1 < sz && arr[child] < arr[child + 1])
{
child++;
}
if (arr[child] > arr[parent])
{
swap(&arr[child], &arr[parent]);
parent = child;
child = 2 * parent + 1;
}
else
{
break;
}
}
}
void AdjustUp(int* arr, int sz, int child)
{
while (child > 0)
{
int parent = (child - 1) / 2;
if (arr[parent] < arr[child])
{
swap(&arr[parent], &arr[child]);
}
child = parent;
}
}
int main()
{
int arr[] = { 2, 6, 9, 3, 1, 7 };
int sz = sizeof(arr) / sizeof(arr[0]);
for (int i = (sz - 1 - 1) / 2; i >= 0; i--)
{
AdjustDown(arr, sz, i);
}//向下调整算法
//for (int i = 1; i 0)
{
swap(&arr[0], &arr[end]);
AdjustDown(arr, end, 0);
--end;
}
return 0;
} 第一步:建堆
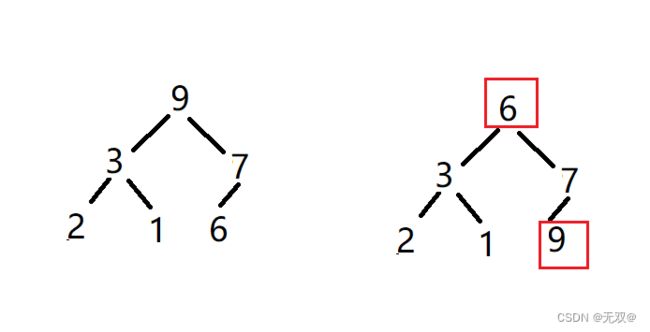
利用《堆》可以方便我们对一个给定的乱序数组实现排序,首先我们应当选择大堆来进行排序操作。
为什么我们不选择使用小堆来进行建堆呢?
通过之前对《堆》的blog说明,小堆就是对顶元素为最小元素,其他的节点数都比第一个元素小,那么如果是小堆,最小的数字已经就是第一个元素,若要找出次小的元素,则又需要在剩下的元素中再进行建堆,重复循环才能完成排序,这样子的时间复杂度高,不利于排序。
因此我们选择利用大堆来建堆,实现大堆后,再将首尾的元素进行交换,再利用向下调整法调整法对剩下的n-1个元素进行调整,再进行交换,如此能实现排序。
int arr[] = { 2, 6, 9, 3, 1, 7 };
int sz = sizeof(arr) / sizeof(arr[0]);
for (int i = 1; i对如图的数组进行向上 调整法建堆:
第二步:排序
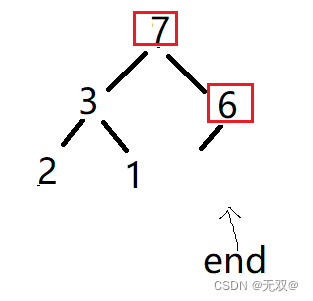
首先我们将首尾元素进行交换:
对除最后一个元素外的其他元素进行向下调整法,将其继续成大堆
重复上述步骤
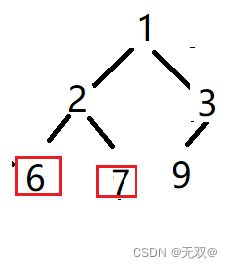
最终可得堆为:
如此则完成了堆排序。
《Top—K问题》
关于Top—k问题:
即求数据结合中前K个最大的元素或者最小的元素,一般情况下数据量都比较大。
比如:专业前10名、世界500强、富豪榜、游戏中前100的活跃玩家等。我们以求n个数据中前K个最大的元素为例进行说明:(假设n=10000) (假设k=10)
#define _CRT_SECURE_NO_WARNINGS 1
#include
#include
#include
const char* file = "data.txt";
void swap(int* a, int* b)
{
int tmp = *a;
*a = *b;
*b = tmp;
}
void AdjustDown(int* arr, int sz, int parent)
{
int child = 2 * parent + 1;
while (child < sz)
{
if (child + 1 < sz && arr[child + 1] < arr[child])
{
child++;
}
if (arr[child] < arr[parent])
{
swap(&arr[child], &arr[parent]);
parent = child;
child = 2 * parent + 1;
}
else
{
break;
}
}
}
void CreateFile()
{
//创建随机数的种子
srand((unsigned int)time(NULL));
FILE* Fin = fopen(file, "w");
if (Fin == NULL)
{
perror("Fopen error");
exit(-1);
}
int n = 10000000;
for (int i = 0; i < n; i++)
{
int x = (rand() + i) % n;
fprintf(Fin, "%d\n", x);
}
fclose(Fin);
Fin = NULL;
}
void Print()
{
FILE* Fout = fopen(file, "r");
if (Fout == NULL)
{
perror("Fout error");
exit(-1);
}
//取前k个数进小堆
int* minheap = (int*)malloc(sizeof(int) * 5);
if (minheap == NULL)
{
perror("minheap -> malloc");
return;
}
for (int i = 0; i < 5; i++)
{
fscanf(Fout, "%d", &minheap[i]);
}
for (int i = (5-1-1)/2; i >=0; --i)
{
AdjustDown(minheap, 5, i);
}
//读取数据
int x = 0;
while (fscanf(Fout, "%d", &x) != EOF)
{
if (minheap[0] < x)
{
minheap[0] = x;
}
AdjustDown(minheap, 5, 0);
}
for (int i = 0; i < 5; i++)
{
printf("%d ", minheap[i]);
}
fclose(Fout);
Fout = NULL;
}
int main()
{
//CreateFile();
Print();
return 0;
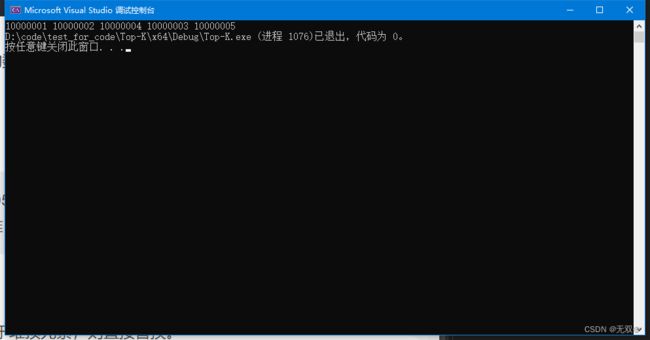
} 首先我们先创建10000000个随机数,再对其中的数字进行修改,随机抽5个数,分别修改为
10000001,10000002,10000003,10000004,10000005
再建一个小堆,注意,这里一定是小堆!
如果建的是大堆,若数据先搜索到了10000005,那么该数字一定是在堆顶,当我们查找到次小的数字后,却无法进堆,所以我们采用小堆!
然后将数据的前5个元素进入小堆中,
再对剩下的9999995个数进行遍历和比较,若大于堆顶元素,则直接替换。
替换完后再进行一次向下调整,当遍历完整个数据后,堆中就是插入的
10000001,10000002,10000003,10000004,10000005