rotate the clock

A program test： 

You are given N round clocks.

Every clock has M hands, and these hands can point to positions 1, 2, 3, ..., P (yes, these represent numbers around each face). The clocks are represented by the matrix A consisting of N rows and M columns of integers. The first row represents the hands of the first clock, and so on.

For example, you are given matrix A consisting of five rows and two columns, and P = 4:

 

  A[0][0] = 1    A[0][1] = 2

  A[1][0] = 2    A[1][1] = 4

  A[2][0] = 4    A[2][1] = 3

  A[3][0] = 2    A[3][1] = 3

  A[4][0] = 1    A[4][1] = 3

 

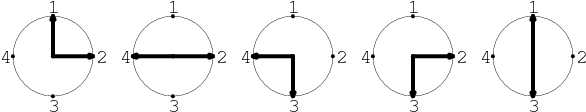
You can rotate the clocks to obtain several clocks that look identical. For example, if you rotate the third, fourth and fifth clocks you can obtain the following clocks:

After rotation, you have four pairs of clocks that look the same: (1, 3), (1, 4), (2, 5) and (3, 4).

type TMatrix = array of array of longint;

Write a function:

int solution(int **A, int N, int M, int P);

int solution(NSMutableArray *A, int P);

int solution(const vector< vector<int> > &A, int P);

class Solution { int solution(int[][] A, int P); }

class Solution { public int solution(int[][] A, int P); }

object Solution { def solution(A: Array[Array[Int]], P: Int): Int }

function solution(A, P);

function solution(A, P)

function solution($A, $P);

function solution(A: TMatrix; N: longint; M: longint; P: longint): longint;

def solution(A, P)

sub solution { my ($A, $P)=@_; my @A=@$A; ... }

def solution(a, p)

Private Function solution ( A As Integer()(), P As Integer ) as Integer

that, given a zero-indexed matrix A consisting of N rows and M columns of integers and integer P, returns the maximal number of pairs of clocks that can look the same after rotation.

For example, given the following array A and P = 4:

    A[0][0] = 1     A[0][1] = 2

    A[1][0] = 2     A[1][1] = 4

    A[2][0] = 4     A[2][1] = 3

    A[3][0] = 2     A[3][1] = 3

    A[4][0] = 1     A[4][1] = 3

the function should return 4, as explained above.

Assume that:

• N is an integer within the range [1..500];
• M is an integer within the range [1..500];
• P is an integer within the range [1..1,000,000,000];
• each element of matrix A is an integer within the range [1..P];
• the elements of each row of matrix A are all distinct.

Complexity:

• expected worst-case time complexity is O(N*M*log(M)+N*log(N));
• expected worst-case space complexity is O(N*M).

 Here is my solution:

 1     class Program

 2     {

 3         static void Main(string[] args)

 4         {

 5             int[][] Testcase = new int[][] { new int[] { 7, 16, 20, 24 }, new int[] { 5, 14, 18, 22 }, 

 6                                             new int[] { 6, 7, 10, 15 }, new int[]{ 6, 7, 10, 15 }, 

 7                                             new int[]{ 3, 7, 11, 18 }, new int[]{ 4, 8, 12, 19 } };

 8             //result should be 7

 9             Console.WriteLine(new Solution().solution(Testcase, 24));

10         }

11     }

12 

13     class Solution

14     {

15         public int solution(int[][] A, int P)

16         {

17             int result = 0;

18             int hands =  A[0].Length;

19 

20             Dictionary<int[], int> buckets = new Dictionary<int[], int>();

21             buckets.Add (A[0],0);

22 

23             //fill the buckets

24             bool flgFind = false;

25             foreach(int[] oneClock in A)

26             {

27                 flgFind = false;

28                 foreach(int[] bucket in buckets.Keys)

29                 {

30                     if (CanbeRotatedToEqual(oneClock, bucket,P) == true)

31                     {

32                         buckets[bucket] += 1;

33                         flgFind = true;

34                         break;

35                     }

36                 }

37                 if(flgFind == false)

38                     buckets.Add(oneClock, 1);

39 

40             }

41 

42             //calculate the total pairs

43             foreach (int k in buckets.Values)

44                 result += k * (k - 1) / 2;

45 

46             return result;

47 

48         }

49 

50         bool CanbeRotatedToEqual(int[] source, int[] target, int P)

51         {

52             bool flgJ = false;

53             int hands = source.Length;

54             for (int i = 0; i < hands; i++)

55             {

56                 int subValue = target[0] - source[i];

57 

58                 flgJ = false;

59                 for (int j = 0; j < hands; j++)

60                 {

61                     int newS = source[(i + j) % hands] + subValue;

62                     if (newS <= 0)

63                         newS += P;

64                     if (newS != target[j])

65                     {

66                         flgJ = true;

67                         break;

68                     }

69                 }

70                 //When flgJ is still false, that means after the source clock rotates subValue steps, 

71                 //it is identical with the target one.

72                 if (flgJ == false)

73                     return true;

74             }

75             //if this loop end normally, that means the source clock cannot rotate to the target one.

76             return false;

77         }

78     }

2013/8/18: This is not valid version.