【POJ 3440】 Coin Toss(概率公式)
【POJ 3440】 Coin Toss(概率公式)
| Time Limit: 5000MS | Memory Limit: 65536K | |
| Total Submissions: 3591 | Accepted: 957 |
Description
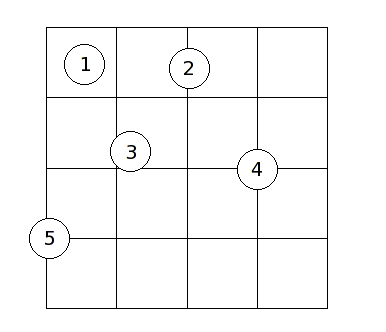
In a popular carnival game, a coin is tossed onto a table with an area that is covered with square tiles in a grid. The prizes are determined by the number of tiles covered by the coin when it comes to rest: the more tiles it covers, the better the prize. In the following diagram, the results from five coin tosses are shown:
In this example:
- coin 1 covers 1 tile
- coin 2 covers 2 tiles
- coin 3 covers 3 tiles
- coin 4 covers 4 tiles
- coin 5 covers 2 tiles
Notice that it is acceptable for a coin to land on the boundary of the playing area (coin 5). In order for a coin to cover a tile, the coin must cover up a positive area of the tile. In other words, it is not enough to simply touch the boundary of the tile. The center of the coin may be at any point of the playing area with uniform probability. You may assume that (1) the coin always comes to a rest lying flat, and (2) the player is good enough to guarantee that the center of the coin will always come to rest on the playing area (or the boundary).
The probability of a coin covering a certain number of tiles depends on the tile and coin sizes, as well as the number of rows and columns of tiles in the playing area. In this problem, you will be required to write a program which computes the probabilities of a coin covering a certain number of tiles.
Input
Output
Separate the output of consecutive cases by a blank line.
Sample Input
3 5 5 10 3 7 4 25 20 10 10 10 4
Sample Output
Case 1: Probability of covering 1 tile = 57.7600% Probability of covering 2 tiles = 36.4800% Probability of covering 3 tiles = 1.2361% Probability of covering 4 tiles = 4.5239% Case 2: Probability of covering 1 tile = 12.5714% Probability of covering 2 tiles = 46.2857% Probability of covering 3 tiles = 8.8293% Probability of covering 4 tiles = 32.3135% Case 3: Probability of covering 1 tile = 40.9600% Probability of covering 2 tiles = 46.0800% Probability of covering 3 tiles = 2.7812% Probability of covering 4 tiles = 10.1788%
题目大意:有n*m块瓷砖,摆成一个大矩形。每块瓷砖是一个t*t的正方形
随意扔一个直径为d的圆盘,问能覆盖1/2/3/4个瓷砖的概率
其实就是问覆盖i个瓷砖情况的圆心可处在的面积和除上总面积
公式嘛……无脑推就好,注意下细节,然后就是加减乘除恶心点,还有记得都用double int*int可能会爆
代码如下:
#include <iostream>
#include <cmath>
#include <vector>
#include <cstdlib>
#include <cstdio>
#include <cstring>
#include <queue>
#include <stack>
#include <list>
#include <algorithm>
#include <map>
#include <set>
#define LL long long
#define Pr pair<int,int>
#define fread() freopen("in.in","r",stdin)
#define fwrite() freopen("out.out","w",stdout)
using namespace std;
const int INF = 0x3f3f3f3f;
const int msz = 10000;
const int mod = 1e9+7;
const double eps = 1e-8;
const double PI = acos(-1.0);
int main()
{
//fread();
//fwrite();
int t;
double n,m,per,d,ans[4],sum;
scanf("%d",&t);
for(int z = 1; z <= t; ++z)
{
scanf("%lf%lf%lf%lf",&n,&m,&per,&d);
if(z != 1) puts("");
printf("Case %d:\n",z);
sum = per*per*n*m;
ans[0] = (per-d)*(per-d)*n*m+(per-d)*(d/2)*(n*2+m*2)+d*d;
ans[0] /= sum;
ans[1] = (per-d)*d*(m*(n-1)+n*(m-1))+d*d/2*((m-1)*2+(n-1)*2);
ans[1] /= sum;
ans[2] = (d*d-PI*d*d/4)*((m-1)*(n-1));
ans[2] /= sum;
ans[3] = PI*d*d/4*((m-1)*(n-1));
ans[3] /= sum;
printf("Probability of covering 1 tile = %.4f%%\n",ans[0]*100);
printf("Probability of covering 2 tiles = %.4f%%\n",ans[1]*100);
printf("Probability of covering 3 tiles = %.4f%%\n",ans[2]*100);
printf("Probability of covering 4 tiles = %.4f%%\n",ans[3]*100);
}
return 0;
}